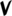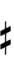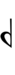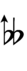27edo

Prime factorization

3³

Step size

44.4444¢

Fifth

16\27 (711.111¢)

Semitones (A1:m2)

4:1 (177.8¢ : 44.44¢)

Consistency limit

9

Distinct consistency limit

7

Special properties

Zeta peak

27 equal divisions of the octave (abbreviated 27edo or 27ed2), also called 27-tone equal temperament (27tet) or 27 equal temperament (27et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 27 equal parts of about 44.4 ¢ each. Each step represents a frequency ratio of 2^1/27, or the 27th root of 2.

Theory

27edo divides the octave in 27 equal parts each exactly 444⁄9 cents in size. However, since the harmonics whose intervals it approximates well (3, 5, 7, 13, and 19) are all tuned sharp of just, 27edo is a prime candidate for octave compression. The local zeta peak around 27 is at 27.086614, which corresponds to a step size of 44.3023 cents. More generally, narrowing the steps to between 44.2 and 44.35 cents would be better in theory; 43edt, 70ed6, and 97ed12 are good options if octave compression is acceptable, and these narrow the octaves by 5.75, 3.53, and 2.55 cents, respectively.

However, assuming just octaves, 27edo's fifth and harmonic seventh are both sharp by nine cents, and the major third is the same 400 cent major third as 12edo, sharp by 13.7 cents. The result is that 6/5, 7/5, and especially 7/6 are all tuned more accurately than this. It can be considered the superpythagorean counterpart of 19edo, as its 5th is audibly indistinguishable from 1/3 septimal comma superpyth in the same way that 19edo is audibly indistinguishable from 1/3 syntonic comma meantone, where three fifths in 19edo reach a near-perfect 6/5 and 5/3 and three fifths in 27edo reaching a near-perfect 7/6 and 12/7.

27edo, with its 400 cent major third, tempers out the lesser diesis, 128/125, and the septimal comma, 64/63, and hence 126/125 as well. These it shares with 12edo, making some relationships familiar, and they both support the augene temperament. It shares with 22edo tempering out the allegedly Bohlen-Pierce comma 245/243 as well as 64/63, so that they both support the superpyth temperament, with four quite sharp "superpythagorean" fifths giving a sharp 9/7 in place of meantone's 5/4.

Though 27edo's 7-limit tuning is not highly accurate, it nonetheless is the smallest equal division to represent the 7-odd-limit both consistently and distinctly—that is, everything in the 7-odd-limit diamond is uniquely represented by a certain number of steps of 27edo. It also represents the 13th harmonic very well, and performs quite decently as a 2.3.5.7.13.19 (no-11s, no-17s 19-limit) temperament. It also approximates 19/10, 19/12, and 19/14, so 0–7–13–25 does quite well as a 10:12:14:19 chord, with the major seventh 25\27 being less than one cent off from 19/10. Octave-inverted, these also form a quite convincing approximation of the main Bohlen–Pierce triads, 3:5:7 and 5:7:9, making 27 the smallest edo that can simulate tritave harmony, although it rapidly becomes rough if extended to the 11 and above, unlike a true tritave based system.

Its step, as well as the octave-inverted and octave-equivalent versions of it, has some of the highest harmonic entropy possible and thus is, in theory, one of the most dissonant intervals possible, assuming the relatively common values of a = 2 and s = 1%. This property is shared with all edos between around 24 and 30. Intervals smaller than this tend to be perceived as unison and are more consonant as a result; intervals larger than this have less "tension" and thus are also more consonant.

Odd harmonics

Approximation of odd harmonics in 27edo
Harmonic		3	5	7	9	11	13	15	17	19	21	23
Error	Absolute (¢)	+9.2	+13.7	+9.0	+18.3	-18.0	+3.9	-21.6	-16.1	+13.6	+18.1	-6.1
Error	Relative (%)	+20.6	+30.8	+20.1	+41.2	-40.5	+8.8	-48.6	-36.1	+30.6	+40.7	-13.6
Steps (reduced)		43 (16)	63 (9)	76 (22)	86 (5)	93 (12)	100 (19)	105 (24)	110 (2)	115 (7)	119 (11)	122 (14)

Intervals

#	Cents	Approximate Ratios^{[note 1]}	Ups and Downs Notation			Walker Brightness Notation	Solfeges
0	0.00	1/1	P1	perfect unison	D	unison	da	do
1	44.44	28/27, 36/35, 39/38, 49/48, 50/49, 81/80	^1, m2	up unison, minor 2nd	^D, Eb	diesis	fra	di
2	88.89	16/15, 21/20, 25/24, 19/18, 20/19	^^1, ^m2	dup unison, upminor 2nd	^^D, ^Eb	minor second	fru	ra
3	133.33	15/14, 14/13, 13/12	vA1, ~2	downaug 1sn, mid 2nd	vD#, vvE	neutral second	ri	ru
4	177.78	10/9	A1, vM2	aug 1sn, downmajor 2nd	D#, vE	small major second	ro	reh
5	222.22	8/7, 9/8	M2	major 2nd	E	large major second	ra	re
6	266.67	7/6	m3	minor 3rd	F	subminor third	na	ma
7	311.11	6/5, 19/16	^m3	upminor 3rd	Gb	minor third	nu	me
8	355.56	16/13	~3	mid 3rd	^Gb	neutral third	mi	mu
9	400.00	5/4, 24/19	vM3	downmajor 3rd	vF#	major third	mo	mi
10	444.44	9/7, 13/10	M3	major 3rd	F#	supermajor third	ma	mo
11	488.89	4/3	P4	perfect 4th	G	fourth	fa	fa
12	533.33	27/20, 48/35, 19/14, 26/19	^4	up 4th	Ab	superfourth	fu/sha	fih
13	577.78	7/5, 18/13	~4, ^d5	mid 4th, updim 5th	^^G, ^Ab	small tritone	fi/shu	fi
14	622.22	10/7, 13/9	vA4, ~5	downaug 4th, mid 5th	vG#, vvA	large tritone	po/si	se
15	666.67	40/27, 35/24, 19/13, 28/19	v5	down fifth	G#	subfifth	pa/so	sih
16	711.11	3/2	P5	perfect 5th	A	fifth	sa	so/sol
17	755.56	14/9, 20/13	m6	minor 6th	Bb	subminor sixth	fla	lo
18	800.00	8/5, 19/12	^m6	upminor 6th	^Bb	minor sixth	flu	le
19	844.44	13/8	~6	mid 6th	vA#	neutral sixth	li	lu
20	888.89	5/3, 32/19	vM6	downmajor 6th	A#	major sixth	lo	la
21	933.33	12/7	M6	major 6th	B	supermajor sixth	la	li
22	977.78	7/4, 16/9	m7	minor 7th	C	harmonic seventh	tha	ta
23	1022.22	9/5	^m7	upminor 7th	Db	large minor seventh	thu	te
24	1066.67	28/15, 13/7, 24/13	~7	mid 7th	^Db	neutral seventh	ti	tu
25	1111.11	15/8, 40/21, 48/25, 19/10, 36/19	vM7	downmajor 7th	vC#	major seventh	to	ti
26	1155.56	27/14, 35/18, 96/49, 49/25, 160/81	M7	major 7th	C#	supermajor seventh	ta	da
27	1200.00	2/1	P8	8ve	D	octave	da	do

Interval quality and chord names in color notation

Combining ups and downs notation with color notation, qualities can be loosely associated with colors:

Quality	Color Name	Monzo Format	Examples
minor	zo	{a, b, 0, 1}	7/6, 7/4
minor	fourthward wa	{a, b}, b < −1	32/27, 16/9
upminor	gu	{a, b, -1}	6/5, 9/5
mid	tho	{a, b, 0, 0, 0, 1}	13/12, 13/8
mid	thu	{a, b, 0, 0, 0, -1}	16/13, 24/13
downmajor	yo	{a, b, 1}	5/4, 5/3
major	fifthward wa	{a, b}, b > 1	9/8, 27/16
major	ru	{a, b, 0, -1}	9/7, 12/7

All 27edo chords can be named using ups and downs. Alterations are always enclosed in parentheses, additions never are. An up or down after the chord root affects the 3rd, 6th, 7th, and/or the 11th (every other note of a stacked-3rds chord 6-1-3-5-7-9-11-13). Here are the zo, gu, ilo, yo and ru triads:

Color of the 3rd	JI Chord	Notes as Edosteps	Notes of C Chord	Written Name	Spoken Name
zo	6:7:9	0–6–16	C–E♭–G	Cm	C minor
gu	10:12:15	0–7–16	C–F♭–G, C–E –G	C^m	C upminor
ilo	18:22:27	0–8–16	C–E –G	C~	C mid
yo	4:5:6	0–9–16	C–D♯–G, C–E –G	Cv	C downmajor or C down
ru	14:18:21	0–10–16	C–E–G	C	C major or C

For a more complete list, see Ups and Downs Notation #Chords and Chord Progressions. See also the 22edo page.

Notation

Circle of fifths in 27edo
Cents	Note from C
Cents	Standard notation		Quarter tone notation
0	C		A
711.11	G		E
222.22	D		B	F
933.33	A		C
444.44	E		G
1155.55	B		D
666.66	F♯		A
177.77	C♯		E
888.88	G♯		B
400	D♯		F
1111.11	A♯		C
622.22	E♯		G
133.33	B♯	F𝄫	D
844.44	F𝄪	C𝄫	A
355.56	C𝄪	G𝄫	E
1066.67	G𝄪	D𝄫	B
577.78	D𝄪	A𝄫	F
88.89	A𝄪	E𝄫	C
800	E𝄪	B𝄫	G
311.11	B𝄪	F♭	D
1022.22	C♭		A
533.33	G♭		E
44.44	D♭		B
755.56	A♭		F
266.67	E♭		C
977.78	B♭		G
488.89	F		D
0	C		A

The 27-note system can be notated using ups and downs notation, in which case arrows or Helmholtz–Ellis accidentals can be used, or with a variation on quarter tone accidentals. With standard circle-of-fifths notation, a sharp raises a note by 4 steps, just one step beneath the following nominal (for example C to C♯ describes the approximate 10/9 and 11/10 interval) and the flat conversely lowers: these are augmented unisons and diminished unisons. Just so, one finds that an accidental can be divided in half, and the remaining places can then be filled in with half-sharps, half-flats, sesquisharps, and sesquiflats, reducing the need for double sharps and double flats.The notes from C to D are C, D♭, C , D , C♯, and D, with some ascending intervals appearing to be descending on the staff.

Another notational implication is that, being a Superpythagorean system, the 5/4 major third present in the 4:5:6 chord is technically an augmented second, since (for example) C–E is a 9/7 supermajor third and so the note located one major third above C must be notated as D♯ or E . Conversely, the 6/5 minor third of a 10:12:15 chord is actually reached by a diminished fourth, since (for example) D–F is a 7/6 subminor third and so the note located one minor third above D must be notated as either G♭ or F . The composer can decide for themselves which additional accidental pair is appropriate if they will need redundancy to remedy these problems, and to keep the chromatic pitches within a compass on paper relative to the natural names (C, D, E etc.). Otherwise it is simple enough, and the same tendency for A♯ to be higher than B♭ is not only familiar, though here very exaggerated, to those working with the Pythagorean scale (see 53edo), but also to many classically trained violinists.

Step Offset	0	1	2	3	4	5	6	7	8	9
Sharp Symbol
Flat Symbol

6L 1s (archeotonic) notation

The notation of Tetracot[7]. Notes are denoted as LLLLLLs = CDEFGABC, and raising and lowering by a chroma (L − s), 1 step in this instance, is denoted by ♯ and ♭.

#	Cents	Note	Name	Associated ratio
0	0.0	C	perfect unison	1/1
1	44.4	C#, Dbbb	aug 1sn, double-dim 2nd	40/39, 45/44, 55/54, 81/80
2	88.9	Cx, Dbb	double-aug 1sn, dim 2nd	16/15, 25/24
3	133.3	Db	minor 2nd	12/11, 13/12
4	177.8	D	major 2nd	10/9, 11/10
5	222.2	D#, Ebbb	aug 2nd, double-dim 3rd	9/8
6	266.7	Dx, Ebb	double-aug 2nd, dim 3rd	15/13
7	311.1	Eb	minor 3rd	6/5
8	355.6	E	major 3rd	11/9, 16/13
9	400.0	E#, Fbbb	aug 3rd, double-dim 4th	5/4
10	444.4	Ex, Fbb	double-aug 3rd, dim 4th	13/10
11	488.9	Ex#, Fb	minor 4th	4/3
12	533.3	F	major 4th	15/11, 27/20
13	577.8	F#, Gbbb	aug 4th, double-dim 5th	11/8, 18/13
14	622.2	Fx, Gbb	double-aug 4th, dim 5th	13/9, 16/11
15	666.7	Fx#, Gb	minor 5th	22/15, 40/27
16	711.1	G	major 5th	3/2
17	755.6	G#, Abbb	aug 5th, double-dim 6th	20/13
18	800.0	Gx, Abb	double-aug 5th, dim 6th	8/5
19	844.4	Ab	minor 6th	13/8, 18/11
20	888.9	A	major 6th	5/3
21	933.3	A#, Bbbb	aug 6th, double-dim 7th	26/15
22	977.8	Ax, Bbb	double-aug 6th, dim 7th	16/9
23	1022.2	Bb	minor 7th	9/5, 20/11
24	1066.7	B	major 7th	11/6, 24/13
25	1111.1	B#, Cbb	aug 7th, double-dim 8ve	15/8, 48/25
26	1155.6	Bx, Cb	double-aug 7th, dim 8ve	39/20, 88/45, 108/55, 160/81
27	1200.0	C	8ve	2/1

Approximation to JI

Selected 19-limit intervals approximated in 27edo

Interval mappings

The following tables show how 15-odd-limit intervals are represented in 27edo. Prime harmonics are in bold; inconsistent intervals are in italics.

15-odd-limit intervals in 27edo (direct approximation, even if inconsistent)
Interval and complement	Error (abs, ¢)	Error (rel, %)
1/1, 2/1	0.000	0.0
7/6, 12/7	0.204	0.5
15/11, 22/15	3.617	8.1
13/8, 16/13	3.917	8.8
5/3, 6/5	4.530	10.2
9/5, 10/9	4.626	10.4
7/5, 10/7	4.734	10.7
13/7, 14/13	5.035	11.3
13/12, 24/13	5.239	11.8
11/9, 18/11	8.148	18.3
7/4, 8/7	8.952	20.1
3/2, 4/3	9.156	20.6
9/7, 14/9	9.360	21.1
13/10, 20/13	9.770	22.0
11/10, 20/11	12.774	28.7
5/4, 8/5	13.686	30.8
15/14, 28/15	13.891	31.3
13/9, 18/13	14.395	32.4
11/6, 12/11	17.304	38.9
11/7, 14/11	17.508	39.4
11/8, 16/11	17.985	40.5
9/8, 16/9	18.312	41.2
15/13, 26/15	18.926	42.6
15/8, 16/15	21.602	48.6
13/11, 22/13	21.901	49.3

15-odd-limit intervals in 27edo (patent val mapping)
Interval and complement	Error (abs, ¢)	Error (rel, %)
1/1, 2/1	0.000	0.0
7/6, 12/7	0.204	0.5
13/8, 16/13	3.917	8.8
5/3, 6/5	4.530	10.2
9/5, 10/9	4.626	10.4
7/5, 10/7	4.734	10.7
13/7, 14/13	5.035	11.3
13/12, 24/13	5.239	11.8
7/4, 8/7	8.952	20.1
3/2, 4/3	9.156	20.6
9/7, 14/9	9.360	21.1
13/10, 20/13	9.770	22.0
5/4, 8/5	13.686	30.8
15/14, 28/15	13.891	31.3
13/9, 18/13	14.395	32.4
11/8, 16/11	17.985	40.5
9/8, 16/9	18.312	41.2
15/13, 26/15	18.926	42.6
13/11, 22/13	21.901	49.3
15/8, 16/15	22.842	51.4
11/7, 14/11	26.936	60.6
11/6, 12/11	27.141	61.1
11/10, 20/11	31.671	71.3
11/9, 18/11	36.297	81.7
15/11, 22/15	40.827	91.9

15-odd-limit intervals by 27e val mapping
Interval and complement	Error (abs, ¢)	Error (rel, %)
1/1, 2/1	0.000	0.0
7/6, 12/7	0.204	0.5
15/11, 22/15	3.617	8.1
13/8, 16/13	3.917	8.8
5/3, 6/5	4.530	10.2
9/5, 10/9	4.626	10.4
7/5, 10/7	4.734	10.7
13/7, 14/13	5.035	11.3
13/12, 24/13	5.239	11.8
11/9, 18/11	8.148	18.3
7/4, 8/7	8.952	20.1
3/2, 4/3	9.156	20.6
9/7, 14/9	9.360	21.1
13/10, 20/13	9.770	22.0
11/10, 20/11	12.774	28.7
5/4, 8/5	13.686	30.8
15/14, 28/15	13.891	31.3
13/9, 18/13	14.395	32.4
11/6, 12/11	17.304	38.9
11/7, 14/11	17.508	39.4
9/8, 16/9	18.312	41.2
15/13, 26/15	18.926	42.6
13/11, 22/13	22.543	50.7
15/8, 16/15	22.842	51.4
11/8, 16/11	26.460	59.5

Regular temperament properties

Subgroup	Comma list	Mapping	Optimal 8ve stretch (¢)	Tuning error
Subgroup	Comma list	Mapping	Optimal 8ve stretch (¢)	Absolute (¢)	Relative (%)
2.3	[43 -27⟩	[⟨27 43]]	−2.89	2.88	6.50
2.3.5	128/125, 20000/19683	[⟨27 43 63]]	−3.88	2.74	6.19
2.3.5.7	64/63, 126/125, 245/243	[⟨27 43 63 76]]	−3.70	2.39	5.40
2.3.5.7.13	64/63, 91/90, 126/125, 169/168	[⟨27 43 63 76 100]]	−3.18	2.39	5.39
2.3.5.7.13.19	64/63, 76/75, 91/90, 126/125, 169/168	[⟨27 43 63 76 100 115]]	−3.18	2.18	4.92

27et (27eg val) is lower in relative error than any previous equal temperaments in the 13-, 17-, and 19-limit. The next equal temperaments doing better in those subgroups are 31, 31, and 46, respectively.
27et is particularly strong in the 2.3.5.7.13.19 subgroup. The next equal temperament that does better in this subgroup is 53.

Rank-2 temperaments

Periods per 8ve	Generator	Temperaments	MOS scales
1	1\27	Quartonic / quarto (27e) / quartz (27)
1	2\27	Octacot / octocat (27e)	1L 12s, 13L 1s
1	4\27	Tetracot (27e) / modus (27e) / wollemia (27e)	1L 5s, 6L 1s, 7L 6s, 7L 13s
1	5\27	Machine (27) Kumonga (27e)	1L 4s, 5L 1s, 5L 6s, 11L 5s
1	7\27	Myna (27e) / coleto (27e) / myno (27) Oolong (27e)	4L 3s, 4L 7s, 4L 11s, 4L 15s, 4L 19s
1	8\27	Beatles (27e) / ringo (27e) / beetle (27)	3L 4s, 7L 3s, 10L 7s
1	10\27	Sensi	3L 2s, 3L 5s, 8L 3s, 8L 11s
1	11\27	Superpyth (27e)	5L 2s, 5L 7s, 5L 12s, 5L 17s
1	13\27	Fervor (27e)	2L 3s, 2L 5s, 2L 7s, 2L 9s, 2L 11s, etc. … 2L 23s
3	1\27	Hemiaug (27e)
3	2\27	Augene (27e) / Eugene (27)	3L 3s, 3L 6s, 3L 9s, 12L 3s
3	4\27	Oodako (27e) Terrain	3L 3s, 6L 3s, 6L 9s, 6L 15s
9	1\27	Niner (27e) Ennealimmal (out of tune)	9L 9s

Commas

27edo tempers out the following commas. (Note: This assumes the patent val, ⟨27 43 63 76 93 100].)

Prime limit	Ratio^{[note 2]}	Monzo	Cents	Color name	Name
5	128/125	[7 0 -3⟩	41.06	Trigu	Augmented comma, lesser diesis
5	20000/19683	[5 -9 4⟩	27.66	Saquadyo	Tetracot comma, minimal diesis
5	78732/78125	[2 9 -7⟩	13.40	Sepgu	Sensipent comma
5	(26 digits)	[1 -27 18⟩	0.86	Satritribiyo	Ennealimma
7	686/675	[1 -3 -2 3⟩	27.99	Trizo-agugu	Senga
7	64/63	[6 -2 0 -1⟩	27.26	Ru	Septimal comma
7	50421/50000	[-4 1 -5 5⟩	14.52	Quinzogu	Trimyna comma
7	245/243	[0 -5 1 2⟩	14.19	Zozoyo	Sensamagic comma
7	126/125	[1 2 -3 1⟩	13.79	Zotrigu	Starling comma
7	4000/3969	[5 -4 3 -2⟩	13.47	Rurutriyo	Octagar comma
7	1728/1715	[6 3 -1 -3⟩	13.07	Triru-agu	Orwellisma
7	(16 digits)	[-11 -9 0 9⟩	1.84	Tritrizo	Septimal ennealimma
7	(12 digits)	[-6 -8 2 5⟩	1.12	Quinzo-ayoyo	Wizma
7	2401/2400	[-5 -1 -2 4⟩	0.72	Bizozogu	Breedsma
7	4375/4374	[-1 -7 4 1⟩	0.40	Zoquadyo	Ragisma
7	(12 digits)	[-4 6 -6 3⟩	0.33	Trizogugu	Landscape comma
11	55/54	[-1 -3 1 0 1⟩	31.77	Loyo	Telepathma, undecimal diasecundal comma
11	99/98	[-1 2 0 -2 1⟩	17.58	Loruru	Mothwellsma
11	896/891	[7 -4 0 1 -1⟩	9.69	Saluzo	Pentacircle comma
11	385/384	[-7 -1 1 1 1⟩	4.50	Lozoyo	Keenanisma
13	66/65	[1 1 -1 0 1 -1⟩	26.43	Thulogu	Winmeanma
13	91/90	[-1 -2 -1 1 0 1⟩	19.13	Thozogu	Superleap comma, biome comma
13	512/507	[9 -1 0 0 0 -2⟩	16.99	Thuthu	Tridecimal neutral thirds comma
13	325/324	[-2 -4 2 0 0 1⟩	5.34	Thoyoyo	Marveltwin comma
13	351/350	[-1 3 -2 -1 0 1⟩	4.94	Thorugugu	Ratwolfsma
13	31213/31104	[-7 -5 0 4 0 1⟩	6.06	Thoquadzo	Praveensma
17	85/84	[-2 -1 1 -1 0 0 1⟩	20.49	Soruyo	Monk comma
17	154/153	[1 -2 0 1 1 0 -1⟩	11.28	Sulozo	Augustma
19	77/76	[2 -1 -2 0 0 0 0 1⟩	22.63	Nulozo	Small undevicesimal ninth tone
19	96/95	[5 1 -1 0 0 0 0 -1⟩	18.13	Nugu	19th-partial chroma

Scales

MOS scales

Main article: List of MOS scales in 27edo

Superpyth pentatonic – Superpyth[5] 2L 3s (gen = 11\27): 5 5 6 5 6
Superpyh diatonic – Superpyth[7] 5L 2s (gen = 11\27): 5 5 1 5 5 5 1
Superpyth chromatic – Superpyth[12] 5L 7s (gen = 11\27): 4 1 1 4 1 4 1 4 1 1 4 1
Superpyth hyperchromatic – Superpyth[17] 5L 12s (gen = 11\27): 1 3 1 1 3 1 1 1 3 1 1 3 1 1 3 1 1
Augene[6] 3L 3s (period = 9\27, gen = 2\27): 7 2 7 2 7 2
Augene[9] 3L 6s (period = 9\27, gen = 2\27): 5 2 2 5 2 2 5 2 2
Augene[12] 3L 9s (period = 9\27, gen = 2\27): 3 2 2 2 3 2 2 2 3 2 2 2
Augene[15] 12L 3s (period = 9\27, gen = 2\27): 1 2 2 2 2 1 2 2 2 2 1 2 2 2 2
Beatles[7] 3L 4s (gen = 8\27): 3 5 3 5 3 5 3
Beatles[10] 7L 3s (gen = 8\27): 3 3 2 3 3 2 3 3 2 3
Beatles[17] 10L 7s (gen = 8\27): 2 1 2 1 2 2 1 2 1 2 2 1 2 1 2 2 1
Sensi[5] 3L 2s (gen = 10\27): 7 3 7 3 7
Sensi[8] 3L 5s (gen = 10\27): 3 4 3 3 4 3 3 4
Sensi[11] 8L 3s (gen = 10\27): 3 3 1 3 3 3 1 3 3 3 1
Machine[5] 1L 4s (gen = 5\27): 5 5 5 5 7
Machine[6] 5L 1s (gen = 5\27): 5 5 5 5 5 2
Machine[11] 5L 6s (gen = 5\27): 2 3 2 3 2 3 2 3 2 3 2
Machine[16] 11L 5s (gen = 5\27): 2 2 1 2 2 1 2 2 1 2 2 1 2 2 1 2
Tetracot[6] 1L 5s (gen = 4\27): 4 4 4 4 4 7
Tetracot[7] 6L 1s (gen = 4\27): 4 4 4 4 4 4 3
Tetracot[13] 7L 6s (gen = 4\27): 3 1 3 1 3 1 3 1 3 1 3 1 3
Tetracot[20] 7L 13s (gen = 4\27): 2 1 1 2 1 1 2 1 1 2 1 1 2 1 1 2 1 1 2 1
Octacot[13] 1L 12s (gen = 2\27): 2 2 2 2 2 2 2 2 2 2 2 2 3
Octacot[14] 13L 1s (gen = 2\27): 2 2 2 2 2 2 2 2 2 2 2 2 2 1
Myna[7] 4L 3s (gen = 7\27): 6 1 6 1 6 1 6
Myna[11] 4L 7s (gen = 7\27): 5 1 1 5 1 1 5 1 1 5 1
Myna[15] 4L 11s (gen = 7\27): 4 1 1 1 4 1 1 1 4 1 1 1 4 1 1
Myna[19] 4L 15s (gen = 7\27): 3 1 1 1 1 3 1 1 1 1 3 1 1 1 1 3 1 1 1

Other scales

5-limit / pental / Pinetone major pentatonic: 5 4 7 4 7
5-limit / pental / Pinetone minor pentatonic: 7 4 5 7 4
enharmonic trichord octave species: 9 2 5 9 2, 2 9 5 2 9
5-limit / pental double harmonic hexatonic (Augmented[6] 4M): 2 7 2 7 7 2, 7 7 2 2 7 2
Superpyth melodic minor – Superpyth 2|4 #6 #7 or 5|1 b3: 5 1 5 5 5 5 1
Superpyth harmonic minor – Superpyth 2|4 #7: 5 1 5 5 1 9 1
Superpyth harmonic major – Superpyth 5|1 b6: 5 5 1 5 1 9 1
Superpyth double harmonic major – Superpyth 5|1 b2 b6: 1 9 1 5 1 9 1
Zarlino / Ptolemy diatonic, "just" major: 5 4 2 5 4 5 2
"Just" minor (inverse of "just" major): 5 2 4 5 2 5 4
5-limit / pental tetrachordal major: 5 4 2 5 5 4 2
5-limit / pental tetrachordal minor: 5 2 4 5 5 2 4
5-limit / pental melodic minor: 5 2 4 5 4 5 2
5-limit / pental harmonic minor: 5 2 4 5 2 7 2
5-limit / pental harmonic major: 5 4 2 5 2 7 2
5-odd limit tonality diamond: 7 2 2 5 2 2 7
7-odd limit tonality diamond: 5 1 1 2 2 2 1 2 2 2 1 1 5
9-odd limit tonality diamond: 4 1 1 1 2 1 1 2 1 2 1 1 2 1 1 1 4
5-limit / pental double harmonic major: 2 7 2 5 2 7 2
enharmonic tetrachord octave species: 9 1 1 5 9 1 1, 1 9 1 5 1 9 1 (also Superpyth double harmonic major), 1 1 9 5 1 1 9
Pinetone diatonic: 4 3 4 5 4 3 4
Pinetone major-harmonic octatonic: 4 3 4 2 3 4 3 4
Pinetone minor-harmonic octatonic: 4 3 2 4 3 4 4 3
Pinetone diminished octatonic / Porcusmine: 3 4 2 4 3 4 3 4
Pinetone harmonic diminished: 3 4 2 5 2 4 3 4
Pinetone chromatic / pinechrome: 1 3 3 1 3 2 3 1 3 3 1 3
5-limit / pental double harmonic nonatonic (subset of Augene[12]): 2 5 2 2 5 2 5 2 2, 2 2 5 2 5 2 2 5 2 (Augene[9] 4M)
5-limit / pental double harmonic decatonic (subset of Augene[12]): 2 5 2 2 3 2 2 5 2 2
5-limit / pental double harmonic chromatic: 2 2 3 2 2 3 2 2 2 3 2 2, 2 2 3 2 2 2 3 2 2 3 2 2 (Augene[12] 4M)
Blackdye / syntonic dipentatonic (superset of Zarlino): 1 4 2 4 1 4 2 4 1 4
Blackville / 5-limit dipentatonic (superset of Zarlino): 3 2 4 2 3 2 4 2 3 2

Direct sunlight (this is its original/default tuning; subset of Sensi[19])

44.444
133.333
488.889
711.111
755.555
1155.555
1200.000

Hypersakura (this is its original/default tuning; subset of Sensi[19])

44.444
488.889
711.111
755.555
1200.000

Instruments

Brendan Byrnes, guitarist

While playing 27edo instruments requires significantly more frets or keys than 12edo, it is still possible to create physical instruments that can play all its notes. Probably the most notable of these is owned by Brendan Byrnes and played on some of his tracks listed in the music section.

However, the frets are very close together and playing high up the neck requires careful use of fingernails for fretting. A skip-fretted guitar would have notes only slightly closer together than 12edo and be easier to play, particularly when tuned in the configuration detailed below.

Skip fretting system 27 2 9

27edo can also be played on the Lumatone, with various layouts discussed here.

Lumatone mapping for 27edo

Music

See also: Category:27edo tracks

Abnormality

Boiling (2024)

Nae Ayy

What Happens Next (2021)

Beheld

Thick vibe (2023)

Gregoire Blanc

A microtonal teatime jam (2023)

Brendan Byrnes

Sunspots (2022)

Bryan Deister

microtonal improvisation in 27edo (2023)

Igliashon Jones

Sad Like Winter Leaves – in Augene[12] tuned to 27edo
Superpythagorean Waltz (2012)
Stuttering Anticipation (2021)

Peter Kosmorsky

miniature prelude and fugue (2011)

Claudi Meneghin

Chorale in 27edo for Organ (2019)

NullPointerException Music

Edolian - Adventure (2020)

Dustin Schallert

Tetracot Perc-Sitar ^{[dead link]} (on SoundCloud) ^{[dead link]}
Tetracot Jam ^{[dead link]} (on SoundCloud) ^{[dead link]}
Tetracot Pump ^{[dead link]} (on SoundCloud) ^{[dead link]}
27-EDO Guitar 1 ^{[dead link]}

Gene Ward Smith

Music For Your Ears play – the central portion is in 27edo, the rest in 46edo.

Joel Taylor

Galticeran Sonatina – in Augene[12] tuned to 27edo

Tristan Bay

Pitchblende (2023)

Chris Vaisvil

Chicago Pile-1 (2011)

Xotla

"Funkrotonal" from Microtonal Allsorts (2023) – Spotify | Bandcamp | YouTube

Notes

↑ Based on treating 2.3.5.7.13.19 subgroup as a 5-limit temperament; other approaches are also possible.
↑ Ratios longer than 10 digits are presented by placeholders with informative hints.

[1] Based on treating 2.3.5.7.13.19 subgroup as a 5-limit temperament; other approaches are also possible.

[2] Ratios longer than 10 digits are presented by placeholders with informative hints.

[note 1]

[note 2]