53edo
| ← 52edo | 53edo | 54edo → |
(convergent)
53 equal divisions of the octave (abbreviated 53edo or 53ed2), also called 53-tone equal temperament (53tet) or 53 equal temperament (53et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 53 equal parts of about 22.6 ¢ each. Each step represents a frequency ratio of 21/53, or the 53rd root of 2.
Theory
53edo is notable as an excellent 5-limit system, a fact apparently first noted by Isaac Newton[1]. It is the seventh strict zeta edo. In the opinion of some, 53edo is the first equal division to deal adequately with the 13-limit, while others award that distinction to 41edo or 46edo. Like 41 and 46, 53 is distinctly consistent in the 9-odd-limit (and if we exclude the most damaged interval pair, 7/5 and 10/7, is consistent to distance 2), but among them, 53 is the first that finds the interseptimal intervals 15/13 and 13/10 distinctly from adjacent septimal intervals 8/7 and 7/6, and 9/7 and 21/16, respectively, which is essential to its 13-limit credibility. It also avoids equating 11/9 with 16/13, so that the former is tuned very flat to equate it with a slightly flat ~39/32 – a feature shared by 46edo. It is almost consistent to the entire 15-odd-limit, with the only inconsistency occurring at 14/11 (and its octave complement), which is mapped inconsistently sharp and equated with 9/7, but it has the benefit of doing very well in larger prime/subgroup-limited odd-limits. It can be treated as a no-11's, no-17's tuning, on which it is consistent all the way up to the 27-odd-limit.
As an equal temperament, it notably tempers out Mercator's comma (353/284), the schisma (32805/32768), kleisma (15625/15552), and amity comma (1600000/1594323). In the 7-limit it tempers out the marvel comma (225/224) for which it is a relatively efficient tuning, orwellisma (1728/1715), gariboh comma (3125/3087), and ragisma (4375/4374). In the 11-limit, it tempers out 99/98 and 121/120 (in addition to their difference, 540/539), and is the optimal patent val for big brother temperament, which tempers out both, as well as 11-limit orwell temperament, which also tempers out the 11-limit commas 176/175 and 385/384. In the 13-limit, it tempers out 169/168, 275/273, 325/324, 625/624, 676/675, 1001/1000, 2080/2079, and 4096/4095, and gives the optimal patent val for athene temperament.
53edo has also found a certain dissemination as an edo tuning for Arabic, Turkish, and Persian music. It can also be used as an extended Pythagorean tuning, since its fifths are almost indistinguishable from just.
53edo's step is sometimes called the "Holdrian comma", despite the 53rd root of 2 being an irrational number; the step's role as a "comma" comes from it being an approximation of the Pythagorean comma and syntonic comma.
Prime harmonics
| Harmonic | 2 | 3 | 5 | 7 | 11 | 13 | 17 | 19 | 23 | |
|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +0.00 | -0.07 | -1.41 | +4.76 | -7.92 | -2.79 | +8.25 | -3.17 | +5.69 |
| Relative (%) | +0.0 | -0.3 | -6.2 | +21.0 | -35.0 | -12.3 | +36.4 | -14.0 | +25.1 | |
| Steps (reduced) |
53 (0) |
84 (31) |
123 (17) |
149 (43) |
183 (24) |
196 (37) |
217 (5) |
225 (13) |
240 (28) | |
| Harmonic | 29 | 31 | 37 | 41 | 43 | 47 | 53 | 59 | 61 | 67 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -10.71 | +9.68 | -2.29 | +1.13 | +9.24 | -8.90 | +9.51 | +4.98 | -7.45 | +11.26 |
| Relative (%) | -47.3 | +42.8 | -10.1 | +5.0 | +40.8 | -39.3 | +42.0 | +22.0 | -32.9 | +49.7 | |
| Steps (reduced) |
257 (45) |
263 (51) |
276 (11) |
284 (19) |
288 (23) |
294 (29) |
304 (39) |
312 (47) |
314 (49) |
322 (4) | |
See #Approximation to JI for details and a more in-depth discussion.
Subsets and supersets
53edo is the 16th prime edo, following 47edo and coming before 59edo.
Many of its multiples such as 159edo, 212edo, 742edo, 901edo and 954edo have good consistency limits and are each of their own interest. The mercator family comprises rank-2 temperaments with 1/53-octave periods.
Intervals
| # | Cents | Approximate ratios[note 1] | Ups and downs notation (EUs: v5A1 and ^d2) | Solfeges | |||
|---|---|---|---|---|---|---|---|
| 0 | 0.0 | 1/1 | P1 | unison | D | Da | Do |
| 1 | 22.6 | 50/49, 64/63, 81/80 | ^1 | up unison | ^D | Du | Di |
| 2 | 45.3 | 33/32, 36/35, 49/48, 128/125 | ^^1, vvm2 | dup unison, dudminor 2nd | ^^D, vvEb | Di / Fre | Daw |
| 3 | 67.9 | 22/21, 25/24, 26/25, 27/26, 28/27 | vvA1, vm2 | dudaug 1sn, downminor 2nd | vvD#, vEb | Fro | Ro |
| 4 | 90.6 | 19/18, 20/19, 21/20, 256/243 | vA1, m2 | downaug 1sn, minor 2nd | vD#, Eb | Fra | Rih |
| 5 | 113.2 | 15/14, 16/15 | A1, ^m2 | aug 1sn, upminor 2nd | D#, ^Eb | Fru | Ra |
| 6 | 135.8 | 13/12, 14/13, 27/25 | ^^m2 | dupminor 2nd | ^^Eb | Fri | Ru |
| 7 | 158.5 | 11/10, 12/11, 35/32, 57/52, 800/729 | vvM2 | dudmajor 2nd | vvE | Re | Ruh |
| 8 | 181.1 | 10/9 | vM2 | downmajor 2nd | vE | Ro | Reh |
| 9 | 203.8 | 9/8 | M2 | major 2nd | E | Ra | Re |
| 10 | 226.4 | 8/7, 256/225 | ^M2 | upmajor 2nd | ^E | Ru | Ri |
| 11 | 249.1 | 15/13, 22/19, 125/108, 144/125 | ^^M2, vvm3 | dupmajor 2nd, dudminor 3rd | ^^E, vvF | Ri / Ne | Raw |
| 12 | 271.7 | 7/6, 75/64 | vm3 | downminor 3rd | vF | No | Ma |
| 13 | 294.3 | 13/11, 19/16, 32/27 | m3 | minor 3rd | F | Na | Meh |
| 14 | 317.0 | 6/5 | ^m3 | upminor 3rd | ^F | Nu | Me |
| 15 | 339.6 | 11/9, 243/200 | ^^m3 | dupminor 3rd | ^^F | Ni | Mu |
| 16 | 362.3 | 16/13, 100/81 | vvM3 | dudmajor 3rd | vvF# | Me | Muh |
| 17 | 384.9 | 5/4 | vM3 | downmajor 3rd | vF# | Mo | Mi |
| 18 | 407.5 | 19/15, 24/19, 81/64 | M3 | major 3rd | F# | Ma | Maa |
| 19 | 430.2 | 9/7, 14/11 | ^M3 | upmajor 3rd | ^F# | Mu | Mo |
| 20 | 452.8 | 13/10, 125/96, 162/125 | ^^M3, vv4 | dupmajor 3rd, dud 4th | ^^F#, vvG | Mi / Fe | Maw |
| 21 | 475.5 | 21/16, 25/19, 320/243, 675/512 | v4 | down 4th | vG | Fo | Fe |
| 22 | 498.1 | 4/3 | P4 | perfect 4th | G | Fa | Fa |
| 23 | 520.8 | 19/14, 27/20 | ^4 | up 4th | ^G | Fu | Fih |
| 24 | 543.4 | 11/8, 15/11, 26/19 | ^^4 | dup 4th | ^^G | Fi / She | Fu |
| 25 | 566.0 | 18/13 | vvA4, vd5 | dudaug 4th, downdim 5th | vvG#, vAb | Pe / Sho | Fuh |
| 26 | 588.7 | 7/5, 45/32 | vA4, d5 | downaug 4th, dim 5th | vG#, Ab | Po / Sha | Fi |
| 27 | 611.3 | 10/7, 64/45 | A4, ^d5 | aug 4th, updim 5th | G#, ^Ab | Pa / Shu | Se |
| 28 | 634.0 | 13/9 | ^A4, ^^d5 | upaug 4th, dupdim 5th | ^G#, ^^Ab | Pu / Shi | Suh |
| 29 | 656.6 | 16/11, 19/13, 22/15 | vv5 | dud 5th | vvA | Pi / Se | Su |
| 30 | 679.2 | 28/19, 40/27 | v5 | down 5th | vA | So | Sih |
| 31 | 701.9 | 3/2 | P5 | perfect 5th | A | Sa | Sol |
| 32 | 724.5 | 32/21, 38/25, 243/160, 1024/675 | ^5 | up 5th | ^A | Su | Si |
| 33 | 747.2 | 20/13, 125/81, 192/125 | ^^5, vvm6 | dup 5th, dudminor 6th | ^^A, vvBb | Si / Fle | Saw |
| 34 | 769.8 | 11/7, 14/9, 25/16 | vm6 | downminor 6th | vBb | Flo | Lo |
| 35 | 792.5 | 19/12, 30/19, 128/81 | m6 | minor 6th | Bb | Fla | Leh |
| 36 | 815.1 | 8/5 | ^m6 | upminor 6th | ^Bb | Flu | Le |
| 37 | 837.7 | 13/8, 81/50 | ^^m6 | dupminor 6th | ^^Bb | Fli | Lu |
| 38 | 860.4 | 18/11, 400/243 | vvM6 | dudmajor 6th | vvB | Le | Luh |
| 39 | 883.0 | 5/3 | vM6 | downmajor 6th | vB | Lo | La |
| 40 | 905.7 | 22/13, 27/16, 32/19 | M6 | major 6th | B | La | Laa |
| 41 | 928.3 | 12/7 | ^M6 | upmajor 6th | ^B | Lu | Li |
| 42 | 950.9 | 19/11, 26/15, 125/72, 216/125 | ^^M6, vvm7 | dupmajor 6th, dudminor 7th | ^^B, vvC | Li / The | Law |
| 43 | 973.6 | 7/4 | vm7 | downminor 7th | vC | Tho | Ta |
| 44 | 996.2 | 16/9 | m7 | minor 7th | C | Tha | Teh |
| 45 | 1018.9 | 9/5 | ^m7 | upminor 7th | ^C | Thu | Te |
| 46 | 1041.5 | 11/6, 20/11, 64/35, 729/400 | ^^m7 | dupminor 7th | ^^C | Thi | Tu |
| 47 | 1064.2 | 13/7, 24/13, 50/27 | vvM7 | dudmajor 7th | vvC# | Te | Tuh |
| 48 | 1086.8 | 15/8 | vM7 | downmajor 7th | vC# | To | Ti |
| 49 | 1109.4 | 19/10, 36/19, 40/21, 243/128 | M7 | major 7th | C# | Ta | Tih |
| 50 | 1132.1 | 21/11, 25/13, 27/14, 52/27, 48/25 | ^M7 | upmajor 7th | ^C# | Tu | To |
| 51 | 1154.7 | 35/18, 64/33, 96/49, 125/64 | ^^M7, vv8 | dupmajor 7th, dud 8ve | ^^C#, vvD | Ti / De | Taw |
| 52 | 1177.4 | 49/25, 63/32, 160/81 | v8 | down 8ve | vD | Do | Da |
| 53 | 1200.0 | 2/1 | P8 | perfect 8ve | D | Da | Do |
Interval quality and chord names in color notation
Combining ups and downs notation with color notation, qualities can be loosely associated with colors:
| Quality | Color | Monzo format | Examples |
|---|---|---|---|
| downminor | zo | (a, b, 0, 1) | 7/6, 7/4 |
| minor | fourthward wa | (a, b) with b < −1 | 32/27, 16/9 |
| upminor | gu | (a, b, −1) | 6/5, 9/5 |
| dupminor | ilo | (a, b, 0, 0, 1) | 11/9, 11/6 |
| dudmajor | lu | (a, b, 0, 0, −1) | 12/11, 18/11 |
| downmajor | yo | (a, b, 1) | 5/4, 5/3 |
| major | fifthward wa | (a, b) with b > 1 | 9/8, 27/16 |
| upmajor | ru | (a, b, 0, −1) | 9/7, 12/7 |
All 53edo chords can be named using ups and downs. An up, down or mid after the chord root affects the 3rd, 6th, 7th, and/or the 11th (every other note of a stacked−3rds chord 6 – 1 – 3 – 5 – 7 – 9 – 11 – 13). Alterations are always enclosed in parentheses, additions never are.
Here are the zo, gu, ilo, lu, yo and ru triads:
| Color of the 3rd | JI chord | Notes as edosteps | Notes of C chord | Written name | Spoken name |
|---|---|---|---|---|---|
| zo | 6:7:9 | 0–12–31 | C vEb G | Cvm | C downminor |
| gu | 10:12:15 | 0–14–31 | C ^Eb G | C^m | C upminor |
| ilo | 18:22:27 | 0–15–31 | C ^^Eb G | C^^m | C dupminor |
| lu | 22:27:33 | 0–16–31 | C vvE G | Cvv | C dudmajor or C dud |
| yo | 4:5:6 | 0–17–31 | C vE G | Cv | C downmajor or C down |
| ru | 14:18:21 | 0–19–31 | C ^E G | C^ | C upmajor or C up |
For a more complete list, see Ups and downs notation #Chords and chord progressions.
Notation
Ups and downs notation
53edo can be notated with ups and downs, spoken as up, dup, dudsharp, downsharp, sharp, upsharp etc. and down, dud, dupflat etc. Note that dudsharp is equivalent to trup (triple-up) and dupflat is equivalent to trud (triple-down).
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |||||||||||||
| Flat symbol |
Another notation uses alternative ups and downs. Here, this can be done using sharps and flats with arrows, borrowed from extended Helmholtz–Ellis notation:
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |||||||||||||
| Flat symbol |
Sagittal notation
Evo flavor
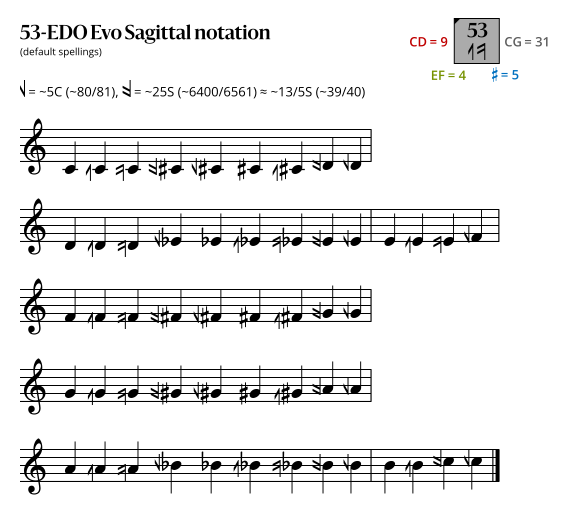
Revo flavor

In the diagrams above, a sagittal symbol followed by an equals sign (=) means that the following comma is the symbol's primary comma (the comma it exactly represents in JI), while an approximately equals sign (≈) means it is a secondary comma (a comma it approximately represents in JI). In both cases the symbol exactly represents the tempered version of the comma in this EDO.
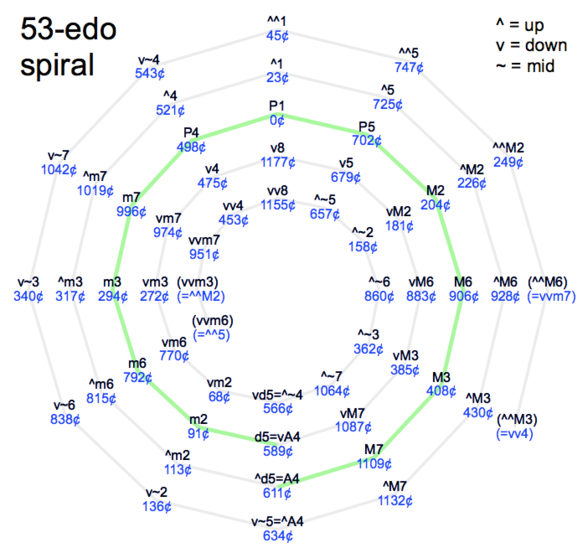
Relationship to 12edo
53edo's circle of 53 fifths can be bent into a 12-spoked "spiral of fifths". This makes sense to do because going up by 12 fifths results in the Pythagorean comma (by definition), which is mapped to one edostep and is thus also the syntonic and septimal comma, introducing a simple second accidental in the form of the arrow to reach useful intervals from the basic 12-chromatic scale. The one-edostep comma is a requirement in Kite's theory, and implies that 31\53 is on the 7\12 kite in the scale tree.
This "spiral of fifths" can be a useful construct for introducing 53edo to musicians unfamiliar with microtonal music. It may help composers and musicians to make visual sense of the notation, and to understand what size of a jump is likely to land them where compared to 12edo.
The two innermost and two outermost intervals on the spiral are duplicates, reflecting the fact that it is a repeating circle at heart and the spiral shape is only a helpful illusion.
Approximation to JI
53edo provides excellent approximations for the classic 5-limit just chords and scales, such as the Ptolemy–Zarlino "just major" scale.
| Interval | Ratio | Size | Difference |
|---|---|---|---|
| Perfect fifth | 3/2 | 31 | −0.07 cents |
| Major third | 5/4 | 17 | −1.40 cents |
| Minor third | 6/5 | 14 | +1.34 cents |
| Major second | 9/8 | 9 | −0.14 cents |
| 10/9 | 8 | −1.27 cents | |
| Minor second | 16/15 | 5 | +1.48 cents |
Because the 5th is so accurate, 53edo also offers good approximations for Pythagorean thirds. In addition, the 43\53 interval is only 4.8 cents wider than 7/4, so 53edo can also be used for 7-limit harmony, in which it tempers out the septimal kleisma, 225/224.
15-odd-limit interval mappings
The following tables show how 15-odd-limit intervals are represented in 53edo. Prime harmonics are in bold; inconsistent intervals are in italics.
| Interval and complement | Error (abs, ¢) | Error (rel, %) |
|---|---|---|
| 1/1, 2/1 | 0.000 | 0.0 |
| 3/2, 4/3 | 0.068 | 0.3 |
| 9/8, 16/9 | 0.136 | 0.6 |
| 9/5, 10/9 | 1.272 | 5.6 |
| 15/13, 26/15 | 1.316 | 5.8 |
| 5/3, 6/5 | 1.340 | 5.9 |
| 13/10, 20/13 | 1.384 | 6.1 |
| 5/4, 8/5 | 1.408 | 6.2 |
| 15/8, 16/15 | 1.476 | 6.5 |
| 13/9, 18/13 | 2.655 | 11.7 |
| 13/12, 24/13 | 2.724 | 12.0 |
| 13/8, 16/13 | 2.792 | 12.3 |
| 7/4, 8/7 | 4.759 | 21.0 |
| 7/6, 12/7 | 4.827 | 21.3 |
| 9/7, 14/9 | 4.895 | 21.6 |
| 13/11, 22/13 | 5.130 | 22.7 |
| 7/5, 10/7 | 6.167 | 27.2 |
| 15/14, 28/15 | 6.235 | 27.5 |
| 15/11, 22/15 | 6.445 | 28.5 |
| 11/10, 20/11 | 6.514 | 28.8 |
| 13/7, 14/13 | 7.551 | 33.3 |
| 11/9, 18/11 | 7.785 | 34.4 |
| 11/6, 12/11 | 7.854 | 34.7 |
| 11/8, 16/11 | 7.922 | 35.0 |
| 11/7, 14/11 | 9.961 | 44.0 |
| Interval and complement | Error (abs, ¢) | Error (rel, %) |
|---|---|---|
| 1/1, 2/1 | 0.000 | 0.0 |
| 3/2, 4/3 | 0.068 | 0.3 |
| 9/8, 16/9 | 0.136 | 0.6 |
| 9/5, 10/9 | 1.272 | 5.6 |
| 15/13, 26/15 | 1.316 | 5.8 |
| 5/3, 6/5 | 1.340 | 5.9 |
| 13/10, 20/13 | 1.384 | 6.1 |
| 5/4, 8/5 | 1.408 | 6.2 |
| 15/8, 16/15 | 1.476 | 6.5 |
| 13/9, 18/13 | 2.655 | 11.7 |
| 13/12, 24/13 | 2.724 | 12.0 |
| 13/8, 16/13 | 2.792 | 12.3 |
| 7/4, 8/7 | 4.759 | 21.0 |
| 7/6, 12/7 | 4.827 | 21.3 |
| 9/7, 14/9 | 4.895 | 21.6 |
| 13/11, 22/13 | 5.130 | 22.7 |
| 7/5, 10/7 | 6.167 | 27.2 |
| 15/14, 28/15 | 6.235 | 27.5 |
| 15/11, 22/15 | 6.445 | 28.5 |
| 11/10, 20/11 | 6.514 | 28.8 |
| 13/7, 14/13 | 7.551 | 33.3 |
| 11/9, 18/11 | 7.785 | 34.4 |
| 11/6, 12/11 | 7.854 | 34.7 |
| 11/8, 16/11 | 7.922 | 35.0 |
| 11/7, 14/11 | 12.681 | 56.0 |
Higher-limit JI
53edo has only 5 pairs of inconsistent intervals in the full 27-odd-limit: {11/7, 14/11}, {17/11, 22/17}, {19/17, 34/19}, {21/11, 22/21}, and {23/22, 44/23}. This is perhaps remarkable compared to 9 pairs in 46edo and 11 pairs in 41edo, because the smallest edo after 53edo to get 5 or less inconsistencies in the 27-odd-limit is 99edo (using the 99ef val), followed by 111edo (patent val), 130edo (patent val) and 159edo (also patent); all of these get 5 inconsistencies as well except 159edo which gets 1 and which is itself a superset of 53edo. However, most interpret the approximation of prime 17 in 53edo as too off for all but the most opportunistic harmonies, and some question the 23 and possibly also 11, so the practical significance of this is debatable.
As shown below, there is also a cluster of usable higher primes starting at 71; even 89 (4.84 ¢ flat), 97 (4.63 ¢ sharp) and 101 (2.6 ¢ sharp) are usable if placed in just the right context. (Note that prime 67 is almost perfectly off.)
| Harmonic | 71 | 73 | 79 | 83 | |
|---|---|---|---|---|---|
| Error | Absolute (¢) | +1.44 | -1.37 | -2.27 | +2.78 |
| Relative (%) | +6.3 | -6.1 | -10.0 | +12.3 | |
| Steps (reduced) |
326 (8) |
328 (10) |
334 (16) |
338 (20) | |
This makes 53edo excellent (for its size) in the 2.3.5.7.11.13.19.23.37.41.71.73(.79.83.101) subgroup, although some higher error primes like 11 and 23 require the right context to be convincing.
Note that the high primes, in rooted (/2n) position, essentially act as alternate interpretations of LCJI intervals, if you want to force a rooted interpretation; namely: 71/64 as ~10/9, 73/64 as ~8/7, 79/64 as ~16/13, and perhaps most questionably in the context of 53edo, 83/64 as ~13/10.
Regular temperament properties
| Subgroup | Comma list | Mapping | Optimal 8ve stretch (¢) |
Tuning error | |
|---|---|---|---|---|---|
| Absolute (¢) | Relative (%) | ||||
| 2.3 | [-84 53⟩ | [⟨53 84]] | +0.022 | 0.022 | 0.10 |
| 2.3.5 | 15625/15552, 32805/32768 | [⟨53 84 123]] | +0.216 | 0.276 | 1.22 |
| 2.3.5.7 | 225/224, 1728/1715, 3125/3087 | [⟨53 84 123 149]] | −0.262 | 0.861 | 3.81 |
| 2.3.5.7.11 | 99/98, 121/120, 176/175, 2200/2187 | [⟨53 84 123 149 183]] | +0.248 | 1.279 | 5.64 |
| 2.3.5.7.11.13 | 99/98, 121/120, 169/168, 176/175, 275/273 | [⟨53 84 123 149 183 196]] | +0.332 | 1.183 | 5.22 |
| 2.3.5.7.11.13.19 | 99/98, 121/120, 169/168, 176/175, 209/208, 275/273 | [⟨53 84 123 149 183 196 225]] | +0.391 | 1.105 | 4.88 |
- 53et is lower in relative error than any previous equal temperaments in the 3-, 5-, and 13-limit. The next equal temperaments doing better in these subgroups are 306, 118, and 58, respectively. It is even more prominent in the 2.3.5.7.13.19 and 2.3.5.7.13.19.23 subgroups, and the next equal temperament doing better in either subgroup is 130.
Commas
Commas that 53edo tempers out using its patent val, ⟨53 84 123 149 183 196 225], include:
| Prime limit |
Ratio[note 2] | Monzo | Cents | Color name | Name(s) |
|---|---|---|---|---|---|
| 3 | (52 digits) | [-84 53⟩ | 3.62 | Tribilawa | 53-comma, Mercator's comma |
| 5 | (14 digits) | [-21 3 7⟩ | 10.06 | Lasepyo | Semicomma |
| 5 | 15625/15552 | [-6 -5 6⟩ | 8.11 | Tribiyo | Kleisma |
| 5 | (14 digits) | [9 -13 5⟩ | 6.15 | Saquinyo | Amity comma |
| 5 | (22 digits) | [24 -21 4⟩ | 4.20 | Sasaquadyo | Vulture comma |
| 5 | 32805/32768 | [-15 8 1⟩ | 1.95 | Layo | Schisma |
| 7 | 3125/3087 | [0 -2 5 -3⟩ | 21.18 | Triru-aquinyo | Gariboh comma |
| 7 | 1728/1715 | [6 3 -1 -3⟩ | 13.07 | Triru-agu | Orwellisma |
| 7 | 225/224 | [-5 2 2 -1⟩ | 7.71 | Ruyoyo | Marvel comma, septimal kleisma |
| 7 | 4375/4374 | [-1 -7 4 1⟩ | 0.40 | Zoquadyo | Ragisma |
| 11 | 99/98 | [-1 2 0 -2 1⟩ | 17.58 | Loruru | Mothwellsma |
| 11 | 121/120 | [-3 -1 -1 0 2⟩ | 14.37 | Lologu | Biyatisma |
| 11 | 176/175 | [4 0 -2 -1 1⟩ | 9.86 | Lorugugu | Valinorsma |
| 11 | (22 digits) | [33 -23 0 0 1⟩ | 6.35 | Trisalo | Pythrabian comma |
| 11 | 385/384 | [-7 -1 1 1 1⟩ | 4.50 | Lozoyo | Keenanisma |
| 11 | 540/539 | [2 3 1 -2 -1⟩ | 3.21 | Lururuyo | Swetisma |
| 13 | 275/273 | [0 -1 2 -1 1 -1⟩ | 12.64 | Thuloruyoyo | Gassorma |
| 13 | 169/168 | [-3 -1 0 -1 0 2⟩ | 10.27 | Thothoru | Buzurgisma, dhanvantarisma |
| 13 | 625/624 | [-4 -1 4 0 0 -1⟩ | 2.77 | Thuquadyo | Tunbarsma |
| 13 | 676/675 | [2 -3 -2 0 0 2⟩ | 2.56 | Bithogu | Island comma, parizeksma |
| 13 | 1001/1000 | [-3 0 -3 1 1 1⟩ | 1.73 | Tholozotrigu | Fairytale comma, sinbadma |
| 13 | 2080/2079 | [5 -3 1 -1 -1 1⟩ | 0.83 | Tholuruyo | Ibnsinma, sinaisma |
| 13 | 4096/4095 | [12 -2 -1 -1 0 -1⟩ | 0.42 | Sathurugu | Schismina |
Linear temperaments
| Periods per 8ve |
Generator* | Cents* | Associated ratio* |
Temperament |
|---|---|---|---|---|
| 1 | 2\53 | 45.3 | 36/35 | Quartonic |
| 1 | 5\53 | 113.2 | 16/15 | Misneb |
| 1 | 6\53 | 135.8 | 13/12~14/13 | Doublethink |
| 1 | 7\53 | 158.5 | 11/10 | Hemikleismic |
| 1 | 9\53 | 203.8 | 9/8 | Baldy |
| 1 | 10\53 | 226.4 | 8/7 | Semaja |
| 1 | 11\53 | 249.1 | 15/13 | Hemischis / hemigari |
| 1 | 12\53 | 271.7 | 7/6 | Orwell |
| 1 | 13\53 | 294.3 | 25/21 | Kleiboh |
| 1 | 14\53 | 317.0 | 6/5 | Hanson / catakleismic / countercata |
| 1 | 15\53 | 339.6 | 11/9 | Amity / houborizic |
| 1 | 16\53 | 362.3 | 16/13 | Submajor |
| 1 | 18\53 | 407.5 | 1225/972 | Ditonic / coditone |
| 1 | 19\53 | 430.2 | 9/7 | Hamity |
| 1 | 20\53 | 452.8 | 13/10 | Maja |
| 1 | 21\53 | 475.5 | 21/16 | Vulture / buzzard |
| 1 | 22\53 | 498.1 | 4/3 | Garibaldi / pontiac |
| 1 | 23\53 | 520.8 | 4/3 | Mavila (53bbcc) |
| 1 | 25\53 | 566.0 | 18/13 | Alphatrimot |
| 1 | 26\53 | 588.7 | 45/32 | Untriton / aufo |
* Octave-reduced form, reduced to the first half-octave
Scales
Mos scales
While there is only one possible generator for the diatonic mos scale supported by this edo, there are a greater number of generators for other mosses such as the antidiatonic scale.
Scales approximated from JI
- The eagle 53 scale described by John O'Sullivan
- Ptolmey–Zarlino justly-intonated major: 9 8 5 9 8 9 5
- Ptolmey–Zarlino justly-intonated minor: 9 5 8 9 5 9 8
- From AFDOs
| This article or section contains multiple idiosyncratic terms. Such terms are used by only a few people and are not regularly used within the community. |
- Composite Cliffedge (approximated from 60afdo): 12 10 9 19 3
- Composite Deja Vu (approximated from 101afdo): 14 17 5 9 8
- Composite Dungeon (approximated from 30afdo): 17 5 9 4 18
- Composite Freeway (approximated from 6afdo): 12 10 9 8 7 7
- Composite Geode (approximated from 6afdo): 12 10 9 15 7
- Composite Labyrinth (approximated from 30afdo): 7 7 17 5 17
- Composite Mushroom (approximated from 30afdo): 12 10 9 3 19
- Composite Underpass (approximated from 10afdo): 14 17 10 4 8
- Spectral Arcade (approximated from 32afdo): 17 4 10 12 10
- Spectral Mechanical (approximated from 16afdo): 13 4 14 12 10
- Spectral Moonbeam (approximated from 16afdo): 9 4 18 17 5
- Spectral Springwater (approximated from 8afdo): 9 8 14 12 10
- Spectral Starship (approximated from 68ifdo): 4 13 4 10 12 10
- Spectral Volcanic (approximated from 16afdo): 5 12 14 12 10
Other scales
- cthon5m: 6 3 6 2 3 6 2 3 6 3 2 6 3 2
- Palace[idiosyncratic term] (approximated from Porky in 29edo): 7 7 8 9 7 7 8
Instruments
Music
- See also: Category:53edo tracks
Modern renderings
- "Jesus bleibet meine Freude" from Herz und Mund und Tat und Leben, BWV 147 (1723) – tuned in 53edo, rendered by Claudi Meneghin (2021)
- Prelude and Fugue in C Major, No. 1, BWV 846, from The Well-Tempered Clavier, Book I (1722) – rendered by Mykhaylo Khramov
- "Ricercar a 3" from The Musical Offering, BWV 1079 (1747) – rendered by Claudi Meneghin (2024)
- "Ricercar a 6" from The Musical Offering, BWV 1079 (1747) – rendered by Claudi Meneghin (2025)
- "Ricercar a 6" from The Musical Offering, BWV 1079 (1747) – with syntonic-comma adjustment, rendered by Claudi Meneghin (2025)
- "Contrapunctus 4" from The Art of Fugue, BWV 1080 (1742–1749) – rendered by Claudi Meneghin (2024)
- "Contrapunctus 11" from The Art of Fugue, BWV 1080 (1742–1749) – rendered by Claudi Meneghin (2024)
- Prelude in E Minor "The Great" – rendered by Claudi Meneghin (2023)
- Prelude in E Minor "The Little" – rendered by Claudi Meneghin (2024)
- Prelude Op. 28, No. 4 in E minor « Suffocation » (1839), arranged for harpsichord, tuned into 53-edo – rendered by Claudi Meneghin (2025)
- Suite in D minor HWV 428 for Harpsichord - Allemande (1720) – rendered by Claudi Meneghin (2024)
- Maple Leaf Rag (1899) – arranged for harpsichord and rendered by Claudi Meneghin (2024)
- Maple Leaf Rag (1899) – with syntonic comma adjustment, arranged for harpsichord and rendered by Claudi Meneghin (2024)
- Qui veut faire l'ange fait la bete – rendered by MortisTheneRd (2024)
- Bande-announce – rendered by MortisTheneRd (2024)
21st century
- Space Race (2022)
- "strange worlds" from hope in dark times (2024) – Spotify | Bandcamp | YouTube – in Hanson[11], 53edo tuning
- "Blasphemous Rumors" from TOTMC September to December 2024 (2024) – Spotify | Bandcamp | YouTube – in Blackdye, 53edo tuning
- "It's a Good Idea to Have a Good Time." from Random Sentences (2025) – Spotify | Bandcamp | YouTube
- "Decearing Egg" from Eggs (2025) – Spotify | Bandcamp | YouTube
- "Husband Head Void" from Void (2025) – Spotify | Bandcamp | YouTube
- "Lasagna Cat" from Microtonal Six-Dimensional Cats (2025) – Spotify | Bandcamp | YouTube
- Like Uminari (2021)
- from No Fun House (2025)
- Whisper Song (2007) – blog | play | SoundCloud
- "Droplet", from Rhythm and Xen (2015) – Bandcamp | SoundCloud | YouTube – drum and bass in Orwell[9], 53edo tuning
- "Stranges" (2021)
- "Initialising", from Microwave (2019) – Bandcamp | SoundCloud
- mothers (2014)
- Schumann: The Poet Speaks in 53-equal (5-limit) on the Lumatone (2022)
- 53-equal: lydian/aeolian pentatonic (2023)
- 53-equal Luma MKI: around a drone on middle C (2023)
- 22 shrutis as Schismatic[22 in A446Hz (53-equal)] (2024)
- A meander around 53-equal on the Lumatone (2025) (this is actually a keyboard mapping guide)
- Hero (2023)
- Ficta (2021)
- "Taking Flight" from Nano Particular (2019) – Spotify | Bandcamp | YouTube
- "Detective Duckweed" from Jazzbeetle (2023) – Spotify | Bandcamp | YouTube – jazzy big band electronic hybrid
Notes
- ↑ Based on treating 53edo as a no-17's 19-limit temperament; other approaches are also possible. Italics represent inconsistent intervals which are mapped by the 19-limit patent val to their second-closest (as opposed to closest) approximation in 53edo.
- ↑ Ratios longer than 10 digits are presented by placeholders with informative hints.