Spiral chart
A spiral chart is an illustration which converts a circle of repeats of an interval in one edo into a self-similar spiral shape, so that it may be compared with a circle of the same interval in a smaller coprime edo.
Spiral charts were invented by Kite Giedraitis no later than April 2014.
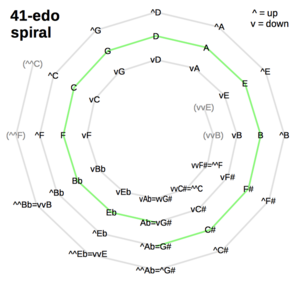
Spirals of twelve fifths
The spiral charts for 31edo, 41edo and 53edo relate each of those edos to 12edo. Each chart has 12 wheel-spokes.
The larger edo's spiral of fifths is not really a spiral, it's a larger circle of fifths that is broken into a chain to make several smaller 12-note loops. Then a few duplicates are added at each end of the chain, so that one can reconnect the ends mentally to get the original larger circle.
A 12-spoke spiral chart of fifths is only possible if the dodeca-sharpness (edosteps per Pythagorean comma) of the larger edo is 1 or -1. A 12-spoke spiral of semitones is possible for edos of the form 12n+1 or 12n-1, but those spirals are less interesting because they convey very little info that isn't already in the table of edosteps.
Gallery
31edo spiral chart
41edo spiral chart
41edo spiral chart (notation)
53edo spiral chart
The same information can be presented as a table. To follow the circle of 5ths, read the columns left to right, and within each column read top to bottom.
| Tritones | vd5 = ~4 = 556¢ | d5 = vA4 = 585¢ | ^d5 = A4 = 615¢ | ~5 = ^A4 = 644¢ | |
|---|---|---|---|---|---|
| Minorish 2nds | vm2 = 59¢ | m2 = 88¢ | ^m2 = 117¢ | ~2 = 146¢ | |
| Minorish 6ths | vm6 = 761¢ | m6 = 790¢ | ^m6 = 820¢ | ~6 = 849¢ | |
| Minorish 3rds | vm3 = 263¢ | m3 = 293¢ | ^m3 = 322¢ | (~3) | |
| Minorish 7ths | vm7 = 966¢ | m7 = 995¢ | ^m7 = 1024¢ | (~7) | |
| Perfectish 4ths | v4 = 468¢ | P4 = 498¢ | ^4 = 527¢ | ||
| Perfectish 1sns / 8ves | v8 = 1171¢ | P1 = 0¢ | ^1 = 29¢ | ||
| Perfectish 5ths | v5 = 673¢ | P5 = 702¢ | ^5 = 732¢ | ||
| Majorish 2nds | (~2) | vM2 =176 ¢ | M2 = 205¢ | ^M2 = 234¢ | |
| Majorish 6ths | (~6) | vM6 = 878¢ | M6 = 907¢ | ^M6 = 937¢ | |
| Majorish 3rds | ~3 = 351¢ | vM3 = 380¢ | M3 = 410¢ | ^M3 = 439¢ | |
| Majorish 7ths | ~7 = 1054¢ | vM7 = 1083¢ | M7 = 1112¢ | ^M7 = 1141¢ | |
| Tritones | ~4 = vd5 = 556¢ | vA4 = d5 = 585¢ | A4 = ^d5 = 615¢ | ^A4 = ~5 = 644¢ |
Spirals of other amounts, other intervals
Such a spiral chart can be made for any two edos, as long as they are coprime. It's often a spiral of something other than fifths. In fact, it's a spiral of the nearest miss.
For example, consider 8edo and 27edo. The near misses are 3\8 and 10\27. You get an 8-spoke spiral of 27edo major 3rds. This might be useful for someone researching octatonic scales in 27edo. To follow the circle of 3rds, read the columns left to right, and within each column read top to bottom.
| Tritones | A4 = v5 | vA4 = vv5 | ^^4 = ^d5 | ^4 = d5 | |
|---|---|---|---|---|---|
| Middish 7ths | vM7 | ~7 | ^m7 | m7 | |
| Minorish 3rds | ~3 | ^m3 | m3 | (M2) | |
| Minorish 6ths | ^m6 | m6 | vm6 | ||
| Perfectish 1sns / 8ves | ^1 | P1 | v8 | ||
| Majorish 3rds | ^M3 | M3 | vM3 | ||
| Majorish 6ths | (m7) | M6 | vM6 | ~6 | |
| Middish 2nds | M2 | vM2 | ~2 | ^m2 | |
| Tritones | A4 = v5 | vA4 = vv5 | ^^4 = ^d5 | ^4 = d5 |
Relationship to the scale tree
 |
Todo: complete section |