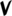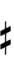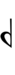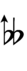34edo
| ← 33edo | 34edo | 35edo → |
34 equal divisions of the octave (abbreviated 34edo or 34ed2), also called 34-tone equal temperament (34tet) or 34 equal temperament (34et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 34 equal parts of about 35.3 ¢ each. Each step represents a frequency ratio of 21/34, or the 34th root of 2.
Theory
34edo contains two 17edo's and the half-octave tritone of 600 cents. It excels in approximating harmonics 3, 5, 13, 17, and 23 (2.3.5.13.17.23 subgroup a.k.a. the no-7's no-11's no-19's 23-limit), with tuning even more accurate than 31edo in the 5-limit, but with a sharp tendency and fifth rather than a flat one, and not tempering out 81/80 unlike 31edo.
34edo's significance in regards to JI approximation comes from making many simple and natural equivalences between JI intervals. For example, a key characteristic of 34edo is that it splits the standard whole tone of 9/8 into six parts, such that three chromatic semitones of 25/24 or two diatonic semitones of 16/15 result in 9/8. Additionally, if you stack a five-step 10/9 interval four times, you reach the perfect fifth 3/2, supporting tetracot. This also means that the perfect fifth is mapped to 20 steps. Given that and the fact that the major third 5/4 is mapped to 11 steps, one can see that 34edo takes advantage of a natural logarithmic approximation of 5/4 as a portion of 3/2, or equivalently 6/5 as a portion of 5/4, resulting in gammic temperament. It also has the thirds from 17edo: "neogothic" minor and major thirds of about 282 and 424 cents, and a neutral third of 353 cents. For extraclassical tonality, a tendo third of 459 cents and an arto third of 247 cents are also available, approximating 13/10 and 15/13 respectively.
34edo supports the diatonic scale, both the simpler 5L 2s moment-of-symmetry form and a more complex nicetone scale representing the zarlino diatonic. This can be extended into a 12-note chromatic scale of 10L 2s by stacking the two different varieties of semitones, with an intuitive non-MOS form appearing at LLsLLLLLLsLL (created by first subdividing 34edo into the standard pentic scale and then splitting that into further smaller steps).
Odd harmonics
| Harmonic | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 | 21 | 23 | 25 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +3.9 | +1.9 | -15.9 | +7.9 | +13.4 | +6.5 | +5.8 | +0.9 | -15.2 | -12.0 | +7.0 | +3.8 |
| Relative (%) | +11.1 | +5.4 | -45.0 | +22.3 | +37.9 | +18.5 | +16.6 | +2.6 | -43.0 | -33.9 | +19.9 | +10.9 | |
| Steps (reduced) |
54 (20) |
79 (11) |
95 (27) |
108 (6) |
118 (16) |
126 (24) |
133 (31) |
139 (3) |
144 (8) |
149 (13) |
154 (18) |
158 (22) | |
Intervals
| Cents | Approx. ratios[note 1] | Ratios of 7 Using the 34 Val |
Ratios of 7 Using the 34d Val |
Ups and Downs Notation
(EUs: v4A1 and ^^d2) |
Solfeges | ||||
|---|---|---|---|---|---|---|---|---|---|
| 0 | 0.000 | 1/1 | P1 | perfect unison | D | da | do | ||
| 1 | 35.294 | 81/80, 128/125, 51/50 | 28/27, 64/63 | 36/35 | ^1, vm2 | up 1sn, downminor 2nd | ^D, vEb | du/fro | di |
| 2 | 70.588 | 25/24, 26/25, 24/23, 27/26, 23/22, 648/625, 33/32 | 21/20, 36/35, 50/49 | 28/27, 49/48 | ^^1, m2 | dup 1sn, minor 2nd | ^^D, Eb | fra | rih |
| 3 | 105.882 | 17/16, 18/17, 16/15 | 14/13 | 15/14, 21/20 | vA1, ^m2 | downaug 1sn, upminor 2nd |
vD#, ^Eb | fru | ra |
| 4 | 141.176 | 13/12, 12/11, 25/23 | 15/14 | 14/13 | A1, ~2 | aug 1sn, mid 2nd | D#, vvE | ri | ru |
| 5 | 176.471 | 10/9, 11/10 | vM2 | downmajor 2nd | vE | ro | reh | ||
| 6 | 211.765 | 9/8, 17/15, 26/23 | 8/7 | M2 | major 2nd | E | ra | re | |
| 7 | 247.059 | 15/13, 23/20 | 7/6, 8/7 | ^M2, vm3 | upmajor 2nd, downminor 3rd |
^E, vF | ru/no | raw | |
| 8 | 282.353 | 20/17, 75/64, 27/23, 13/11 | 7/6 | m3 | minor 3rd | F | na | meh | |
| 9 | 317.647 | 6/5 | 17/14 | ^m3 | upminor 3rd | ^F | nu | me | |
| 10 | 352.941 | 16/13, 11/9, 27/22 | 17/14, 21/17 | ~3 | mid 3rd | ^^F | mi | mu | |
| 11 | 388.235 | 5/4 | 14/11 | 21/17 | vM3 | downmajor 3rd | vF# | mo | mi |
| 12 | 423.529 | 51/40, 32/25, 23/18 | 9/7, 14/11 | M3 | major 3rd | F# | ma | maa | |
| 13 | 458.824 | 13/10, 30/23, 17/13, 22/17 | 9/7, 21/16 | ^M3, v4 | upmajor 3rd, down 4th | ^F#, vG | mu/fo | maw | |
| 14 | 494.118 | 4/3 | 21/16 | P4 | 4th | G | fa | fa | |
| 15 | 529.412 | 27/20, 34/25, 15/11, 23/17 | ^4 | up 4th | ^G | fu | fih | ||
| 16 | 564.706 | 25/18, 18/13, 11/8, 32/23 | 7/5 | ~4, d5 | mid 4th, dim 5th | ^^G, Ab | fi/sha | fu | |
| 17 | 600.000 | 45/32, 64/45, 17/12, 24/17 | 7/5, 10/7 | vA4, ^d5 | downaug 4th, updim 5th | vG#, ^Ab | po/shu | fi/se | |
| 18 | 635.294 | 36/25, 13/9, 16/11, 23/16 | 10/7 | A4, ~5 | aug 4th, mid 5th | G#, vvA | pa/si | su | |
| 19 | 670.588 | 40/27, 25/17, 22/15, 34/23 | v5 | down 5th | vA | so | sih | ||
| 20 | 705.882 | 3/2 | 32/21 | P5 | perfect 5th | A | sa | sol | |
| 21 | 741.176 | 20/13, 23/15, 26/17, 17/11 | 14/9, 32/21 | ^5, vm6 | up 5th, downminor 6th | ^A, vBb | su/flo | saw | |
| 22 | 776.471 | 25/16, 80/51, 36/23 | 14/9, 11/7 | m6 | minor 6th | Bb | fla | leh | |
| 23 | 811.765 | 8/5 | 11/7 | 34/21 | ^m6 | upminor 6th | ^Bb | flu | le |
| 24 | 847.059 | 13/8, 18/11, 44/27 | 28/17, 34/21 | ~6 | mid 6th | vvB | li | lu | |
| 25 | 882.353 | 5/3 | 28/17 | vM6 | downmajor 6th | vB | lo | la | |
| 26 | 917.647 | 17/10, 128/75, 46/27, 22/13 | 12/7 | M6 | major 6th | B | la | laa | |
| 27 | 952.941 | 26/15, 40/23 | 7/4, 12/7 | ^M6, vm7 | upmajor 6th, downminor 7th |
^B, vC | lu/tho | law | |
| 28 | 988.235 | 16/9, 30/17, 23/13 | 7/4 | m7 | minor 7th | C | tha | teh | |
| 29 | 1023.529 | 9/5, 20/11 | ^m7 | upminor 7th | ^C | thu | te | ||
| 30 | 1058.824 | 24/13, 11/6, 46/25 | 28/15 | 13/7 | ~7 | mid 7th | ^^C | ti | tu |
| 31 | 1094.118 | 32/17, 17/9, 15/8 | 13/7 | 28/15, 40/21 | vM7 | downmajor 7th | vC# | to | ti |
| 32 | 1129.412 | 48/25, 25/13, 23/12, 625/324, 64/33 | 40/21, 35/18, 49/25 | 27/14, 96/49 | M7 | major 7th | C# | ta | taa |
| 33 | 1164.706 | 160/81, 125/64, 100/51 | 27/14, 63/32 | 35/18 | ^M7, v8 | upmajor 7th, down 8ve | vD | tu/do | da |
| 34 | 1200.000 | 2/1 | P8 | 8ve | D | da | do | ||
Chords can be named using ups and downs as C upminor, D downmajor seven, etc. See Ups and Downs Notation #Chord names in other EDOs.
Notation
Ups and downs notation
34edo can be notated with ups and downs, spoken as up, dup, downsharp, sharp, upsharp etc. and down, dud, upflat etc. Note that dup is equivalent to dudsharp and dud is equivalent to dupflat.
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |
|||||||||
| Flat symbol | |
|
Alternative symbols for ups and downs notation uses sharps and flats with arrows, borrowed from extended Helmholtz–Ellis notation:
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |
|
|
|
|
|
|
| ||
| Flat symbol | |
|
|
|
|
|
Sagittal notation
This notation uses the same sagittal sequence as 41-EDO, and is a superset of the notation for 17-EDO.
Evo flavor
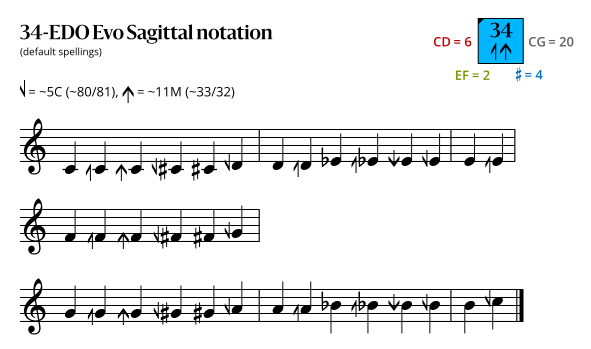
Revo flavor

Evo-SZ flavor

Kosmorsky's thoughts
The chain of fifths gives you the seven naturals, and their sharps and flats. The sharp or flat of a note is (what is commonly called) a neutral second away – the double-sharp means a minor third away from the natural. This has led certain "complainers", in seeking to notate 17 edo, to create an extra character to raise something a small step of which. To render this symbol philosophically harmonious with 34 tone equal temperament, a symbol indicating an adjustment of 1/34 up or down serves the purpose by using two of it, doubled laterally or vertically as composer. This however emphasizes certain aspects of 34edo which may not be most efficient expressions of some musical purposes. Users can construct their own notation to the needs of the music and performer. As an example, a system with 15 "nominals" like A, B, C … F, instead of seven, might be waste – of paper, or space, or memory if they aren't used consecutively frequently. The system spelled out here has familiarity as an advantage and disadvantage. The spacing of the nominals and lines is the same. Dense chords of certain types would be very impossible to notate. Finally, the table uses ^ and v for "up" and "down", but these might be reserved for adjustments of 1/68th of an octave, being hollow, and filled in triangles are recommended.
Approximation to JI

Like 17edo, 34edo contains good approximations of just intervals involving 3, 11, and 13 – specifically, 13/8, 13/12, 13/11, 13/9, 11/9 and their inversions – while failing to closely approximate ratios of 7 given its step size. 34edo adds ratios of 5 into the mix – including 5/4, 6/5, 9/5, 15/8, 13/10, 15/13, and their inversions – as well as 17 – including 17/16, 18/17, 17/12, 17/11, 17/10, 17/13, 17/15 and their inversions. Since it distinguishes between 9/8 and 10/9 (exaggerating the difference between them, the syntonic comma of 81/80, from 21.5 cents to 35.3 cents), it is suitable for quasi-5-limit JI but is not a meantone system. While no number of fifths (3/2) land on major or minor thirds, an even number of major or minor thirds will be the same pitch as a pitch somewhere in the circle of seventeen fifths.
The sharpening of ~13 ¢ of 11/8 can fit with the 9/8 and 13/8 which both are about 7 cents sharp. This is the basis of a subtle trick: the guitarist tunes the high 'E' string flat by several cents, enough to be imperceptible in many contexts, but which makes chords/harmonies against those several intervals tuned more justly.
Likewise the 16 ¢ flat 27\34 approximate 7/4 can be musically useful especially in kleismic or 4L 3s contexts (with generator a 9\34 minor third). On the other hand, the slightly worse and sharper 7/4, 28\34, sounds more like the "dominant seventh" found in blues and jazz – which some listeners are accustomed to. (68edo contains a copy of 34edo and has the intervals 7/4 and 11/8 tuned nearly just.)
Interval mappings
The following tables show how 15-odd-limit intervals are represented in 34edo. Prime harmonics are in bold; inconsistent intervals are in italics.
| Interval and complement | Error (abs, ¢) | Error (rel, %) |
|---|---|---|
| 1/1, 2/1 | 0.000 | 0.0 |
| 15/13, 26/15 | 0.682 | 1.9 |
| 13/9, 18/13 | 1.324 | 3.8 |
| 5/4, 8/5 | 1.922 | 5.4 |
| 5/3, 6/5 | 2.006 | 5.7 |
| 13/12, 24/13 | 2.604 | 7.4 |
| 3/2, 4/3 | 3.927 | 11.1 |
| 13/10, 20/13 | 4.610 | 13.1 |
| 11/9, 18/11 | 5.533 | 15.7 |
| 15/8, 16/15 | 5.849 | 16.6 |
| 9/5, 10/9 | 5.933 | 16.8 |
| 11/7, 14/11 | 6.021 | 17.1 |
| 13/8, 16/13 | 6.531 | 18.5 |
| 13/11, 22/13 | 6.857 | 19.4 |
| 15/11, 22/15 | 7.539 | 21.4 |
| 9/8, 16/9 | 7.855 | 22.3 |
| 11/6, 12/11 | 9.461 | 26.8 |
| 11/10, 20/11 | 11.466 | 32.5 |
| 9/7, 14/9 | 11.555 | 32.7 |
| 13/7, 14/13 | 12.878 | 36.5 |
| 11/8, 16/11 | 13.388 | 37.9 |
| 15/14, 28/15 | 13.560 | 38.4 |
| 7/6, 12/7 | 15.482 | 43.9 |
| 7/4, 8/7 | 15.885 | 45.0 |
| 7/5, 10/7 | 17.488 | 49.5 |
| Interval and complement | Error (abs, ¢) | Error (rel, %) |
|---|---|---|
| 1/1, 2/1 | 0.000 | 0.0 |
| 15/13, 26/15 | 0.682 | 1.9 |
| 13/9, 18/13 | 1.324 | 3.8 |
| 5/4, 8/5 | 1.922 | 5.4 |
| 5/3, 6/5 | 2.006 | 5.7 |
| 13/12, 24/13 | 2.604 | 7.4 |
| 3/2, 4/3 | 3.927 | 11.1 |
| 13/10, 20/13 | 4.610 | 13.1 |
| 11/9, 18/11 | 5.533 | 15.7 |
| 15/8, 16/15 | 5.849 | 16.6 |
| 9/5, 10/9 | 5.933 | 16.8 |
| 13/8, 16/13 | 6.531 | 18.5 |
| 13/11, 22/13 | 6.857 | 19.4 |
| 15/11, 22/15 | 7.539 | 21.4 |
| 9/8, 16/9 | 7.855 | 22.3 |
| 11/6, 12/11 | 9.461 | 26.8 |
| 11/10, 20/11 | 11.466 | 32.5 |
| 11/8, 16/11 | 13.388 | 37.9 |
| 7/4, 8/7 | 15.885 | 45.0 |
| 7/5, 10/7 | 17.806 | 50.5 |
| 7/6, 12/7 | 19.812 | 56.1 |
| 15/14, 28/15 | 21.734 | 61.6 |
| 13/7, 14/13 | 22.416 | 63.5 |
| 9/7, 14/9 | 23.739 | 67.3 |
| 11/7, 14/11 | 29.273 | 82.9 |
| Interval and complement | Error (abs, ¢) | Error (rel, %) |
|---|---|---|
| 1/1, 2/1 | 0.000 | 0.0 |
| 15/13, 26/15 | 0.682 | 1.9 |
| 13/9, 18/13 | 1.324 | 3.8 |
| 5/4, 8/5 | 1.922 | 5.4 |
| 5/3, 6/5 | 2.006 | 5.7 |
| 13/12, 24/13 | 2.604 | 7.4 |
| 3/2, 4/3 | 3.927 | 11.1 |
| 13/10, 20/13 | 4.610 | 13.1 |
| 11/9, 18/11 | 5.533 | 15.7 |
| 15/8, 16/15 | 5.849 | 16.6 |
| 9/5, 10/9 | 5.933 | 16.8 |
| 11/7, 14/11 | 6.021 | 17.1 |
| 13/8, 16/13 | 6.531 | 18.5 |
| 13/11, 22/13 | 6.857 | 19.4 |
| 15/11, 22/15 | 7.539 | 21.4 |
| 9/8, 16/9 | 7.855 | 22.3 |
| 11/6, 12/11 | 9.461 | 26.8 |
| 11/10, 20/11 | 11.466 | 32.5 |
| 9/7, 14/9 | 11.555 | 32.7 |
| 13/7, 14/13 | 12.878 | 36.5 |
| 11/8, 16/11 | 13.388 | 37.9 |
| 15/14, 28/15 | 13.560 | 38.4 |
| 7/6, 12/7 | 15.482 | 43.9 |
| 7/5, 10/7 | 17.488 | 49.5 |
| 7/4, 8/7 | 19.409 | 55.0 |
Of particular interest is the fact that the 34d val allows all 15-odd-limit intervals to be mapped consistently except for 7/4 and 8/7.
Zeta peak index
| Tuning | Strength | Octave (cents) | Integer limit | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| ZPI | Steps per 8ve |
Step size (cents) |
Height | Integral | Gap | Size | Stretch | Consistent | Distinct | |
| Tempered | Pure | |||||||||
| 144zpi | 34.044841 | 35.247631 | 6.685147 | 5.810937 | 1.241437 | 16.236989 | 1198.419461 | −1.580539 | 6 | 6 |
Tuning by ear
In principle, one can approximate 34edo by ear using only 5-limit intervals, using the fact that 17edo is very close to a circle of seventeen 25/24 chromatic semitones to within 1.5 ¢, and using a pure 5/4 which is less than 2 ¢ off for the second chain. The overall tuning error, assuming everything is tuned perfectly, will be less than 3.5 ¢, or a relative error of less than 10%.
Approximation to irrational intervals
As a Fibonacci number, 34edo contains a fraction of an octave which is a close approximation to the logarithmic phi – 21 degrees of 34edo, approximately 741.2 ¢. Repeated iterations of this interval generates moment of symmetry scales with near-phi relationships between the step sizes. As a 2.3.5.13 temperament, the 21\34 generator is an approximate 20/13, and the temperament tempers out 512/507 and [-6 2 6 0 0 -13⟩. From the tempering of 512/507, two 16/13 neutral thirds are an approximate 3/2, defining an essentially tempered neutral triad with a sharp rather than a flat fifth. (On the other hand, the frequency ratio phi is ~ 833 ¢, and the equal divisions of octave approximating this interval closely are 13edo and 36edo.)
Counterpoint
34edo has such an excellent sqrt(25/24) that the next edo to have a better one is 441. Every sequence of intervals available in 17edo are reachable by strict contrary motion in 34edo.
Regular temperament properties
| Subgroup | Comma list | Mapping | Optimal 8ve stretch (¢) |
Tuning error | |
|---|---|---|---|---|---|
| Absolute (¢) | Relative (%) | ||||
| 2.3.5 | 2048/2025, 15625/15552 | [⟨34 54 79]] | −1.10 | 1.03 | 2.92 |
| 2.3.5.7 | 50/49, 64/63, 4375/4374 | [⟨34 54 79 96]] (34d) | −2.56 | 2.66 | 7.57 |
| 2.3.5.7.11 | 50/49, 64/63, 99/98, 243/242 | [⟨34 54 79 96 118]] (34d) | −2.82 | 2.44 | 6.93 |
| 2.3.5.7.11.13 | 50/49, 64/63, 78/77, 99/98, 144/143 | [⟨34 54 79 96 118 126]] (34d) | −2.64 | 2.26 | 6.42 |
| 2.3.5.7.11.13.17 | 50/49, 64/63, 78/77, 85/84, 99/98, 144/143 | [⟨34 54 79 96 118 126 139]] (34d) | −2.30 | 2.26 | 6.41 |
In the 5-limit, 34edo supports hanson, srutal, tetracot, würschmidt, and vishnu temperaments. It does less well in the 7-limit, with two mappings possible for 7/4: a flat one from the patent val, and a sharp one from the 34d val. By way of the patent val 34 supports keemun temperament, and 34d is an excellent alternative to 22edo for 7-limit pajara temperament. In the 11-limit, 34de supports 11-limit pajaric, and in fact is quite close to the POTE tuning; it adds 4375/4374 to the commas of 11-limit pajaric. On the other hand, the 34d val supports pajara, vishnu and würschmidt, adding 4375/4374 to the commas of pajara. Among subgroup temperaments, the patent val supports semaphore on the 2.3.7 subgroup.
Uniform maps
| Min. size | Max. size | Wart notation | Map |
|---|---|---|---|
| 33.7547 | 33.8081 | 34cef | ⟨34 54 78 95 117 125] |
| 33.8081 | 33.9149 | 34ef | ⟨34 54 79 95 117 125] |
| 33.9149 | 33.9651 | 34e | ⟨34 54 79 95 117 126] |
| 33.9651 | 34.0178 | 34 | ⟨34 54 79 95 118 126] |
| 34.0178 | 34.1851 | 34d | ⟨34 54 79 96 118 126] |
| 34.1851 | 34.2388 | 34dff | ⟨34 54 79 96 118 127] |
Rank-2 temperaments
| Periods per 8ve |
Generator | Cents | Mosses | Temperaments |
|---|---|---|---|---|
| 1 | 1\34 | 35.294 | Gammic | |
| 3\34 | 105.88 | 11L 1s 11L 12s |
||
| 5\34 | 176.471 | 6L 1s 7L 6s 7L 13s |
Tetracot, bunya (34d), modus (34d), monkey (34), wollemia (34) | |
| 7\34 | 247.059 | 5L 4s 5L 9s 5L 14s 5L 19s |
Immunity (34), immunized (34d) | |
| 9\34 | 317.647 | 4L 3s 4L 7s 4L 11s 15L 4s |
Hanson, keemun (34), catalan (34d), catakleismic (34d) | |
| 11\34 | 388.235 | 3L 7s 3L 10s 3L 13s 3L 16s 3L 19s 3L 22s |
Würschmidt (34d), worschmidt (34) | |
| 13\34 | 458.824 | 3L 2s 5L 3s 8L 5s 13L 8s |
Petrtri | |
| 15\34 | 529.412 | 2L 3s 2L 5s 7L 2s 9L 7s |
Mabila | |
| 2 | 2\34 | 70.588 | 16L 2s | Vishnu |
| 3\34 | 105.882 | 2L 6s 2L 8s 10L 2s 12L 10s |
Srutal (34d), pajara (34d), diaschismic (34) | |
| 4\34 | 141.176 | 2L 6s 8L 2s 8L 10s |
Fifive, crepuscular (34d), fifives (34) | |
| 5\34 | 176.471 | 6L 2s 6L 8s 14L 6s |
Stratosphere | |
| 6\34 | 211.765 | 4L 2s 6L 4s 6L 10s 6L 16s |
Antikythera | |
| 7\34 | 247.059 | 4L 2s 4L 6s 10L 4s |
Tobago | |
| 8\34 | 282.353 | 2L 2s 4L 2s 4L 6s 4L 10s 4L 14s |
Bikleismic |
Commas
34et tempers out the following commas. This assumes the patent val ⟨34 54 79 95 118 126].
| Prime limit |
Ratio[note 2] | Monzo | Cents | Color name | Name |
|---|---|---|---|---|---|
| 3 | (18 digits) | [27 -17⟩ | 66.765 | Sasawa | 17-comma |
| 5 | 20000/19683 | [5 -9 4⟩ | 27.660 | Saquadyo | Tetracot comma |
| 5 | 2048/2025 | [11 -4 -2⟩ | 19.553 | Sagugu | Diaschisma |
| 5 | (12 digits) | [17 1 -8⟩ | 11.445 | Saquadbigu | Würschmidt comma |
| 5 | 15625/15552 | [-6 -5 6⟩ | 8.107 | Tribiyo | Kleisma |
| 5 | (20 digits) | [23 6 -14⟩ | 3.338 | Sasepbigu | Vishnuzma |
| 7 | 1029/1000 | [-3 1 -3 3⟩ | 49.492 | Trizogu | Keega |
| 7 | 49/48 | [-4 -1 0 2⟩ | 35.697 | Zozo | Semaphoresma, slendro diesis |
| 7 | 875/864 | [-5 -3 3 1⟩ | 21.902 | Zotriyo | Keema |
| 7 | 126/125 | [1 2 -3 1⟩ | 13.795 | Zotrigu | Starling comma |
| 11 | 100/99 | [2 -2 2 0 -1⟩ | 17.399 | Luyoyo | Ptolemisma |
| 11 | 243/242 | [-1 5 0 0 -2⟩ | 7.139 | Lulu | Rastma |
| 11 | 385/384 | [-7 -1 1 1 1⟩ | 4.503 | Lozoyo | Keenanisma |
| 13 | 91/90 | [-1 -2 -1 1 0 1⟩ | 19.120 | Thozogu | Superleap |
Scales
MOS scales
Ternary scales
Instruments
Lumatone
Skip fretting
Music
Modern renderings
- "Ricercar a 6" from The Musical Offering, BWV 1079 (1747) – with syntonic-comma adjustment, rendered by Claudi Meneghin (2025)
- Maple Leaf Rag (1899) – rendered by Claudi Meneghin (2024)
- Aria Sopra La Bergamasca – arranged for Organ and rendered by Claudi Meneghin (2024)
21st century
- "October Dieting Plan" from TOTMC Suite Vol. 1 (2023) – modus in 34edo tuning
- Kythira's Wake (2019)
- Septendecimal Samsara (2019) – synthwave
- Dodecahedron (2019) – contemporary jazz
- No Threes For You (2019)
- "Elements - Water" from Elements (2019–2020)
- "Travel To Stay" from Mysteries (2023) – Spotify | Bandcamp | YouTube
- "Locksmiths" from The Decatonic Album (2024) – Spotify | Bandcamp | YouTube
- Plane Sonatina No. 2 (2025)
- cucumber service (2025)
- Austice (2023)
- Like refracted light (2023)
- Ascension (2010)
- look (2023)
- 34-equal Luma: a little sentimental (2023)
- 34 equal: classic triads (2023)
- Diaschismatic/Srutal[12] in 34-equal on the harpsichord (2024)
- Perspective (2021)
- 傘がなくても嬉しい (The Puddle Song) (2021)
- from Lesser Groove (2020)
- from Xotla's Microtonal Funk & Blues Vol. 2 (2020)
- Between Space (2022) – ambient sci-fi
- Modal Studies in Tetracot (2021)
See also
External links
- 34 Equal Guitar by Larry Hanson [dead link]
- Websites of Neil Haverstick
- [1] – somehow broken (if you scroll to right, you'll find the songs, playing them, you can't hear anything)