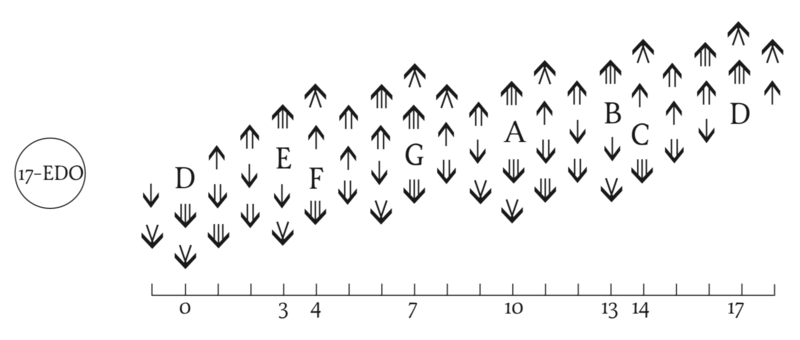
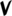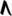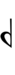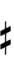17edo

← 16edo

17edo

18edo →

Prime factorization

17 (prime)

Step size

70.5882 ¢

Fifth

10\17 (705.882 ¢)
(semiconvergent)

Semitones (A1:m2)

2:1 (141.2 ¢ : 70.59 ¢)

Consistency limit

3

Distinct consistency limit

3

17 equal divisions of the octave (abbreviated 17edo or 17ed2), also called 17-tone equal temperament (17tet) or 17 equal temperament (17et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 17 equal parts of about 70.6 ¢ each. Each step represents a frequency ratio of 2^1/17, or the 17th root of 2.

English Wikipedia has an article on:

17 equal temperament

Theory

17edo's perfect fifth is around 4 cents sharp of just, and around 6 cents sharp of 12edo's, lending itself to a diatonic scale with more constrasting large and small steps, so it can be seen as a tuning that emphasizes the hardness of Pythagorean tuning rather than mellowing it out as in meantone. Meanwhile, it approximates harmonics 7, 11, 13, and 23 to reasonable degrees, despite completely missing harmonic 5. Thus it can plausibly be treated as a temperament of the 2.3.25.7.11.13.23 subgroup, for which it is quite accurate (though the 7-limit ratios are generally not as well-represented as those of the other integers).

Because the 5th harmonic is not well-approximated, using timbres with attenuated 5th harmonics (and its multiples) may reduce audible beating.

The standard major triad is quite dissonant as the major third is closer to 9/7 than the traditional 5/4. Instead, the tonic chords of 17edo could be considered to be the tetrad 6:7:8:9 and its utonal inversion (representing 14:16:18:21 as 64/63 is tempered out), the former of which is a subminor chord with added fourth, and the latter a supermajor chord with added second (resembling the mu chord of Steely Dan fame). These are realized in 17edo as 0–4–7–10 and 0–3–6–10, respectively. Both of these have distinct moods, and are stable and consonant, if somewhat more sophisticated than their classic 5-limit counterparts. To this group we could also add the 0–3–7–10 (a sus2-4 chord). These three chords comprise the three ways to divide the 17edo perfect fifth into two whole tones and one subminor third. Chromatic alterations of them also exist, for example, the 0–3–7–10 chord may be altered to 0–2–7–10 (which approximates 12:13:16:18) or 0–3–8–10 (which approximates 8:9:11:12). The 0–3–8–10 chord is impressive-sounding, resembling a sus4 but with even more tension; it resolves quite nicely to 0–3–6–10.

Another construction of septimal chords involves 4:7:12 and its inversion 7:12:21. These triads span a twelfth, realized in 17edo as 0–14–27 and 0–13–27, respectively. To this we may add 0–12–14–27, representing 8:13:14:24, or 0–13–15–27, representing 7:12:13:21.

Odd harmonics

Approximation of odd harmonics in 17edo
Harmonic		3	5	7	9	11	13	15	17	19	21	23
Error	Absolute (¢)	+3.9	-33.4	+19.4	+7.9	+13.4	+6.5	-29.4	-34.4	-15.2	+23.3	+7.0
Error	Relative (%)	+5.6	-47.3	+27.5	+11.1	+19.0	+9.3	-41.7	-48.7	-21.5	+33.1	+9.9
Steps (reduced)		27 (10)	39 (5)	48 (14)	54 (3)	59 (8)	63 (12)	66 (15)	69 (1)	72 (4)	75 (7)	77 (9)

Approximation of odd harmonics in 17edo (continued)
Harmonic		25	27	29	31	33	35	37	39	41	43	45	47
Error	Absolute (¢)	+3.8	+11.8	+29.2	-15.6	+17.3	-14.0	+31.0	+10.5	-5.5	-17.4	-25.5	-30.2
Error	Relative (%)	+5.4	+16.7	+41.4	-22.1	+24.5	-19.8	+43.9	+14.8	-7.8	-24.7	-36.2	-42.8
Steps (reduced)		79 (11)	81 (13)	83 (15)	84 (16)	86 (1)	87 (2)	89 (4)	90 (5)	91 (6)	92 (7)	93 (8)	94 (9)

Octave stretch

17edo's approximations of harmonics 3, 7, 11, and 13 are all tempered sharp, so 17edo adapts well to slightly compressing the octave, if that is acceptable. 27edt and 44ed6 are great demonstrations of this, where the octaves are flattened by about 2.5 and 1.5 cents, respectively.

Subsets and supersets

17edo is the seventh prime edo, following 13edo and coming before 19edo, so it does not contain any nontrivial subset edos, though it contains 17ed4. 34edo, which doubles it, provides a good correction to harmonics 5 and 17; while 68edo, which quadruples it, provides additionally the primes 7, 19, and 31.

Intervals

See also: 17edo solfege

#	Cents	Approximate ratios^{[note 1]}	Circle-of-fifths notation^†		Ups and downs notation (EUs: vvA1 and ^d2)			SKULO notation (U = 1)
0	0.0	1/1	Unison	D	unison	P1	D	unison	P1	D
1	70.6	24/23, 25/24, 26/25, 27/26, 28/27	Minor 2nd (Semiaugmented 1sn)	Eb (Dt)	up unison, minor 2nd	^1, m2	Eb	uber unison, minor 2nd	U1, m2	UD, Eb
2	141.2	12/11, 13/12, 14/13, 25/23	Augmented 1sn (Neutral 2nd)	D# (Ed)	augmented 1sn, mid 2nd	A1, ~2	vE	neutral 2nd	N2	UEb, uE
3	211.8	8/7, 9/8, 17/15, 25/22, 26/23	Major 2nd	E	major 2nd	M2	E	major 2nd	M2	E
4	282.4	7/6, 13/11, 20/17	Minor 3rd	F	minor 3rd	m3	F	minor 3rd	m3	F
5	352.9	11/9, 27/22, 16/13, 39/32	Diminished 4th (Neutral 3rd)	Gb (Ft)	mid 3rd	~3	^F	neutral 3rd	N3	UF, uF#
6	423.5	9/7, 14/11, 23/18, 32/25, 51/40	Major 3rd (Semidiminished 4th)	F# (Gd)	major 3rd	M3	F#	major 3rd	M3	F#
7	494.1	4/3, 21/16, 85/64	Perfect 4th	G	perfect 4th	P4	G	perfect 4th	P4	G
8	564.7	11/8, 18/13, 25/18, 32/23	Diminished 5th (Semiaugmented 4th)	Ab (Gt)	mid 4th, diminished 5th	~4, d5	^G, Ab	uber 4th/ neutral 4th	U4/N4	UG
9	635.3	13/9, 16/11, 23/16, 36/25	Augmented 4th (Semidiminished 5th)	G# (Ad)	augmented 4th, mid 5th	A4, ~5	G#, vA	unter 5th/ neutral 5th	u5/N5	uA
10	705.9	3/2, 32/21, 128/85	Perfect 5th	A	perfect 5th	P5	A	perfect 5th	P5	A
11	776.5	11/7, 14/9, 25/16, 36/23, 80/51	Minor 6th (Semiaugmented 5th)	Bb (At)	minor 6th	m6	Bb	minor 6th	m6	Bb
12	847.1	13/8, 18/11, 44/27, 64/39	Augmented 5th (Neutral 6th)	A# (Bd)	mid 6th	~6	vB	neutral 6th	N6	UBb, uB
13	917.6	12/7, 17/10, 22/13	Major 6th	B	major 6th	M6	B	major 6th	M6	B
14	988.2	7/4, 16/9, 23/13, 30/17, 44/25	Minor 7th	C	minor 7th	m7	C	minor 7th	m7	C
15	1058.8	11/6, 13/7, 24/13, 46/25	Diminished 8ve (Neutral 7th)	Db (Ct)	mid 7th	~7	^C	neutral 7th	N7	UC, uC#
16	1129.4	23/12, 25/13, 27/14, 48/25, 52/27	Major 7th (Semidiminished 8ve)	C# (Dd)	major 7th, down 8ve	M7, v8	C#	major 7th, unter octave	M7, u8	C#, uD
17	1200.0	2/1	Octave	D	octave	P8	D	octave	P8	D

^† Half-sharps and half-flats (denoted "t" and "d", respectively) can be used to alter the note by a single step, since sharps and flats each span two edosteps. Using half-sharps and half-flats may be preferable for compatibility with the ups-and-downs notation in 34edo, in which an up or down respectively constitute a quarter-sharp or quarter-flat.

Interval quality and chord names in color notation

Combining ups and downs notation with color notation, qualities can be loosely associated with colors:

Quality	Color	Monzo format	Examples
minor	zo	(a, b, 0, 1)	7/6, 7/4
minor	fourthward wa	(a, b), b < -1	32/27, 16/9
mid	ilo	(a, b, 0, 0, 1)	11/9, 11/6
mid	lu	(a, b, 0, 0, -1)	12/11, 18/11
major	fifthward wa	(a, b), b > 1	9/8, 27/16
major	ru	(a, b, 0, -1)	9/7, 12/7

All 17edo chords can be named using ups and downs. Here are the zo, ilo and ru triads:

Color of the 3rd	JI chord	Notes as edosteps	Notes of C chord	Written name	Spoken name
zo	6:7:9	0-4-10	C Eb G	Cm	C minor
ilo	18:22:27	0-5-10	C vE G	C~	C mid
ru	14:18:21	0-6-10	C E G	C	C major or C

Alterations are always enclosed in parentheses, additions never are. An up, down or mid immediately after the chord root affects the 3rd, 6th, 7th, and/or the 11th (every other note of a stacked-3rds chord 6-1-3-5-7-9-11-13).

0-4-9 = C Eb vG = Cm(v5) = C minor down-five

0-5-9 = C vE vG = C~(v5) = C mid down-five

0-6-11 = C E ^G = C(^5) = C up-five

0-4-10-14 = C Eb G Bb = Cm7 = C minor seven

0-5-10-14 = C vE G Bb = C~,7 = C mid add seven

0-6-10-15 = C E G vB = C,~7 = C add mid-seven

0-5-10-15 = C vE G vB = C~7 = C mid-seven

For a more complete list, see Ups and downs notation #Chords and chord progressions.

Notation

Ups and downs notation

Spoken as up, sharp, upsharp, etc. Note that up can be respelled as downsharp. The gamut runs D, ^D/Eb, D#/vE, E, F etc.

Step offset	−4	−3	−2	−1	0	+1	+2	+3	+4
Symbol

Quarter tone notation

Since a sharp raises by 2 steps, 17edo can be notated using quarter-tone accidentals.

Step offset	−4	−3	−2	−1	0	+1	+2	+3	+4
Symbol

Sagittal notation

This notation uses the same sagittal sequence as edos 24, 31, and 38, and is a subset of the notation for 34edo.

Evo and Revo flavors

Alternative Evo flavor

Evo-SZ flavor

Because it contains no Sagittal symbols, this Evo-SZ Sagittal notation is identical to the Stein-Zimmerman notation.

Sagittal songbook diagram

From the appendix to The Sagittal Songbook by Jacob A. Barton, a diagram of how to notate 17edo in the Revo flavor of Sagittal:

3L 4s (mosh) notation

The notation of Neutral[7]. The generator is the perfect 3rd. Notes are denoted as sLsLsLs = DEFGABCD, and raising and lowering by a chroma (L − s), 1 edostep in this instance, is denoted by ♯ and ♭.

#	Cents	Note	Name	Associated ratios
0	0.0	D	Perfect 1sn	1/1
1	70.6	D#	Augmented 1sn	33/32
2	141.2	Eb	Minor 2nd	12/11
3	211.8	E	Major 2nd	9/8
4	282.4	Fb	Diminished 3rd	32/27
5	352.9	F	Perfect 3rd	11/9, 27/22
6	423.5	F#	Augmented 3rd	81/64
7	494.1	G	Minor 4th	4/3
8	564.7	G#	Major 4th	11/8
9	635.3	Ab	Minor 5th	16/11
10	705.9	A	Major 5th	3/2
11	776.5	Bb	Diminished 6th	128/81
12	847.1	B	Perfect 6th	18/11, 44/27
13	917.6	B#	Augmented 6th	27/16
14	988.2	Cb	Minor 7th	16/9
15	1058.8	C	Major 7th	11/6
16	1129.4	Db	Diminished 8ve	64/33
17	1200.0	D	Perfect 8ve	2/1

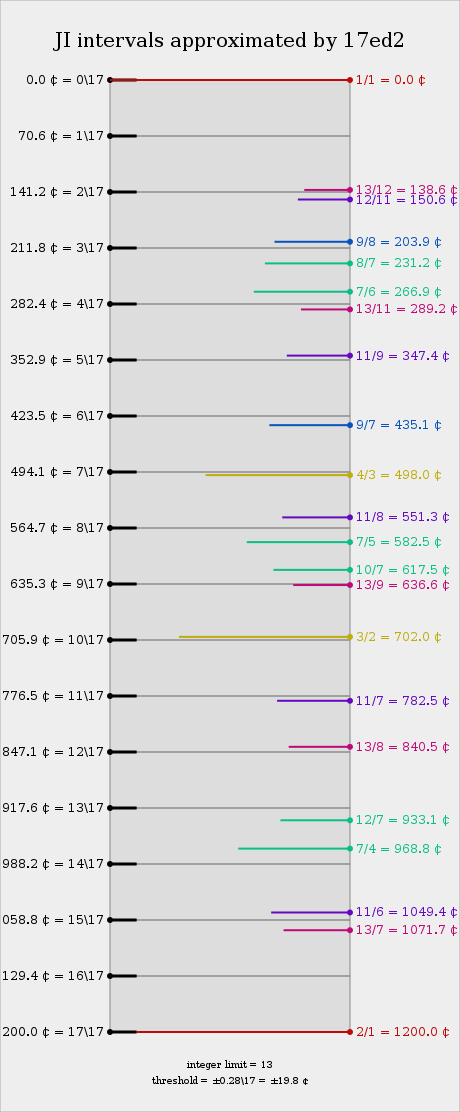
Approximation to JI

15-odd-limit interval mappings

The following tables show how 15-odd-limit intervals are represented in 17edo. Prime harmonics are in bold; inconsistent intervals are in italics.

15-odd-limit intervals in 17edo (direct approximation, even if inconsistent)
Interval and complement	Error (abs, ¢)	Error (rel, %)
1/1, 2/1	0.000	0.0
13/9, 18/13	1.324	1.9
13/12, 24/13	2.604	3.7
3/2, 4/3	3.927	5.6
11/9, 18/11	5.533	7.8
11/7, 14/11	6.021	8.5
13/8, 16/13	6.531	9.3
13/11, 22/13	6.857	9.7
9/8, 16/9	7.855	11.1
11/6, 12/11	9.461	13.4
9/7, 14/9	11.555	16.4
13/7, 14/13	12.878	18.2
11/8, 16/11	13.388	19.0
7/6, 12/7	15.482	21.9
7/5, 10/7	17.806	25.2
7/4, 8/7	19.409	27.5
15/14, 28/15	21.734	30.8
11/10, 20/11	23.828	33.8
15/11, 22/15	27.755	39.3
9/5, 10/9	29.361	41.6
15/8, 16/15	29.445	41.7
13/10, 20/13	30.685	43.5
5/3, 6/5	33.288	47.2
5/4, 8/5	33.373	47.3
15/13, 26/15	34.612	49.0

15-odd-limit intervals in 17edo (patent val mapping)
Interval and complement	Error (abs, ¢)	Error (rel, %)
1/1, 2/1	0.000	0.0
13/9, 18/13	1.324	1.9
13/12, 24/13	2.604	3.7
3/2, 4/3	3.927	5.6
11/9, 18/11	5.533	7.8
11/7, 14/11	6.021	8.5
13/8, 16/13	6.531	9.3
13/11, 22/13	6.857	9.7
9/8, 16/9	7.855	11.1
11/6, 12/11	9.461	13.4
9/7, 14/9	11.555	16.4
13/7, 14/13	12.878	18.2
11/8, 16/11	13.388	19.0
7/6, 12/7	15.482	21.9
7/4, 8/7	19.409	27.5
15/8, 16/15	29.445	41.7
5/4, 8/5	33.373	47.3
15/13, 26/15	35.976	51.0
5/3, 6/5	37.300	52.8
13/10, 20/13	39.904	56.5
9/5, 10/9	41.227	58.4
15/11, 22/15	42.833	60.7
11/10, 20/11	46.760	66.2
15/14, 28/15	48.855	69.2
7/5, 10/7	52.782	74.8

15-odd-limit intervals by 17c val mapping
Interval and complement	Error (abs, ¢)	Error (rel, %)
1/1, 2/1	0.000	0.0
13/9, 18/13	1.324	1.9
13/12, 24/13	2.604	3.7
3/2, 4/3	3.927	5.6
11/9, 18/11	5.533	7.8
11/7, 14/11	6.021	8.5
13/8, 16/13	6.531	9.3
13/11, 22/13	6.857	9.7
9/8, 16/9	7.855	11.1
11/6, 12/11	9.461	13.4
9/7, 14/9	11.555	16.4
13/7, 14/13	12.878	18.2
11/8, 16/11	13.388	19.0
7/6, 12/7	15.482	21.9
7/5, 10/7	17.806	25.2
7/4, 8/7	19.409	27.5
15/14, 28/15	21.734	30.8
11/10, 20/11	23.828	33.8
15/11, 22/15	27.755	39.3
9/5, 10/9	29.361	41.6
13/10, 20/13	30.685	43.5
5/3, 6/5	33.288	47.2
15/13, 26/15	34.612	49.0
5/4, 8/5	37.216	52.7
15/8, 16/15	41.143	58.3

Selected 13-limit intervals

Zeta peak index

Tuning			Strength				Octave (cents)		Integer limit
ZPI	Steps per 8ve	Step size (cents)	Height		Integral	Gap	Size	Stretch	Consistent	Distinct
ZPI	Steps per 8ve	Step size (cents)	Tempered	Pure	Integral	Gap	Size	Stretch	Consistent	Distinct
56zpi	17.044589	70.403576	5.056957	4.528893	1.032175	14.269437	1196.860799	−3.139201	4	4

Tuning by ear

17edo is very close to a circle of seventeen 25/24 chromatic semitones: (25/24)¹⁷ is only 1.43131 cents sharp of an octave. This means that if you can tune seventeen 25/24's accurately (by say, tuning 5/4 up, 3/2 down and 5/4 up, taking care to minimize the error at each step), you have a shot at approximating 17edo within melodic just noticeable difference.

Regular temperament properties

Subgroup	Comma list	Mapping	Optimal 8ve stretch (¢)	Tuning error
Subgroup	Comma list	Mapping	Optimal 8ve stretch (¢)	Absolute (¢)	Relative (%)
2.3	[27 -17⟩	[⟨17 27]]	−1.24	1.24	1.76
2.3.7	64/63, 17496/16807	[⟨17 27 48]]	−3.13	2.85	4.05
2.3.7.11	64/63, 99/98, 243/242	[⟨17 27 48 59]]	−3.31	2.49	3.54
2.3.7.11.13	64/63, 78/77, 99/98, 144/143	[⟨17 27 48 59 63]]	−3.00	2.31	3.28

17et is lower in relative error than any previous equal temperaments in the no-5 11- and 13-limit. The next equal temperaments doing better in these subgroups are 41 and 207, respectively.

Uniform maps

13-limit uniform maps between 16.8 and 17.2
Min. size	Max. size	Wart notation	Map
16.7196	16.8899	17def	⟨17 27 39 47 58 62]
16.8899	16.9103	17de	⟨17 27 39 47 58 63]
16.9103	16.9198	17d	⟨17 27 39 47 59 63]
16.9198	17.0117	17	⟨17 27 39 48 59 63]
17.0117	17.1601	17c	⟨17 27 40 48 59 63]
17.1601	17.1994	17cff	⟨17 27 40 48 59 64]
17.1994	17.2760	17ceeff	⟨17 27 40 48 60 64]

Commas

17et tempers out the following commas. (Note: This assumes patent val ⟨17 27 39 48 59 63 69], cent values rounded to 5 digits.)

Prime limit	Ratio^[1]	Monzo	Cents	Color name	Name
3	(18 digits)	[27 -17⟩	66.765	Sasawa	17-comma
5	25/24	[-3 -1 2⟩	70.762	Yoyo	Dicot comma
5	32805/32768	[-15 8 1⟩	1.9537	Layo	Schisma
7	525/512	[-9 1 2 1⟩	43.408	Lazoyoyo	Avicennma
7	64/63	[6 -2 0 -1⟩	27.264	Ru	Septimal comma
7	245/243	[0 -5 1 2⟩	14.191	Zozoyo	Sensamagic comma
7	1728/1715	[6 3 -1 -3⟩	13.074	Triru-agu	Orwellisma
7	(12 digits)	[-6 -8 2 5⟩	1.1170	Quinzo-ayoyo	Wizma
11	99/98	[-1 2 0 -2 1⟩	17.576	Loruru	Mothwellsma
11	896/891	[7 -4 0 1 -1⟩	9.6880	Saluzo	Pentacircle comma
11	243/242	[-1 5 0 0 -2⟩	7.1391	Lulu	Rastma
11	385/384	[-7 -1 1 1 1⟩	4.5026	Lozoyo	Keenanisma
13	1352/1331	[3 0 0 0 -3 2⟩	27.101	Bithotrilu	Lovecraft comma
13	364/363	[2 -1 0 1 -2 1⟩	4.763	Tholuluzo	Minor minthma
17	136/135	[3 -3 -1 0 0 0 1⟩	12.776	Sogu 2nd	Diatisma

Note that despite their relatively large size, the 17-comma, the avicennma and the chromatic semitone are all tempered out by the 13-limit patent val, as stated.

Rank-2 temperaments

Table of rank-2 temperaments by generator
Periods per 8ve	Generator	Cents	Associated ratio	Temperament
1	2\17	141.18	13/12	Bleu / progression (17c) / glacier
1	3\17	211.76	8/7~9/8	Machine
1	4\17	282.35	13/11	Huxley / lovecraft / subklei (17c)
1	5\17	352.94	11/9	Suhajira / neutrominant (17c) / beatles (17c) / dicotic (17) Hemif / mohamaq (17c) / salsa (17)
1	6\17	423.53	9/7	Skwares / squares (17c) / sentinel (17) / sidi (17)
1	7\17	494.12	4/3	Archy / supra / quasisuper (17c) / dominant (17c) / superpyth (17) / schism (17) Fiventeen
1	8\17	564.71	7/5	Lee / liese (17c) / pycnic (17) Progress (17c)

Scales

Antipental blues: 4 3 1 2 4 3
Blues Peruvian: 4 3 1 1 1 4 3
Hydra: 3 3 1 1 2 3 2 1 1
Husayni Ascending: 2 2 3 3 2 2 3
Otonal 17: 3 2 3 2 2 2 3
Scorp: 3 2 3 1 3 2 3
Screamapillar: 3 3 2 2 3 3 1
sLmLs: 2 5 3 5 2

MOS scales

Main article: MOS scales of 17edo

diatonic (leapfrog/archy) 5L 2s 3 3 3 1 3 3 1 (10\17, 1\1)
neutrominant 3L 4s 3 2 3 2 3 2 2 (5\17, 1\1)
neutrominant 7L 3s 2 2 2 1 2 2 1 2 2 1 (5\17, 1\1)
squares 3L 5s 1 1 4 1 4 1 4 (6\17, 1\1)
squares 3L 8s 1 3 1 1 3 1 1 3 (6\17, 1\1)
lovecraft 4L 5s 3 1 3 1 3 1 3 1 1 (4\17, 1\1)

Well temperaments

Introductory materials

SeventeenTheory, an introduction to 17edo theory, through the eyes of the SeventeenTonePianoProject.
The 17-tone Puzzle by George Secor, another introduction into 17edo theory.
17edo tetrachords
Proyect 17-Perú ^[forbidden]

Music

Main article: 17edo/Music

See also: Category:17edo tracks

YouTube playlist of 17edo pieces
YouTube videos tagged with 17edo

Compositions from the Seventeen Tone Piano Project

Instruments

17 note per octave conversion from a "standard" Stratocaster copy - conversion by Brad Smith

17edo soprano Harmony ukulele with a 3D printed fretboard - conversion by Tristan Bay

Notes

↑ Based on treating 17edo as a 2.3.25.7.11.13.85.23 subgroup temperament; other approaches are also possible.

↑ Ratios longer than 10 digits are presented by placeholders with informative hints

[1] Based on treating 17edo as a 2.3.25.7.11.13.85.23 subgroup temperament; other approaches are also possible.

[2] Ratios longer than 10 digits are presented by placeholders with informative hints

[note 1]

[1]

17edo

Contents