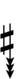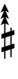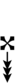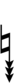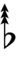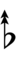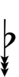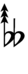63edo

← 62edo

63edo

64edo →

Prime factorization	32 × 7
Step size	19.0476 ¢
Fifth	37\63 (704.762 ¢)
Semitones (A1:m2)	7:4 (133.3 ¢ : 76.19 ¢)
Consistency limit	7
Distinct consistency limit	7

63 equal divisions of the octave (abbreviated 63edo or 63ed2), also called 63-tone equal temperament (63tet) or 63 equal temperament (63et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 63 equal parts of about 19 ¢ each. Each step represents a frequency ratio of 2^1/63, or the 63rd root of 2.

Theory

63edo is almost consistent to the 15-odd-limit; the only inconsistency is that 10/9 is mapped to 9\63 (1\7, the same as what 11/10 is mapped to consistently) so that it is almost 11 ¢ out of tune. This corresponds to 63edo exaggerating the syntonic comma, 81/80, to two steps, so that it finds a somewhat flat mean-tone between ~10/9 and ~9/8.

As an equal temperament, it tempers out 3125/3072 in the 5-limit and 225/224, 245/243, and 875/864 in the 7-limit, so that it supports magic temperament. In the 11-limit it tempers out 100/99, supporting 11-limit magic, plus 385/384 and 540/539, 896/891. In the 13-limit it tempers out 169/168, 275/273, 640/637, 352/351, 364/363 and 676/675. It provides the optimal patent val for immune, the 29 & 34d temperament in the 7-, 11- and 13-limit.

63 is also a fascinating division to look at in the 47-limit. Although it does not deal as well with primes 5, 17, 19, 37 and 41, it excels in the 2.3.7.11.13.23.29.31.43.47 subgroup, and is a great candidate for a gentle tuning. Its regular augmented fourth (+6 fifths) is less than 0.3 cents sharp of 23/16, therefore tempering out 736/729. Its diesis (+12 fifths) can represent 33/32, 32/31, 30/29, 29/28, 28/27, as well as 91/88, and more, so it is very versatile, making chains of fifths of 12 tones or longer very useful in covering harmonic and melodic ground while providing a lot of different colour in different keys. We can take advantage of the representation of 27:28:29:30:31:32:33, which splits 11/9 into six "small dieses" as a result; here it can be seen more clearly why these are not regular quarter-tones so are best distinguished from such with the qualifier "large", as otherwise we would expect to see some flavour of minor third after six of them.

A 17-tone fifths chain looks on the surface a little similar to 17edo, but as −17 fifths gets us to 64/63, observing the comma becomes an essential part in progressions favouring prime 7. Furthermore, its prime 5 is far from unusable; although 25/16 is barely inconsistent, this affords the tuning supporting 7-limit magic, which may be considered interesting or desirable in of itself. And if this was not enough, if you really want to, it offers reasonable approximations to some yet higher primes too; namely 43/32, 47/32, and 53/32; see the tables below.

Prime harmonics

Approximation of prime harmonics in 63edo

Harmonic		2	3	5	7	11	13	17	19	23	29	31	37
Error	Absolute (¢)	+0.00	+2.81	-5.36	+2.60	+1.06	-2.43	+9.33	+7.25	+0.30	-1.01	-2.18	-3.72
	Relative (%)	+0.0	+14.7	-28.1	+13.7	+5.6	-12.8	+49.0	+38.1	+1.6	-5.3	-11.4	-19.6
Steps (reduced)		63 (0)	100 (37)	146 (20)	177 (51)	218 (29)	233 (44)	258 (6)	268 (16)	285 (33)	306 (54)	312 (60)	328 (13)

Approximation of prime harmonics in 63edo (continued)

Harmonic		41	43	47	53	59	61	67	71	73	79	83	89
Error	Absolute (¢)	+9.03	+2.77	+1.16	+2.69	+7.50	+6.92	-3.12	-8.27	+0.78	-2.63	+7.10	+0.55
	Relative (%)	+47.4	+14.5	+6.1	+14.1	+39.3	+36.4	-16.4	-43.4	+4.1	-13.8	+37.3	+2.9
Steps (reduced)		338 (23)	342 (27)	350 (35)	361 (46)	371 (56)	374 (59)	382 (4)	387 (9)	390 (12)	397 (19)	402 (24)	408 (30)

Subsets and supersets

Since 63 factors into primes as 3² × 7, 63edo has subset edos 3, 7, 9, and 21.

Its representation of the 2.3.5.7.13 subgroup (no-11's 13-limit) can uniquely be described in terms of accurate approximations contained in its main subsets of 7edo and 9edo:

• 1\9 = 14/13~13/12, implying the much more accurate 2\9 = ~7/6 (septiennealic)
• 2\7 = 39/32~128/105, via 4096/4095 and the akjaysma (which are naturally paired)

If we avoid equating 14/13 and 13/12 (which is by far the highest damage equivalence) so that we achieve 7/6 = 2\9 directly, we get the 63 & 441 microtemperament in the same subgroup.

Intervals

Degree	Cents	Approximate ratios*
0	0.0	1/1
1	19.0	50/49, 55/54, 64/63, 65/64, 91/90, 105/104
2	38.1	45/44, 46/45, 49/48, 56/55, 66/65, 81/80
3	57.1	25/24, 28/27, 29/28, 30/29, 31/30, 32/31, 33/32, 36/35
4	76.2	22/21, 23/22, 24/23, 26/25, 27/26
5	95.2	21/20, 35/33
6	114.3	15/14, 16/15
7	133.3	13/12, 14/13
8	152.4	12/11
9	171.4	10/9, 11/10, 31/28, 32/29
10	190.5	29/26, 39/35, 49/44
11	209.5	9/8
12	228.6	8/7
13	247.6	15/13
14	266.7	7/6
15	285.7	13/11
16	304.8	31/26
17	323.8	6/5
18	342.9	11/9, 28/23, 39/32
19	361.9	16/13, 26/21, 27/22
20	381.0	5/4
21	400.0	29/23, 44/35, 49/39
22	419.0	14/11
23	438.1	9/7
24	457.1	13/10
25	476.2	21/16
26	495.2	4/3
27	514.3	35/26
28	533.3	15/11, 27/20
29	552.4	11/8
30	571.4	18/13, 32/23
31	590.5	7/5
32	609.5	10/7
33	628.6	13/9, 23/16
34	647.6	16/11
35	666.7	22/15
36	685.7	52/35
37	704.8	3/2
38	723.8	32/21
39	742.9	20/13
40	761.9	14/9
41	781.0	11/7
42	800.0	35/22, 46/29
43	819.0	8/5
44	838.1	13/8, 21/13, 44/27
45	857.1	18/11, 23/14, 64/39
46	876.2	5/3
47	895.2	52/31
48	914.3	22/13
49	933.3	12/7
50	952.4	26/15
51	971.4	7/4
52	990.5	16/9
53	1009.5	52/29, 70/39, 88/49
54	1028.6	9/5, 20/11, 29/16, 56/31
55	1047.6	11/6
56	1066.7	13/7, 24/13
57	1085.7	15/8, 28/15
58	1104.8	40/21, 66/35
59	1123.8	21/11, 23/12, 25/13, 44/23, 52/27
60	1142.9	27/14, 29/15, 31/16, 35/18, 48/25, 56/29, 60/31, 64/33
61	1161.9	45/23, 55/28, 88/45, 96/49, 160/81
62	1181.0	49/25, 63/32, 65/33, 108/55, 180/91, 208/105
63	1200.0	2/1

* As a 2.3.5.7.11.13.23.29.31-subgroup (no-17 no-19 31-limit) temperament, inconsistent intervals in italics

See the below section for a machine-generated table including higher-limit ratios selected with a mind towards higher accuracy.

Higher-accuracy interpretations

The following table was created using Godtone's code with the command interpret_edo(63,ol=53,no=[5,17,19,25,27,37,41,51],add=[73,75,87,89,91,93,105],dec="''",wiki=23) (run in a Python 3 interactive console) plus manual correction of the order of some inconsistent intervals, removal of unsimplified intervals of 75, and adding of (the inconsistent but simple) 10/9, 21/20 and their octave-complements.

As the command and description indicates, it is a(n accurate) "no-5's"* no-17's no-19's no-25's no-27's no-37's no-41's 49-odd-limit add-53 add-63 add-73 add-87 add-89 add-91 add-93 add-105 interpretation, tuned to the strengths of 63edo. * Note that because of the cancellation of factors, some odd harmonics of 5 (the simpler/more relevant ones) are present, EG 75/3 = 25, 45/3 = 15, 105/75 = 7/5, 75/35/2 = 15/14, and 45/9 = 5, so it isn't really "no-5's", just has a de-emphasized focus.

Intervals are listed in order of size, so that one can know their relative order at a glance and deem the value of the interpretation for a harmonic context, and 23-limit intervals are highlighted for navigability as 13-limit intervals are more likely to already have pages, and as we are excluding primes 17 and 19, we are only adding prime 23 to the 13-limit.

Inconsistent intervals are in italics.

Degree	Cents	Approximate ratios
0	0.0	1/1
1	19.05	106/105, 105/104, 94/93, 93/92, 92/91, 91/90, 90/89, 89/88, 88/87, 87/86, 73/72, 65/64, 64/63
2	38.1	66/65, 53/52, 49/48, 48/47, 47/46, 93/91, 46/45, 91/89, 45/44, 89/87, 44/43, 43/42
3	57.14	36/35, 33/32, 32/31, 94/91, 31/30, 92/89, 91/88, 30/29, 89/86, 29/28, 25/24
4	76.19	26/25, 49/47, 73/70, 24/23, 47/45, 93/89, 23/22, 91/87, 45/43, 22/21
5	95.24	21/20, 98/93, 96/91, 94/89, 56/53, 93/88, 92/87, 91/86, 89/84, 35/33, 52/49
6	114.29	33/31, 49/46, 16/15, 47/44, 78/73, 31/29, 46/43, 15/14
7	133.33	14/13, 96/89, 94/87, 93/86, 53/49, 13/12
8	152.38	49/45, 12/11, 47/43, 35/32, 58/53, 23/21
9	171.43	11/10, 98/89, 43/39, 32/29, 53/48, 116/105, 73/66, 52/47, 31/28, 10/9
10	190.48	49/44, 39/35, 29/26, 48/43, 105/94, 104/93, 47/42
11	209.52	28/25, 9/8, 98/87, 53/47, 44/39, 35/31, 26/23, 60/53, 25/22
12	228.57	33/29, 49/43, 106/93, 73/64, 89/78, 105/92, 8/7
13	247.62	84/73, 53/46, 15/13, 52/45
14	266.67	29/25, 36/31, 106/91, 7/6, 104/89, 62/53
15	285.71	73/62, 53/45, 86/73, 33/28, 46/39, 105/89, 124/105, 13/11, 58/49
16	304.76	106/89, 56/47, 87/73, 31/26, 105/88, 43/36, 104/87
17	323.81	6/5, 112/93, 53/44, 47/39, 88/73, 35/29, 64/53, 29/24, 52/43
18	342.86	73/60, 28/23, 106/87, 39/32, 128/105, 89/73, 105/86, 11/9, 60/49
19	361.9	43/35, 16/13, 53/43, 90/73, 58/47, 89/72, 26/21
20	380.95	31/25, 36/29, 87/70, 56/45, 66/53, 91/73, 116/93, 5/4
21	400.0	49/39, 44/35, 39/31, 112/89, 73/58, 92/73, 29/23, 53/42, 91/72, 62/49
22	419.05	33/26, 89/70, 14/11, 93/73, 116/91, 60/47, 23/18
23	438.1	32/25, 9/7, 112/87, 94/73, 58/45, 40/31, 31/24
24	457.14	13/10, 56/43, 43/33, 116/89, 73/56, 30/23, 47/36, 64/49
25	476.19	21/16, 46/35, 96/73, 29/22, 120/91, 62/47, 70/53
26	495.24	93/70, 4/3
27	514.29	98/73, 47/35, 43/32, 39/29, 35/26, 66/49, 31/23, 120/89, 89/66, 58/43
28	533.33	42/31, 72/53, 53/39, 87/64, 49/36, 64/47, 124/91, 15/11
29	552.38	48/35, 11/8, 128/93, 73/53, 62/45, 91/66, 40/29, 29/21
30	571.43	18/13, 43/31, 146/105, 89/64, 32/23, 39/28, 124/89, 46/33, 60/43
31	590.48	7/5, 87/62, 73/52, 66/47, 45/32, 128/91, 31/22
32	609.52	44/31, 91/64, 64/45, 47/33, 104/73, 124/87, 10/7
33	628.57	43/30, 33/23, 89/62, 56/39, 23/16, 128/89, 105/73, 62/43, 13/9
34	647.62	42/29, 29/20, 132/91, 45/31, 106/73, 93/64, 16/11, 35/24
35	666.67	22/15, 91/62, 47/32, 72/49, 128/87, 78/53, 53/36, 31/21
36	685.71	43/29, 132/89, 89/60, 46/31, 49/33, 52/35, 58/39, 64/43, 70/47, 73/49
37	704.76	3/2, 140/93
38	723.81	53/35, 47/31, 91/60, 44/29, 73/48, 35/23, 32/21
39	742.86	49/32, 72/47, 23/15, 112/73, 89/58, 66/43, 43/28, 20/13
40	761.9	48/31, 31/20, 45/29, 73/47, 87/56, 14/9, 25/16
41	780.95	36/23, 47/30, 91/58, 146/93, 11/7, 140/89, 52/33
42	800.0	49/31, 144/91, 84/53, 46/29, 73/46, 116/73, 89/56, 62/39, 35/22, 78/49
43	819.05	8/5, 93/58, 146/91, 53/33, 45/28, 140/87, 29/18, 50/31
44	838.1	21/13, 144/89, 47/29, 73/45, 86/53, 13/8, 70/43
45	857.14	49/30, 18/11, 172/105, 146/89, 105/64, 64/39, 87/53, 23/14, 120/73
46	876.19	43/26, 48/29, 53/32, 58/35, 73/44, 78/47, 88/53, 93/56, 5/3
47	895.24	87/52, 72/43, 176/105, 52/31, 146/87, 47/28, 89/53
48	914.29	49/29, 22/13, 105/62, 178/105, 39/23, 56/33, 73/43, 90/53, 124/73
49	933.33	53/31, 89/52, 12/7, 91/53, 31/18, 50/29
50	952.38	45/26, 26/15, 92/53, 73/42
51	971.43	7/4, 184/105, 156/89, 128/73, 93/53, 86/49, 58/33
52	990.48	44/25, 53/30, 23/13, 62/35, 39/22, 94/53, 87/49, 16/9, 25/14
53	1009.52	84/47, 93/52, 188/105, 43/24, 52/29, 70/39, 88/49
54	1028.57	9/5', 56/31, 47/26, 132/73, 105/58, 96/53, 29/16, 78/43, 89/49, 20/11
55	1047.62	42/23, 53/29, 64/35, 86/47, 11/6, 90/49
56	1066.67	24/13, 98/53, 172/93, 87/47, 89/48, 13/7
57	1085.71	28/15, 43/23, 58/31, 73/39, 88/47, 15/8, 92/49, 62/33
58	1104.76	49/26, 66/35, 168/89, 172/91, 87/46, 176/93, 53/28, 89/47, 91/48, 93/49, 40/21
59	1123.81	21/11, 86/45, 174/91, 44/23, 178/93, 90/47, 23/12, 140/73, 94/49, 25/13
60	1142.86	48/25, 56/29, 172/89, 29/15, 176/91, 89/46, 60/31, 91/47, 31/16, 64/33, 35/18
61	1161.9	84/43, 43/22, 174/89, 88/45, 178/91, 45/23, 182/93, 92/47, 47/24, 96/49, 104/53
62	1180.95	63/32, 144/73, 172/87, 87/44, 176/89, 89/45, 180/91, 91/46, 184/93, 93/47, 208/105, 105/53
63	1200.0	2/1

Notation

Ups and downs notation

63edo can be notated using ups and downs. Trup is equivalent to quudsharp, trudsharp is equivalent to quup, etc.

Step offset	0	1	2	3	4	5	6	7	8	9	10	11	12	13	14
Sharp symbol
Flat symbol

Alternatively, sharps and flats with arrows borrowed from Helmholtz–Ellis notation can be used:

Step offset	0	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15	16	17
Sharp symbol
Flat symbol

Sagittal notation

This notation uses the same sagittal sequence as 56edo.

Evo flavor

Revo flavor

Approximation to JI

Interval mappings

The following tables show how 15-odd-limit intervals are represented in 63edo. Prime harmonics are in bold; inconsistent intervals are in italics.

15-odd-limit intervals in 63edo (direct approximation, even if inconsistent)

Interval and complement	Error (abs, ¢)	Error (rel, %)
1/1, 2/1	0.000	0.0
15/13, 26/15	0.122	0.6
7/6, 12/7	0.204	1.1
11/8, 16/11	1.063	5.6
11/7, 14/11	1.540	8.1
11/6, 12/11	1.744	9.2
13/8, 16/13	2.432	12.8
15/8, 16/15	2.554	13.4
7/4, 8/7	2.603	13.7
3/2, 4/3	2.807	14.7
13/10, 20/13	2.929	15.4
9/7, 14/9	3.011	15.8
13/11, 22/13	3.495	18.4
15/11, 22/15	3.617	19.0
11/9, 18/11	4.551	23.9
13/7, 14/13	5.035	26.4
15/14, 28/15	5.157	27.1
13/12, 24/13	5.239	27.5
5/4, 8/5	5.361	28.1
9/8, 16/9	5.614	29.5
11/10, 20/11	6.424	33.7
7/5, 10/7	7.964	41.8
13/9, 18/13	8.046	42.2
9/5, 10/9	8.072	42.4
5/3, 6/5	8.168	42.9

15-odd-limit intervals in 63edo (patent val mapping)

Interval and complement	Error (abs, ¢)	Error (rel, %)
1/1, 2/1	0.000	0.0
15/13, 26/15	0.122	0.6
7/6, 12/7	0.204	1.1
11/8, 16/11	1.063	5.6
11/7, 14/11	1.540	8.1
11/6, 12/11	1.744	9.2
13/8, 16/13	2.432	12.8
15/8, 16/15	2.554	13.4
7/4, 8/7	2.603	13.7
3/2, 4/3	2.807	14.7
13/10, 20/13	2.929	15.4
9/7, 14/9	3.011	15.8
13/11, 22/13	3.495	18.4
15/11, 22/15	3.617	19.0
11/9, 18/11	4.551	23.9
13/7, 14/13	5.035	26.4
15/14, 28/15	5.157	27.1
13/12, 24/13	5.239	27.5
5/4, 8/5	5.361	28.1
9/8, 16/9	5.614	29.5
11/10, 20/11	6.424	33.7
7/5, 10/7	7.964	41.8
13/9, 18/13	8.046	42.2
5/3, 6/5	8.168	42.9
9/5, 10/9	10.975	57.6

Zeta peak index

Tuning			Strength				Octave (cents)		Integer limit
ZPI	Steps per 8ve	Step size (cents)	Height		Integral	Gap	Size	Stretch	Consistent	Distinct
			Tempered	Pure
321zpi	63.019289	19.041789	6.768662	6.534208	1.049023	15.41292	1199.632711	−0.367289	8	8

Regular temperament properties

Subgroup	Comma list	Mapping	Optimal 8ve stretch (¢)	Tuning error
				Absolute (¢)	Relative (%)
2.3	[100 -63⟩	[⟨63 100]]	-0.885	0.885	4.65
2.3.5	3125/3072, 1638400/1594323	[⟨63 100 146]]	+0.177	1.67	8.77
2.3.5.7	225/224, 245/243, 51200/50421	[⟨63 100 146 177]]	-0.099	1.52	8.00
2.3.5.7.11	100/99, 225/224, 245/243, 1331/1323	[⟨63 100 146 177 218]]	-0.141	1.37	7.17
2.3.5.7.11.13	100/99, 169/168, 225/224, 245/243, 275/273	[⟨63 100 146 177 218 233]]	-0.008	1.28	6.73

Rank-2 temperaments

Table of rank-2 temperaments by generator

Periods per 8ve	Generator*	Cents*	Associated ratio*	Temperament
1	2\63	38.10	49/48	Slender
1	13\63	247.62	15/13	Immune
1	19\63	361.90	16/13	Submajor
1	20\63	380.95	5/4	Magic
1	25\63	476.19	21/16	Subfourth
3	26\63 (5\63)	495.24 (95.24)	4/3 (21/20)	Fog
7	26\63 (1\63)	495.24 (19.05)	4/3 (81/80)	Sevond
9	13\63 (1\63)	247.62 (19.05)	15/13 (99/98)	Enneaportent

* Octave-reduced form, reduced to the first half-octave, and minimal form in parentheses if distinct

Scales

• Approximation of Pelog lima: 6 9 21 6 21
• Timeywimey (original/default tuning): 16 10 7 4 11 5 10
• Sandcastle (original/default tuning): 8 10 8 11 8 8 10

Instruments

• Skip fretting system 63 3 17

Music

Cam Taylor

• Improvisation in 12-tone fifths chain (2015)
• those early dreams (2016)
• early dreams 2 (2016)
• Seconds and Otonal Shifts (2016)