5edo
| ← 4edo | 5edo | 6edo → |
(convergent)
5 equal divisions of the octave (abbreviated 5edo or 5ed2), also called 5-tone equal temperament (5tet) or 5 equal temperament (5et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 5 equal parts of exactly 240 ¢ each. Each step represents a frequency ratio of 21/5, or the 5th root of 2.
5edo is notable for being the smallest edo containing xenharmonic intervals—1edo, 2edo, 3edo, and 4edo are all subsets of 12edo.
Theory
5edo is the basic example of an equipentatonic scale, containing a sharp but usable perfect fifth, and can be seen as a simplified form of the familiar pentic scale. Tertian harmony is possible in 5edo, but barely: the only chords available are suspended chords, which may also be seen as inframinor (very flat minor) and ultramajor (very sharp major) chords, due to how sharp the fifth is. As a result, many triads will share the same three notes, so rootedness is much more important to explicitly establish.
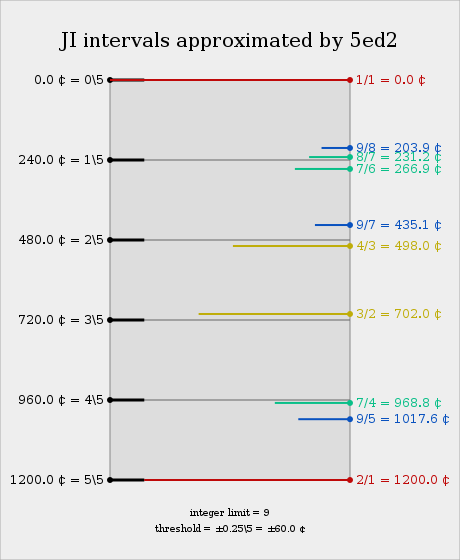
In terms of just intonation, 5edo represents the perfect fifth 3/2 and harmonic seventh 7/4 rather accurately for how wide the steps are, with 3 being about 20 cents sharp, and 7 being about 10 cents flat. In 5edo, the perfect fifth is 3 steps, meaning it can be divided into 3 equal parts, each representing the supermajor second 8/7. This is slendric temperament. Two of these parts make the perfect fourth 4/3, which is semaphore temperament, and finally the harmonic seventh may be found by going up two perfect fourths, which is superpyth or "archy" temperament. This all means that 5edo contains a very simplified form of the 2.3.7 subgroup, and many scales in 2.3.7 take a pentatonic form.
With more complex intervals, however, 5edo becomes increasingly inaccurate. For example, the supermajor third 9/7 is the same interval as the perfect fourth, which is a rather inaccurate equivalence (specifically, trienstonic temperament). However, this can still be used as a third, as referenced in the top paragraph.
If we extend our scope to the full 7-limit (including 5, and thus conventional major and minor thirds), then the most salient fact is that the best approximation of the major third 5/4 is extremely inaccurate, almost a full semitone sharper than just. This results in 5edo supporting several exotemperaments when intervals of 5 are introduced. For example, the best 5/4 of 480 cents is in fact the same interval as 4/3, meaning that the semitone that usually separates them, 16/15, is tempered out (which is the very inaccurate father temperament).
Exploring more complex intervals, we find that the minor tone 10/9 and the minor third 6/5 are best mapped to the same step of 240 cents, meaning that the semitone separating them, 27/25, is tempered out as well - this is bug temperament, which is a little more perverse even than father.
Because these intervals are so large, this sort of analysis is less significant with 5edo than it becomes with larger and more accurate divisions, but it still plays a role. For example, if we attempt to analyze 5edo as supporting standard diatonic harmony, I–IV–V–I is the same as I–III–V–I and involves triads with common intervals because of fourth-thirds equivalence.
If 5edo is taken as only a tuning of the 3-limit, we find that the circle of fifths returns to the unison after only 5 steps, rather than 12. This is called blackwood temperament, and in 5edo, this is a "good" tuning of a circle of fifths - more formally, since the comma being tempered out, the semitone 256/243, is smaller than half a step (120 cents), 5edo demonstrates 3-to-2 telicity (and is the third EDO to do so after 1edo and 2edo).
5edo is the smallest edo representing the 9-odd-limit consistently, giving a distinct value modulo 5 to 1, 3, 5, 7 and 9 - specifically, 3 is mapped to 3 steps (720 cents), 5 is very inaccurately mapped to 2 steps (480 cents), 7 is mapped to 4 steps (960 cents), and 9 is mapped to 1 step (240 cents). However, while 2edo represents the 3-odd-limit consistently, 3edo the 5-odd-limit, 4edo the 7-odd-limit and 5edo the 9-odd-limit, to represent the 11-odd-limit consistently with a patent val requires going all the way to 22edo.
Despite its lack of accuracy in the 5-limit, 5edo is the second zeta integral edo, after 2edo.
Prime harmonics
| Harmonic | 2 | 3 | 5 | 7 | 11 | 13 | 17 | 19 | 23 | 29 | 31 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +0 | +18 | +94 | -9 | -71 | +119 | -105 | -58 | +92 | -70 | +55 |
| Relative (%) | +0.0 | +7.5 | +39.0 | -3.7 | -29.7 | +49.8 | -43.7 | -24.0 | +38.2 | -29.0 | +22.9 | |
| Steps (reduced) |
5 (0) |
8 (3) |
12 (2) |
14 (4) |
17 (2) |
19 (4) |
20 (0) |
21 (1) |
23 (3) |
24 (4) |
25 (0) | |
Subsets and supersets
5edo is the 3rd prime edo, after 2edo and 3edo and before 7edo. It does not contain any nontrivial subset edos, though it contains 5ed4. Multiples such as 10edo, 15edo, … up to 35edo, share the same tuning of the perfect fifth as 5edo, while improving on other intervals.
Intervals
| Degree | Cents | Interval region | Approximated JI intervals (error in ¢) | Audio | |||
|---|---|---|---|---|---|---|---|
| 3-limit | 5-limit | 7-limit | Other | ||||
| 0 | 0 | Unison (prime) | 1/1 (just) | ||||
| 1 | 240 | Second-inter-third | 144/125 (-4.969) 125/108 (-13.076) |
8/7 (+8.826) 7/6 (-26.871) |
224/195 (-0.030) | ||
| 2 | 480 | Fourth | 4/3 (-18.045) | 21/16 (+9.219) | 33/25 (-0.686) | ||
| 3 | 720 | Fifth | 3/2 (+18.045) | 32/21 (-9.219) | 50/33 (+0.686) | ||
| 4 | 960 | Sixth-inter-seventh | 216/125 (+13.076) 125/72 (+4.969) |
12/7 (+26.871) 7/4 (-8.826) |
195/112 (+0.030) | ||
| 5 | 1200 | Octave | 2/1 (just) | ||||
Notation
The usual notation system for 5edo is the heptatonic chain-of-fifths notation, which is directly derived from the standard notation used in 12edo. The enharmonic unison is the minor 2nd, thus E and F are the same pitch.
| Degree | Cents | Chain-of-fifths notation | |
|---|---|---|---|
| Diatonic interval names | Note names (on D) | ||
| 0 | 0 | Perfect unison (P1) Minor second (m2) Diminished third (d3) |
D Eb Fb |
| 1 | 240 | Augmented unison (A1) Major second (M2) Minor third (m3) Diminished fourth (d4) |
D# E F Gb |
| 2 | 480 | Augmented second (A2) Major third (M3) Perfect fourth (P4) Diminished fifth (d5) |
E# F# G Ab |
| 3 | 720 | Augmented fourth (A4) Perfect fifth (P5) Minor sixth (m6) Diminished seventh (d7) |
G# A Bb Cb |
| 4 | 960 | Augmented fifth (A5) Major sixth (M6) Minor seventh (m7) Diminished octave (d8) |
A# B C Db |
| 5 | 1200 | Augmented sixth (A6) Major seventh (M7) Perfect octave (P8) |
B# C# D |
In 5edo:
- ups and downs notation is identical to circle-of-fifths notation;
- mixed sagittal notation is identical to circle-of-fifths notation, but pure sagittal notation exchanges sharps (#) and flats (b) for sagittal sharp (
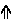 ) and sagittal flat (
) and sagittal flat (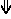 ) respectively.
) respectively.
Sagittal notation
This notation uses the same sagittal sequence as EDOs 12, 19, and 26, and is a subset of the notations for EDOs 10, 15, 20, 25, 30, and 35b.
Because it includes no Sagittal symbols, this Sagittal notation is also a conventional notation.
Alternative notations
- via Reinhard's cents notation
- a four-line hybrid treble/bass staff.
Intervals can be named penta-2nd, penta-3rd, penta-4th, penta-5th and hexave. The circle of fifths: 1sn -- penta-4th -- penta-2nd -- penta-5th -- penta-3rd -- 1sn.
Kite Giedraitis has proposed pentatonic interval names that retain the appearance of heptatonic names, to avoid the confusion caused by one's lifelong association of "fourth" with 4/3, not 3/2. The interval names are unisoid, subthird, fourthoid, fifthoid, subseventh and octoid, or 1d s3 4d 5d s7 8d. The circle of fifths: 1d -- 5d -- s3 -- s7 -- 4d -- 1d. When notating larger edos such as 8 or 13 this way, there are major or minor sub3rds and sub7ths. Note that 15/8 is an octoid and 16/15 is a unisoid.
For note names, Kite often omits B and merges E and F into a new letter, "eef" (rhymes with leaf). Eef, like E, is a 5th above A. Eef, like F, is a 4th above C. The circle of 5ths is C G D A Eef C. Eef is written like an E, but with the bottom horizontal line going not right but left from the vertical line. Eef can be typed as ⺘(unicode 2E98 or 624C) or ꘙ (unicode A619) or 𐐆 (unicode 10406). Eef can also be used to notate 15edo.
Solfege
| Degree | Cents | Standard solfege (movable do) |
Uniform solfege (1 vowel) |
|---|---|---|---|
| 0 | 0 | Do (P1) | Da (P1) |
| 1 | 240 | Re (M2) Me (m3) |
Ra (M2) Na (m3) |
| 2 | 480 | Mi (M3) Fa (P4) |
Ma (M3) Fa (P4) |
| 3 | 720 | So (P5) Le (m6) |
Sa (P5) Fla (m6) |
| 4 | 960 | La (M6) Te (m7) |
La (M6) Tha (m7) |
| 5 | 1200 | Ti (M7) Do (P8) |
Da (P8) |
Approximation to JI
Selected 7-limit intervals
Observations
Related scales
- By its cardinality, 5edo is related to other pentatonic scales, and it is especially close in sound to many Indonesian slendros.
- Due to the interest around the "fifth" interval size, there are many nonoctave "stretch sisters" to 5edo: square root of 4/3, cube root of 3/2, 8th root of 3, etc.
- For the same reason there are many "circle sisters":
- Make a chain of five "bigger fifths" (50/33), which makes three octaves 3.227¢ flat. (50/33)^5 = 7.985099.
Cycles, divisions
5 is a prime number so 5edo contains no sub-edos. Only simple cycles:
- Cycle of seconds: 0-1-2-3-4-0
- Cycle of fourths: 0-2-4-1-3-0
- Cycle of fifths: 0-3-1-4-2-0
- Cycle of sevenths: 0-4-3-2-1-0
Harmony
5edo does not have any strong consonance nor dissonance. It could be considered omniconsonant. The 240 cent interval can serve as either a major second or minor third, and the 960 cent interval as either a major sixth or minor seventh. The fourth is about 18 cents flat of a just fourth, making it rather "dirty" but recognizable. The fifth is likewise about 18 cents sharp of a just fifth, dissonant but still easily recognizable.
In contrast to other edos, all of the notes can be used at once in order to get a functioning scale. (As in Blackwood in 10edo).
Important chords:
- 0+1+3
- 0+2+3
- 0+1+3+4
- 0+2+3+4
Melody
Smallest edo that can be used for melodies in a "standard" way. The relatively large step of 240 cents can be used as major second for the melody construction. The scale has whole-tone as well as pentatonic character.
Chord or scale?
Either way, it is hard to wander very far from where you start. However, it has the scale-like feature that there are (barely) enough notes to create melody, in the form of an equal version of pentatonic.
Regular temperament properties
Uniform maps
| Min. size | Max. size | Wart notation | Map |
|---|---|---|---|
| 4.7696 | 4.8088 | 5cddf | ⟨5 8 11 13 17 18] |
| 4.8088 | 4.9528 | 5cf | ⟨5 8 11 14 17 18] |
| 4.9528 | 4.9994 | 5f | ⟨5 8 12 14 17 18] |
| 4.9994 | 5.0586 | 5 | ⟨5 8 12 14 17 19] |
| 5.0586 | 5.1650 | 5e | ⟨5 8 12 14 18 19] |
| 5.1650 | 5.2696 | 5de | ⟨5 8 12 15 18 19] |
Commas
5et tempers out the following commas. This assumes the val ⟨5 8 12 14 17 19].
| Prime limit |
Ratio[note 1] | Monzo | Cents | Color name | Name(s) |
|---|---|---|---|---|---|
| 3 | 256/243 | [8 -5⟩ | 90.225 | Sawa | Blackwood comma, Pythagorean limma |
| 5 | 27/25 | [0 3 -2⟩ | 133.238 | Gugu | Bug comma, large limma |
| 5 | 16/15 | [4 -1 -1⟩ | 111.731 | Gubi | Dicot comma, classic chroma |
| 5 | 81/80 | [-4 4 -1⟩ | 21.506 | Gu | Syntonic comma, Didymus' comma, meantone comma |
| 5 | (22 digits) | [24 -21 4⟩ | 4.200 | Sasa-quadyo | Vulture comma |
| 7 | 36/35 | [2 2 -1 -1⟩ | 48.770 | Rugu | Mint comma, septimal quartertone |
| 7 | 49/48 | [-4 -1 0 2⟩ | 35.697 | Zozo | Semaphoresma, slendro diesis |
| 7 | 64/63 | [6 -2 0 -1⟩ | 27.264 | Ru | Septimal comma, Archytas' comma, Leipziger Komma |
| 7 | 245/243 | [0 -5 1 2⟩ | 14.191 | Zozoyo | Sensamagic comma |
| 7 | 1728/1715 | [6 3 -1 -3⟩ | 13.074 | Triru-agu | Orwellisma |
| 7 | 1029/1024 | [-10 1 0 3⟩ | 8.433 | Latrizo | Gamelisma |
| 7 | 19683/19600 | [-4 9 -2 -2⟩ | 7.316 | Labiruru | Cataharry comma |
| 7 | 5120/5103 | [10 -6 1 -1⟩ | 5.758 | Saruyo | Hemifamity comma |
| 7 | (18 digits) | [-26 -1 1 9⟩ | 3.792 | Latritrizo-ayo | Wadisma |
| 7 | (12 digits) | [-6 -8 2 5⟩ | 1.117 | Quinzo-ayoyo | Wizma |
| 11 | 11/10 | [-1 0 -1 0 1⟩ | 165.004 | Logu | Large undecimal neutral 2nd |
| 11 | 99/98 | [-1 2 0 -2 1⟩ | 17.576 | Loruru | Mothwellsma |
| 11 | 896/891 | [7 -4 0 1 -1⟩ | 9.688 | Saluzo | Pentacircle comma |
| 11 | 385/384 | [-7 -1 1 1 1⟩ | 4.503 | Lozoyo | Keenanisma |
| 11 | 441/440 | [-3 2 -1 2 -1⟩ | 3.930 | Luzozogu | Werckisma |
| 11 | 3025/3024 | [-4 -3 2 -1 2⟩ | 0.572 | Loloruyoyo | Lehmerisma |
| 13 | 14/13 | [1 0 0 1 0 -1⟩ | 128.298 | Thuzo | Tridecimal 2/3-tone, trienthird |
| 13 | 91/90 | [-1 -2 -1 1 0 1⟩ | 19.130 | Thozogu | Superleap comma, biome comma |
| 13 | 676/675 | [2 -3 -2 0 0 2⟩ | 2.563 | Bithogu | Island comma, parizeksma |
Ear training
5edo ear-training exercises by Alex Ness available here:
For any musician, there is no substitute for the experience of a particular xenharmonic sound. The user going by the name Hyacinth on Wikipedia and Wikimedia Commons has many xenharmonic MIDI's and has graciously copylefted them! This is his 5-TET scale MIDI:
Instruments
Music
- See also: Category:5edo tracks
There is also much 5edo-like world music, just search for "gyil" or "amadinda" or "slendro".
Notes
- ↑ Ratios longer than 10 digits are presented by placeholders with informative hints.