Uniform solfege

Not to be confused with Universal solfege.

Uniform solfeges are a type of solfege devised by Kite Giedraitis. They are closely related to his ups and downs notation. Like the notation, they work with both rank-1 and rank-2 temperaments. They use a uniform vowel sequence for each degree, hence the name. A uniform solfege lets one perform basic interval arithmetic directly within the solfege, without having to translate to note names or interval names and back.

Theory

Uniform solfeges use the conventional consonants D R M F S L T. But all consonants except D have an alternate form that indicates flattening or sharpening:

Fr- = flat Re = minor 2nd (mnemonic: F stands for flat)
N- = flat Mi = minor 3rd
P- = sharp Fa = aug 4th
Sh- = flat So = dim 5th
Fl- = flat La = minor 6th (mnemonic: F stands for flat)
Th- = flat Ti = minor 7th

Sharpening and flattening refers to adding/subtracting the lawa unison (Lw1) aka apotome. Mnemonic for Pa: Sh- sharpens to S-, and Th- sharpens to T-, so if Fa were spelled Pha, it would sharpen to Pa.

The vowel sequence runs from high to low -i -u -a -o -e. The meaning varies slightly depending on the context.

(1) Whenever ups and downs are used (most edos, and all single-pair pergens):

-i = dup (double-up)
-u = up
-a = plain
-o = down
-e = dud (double-down)

However for certain edos such as 34 and 41, -i means mid and -e is not used.

(2) Whenever ups and downs are not used (sharp-1 edos and the unsplit pergen):

-i = double-augmented
-u = augmented
-a = natural
-o = diminished
-e = double-diminished

(3) Whenever both ups/downs and lifts/drops are used (double-pair pergens):

From high to low:

-i = lift
-u = up
-a = plain
-o = down
-e = drop

The 5 vowels are pronounced like those in Spanish or Italian. There are only 5 vowels because those are the most singable, and also additional vowels would make the solfeges harder to learn. Shi is pronounced "she" and She is "shay". Fri is "free".

Example scales


3-limit	Plain major scale	Da	Ra	Ma	Fa	Sa	La	Ta	Da
3-limit	Plain minor scale	Da	Ra	Na	Fa	Sa	Fla	Tha	Da
5-limit	Downmajor scale	Da	Ra	Mo	Fa	Sa	Lo	To	Da
5-limit	Upminor scale	Da	Ra	Nu	Fa	Sa	Flu	Thu	Da
7-limit	Upmajor scale	Da	Ra	Mu	Fa	Sa	Lu	Tu	Da
7-limit	Downminor scale	Da	Ra	No	Fa	Sa	Flo	Tho	Da

Suggestion for learning

Even with many familiar consonants and a consistent vowel sequence, it can take a while to master a large solfege. One might want to take a divide-and-conquer approach. Start with using this simple solfege:

Da - Ra - Ma - Fa - Sa - La - Ta - Da

This helps with unlearning the traditional vowels. Next add in the 6 altered consonants, making a 12-edo-like solfege:

Da - Fra - Ra - Na - Ma - Fa - Pa/Sha - Sa - Fla - La - Tha - Ta - Da

Once this is fully internalized, add in the other vowels.

Interval Arithmetic

Octave complements

To find the octave complement of any interval:

change the degree as usual: 2nd <--> 7th, 3rd <--> 6th, and 4th <--> 5th.
change the quality as usual: major <--> minor and aug <--> dim, but perfect and mid are unchanged.
get the new consonant from the quality and degree.
change the vowel as expected: -u <--> -o, but -a is unchanged. If -e is used, -i <--> -e, otherwise -i is unchanged.

For example, Fru = minor-Re-up becomes major-Ti-down = To.

An edo's circle of fifths

The 13 -a notes form a chain of 5ths running from the dim 5th to the aug 4th:

Sha - Fra - Fla - Na - Tha - Fa - Da - Sa - Ra - La - Ma - Ta - Pa

Each vowel creates a separate chains of 5ths, usually starting with Sh- and ending with P-. But each P- note is enharmonically equivalent to some Sh- note (or S- or F- note). In 12n edos, the vowel of the equivalent note is the same, the chain becomes a circle, and there are multiple circles of 5ths. In other edos, the vowel changes, and the duplicate names connect these separate chains into one circle. (See 34edo for an exception.) This is one rationale for the 13th consonant P-, for it supplies the needed duplicate names.

For example, 31edo uses 3 vowels. Since Pa = Sho, the end of the -a chain connects to the start of the -o chain. Since Po = Fu, the -o chain connects to the -u chain. Since Pu = Sha, the -u chain connects back to the -a chain, making a circle of 31 5ths:

Da Sa Ra La Ma Ta Pa/Sho Fro Flo No Tho Fo Do So Ro Lo Mo To Po/Fu Du Su Ru Lu Mu Tu Pu/Sha Fra Fla Na Tha Fa Da

Thus as long as one spells the tritones correctly, all 5ths in an edo rhyme. This makes interval arithmetic very easy.

Adding/subtracting 4ths and 5ths

The note a 4th or 5th above any note always rhymes, and the consonant is as would be expected from conventional interval arithmetic. Ra plus a 4th is Sa, Fro plus a 5th is Flo, etc. Thus in the example scales above, the 3rd, 6th and 7th always rhyme, as do the tonic, 2nd, 4th and 5th.

However, consider the aug 4th, a P- note. The note a 5th above it would be an augmented 8ve, which doesn't exist in a uniform solfege. Therefore one must rename the tritone as a dim or mid 5th. Thus in 31edo Pa + 5th = Sho + 5th = Fro. Likewise, Sho and Sha need renaming when adding a 4th: Sha + 4th = Pu + 4th = Tu.

Adding/subtracting other intervals

The same rule for 4ths and 5ths mostly holds for plain major 2nds. Keep the vowel, and change the consonant as expected. Ra + M2 = Ma. But an aug 4th must be renamed as a 5th. Beware, this rule breaks down entirely for major and mid 7ths (the T- notes), due to the lack of aug and mid 8ves.

In general, one can add or subtract any conventional (i.e. plain) interval from any note, and the result will be as expected. But only if the expected answer exists in the solfege. It must exist on the 13-note chain of 5ths from dim5 to aug4. In other words, the expected answer must not be augmented or diminished, unless it's an aug4 or a dim5. (Otherwise, one must use an enharmonic equivalent.) For example, one can easily add a M3 to any note other than a L-, M-, T- or P- note. Thus Ro + M3 = Po and Na + M3 = Sa, but La + M3 is a Fr- note. Beware, sometimes a chain is not 13 notes long, and when adding to or subtracting, the expected answer must exist on the shorter chain. For example, in 31edo, the -u chain only runs from P4 to A4.

One can often easily add/subtract an unconventional (upped or downed) interval as well. The ups and downs add up and cancel out as expected. Thus Ra + vM2 = Mo and Ru + vM2 = Ma. Obviously the vowel will change. Again, the expected answer must exist in the solfege. 3-vowel solfeges lack double-ups and double-downs. 4-vowel solfeges lack double-upmajor and double-downminor.

Solfeges for edos

Main article: List of uniform solfeges for EDOs

In the perfect edos (7, 14, 21, 28 and 35), there is no need for the altered consonants, since major and minor are equated. Thus these edos only use 7 consonants. Edos 5, 11 and 13 also omit some of the consonants.

the four vowel sequences, with example edos
1 vowel	5, 7, 9, 12			-a = plain
3 vowels	10, 13b-19, 22		-o = down	-a = plain	-u = up
4 vowels	25, 27, 34, 41	-i = mid	-o = down	-a = plain	-u = up	-i = mid
5 vowels	43, 46, 53, 60	-e = dud	-o = down	-a = plain	-u = up	-i = dup

There is only so much one can do with 5 vowels and 13 consonants. Not all edos are covered. The number of vowels an edo's solfege needs equals the edo's sharpness or penta-sharpness, whichever is larger. Thus an edo with a (penta)sharpness of 6 or higher needs 6 or more vowels and isn't covered. Every edo above 60 is such an edo. The excluded edos are the less efficient ones, with a fairly large size, or a fairly inaccurate 5th for their size. Thus they tend to be the less popular edos.

Because 72edo is such a popular edo, an exception is made and it has 2 additional vowels.

Examples

12edo: Da Fra Ra Na Ma Fa Pa/Sha Sa Fla La Tha Ta Da
21edo: Da Du Ro Ra Ru Mo Ma Mu Fo Fa Fu So Sa Su Lo La Lu To Ta Tu Do Da

Superflat edos (9, 11, 13b, 16, 18b and 23) have a very flat 5th. A uniform solfege can still be used, but the size of the interval won't match what its name implies very well.

In sharp-1 edos, to up an interval means to augment it. Thus Fu = Pa and So = Sha. Fru = Ra and Fra = Ro.

In flat-1 edos (9, 16 and 23), to up an interval means to diminish it. Fo = Pa and Su = Sha. Fro = Ra and Fra = Ru.

In sharp-2 and sharp-4 edos, the mid 2nd/3rd/6th/7th is spelled as downmajor, the mid 4th is spelled as upperfect, and the mid 5th is downperfect.

In edos with an even penta-sharpness, there are interordinal notes with two names. For example, 4\19 is named as both a 2nd and a 3rd (Ru/No).

Correlations with color notation

-u/-o can mean not only up/down, but also under/over, meaning in the ratio's denominator or numerator. A color notation review:

yo/gu = 5-over/5-under = subtract/add 81/80
zo/ru = 7-over/7-under = subtract/add 64/63
ilo/lu = 11-over/11-under = subtract/add 729/704
tho/thu = 13-over/13-under = subtract/add 27/26

(In color notation, the last two commas could instead be 33/32 and 1053/1024, but these are "over" commas, and they must be under to keep the -o/-u correlation.)

If 81/80 maps to 1 edostep, then yo/gu = down/up = -o/-u. Likewise with the other commas. The table below shows that almost every edo has at least one such correlation. Parentheses are used when the prime's relative error is high, e.g. 12edo's prime 11.

colorspeak correlations for all 25 diatonic edos that have uniform solfeges with 5 vowels or less
	12	17	19	22	24	26	27	29	31	33	34	36	38	39	40	41	43	45	46	48	50	53	60
ya		(yo)		yo			yo	yo			yo			(yo)		yo			yo	(yo)		yo	yo
ya		(gu)		gu			gu	gu			gu			(gu)		gu			gu	(gu)		gu	gu
za			zo		zo	zo		zo	zo	zo	zo	zo	zo	(zo)		zo	zo		zo	zo		zo
za			ru		ru	ru		ru	ru	ru	ru	ru	ru	(ru)		ru	ru		ru	ru		ru
ila	(ilo)	ilo			ilo				ilo			(ilo)	(ilo)				ilo				ilo
ila	(lu)	lu			lu				lu			(lu)	(lu)				lu				lu
tha		tho	tho		tho	tho			tho	tho			tho		tho			(tho)
tha		thu	thu		thu	thu			thu	thu			thu		thu			(thu)

Solfeges for rank-2 temperaments

Main article: List of uniform solfeges for pergens

Rank-2 temperaments have an infinite number of notes, so a solfege can only cover a fraction of them. But often one only needs enough notes to make a MOS scale. Pergens tell us how to use ups and downs to notate these temperaments, and the same consonants and vowels can be used. Instead of circles of 5ths, there are fifthchains. Each fifthchain requires its own vowel, so there is a maximum of 5 fifthchains. However this can be extended to 9 fifthchains by using compound vowels such as -iyu, see below.

Genchains are distinct from fifthchains. Each pergen has one or more genchains, each of which contains one or more fifthchains. The number of genchains always equals the number of periods per octave. In general, the number of fifthchains per genchain equals the number of generators per multigen, which is the 2nd interval in the pergen. Thus (P8, P4/3) has 1 genchain that contains 3 interwoven fifthchains. But an exception arises when the multigen is not perfect (i.e. major or minor). Then the fifthchains hop from one genchain to the next. The only such pergen that has a uniform solfege is (P8/2, M2/4). It has 2 genchains and a total of 4 fifthchains.

The 13 consonants and 5 vowels without compound vowels cover 20 pergens. Of course, the genchains can only extend so far with only 13 consonants. But in general, it's enough to cover all the modes of any reasonably-sized MOS scale. TallKite.com/misc_files/notation guide for rank-2 pergens.pdf lists many pergens. The tuning of every interval and every accidental is defined in terms of c = P5 - 700¢. The EI (enharmonic interval, "E" in the pdf) can be added to or subtracted from any note or interval to get an equivalent note or interval. The entire solfege can be derived from the pergen, the EI and the vowel sequence. For each pergen, there is one "official" solfege.

Sometimes -i and -e mean lift/drop not dup/dud. -i never means mid, so there are only two vowel sequences:

single-pair solfeges
-3	-2	-1	0	1	2	3
trud	dud	down	plain	up	dup	trup
-eyo	-e	-o	-a	-u	-i	-iyu

double-pair solfeges
	down	plain	up
lift	-owi	-i	-uwi
plain	-o	-a	-u
drop	-owe	-e	-uwe

Any compound vowel with a "w" is for double-pair only. Mnemonic: w = "double-U" = double-pair.

The whole (or unsplit) pergen (P8, P5) doesn't use ups and downs. It uses the single-pair vowel sequence, with -u and -o repurposed to mean augmented and diminished:

(P8, P5) solfege
-3	-2	-1	0	1	2	3
ddd	dd	dim	plain	aug	AA	AAA
-eyo	-e	-o	-a	-u	-i	-iyu

Applications

EDOs

In any single-ring edo, a prime can be mapped not only to a specific number of edosteps, but also to a specific number of fifths. This is called the fifthspan. The fifthspan of prime 2 is always zero and the fifthspan of prime 3 is always one. The fifthspans of all the primes is called the fifthspan mapping. The mapping can be expressed very concisely as a solfege string, a list of uniform solfege syllables in which -u/-o means aug/dim. Note that this often differs from the EDO solfeges listed above, where -u/-o often refers to up/down. Primes 2 and 3 are always DaSa by definition, so these two primes are omitted from the string.

Fifthspans of various primes in various single-ring edos
	prime 3	prime 5	prime 7	prime 11	prime 13	solfege string	alternates
19-edo	1	4	-9	6	-4	MaThoPaFla	Tho=Lu
22-edo	1	9	-2	-6	-9	RuThaShaTho	Ru=Sho, Tho=Pu
31-edo	1	4	10	-13	15	MaLuShoSi	Sho=Mi, Si=The
41-edo	1	-8	-14	-18	20	FoDeFlePi	Fle=Riyu, Pi=Deyo
53-edo	1	-8	-14	23	20	FoDeRiyuPi	Riyu=Theye

Two edos can have the same mapping. For example both 19edo and 26edo are MaThoPaFla.

The solfege string for all meantone edos starts with Ma, all schismatic edos start with Fo, all archy edos have Tha as the 2nd syllable, and so forth.

Each prime has a second, larger fifthspan which is found by adding/subtracting the edo itself. For example, 31edo's prime 13 fifthspan is 15 but also 15 - 31 = -16. Thus 31edo's alternate solfege string is MaLuShoThe. The alternate fifthspan is usually only of interest if the smaller fifthspan approaches half the edo, and the alternate fifthspan is only slightly more remote.

In a multi-ring edo such as 72, -u/-o must be repurposed to mean up/down. The alternates in the table below are exactly as remote as the primary names.

Solfege strings for various multi-ring edos
	prime 5	prime 7	prime 11	prime 13	solfege string	alternates
15-edo	vM3	m7	^4	(N/A)	MoThaFu
24-edo	M3	vm7	^4 or vA4	^m6 or vM6	MaThoPoLo	Po=Fu, Lo=Flu
34-edo	vM3	(N/A)	^^4	^^m6	MeAPoLo	Po=Fu, Lo=Flu
72-edo	vM3	vvm7	^³P4 or v³A4	^³m6 or v³M6	MoThePeyoLeyo	Peyo=Fiyu, Leyo=Fliyu

34edo is an unusual case. Each ring has 17 notes, which is more than 13 consonants and 1 vowel can cover. So -u/-o means up/down within the ring, and -i/-e means lift/drop by an edostep from one ring to the next. Note the use of -A- to exclude prime 7, which in 34edo has a huge relative error of 45%.

Rank-2 temperaments

The second row of the temperament's mapping directly yields a solfege string (see the previous section). This string, plus the pergen, can serve to concisely name the temperament.

For example, 11-limit Triyo/Porcupine has a mapping [⟨1 2 3 2 4], ⟨0 -3 -5 6 -4]]. The pergen is (P8, P4/3), and its solfege is given here. One simply uses syllables from columns 0, -3, -5, 6 and -4 to get DaSaMoThaFu. Since primes 2 and 3 are always DaSa by definition, they can be omitted. The temperament can be defined by the pergen plus the solfege string as "third-4th MoThaFu". Two 13-limit extensions are MoThaFuLo and MoThaFuSi. More examples: Pajara is "half-8ve MoTha" and Injera is "half-8ve MaThu". You can tell injera is in the meantone family because the first solfege is Ma. You can tell it's a weak extension of meantone because the pergen differs from meantone's.

The solfege string doesn't precisely define the temperament, since the first row of the mapping isn't used, and theoretically those numbers could change. But unless the period is a small fraction of an octave, such alternate mappings will be extremely inaccurate. So this nomenclature only covers reasonably accurate temperaments.

Bosanquet keyboards

Using fixed-solfege, each physical key on the Lumatone can be named. It's best to let -u/-o mean aug/dim not up/down, since the meaning of ups and downs changes in different edos. For example, in 31edo an up equals a step in the 5:00 direction, but in 41edo it's the opposite, a step in the 11:00 direction.

This picture shows the solfege names if Da corresponds to the note C.

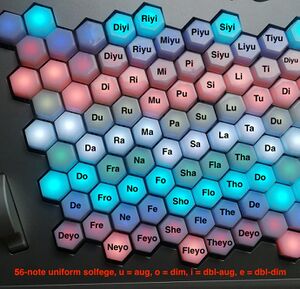
The uppermost few keys use -iyi ("ee-yee"), meaning quadruple-aug. One could set Da to D not C, in order to get a more symmetrical layout, and thus change two of the three -iyi's to -eyo's.

Using movable-solfege, one can name the notes of a scale independently of the key. One can also name any physical interval on the lumatone. For example, one step in the 1:00 direction is always Du, two steps in the 2:30 direction is always Ma, etc.

Solfege strings: The placement of various primes on a Bosanquet keyboard is determined by the fifthspan mapping (see the previous sections). Thus an edo's solfege string tells a lumatone player the physical placement of various primes. Notes ending in -u/-i lie on the top half of the keyboard and those ending-o/-e lie on the bottom half. The alternate fifthspan is sometimes useful to bring one prime nearer the others. For example, 41edo's solfege string is FoDeFlePi, with prime 13 being an outlier. It's alternate string is FoDeFleDeyo, which makes for more compact chord shapes.

The solfege string can be used to compare edos. For example 41edo is FoDeFlePi (or FoDeFleDeyo) and 53edo is FoDeRiyuPi. This tells us that primes 5, 7 and perhaps 13 are placed similarly, but prime 11 differs. Thus any 7-limit chord's shape is the same in both 41edo and 53edo.