Ring number

The ring number of an edo is the number of circles of fifths in it, which equals the GCD of the edostepspans of the octave and the fifth, or more generally the equave and the generator. Every edo has a ring number associated with its approximation to the fifth.

Examples

12edo's best approximation of 3/2 is 7\12. Since 7 and 12 are co-prime, 12edo has only one circle of fifths. Its ring number is 1, and 12edo is said to be single-ring. An edo with a non-co-prime fifth is multi-ring, or "ringy". For example, 15edo's best approximation of 3/2 is 9\15. The ring number is the GCD of 9 and 15, which is 3. Thus 15edo is a triple-ring edo. Using an alternative approximation of 3/2 affects the "ringiness": 18edo is not multi-ring, but 18b-edo is.

Properties

If N is a prime number, N-edo is a single-ring edo. Note that if N is not prime, N-edo may still be single-ring.

Chain-of-fifths notation only works for single-ring edos.

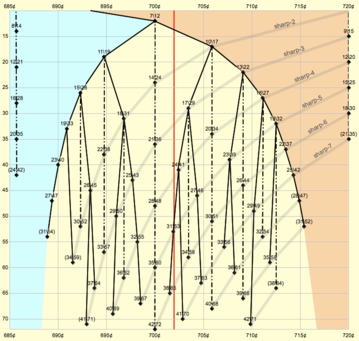
On the scale tree, multi-ring edos appear only on the spines of the kites, shown here as dotted lines:

Generalizations

The ring number can be defined using any two intervals, not just the octave or the fifth. The two intervals are treated as equave and generator. For example, 15-edo can be thought of as generated by 2\15 (Porcupine/Triyo), which makes it single-ring. And 13ed3 can be thought of as generated by 6\13 (a tempered 5/3), again single-ring.

Analogous to ring number, rank-2 temperaments have a chain number. For example, any rank-2 temperament with a pergen of (P8, P5) has a chain number of 1, and is single-chain. All other pergens are multi-chain. For example, Porcupine/Triyo has pergen (P8, P4/3) and is triple-chain. Diaschismatic/Sagugu has pergen (P8/2, P5) and is double-chain. A pergen (P8/m, M/n) has chain number m·n/|f|, where M is the multigen and f is M's fifthspan. For example (P8/2, M2/4) is quadruple-chain.

Like the ring number, the chain number can be generalized to other intervals besides the octave and the fifth.

The terms ring number and chain number were coined by Kite Giedraitis.