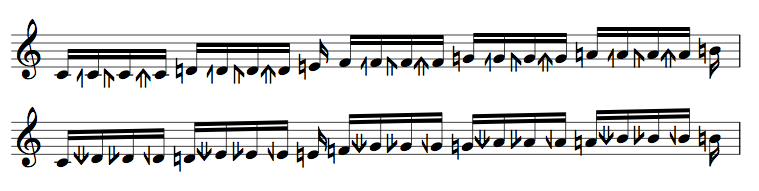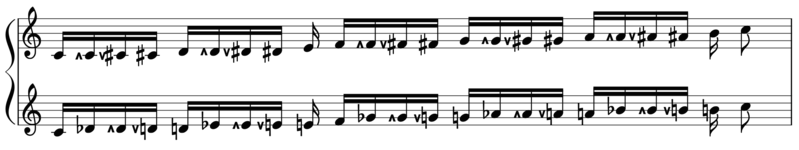User:Triethylamine/draft: 22平均律

This page is a draft of JP translation of 22edo.

Prime factorization

2 × 11

Step size

54.5455¢

Fifth

13\22 (709.091¢)

Semitones (A1:m2)

3:1 (163.6¢ : 54.55¢)

Consistency limit

11

Distinct consistency limit

5

Special properties

Zeta peak
Zeta peak integer

22 平均律、または 22 音平均律（英: 22 equal divisions of the octave, 22 equal temperament, 22EDO, 22ET）は、レギュラー音律の観点から見ると、オクターブを均等な 22 個のステップに分割した調律システムである。

1 ステップあたりの周波数比は 2 の 22 乗根 [math](\sqrt[22]{2})[/math] であり、約 54.545 セントである。9/8 と 10/9 を区別するので、これはTemplate:En仮リンクシステムではない。

理論

歴史

オクターブを同じサイズの 22 のステップに分割するという考えは、19 世紀の音楽理論家R.H.M. Bosanquetに由来しているようである。Template:En仮リンクにおけるオクターブの 22 の不均等な分割に触発され、Bosanquetはそのような均等な分割により 5-リミットの音楽を許容できる精度で表現できることに注目した。この点については、20 世紀に理論家のJosé Würschmidtが続き、彼はこれを 19 平均律の次の可能性として指摘した。また、J. Murray Barbourは、調律の歴史に関する古典的な調査書『Tuning and Temperament』の中で、これに続いた。

純正音程近似のクオリティの概観

22 平均律のシステムは実際には、12 と 19 に次ぐ、5-リミット音程をTemplate:En仮リンク 4 セント/オクターヴ以内に近似することができる 3 番目の平均律である。ゼータ積分やゼータギャップ平均律ではないが、少なくともTemplate:En仮リンクではある。さらに、5-リミットだけではない。12 や 19 とは異なり、 7, 11-リミット音程を 3 セント/オクターヴ以内の誤差で近似できる。31 平均律の方がはるかに優れているが、22 平均律でもこれらのリミットの和声を利用できる。実際、22 は 11-奇数リミットを一貫して表す最小の等分割である。

さらに、22 平均律は 12 や 19 とは異なり、Template:En仮リンクシステムではない。最終的な効果は、22 という数字があまり馴染みのない音楽領域の探求を可能にし、ある程度強制することでもあるが、やはり十分に小さいので、22 音ギターなどの適切に設計された楽器を使用したライブパフォーマンスで使用できることであろう。

22 平均律は、11 平均律の 2.7.9.11.15.17 サブグループに倍音 3 と 5 を追加したものとして扱うこともでき、（かなり正確な）2.3.5.7.11.17 サブグループ音律になる。31 倍音の近似値は 0.5 セント以内であり、かなり正確であることも注目に値する。また、特に 29/24 などの 29 倍音を含むいくつかの間隔も近似しており、これも 0.5 セント以内で一致する。これにより、2.3.5.7.11.17.29.31 がもたらされる。

22 平均律は、拡張された「クォーターコンマarchy」に非常に近い。これはシントニックコンマ 81/80 の代わりにアルキュタスコンマ 64/63 をテンパーアウトすることを除いて、Template:En仮リンクに似たチューニングである。このため、ほぼ純粋な 7 倍音系長 3 度（9/7）を持つ。

素数倍音

Approximation of prime harmonics in 22edo
Harmonic		2	3	5	7	11	13	17	19	23	29	31
Error	Absolute (¢)	+0.0	+7.1	-4.5	+13.0	-5.9	-22.3	+4.1	-24.8	+26.3	+6.8	+0.4
Error	Relative (%)	+0.0	+13.1	-8.2	+23.8	-10.7	-41.0	+7.6	-45.4	+48.2	+12.4	+0.8
Steps (reduced)		22 (0)	35 (13)	51 (7)	62 (18)	76 (10)	81 (15)	90 (2)	93 (5)	100 (12)	107 (19)	109 (21)

部分集合と上位集合

22 は 11 で割り切れるため、12 平均律が 6 平均律（全音音階）を演奏できるのと同じように、22 平均律楽器は 11 平均律のあらゆる音楽を演奏できる。11 平均律は、旋律的には 12 平均律（よく知られた 1:2:3 の比率で全音、半音、短 3 度）に聞こえる点で興味深いが、特に完全 5 度/4 度や 5-リミット長 3 度/短 6 度がないため、和声的には大きく異なる。同様に、22 平均律と 24 平均律は、どちらも 4 分音や短/中/長 2 度を含むため、メロディー的に似ている。しかし、22 平均律は 24 よりもはるかに優れた全体的なハーモニーを提供する。Template:En仮リンクでは、11 は 22 の 1 つおきの音として記譜できる。

音程

See also: 22edo solfege

ステップ	セント	近似音程^[1]	Template:En仮リンク
0	0.000	1/1	完全1度, ユニゾン	P1	D
1	54.545	36/35, 34/33, 33/32, 32/31	短2度	m2	Eb
2	109.091	18/17, 17/16, 16/15, 15/14	アップ短2度	^m2	^Eb
3	163.636	12/11, 11/10, 10/9	ダウン長2度	vM2	vE
4	218.182	9/8, 17/15, 8/7	長2度	M2	E
5	272.727	20/17, 7/6	短3度	m3	F
6	327.273	6/5, 17/14, 11/9	アップ短3度	^m3	^F
7	381.818	5/4, 96/77	ダウン長3度	vM3	vF#
8	436.364	14/11, 9/7, 22/17	長3度	M3	F#
9	490.909	4/3	完全4度	P4	G
10	545.455	15/11, 11/8	アップ4度, 減5度	^4, d5	^G, Ab
11	600.000	7/5, 24/17, 17/12, 10/7	ダウン増4度, アップ減5度	vA4, ^d5	vG#, ^Ab
12	654.545	16/11, 22/15	増4度, ダウン5度	A4, v5	G#, vA
13	709.091	3/2	完全5度	P5	A
14	763.636	17/11, 14/9, 11/7	短6度	m6	Bb
15	818.182	8/5, 77/48	アップ短6度	^m6	^Bb
16	872.727	18/11, 28/17, 5/3	ダウン長6度	vM6	vB
17	927.273	17/10, 12/7	長6度	M6	B
18	981.818	7/4, 30/17, 16/9	短7度	m7	C
19	1036.364	9/5, 11/6, 20/11	アップ短7度	^m7	^C
20	1090.909	28/15, 15/8, 32/17, 17/9	ダウン長7度	vM7	vC#
21	1145.455	31/16, 64/33, 33/17, 35/18	長7度	M7	C#
22	1200.000	2/1	完全8度, オクターヴ	P8	D

↑ 22平均律を2.3.5.7.11.17サブグループ音律として扱うことに基づいて、サイズの大きい順に並べられたいくつかの単純な比率。他のアプローチも可能。

純正音程近似

15-奇数リミット音程のマッピング

The following tables show how 15-odd-limit intervals are represented in 22edo. Prime harmonics are in bold; inconsistent intervals are in italics.

15-odd-limit intervals in 22edo (direct approximation, even if inconsistent)
Interval and complement	Error (abs, ¢)	Error (rel, %)
1/1, 2/1	0.000	0.0
9/7, 14/9	1.280	2.3
11/10, 20/11	1.368	2.5
15/8, 16/15	2.640	4.8
5/4, 8/5	4.496	8.2
7/6, 12/7	5.856	10.7
11/8, 16/11	5.863	10.7
3/2, 4/3	7.136	13.1
15/11, 22/15	8.504	15.6
15/14, 28/15	10.352	19.0
5/3, 6/5	11.631	21.3
7/4, 8/7	12.992	23.8
11/6, 12/11	12.999	23.8
9/8, 16/9	14.272	26.2
13/11, 22/13	16.482	30.2
7/5, 10/7	17.488	32.1
13/10, 20/13	17.850	32.7
13/9, 18/13	17.928	32.9
9/5, 10/9	18.767	34.4
11/7, 14/11	18.856	34.6
13/7, 14/13	19.207	35.2
11/9, 18/11	20.135	36.9
13/8, 16/13	22.346	41.0
15/13, 26/15	24.986	45.8
13/12, 24/13	25.064	46.0

15-odd-limit intervals in 22edo (patent val mapping)
Interval and complement	Error (abs, ¢)	Error (rel, %)
1/1, 2/1	0.000	0.0
9/7, 14/9	1.280	2.3
11/10, 20/11	1.368	2.5
15/8, 16/15	2.640	4.8
5/4, 8/5	4.496	8.2
7/6, 12/7	5.856	10.7
11/8, 16/11	5.863	10.7
3/2, 4/3	7.136	13.1
15/11, 22/15	8.504	15.6
15/14, 28/15	10.352	19.0
5/3, 6/5	11.631	21.3
7/4, 8/7	12.992	23.8
11/6, 12/11	12.999	23.8
9/8, 16/9	14.272	26.2
13/11, 22/13	16.482	30.2
7/5, 10/7	17.488	32.1
13/10, 20/13	17.850	32.7
9/5, 10/9	18.767	34.4
11/7, 14/11	18.856	34.6
11/9, 18/11	20.135	36.9
13/8, 16/13	22.346	41.0
15/13, 26/15	24.986	45.8
13/12, 24/13	29.482	54.0
13/7, 14/13	35.338	64.8
13/9, 18/13	36.618	67.1

22平均律で近似されるいくつかの17-リミット音程

決定づける特徴

(以下未翻訳・推敲)

セプティマル vs シントニックコンマ

おそらく、22 平均律に慣れていない人にとって最も印象的な特徴は、81/80 のシントニックコンマをテンパーアウトしないため、ミーントーン音律のシステムではないことである。つまり、22 平均律は、9/8 と 10/9 の 2 つの全音などの、12 平均律, 19 平均律, 31 平均律が区別しないピタゴラス音程と 5-リミット音程を区別する。実際、これらの区別は、5-リミット純正律(JI)や、34 平均律, 41 平均律, 53 平均律などのより正確な音律と比較すると誇張されている。

22 平均律が作り出すダイアトニックスケールはTemplate:En仮リンク音律から派生したもので、ミーントーンのダイアトニックスケール（LLsLLLs, または 5L 2s）と同じスケール構造を持ちながらも、3 度は 5/4 や 6/5 ではなく、9/7 や 7/6 に近い。つまり、シントニックコンマ (81/80) ではなく、セプティマルコンマ (64/63) が消えるということであり、これは 22 平均律の核となる特徴の一つである。スーパーパイスは、疑似的に等間隔の 5 音階（大全音と下短 3 度(縮 3 度)の大きさがかなり近いため）と、12 平均律やその他のミーントーンシステムと比べてより不均等な 7 音階を持つ点で旋律的に興味深い。ステップパターンはそれぞれ 4 4 5 4 5 と 4 4 1 4 4 4 1 である。

ポーキュパインコンマ

また、250/243 のポーキュパインコンマまたはmaximal diesisをテンパーアウトするため、22 平均律はTemplate:En仮リンクをTemplate:En仮リンクする。ポーキュパインのジェネレーターは低い 10/9 の小全音で、2 つでわずかに高い 6/5、3 つでわずかに低い 4/3 になる。これは、ポーキュパインの特徴である等間隔なテトラコルドの存在を示唆している。ポーキュパインは、良く知られた 12 平均律によっては近似されない 5-リミット音律のうち、悪さが最も少ないものであることで有名である。そのため、22 平均律の倍音特性を調べるための優れた出発点の一つとなる。ポーキュパインは 7 音と 8 音の二つのTemplate:En仮リンクを形成し、22 平均律ではそれぞれ 4 3 3 3 3 3 と 3 1 3 3 3 3 3 3（およびそれぞれのモード）に調律される。

その他の 5-リミットコンマ

22 平均律がテンパーアウトするその他の 5-リミットコンマには、ディアスキスマ(diaschisma)(2048/2025)とマジックコンマまたはsmall diesis(3125/3072)がある。12 平均律や22 平均律などのディアスキスマシステムでは、全音階の 3 度上の 9/8 を表す大全音の長 3 度上である 45/32 のダイアトニック三全音は、そのオクターヴ反転である 64/45 と等しくなる。マジックコンマがテンパーアウトされるということは、22 平均律が 5 つの長 3 度で完全 5 度を構成するマジックシステムであることを意味する。

その他の 7-リミットコンマ

7-リミットでは、22 平均律は 12 平均律によってもテンパーアウトされる特定のコンマをテンパーアウトする。これは、ミーントーンシステムが類似するのとは異なる方法で 12 平均律を 22 平均律に関連付ける。jubilisma (50/49) とセプティマルコンマ (64/63) は、両方のシステムでテンパーアウトされる。したがってどちらの平均律においても、50/49 により 7/5 と 10/7 の 2 つの 7 倍音系三全音が同一視され、さらに 64/63 により属七和音とotonalテトラッドが区別されない。したがって、どちらも (50/49)/(64/63) = 225/224, Template:En仮リンク（セプティマルクレイズマ）をテンパーアウトするため、マーベル増三和音は 22 平均律のコードであり、どのミーントーン調律のコードでもある。12 平均律によってテンパーアウトされないが、22 平均律によってテンパーアウトされる 7 倍音系コンマは 1728/1715, つまりTemplate:En仮リンクである。また、Template:En仮リンクも 22 平均律のコードである。

11-リミットコンマ

11-リミットでは、22 平均律はquartismaをテンパーアウトし、5 つの 33/32 四分音が 1 つの 7/6 下短 3 度(縮 3 度)に等しくなる。これは 24 平均律と共有されている特性だが、驚くべきことに、17 平均律, 26 平均律, 34 平均律などの他の比較的小さな平均律のいくつかと共有されていない。実際、有名な 53 平均律でさえこの特性を持っていない。ただし、関連する 159 平均律にはあることに注意。

その他の特徴

164¢ の「低い小全音」は、22 平均律の重要な音程である。これは、11-リミットで 10/9, 11/10, 12/11 という 3 つもの異なる協和音程比として機能するためである。したがって、非常に曖昧でかつ柔軟性がある。その代償として、12 平均律ピアノの隙間に非常に近いため、ほとんどの 12 平均律のリスナーにとっては慣れるのに時間がかかる。5-リミットの音楽を 22 平均律に単純に変換すると、非常に異なるサウンドになり、より複雑な倍音のクオリティが必然的に生じる。22 平均律には中立 3 度は含まれないが、5-リミットの 3 度は両方とも「中立のような」クオリティを持つ。これは、12 平均律のように離れているのではなく、より近い距離で調律されているためである。

22 平均律は、7 倍音系下短 3 度をジェネレーター（5 ステップ）として使用し、ステップパターン 3 2 3 2 3 2 3 2 2 および 1 2 2 1 2 2 1 2 2 1 2 2 2 でMOSスケールを形成するオーウェル音律もサポートしている。ハーモニー的には、オーウェルは 31 平均律, 53 平均律, 84 平均律など、他の音律でより正確にチューニングできる。しかし、22平均律オーウェルはメロディ的に他よりも優位に立っており、オーウェル[9]の大小のステップは 22 では区別しやすい。

22 平均律は、4 分音と短 2 度、中立 2 度、長 2 度を含む点で 24 平均律と旋律的に似ているが、22 平均律は 24 よりも総合的に優れたハーモニーを提供する。Template:En仮リンクでは、11 は 22 の他のすべての音符として記譜できる。

レギュラー音律の性質

Subgroup	Comma List	Mapping	Optimal 8ve Stretch (¢)	Tuning Error
Subgroup	Comma List	Mapping	Optimal 8ve Stretch (¢)	Absolute (¢)	Relative (%)
2.3	[35 -22⟩	[⟨22 35]]	-2.25	2.25	4.12
2.3.5	250/243, 2048/2025	[⟨22 35 51]]	-0.86	2.70	4.94
2.3.5.7	50/49, 64/63, 245/243	[⟨22 35 51 62]]	-1.80	2.85	5.23
2.3.5.7.11	50/49, 55/54, 64/63, 99/98	[⟨22 35 51 62 76]]	-1.11	2.90	5.33
2.3.5.7.11.17	50/49, 55/54, 64/63, 85/84, 99/98	[⟨22 35 51 62 76 90]]	-1.09	2.65	4.87

22et is lower in relative error than any previous equal temperaments in the 11-limit. The next equal temperament that does better in this subgroup is 31. 22et is even more prominent in the 2.3.5.7.11.17 subgroup, and the next equal temperament that does better in this subgroup is 46.

一様写像

13-limit uniform maps between 21.5 and 22.5
Min. size	Max. size	Wart notation	Map
21.5000	21.5353	22bccdddeeeeff	⟨22 34 50 60 74 80]
21.5353	21.5505	22bccdddeeff	⟨22 34 50 60 75 80]
21.5505	21.7492	22bccdeeff	⟨22 34 50 61 75 80]
21.7492	21.7542	22bdeeff	⟨22 34 51 61 75 80]
21.7542	21.7671	22bdee	⟨22 34 51 61 75 81]
21.7671	21.8244	22dee	⟨22 35 51 61 75 81]
21.8244	21.9067	22d	⟨22 35 51 61 76 81]
21.9067	22.0244	22	⟨22 35 51 62 76 81]
22.0244	22.1135	22f	⟨22 35 51 62 76 82]
22.1135	22.1798	22ef	⟨22 35 51 62 77 82]
22.1798	22.2629	22cef	⟨22 35 52 62 77 82]
22.2629	22.2946	22cddef	⟨22 35 52 63 77 82]
22.2946	22.3980	22cddefff	⟨22 35 52 63 77 83]
22.3980	22.4025	22bbcddefff	⟨22 36 52 63 77 83]
22.4025	22.5000	22bbcddeeefff	⟨22 36 52 63 78 83]

コンマ

22et tempers out the following commas. (Note: This assumes the val ⟨22 35 51 62 76 81].)

Prime limit	Ratio^[1]	Monzo	Cents	Color name	Name
3	(22 digits)	[35 -22⟩	156.98
5	250/243	[1 -5 3⟩	49.17	Triyo	Porcupine comma
5	3125/3072	[-10 -1 5⟩	29.61	Laquinyo	Magic comma
5	2048/2025	[11 -4 -2⟩	19.55	Sagugu	Diaschisma
5	(14 digits)	[-21 3 7⟩	10.06	Lasepyo	Semicomma
5	(20 digits)	[32 -7 -9⟩	9.49	Sasa-tritrigu	Escapade comma
5	(32 digits)	[-53 10 16⟩	0.57	Quadla-quadquadyo	Kwazy
7	50/49	[1 0 2 -2⟩	34.98	Biruyo	Jubilisma
7	64/63	[6 -2 0 -1⟩	27.26	Ru	Septimal comma
7	875/864	[-5 -3 3 1⟩	21.90	Zotriyo	Keema
7	2430/2401	[1 5 1 -4⟩	20.79	Quadru-ayo	Nuwell
7	245/243	[0 -5 1 2⟩	14.19	Zozoyo	Sensamagic
7	1728/1715	[6 3 -1 -3⟩	13.07	Triru-agu	Orwellisma
7	225/224	[-5 2 2 -1⟩	7.71	Ruyoyo	Marvel comma
7	10976/10935	[5 -7 -1 3⟩	6.48	Trizo-agu	Hemimage
7	6144/6125	[11 1 -3 -2⟩	5.36	Saruru-atrigu	Porwell
7	65625/65536	[-16 1 5 1⟩	2.35	Lazoquinyo	Horwell
7	(12 digits)	[-6 -8 2 5⟩	1.12	Quinzo-ayoyo	Wizma
11	99/98	[-1 2 0 -2 1⟩	17.58	Loruru	Mothwellsma
11	100/99	[2 -2 2 0 -1⟩	17.40	Luyoyo	Ptolemisma
11	121/120	[-3 -1 -1 0 2⟩	14.37	Lologu	Biyatisma
11	176/175	[4 0 -2 -1 1⟩	9.86	Lorugugu	Valinorsma
11	896/891	[7 -4 0 1 -1⟩	9.69	Saluzo	Pentacircle
11	65536/65219	[16 0 0 -2 -3⟩	8.39	Satrilu-aruru	Orgonisma
11	385/384	[-7 -1 1 1 1⟩	4.50	Lozoyo	Keenanisma
11	540/539	[2 3 1 -2 -1⟩	3.21	Lururuyo	Swetisma
11	4000/3993	[5 -1 3 0 -3⟩	3.03	Triluyo	Wizardharry
11	9801/9800	[-3 4 -2 -2 2⟩	0.18	Bilorugu	Kalisma
13	65/64	[-6 0 1 0 0 1⟩	26.84	Thoyo	Wilsorma
13	78/77	[1 1 0 -1 -1 1⟩	22.34	Tholuru	Negustma
13	91/90	[-1 -2 -1 1 0 1⟩	19.13	Thozogu	Superleap
31	125/124	[-2 0 3 0 0 0 0 0 0 0 -1⟩	13.91	Thiwutriyo	Twizzler

↑ Ratios longer than 10 digits are presented by placeholders with informative hints

ランク-2 音律

Periods per octave	Generator	Temperaments
1	1\22	Sensa Chromo Ceratitid
1	3\22	Porcupine
1	5\22	Orwell (22) / blair (22) / winston (22f)
1	7\22	Magic / telepathy
1	9\22	Superpyth / suprapyth
2	1\22	Shrutar / hemipaj Comic
2	2\22	Srutal / pajara / pajarous
2	3\22	Hedgehog / echidna
2	4\22	Astrology Antikythera Wizard
2	5\22	Doublewide / fleetwood
11	1\22	Undeka Hendecatonic

スケール

See 22edo modes.

テトラコルド

See 22edo tetrachords.

記譜法

スーパーパイス/ポーキュパイン表記

Superpyth/Porcupine Notation is a system arising from both superpyth and porcupine temperament. It categorizes each 22edo interval as major and minor of one or both of those temperaments. s indicates superpyth and p indicates porcupine. Because p now represents porcupine and not perfect, P in perfect intervals is no longer used in this system. Instead the number is used without P and is read as either just the number or "Natural". Example: P5 becomes 5 or N5 = Perfect fifth becomes Natural fifth.

ポーキュパイン表記

Porcupine Notation uses the porcupine generator to generate the notation as well. The 2nd and 7th are perfect, and the 4th and 5th are imperfect like the 3rd and 6th. The natural notes represent a chain of 2nds ABCDEFG. This is the only way to use a heptatonic notation without additional accidentals.

The keyboard runs D * * E * * F * * G * * * A * * B * * C * * D.

ペンタトニック表記

In Pentatonic Notation, the degrees are unison, subthird, fourthoid, fifthoid, subseventh and octoid. The natural notes represent a chain of 5ths FCGDA. This is the only way to use a chain-of-fifths notation without additional accidentals.

The keyboard runs D * * * * F * * * G * * * A * * * * C * * * D.

デカトニック表記

The Decatonic Notation is based on Paul Erlich's decatonic scales. Unlike typical notation, the decatonic system is based on a scale of 10 tones rather than 7. This approach requires an entire re-learning of chords, intervals, and notation, but it allows 22EDO to be notated using only one pair of accidentals, and gives the opportunity to escape a heptatonic thinking pattern. The system is based on two chains of fifths: one represented by Latin letters, the other by Greek. The two chains can be looked at as two juxtaposed pentatonic scales.

Chain 1: C G D A E

Chain 2: γ δ α ε β

The alphabet is, in ascending order: C δ D ε E γ G α A β C

In this alphabet, a chain of fifths is preserved because equivalent Greek letters also represent fifths if they are the same as their Latin counterparts. For example G-D is a fifth, and so is γ-δ.

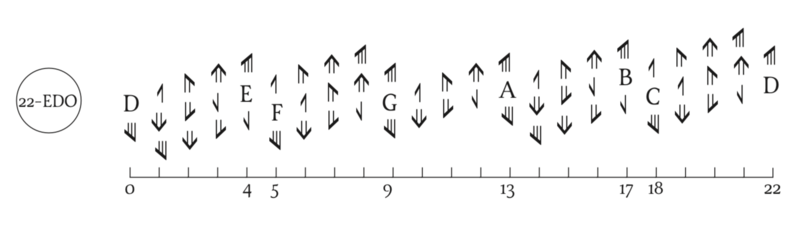
サジタルノーテーション

When 22edo is treated as generated by a cycle of its fifths, the naturals F C G D A E B represent a chain of those 13\22 fifths; consequently, the whole tone comes out to four degrees and the apotome (pythagorean sharp/flat) comes out to three degrees. Three pairs of sagittal symbols, dividing that apotome into three parts, are all that is necessary, and offer plenty of enharmonic equivalents:

This notation is consistent with Sagittal's notation of 5-limit JI harmony: "major" 3rds and 6ths appear as (super)pythagorean intervals flattened by a syntonic comma.

The division of the apotome into three syntonic commas also indicates 22's tempering out of the porcupine comma (which is equivalent to three syntonic commas minus a Pythagorean apotome).

We also have, from the appendix to The Sagittal Songbook by Jacob A. Barton, this diagram of how to notate 22-EDO in the Revo flavor of Sagittal:

アップ&ダウン表記

Treating ups and downs as "fused" with sharps and flats, and never appearing separately:

Treating ups and downs as independent of sharps and flats, and sometimes appearing separately:

A D downmajor scale with mandatory accidentals (no key signature), with minimal accidentals (only when needed to override the key signature), and with independent ups and downs.

Shown below is Paul Erlich's "Tibia" in G, with independent ups and downs.

Tibia in G (page 1)
Tibia in G (page 2)

22平均律の各記譜法の比較

Degree	Cents	Superpyth/Porcupine Notation		Porcupine			Pentatonic			Decatonic			Ups and Downs
0	0	Natural Unison	1	perfect unison	P1	D	perfect unison	P1	D	natural 1st	N1	C	perfect unison	P1	D
1	55	s-minor second	sm2	aug unison	A1	D#	aug unison	A1	D#	flat 2nd	f2	C#, δb	minor 2nd	m2	Eb
2	109	p-diminished second	pd2	dim 2nd	d2	Eb	double-aug unison, double-dim sub3rd	AA1, dds3	Dx, Fb3	natural 2nd	N2	δ	upminor 2nd	^m2	^Eb
3	164	p-minor second	pm2	perfect 2nd	P2	E	dim sub3rd	ds3	Fbb	sharp 2nd, flat 3rd	s2, f3	δ#, Db	downmajor 2nd	vM2	vE
4	218	(s/p) Major second	M2	aug 2nd	A2	E#	minor sub3rd	ms3	Fb	natural 3rd	N3	D	major 2nd	M2	E
5	273	s-minor third	sm3	dim 3rd	d3	Fb	major sub3rd	Ms3	F	sharp 3rd	s3	D#	minor 3rd	m3	F
6	327	p-minor third	pm3	minor 3rd	m3	F	aug sub3rd	As3	F#	flat 4th	f4	εb	upminor 3rd	^m3	^F
7	382	p-Major third	pM3	major 3rd	M3	F#	double-aug sub3rd, double-dim 4thoid	AAs3, dd4d	Fx, Gbb	natural 4th	N4	ε	downmajor 3rd	vM3	vF#
8	436	s-Major third	sM3	aug 3rd, dim 4th	A3, d4	Fx, Gb	dim 4thoid	d4d	Gb	sharp 4th, flat 5th	s4, f5	ε#, Eb	major 3rd	M3	F#
9	491	Natural Fourth	4, N4	minor 4th	m4	G	perfect 4thoid	P4d	G	natural 5th	N5	E	perfect fourth	P4	G
10	545	p-Major fourth, s-dim fifth	pM4, sd5	major 4th	M4	G#	aug 4thoid	A4d	G#	sharp 5th, flat 6th	s5, f6	E#, γb	up-4th, dim 5th	^4, d5	^G, Ab
11	600	p-Augmented Fourth, p-diminished Fifth Half-Octave	A4, HO	aug 4th, dim 5th	A4, d5	Gx, Abb	double-aug 4thoid, double-dim 5thoid	AA4d, dd5d	Gx, Abb	natural 6th	N6	γ	downaug 4th, updim 5th	vA4, ^d5	vG#, ^Ab
12	655	p-minor Fifth, s-aug Fourth	pm5, sA4	minor 5th	m5	Ab	dim 5thoid	d5d	Ab	sharp 6th, flat 7th	s6, f7	γ#, Gb	aug 4th, down-5th	A4, v5	G#, vA
13	709	Natural Fifth	5, N5	major 5th	M5	A	perfect 5thoid	P5d	A	natural 7th	N7	G	perfect 5th	P5	A
14	764	s-minor sixth	sm6	aug 5th, dim 6th	A5, d6	A#, Bbb	aug 5thoid	A5d	A#	sharp 7th	s7	G#	minor 6th	m6	Bb
15	818	p-minor sixth	pm6	minor 6th	m6	Bb	double-aug 5thoid, double-dim sub7th	AA5d, dds7	Ax, Cb3	flat 8th	f8	αb	upminor 6th	^m6	^Bb
16	873	p-Major sixth	pM6	major 6th	M6	B	dim sub7th	ds7	Cbb	natural 8th	N8	α	downmajor 6th	vM6	vB
17	927	s-Major sixth	sM6	aug 6th	A6	B#	minor sub7th	ms7	Cb	sharp 8th, flat 9th	s8, f9	α#, Ab	major 6th	M6	B
18	982	(s/p) minor seventh	m7	dim 7th	d7	Cb	major sub7th	Ms7	C	natural 9th	N9	A	minor 7th	m7	C
19	1036	p-Major seventh	pM7	perfect 7th	P7	C	aug sub7th	As7	C#	sharp 9th, flat 10th	s9, f10	A#, βb	upminor 7th	^m7	^C
20	1091	p-Augmented seventh	pA7	aug 7th	A7	C#	double-aug sub7th, double-dim octave	AAs7, dd8	Cx, Dbb	natural 10th	N10	β	downmajor 7th	vM7	vC#
21	1145	s-Major seventh	sM7	dim 8ve	d8	Db	dim octave	d8	Db	sharp 10th	s10	β#, Cb	major 7th	M7	C#
22	1200	Octave	8	perfect octave	P8	D	perfect octave	P8	D	natural 11th	N11	C	perfect octave	P8	D

コードネーム

Combining ups and downs notation with color notation, qualities can be loosely associated with colors:

quality	color name	monzo format	examples
minor	zo	[a b 0 1>	7/6, 7/4
minor	fourthward wa	[a b> where b < -1	32/27, 16/9
upminor	gu	[a b -1>	6/5, 9/5
downmajor	yo	[a b 1>	5/4, 5/3
major	fifthward wa	[a b> where b > 1	9/8, 27/16
major	ru	[a b 0 -1>	9/7, 12/7

All 22edo chords can be named using ups and downs. Alterations are always enclosed in parentheses, additions never are. An up or down immediately after the chord root affects the 3rd, 6th, 7th, and/or the 11th (every other note of a stacked-3rds chord 6-1-3-5-7-9-11-13).Here are the zo, gu, yo and ru triads:

color of the 3rd	JI chord	notes as edosteps	notes of C chord	written name	spoken name
zo	6:7:9	0-5-13	C Eb G	Cm	C minor
gu	10:12:15	0-6-13	C ^Eb G	C^m	C upminor
yo	4:5:6	0-7-13	C vE G	Cv	C downmajor or C down
ru	14:18:21	0-8-13	C E G	C	C major or C

Examples:

0-4-13 = C D G = C2
0-9-13 = C F G = C4
0-10-13 = C ^F G = C^4 or C(^4)
0-5-10 = C Eb Gb = Cd = Cdim
0-5-11 = C Eb ^Gb = Cd(^5)
0-5-12 = C Eb vG = Cm(v5)

Further discussion of 22edo chord naming:

音楽

Main article: 22edo/Music

See also: Category:22edo tracks

外部リンク

参考文献

Barbour, James Murray, Tuning and temperament, a historical survey, East Lansing, Michigan State College Press, 1953 [c1951]
Bosanquet, R.H.M. On the Hindoo division of the octave, with additions to the theory of higher orders, Proceedings of the Royal Society of London vol. 26, 1879, pp. 272-284. Reproduced in Tagore, Sourindro Mohun, Hindu Music from Various Authors, Chowkhamba Sanskrit Series, Varanasi, India, 1965

[1] 22平均律を2.3.5.7.11.17サブグループ音律として扱うことに基づいて、サイズの大きい順に並べられたいくつかの単純な比率。他のアプローチも可能。

[2] Ratios longer than 10 digits are presented by placeholders with informative hints

[1]

[1]