User:CritDeathX/Sam's Musings
This is the page that I will use to just splatter my thoughts on; I think most of it will center around notation & regular temperaments.
Titanium(9) Notation for 14EDO
To quote the Titanium(9) section of the 14EDO page,
"14edo is also the largest edo whose patent val supports titanium temperament, tempering out the chromatic semitone (21:20), and falling toward the "brittle" (fifths wider than in 9edo) end of that spectrum. Titanium is one of the simplest 7-limit temperaments, although rather inaccurate (the 7:5 is mapped onto 6\14, over 70 cents flat). Its otonal/major and utonal/minor tetrads are inversions of one another, which allows a greater variety of chord progressions (since different inversions of the same chord may have very different expressive qualities). Despite being so heavily tempered, the tetrads are still recognizable and aren't unpleasant-sounding as long as one uses the right timbres ("bell-like" or opaque-sounding ones probably work best). Titanium forms enneatonic modes which are melodically strong and are very similar to diatonic modes, only with two mediants and submediants instead of one. Titanium[9] has similarities to mavila, slendro, and pelog scales as well."[1]
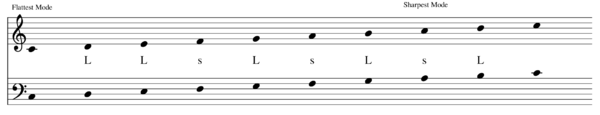
This notation places the clefs in the same places as 5 lines do; the treble clef (G clef) is placed on the 2nd line, and the bass clef (F clef) is placed on the 4th. Starting from the 1st ledger line to the 4th space on the treble clef & the 2nd space to the 1st ledger line above for the bass clef, the note names are C-D-E-F-G-H-J-A-B-C'.
Although (i believe) my notation doesn't follow the interval list it speaks of, I believe it still does its job in notating 14EDO in a 6-line staff.
Diatonic Notation
Chromatic Notation
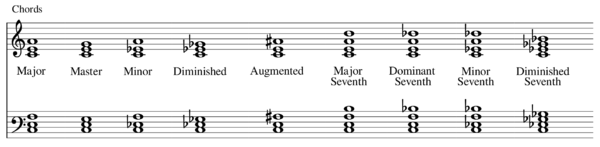
Basic Chords
2 Basic Progressions (and also an example of a time signature)
Christopher Temperament
Christopher temperament is the name I give to the 2.5.7.9.11.13-limit temperament with a generator of a sharp 7/6 that tempers out 99/98, 44/43, 17496/16807, etc. It would be a generator of Orwell if it wasn't for the fact that its too sharp. Two generators give an 8c sharp 11/8, three of them make a 0.7c flat 13/8, and five make a 4c flat 9/8.
Using a scale tree, you can find the EDOs that approximate the generator, starting with 13 & 17EDO; you could also find a zigzag series of EDOs that approximate the generator, starting with 13 & 30.
As of 3/23/2020, I got really bored and I made a little resolution in the 4L5s scale of Christopher. Hopefully this demonstrates the harmony that can be used. 1/1 is A.
Interval Chain
| 1000.28 | 80.22 | 360.17 | 640.11 | 920.06 | 0.0 | 279.94 | 559.89 | 839.83 | 1119.78 | 199.72 |
|---|---|---|---|---|---|---|---|---|---|---|
| 16/9 +4c | 22/21 | 16/13 | 16/11 -8c | 12/7 -12c | 1/1 | 7/6 +12c | 11/8 +8c | 13/8 | 21/11 | 9/8 -4c |
MOS Scale
(charts inspired from Andrew Heathwaite's MOS Investigations)
4L1s
| 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| 0.0 | ||||
| 279.94 | ||||
| 559.89 | ||||
| 839.83 | ||||
| 1119.78 |
L = 279.94 (1x)
s = 80.22 (-4x)
c = 199.72 (5x)
4L5s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|
| 0.0 | ||||||||
| 199.72 | ||||||||
| 279.94 | ||||||||
| 479.67 | ||||||||
| 559.89 | ||||||||
| 759.61 | ||||||||
| 839.83 | ||||||||
| 1039.56 | ||||||||
| 1119.78 |
L = 199.72 (5x)
s = 80.22 (-4x)
c = 119.50 (9x)
4L9s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.0 | ||||||||||||
| 119.50 | ||||||||||||
| 199.72 | ||||||||||||
| 279.94 | ||||||||||||
| 399.44 | ||||||||||||
| 479.67 | ||||||||||||
| 559.89 | ||||||||||||
| 679.39 | ||||||||||||
| 759.61 | ||||||||||||
| 839.83 | ||||||||||||
| 959.33 | ||||||||||||
| 1039.56 | ||||||||||||
| 1119.78 |
L = 119.50 (9x)
s = 80.22 (-4x)
c = 39.28 (13x)
A Pattern for Christopher Temperament
The interesting pattern while writing this was that you had a pretty simple procedure to find sizes of this temperament; your L will usually be s generators away, and visa versa. In order to find the next MOS (and incidentally the size of L in the next MOS), just find the chroma (L-s) in generator steps.
For example, in 4L1s, the L is 1 generator up; s is 4 generators down. The chroma is 9 generators up, and is also the L of 4L5s.
As of 3/29/2020, I'm realizing this may be evident in all of temperaments; if not all, then a lot of them.
Resolutions in Temperaments
As of 3/23/2020, I got really bored and I made a little resolution in the 4L5s scale of Christopher. I decided to try and record some progressions in various temperaments after realizing how easy it is to bust them out and sing microtonally. These are all using 4 voices of varying ranges. I hope you enjoy.
| Christopher 4L5s
Halthird Temperament
Halthird temperament is the name I give to my first half-octave temperament. It has a generator of a slightly sharp 7/6, and a limit of 2.5.7.9.11.
Using a scale tree, you can find EDOs that have this generator, starting with 7 & 11EDO. There's a zigzag pattern starting with 25 and 68EDO, though if you want a zigzag from the starting point, there's one between 7 & 18EDO.
I also don't really know how to properly make MOS scales in this, so there will be no MOS scale diagrams here.
Interval Chain
| 549.99 | 814.28 | 1078.57 | 142.85 | 407.14 | 671.43 | 935.71 | 0.0 | 264.29 | 528.57 | 792.86 | 1057.15 | 121.43 | 385.72 | 650.01 |
| 11/8 | 8/5 | 28/15 | 12/11 -8c | 81/64 | 28/19 | 12/7 | 1/1 | 7/6 | 19/14 | 128/81 | 11/6 +8c | 15/14 | 5/4 | 16/11 |
| 1149.99 | 214.28 | 478.57 | 742.85 | 1007.14 | 71.43 | 335.71 | 600.0 | 864.29 | 1128.57 | 192.86 | 457.15 | 721.43 | 985.72 | 50.01 |
| 64/33 | 26/23 | 29/22 | 20/13 | 16/9 +11c | 25/24 | 17/14 | 7/5 +18c | 28/17 | 48/25 | 9/8 -11c | 13/10 | 44/29 | 23/13 | 33/32 |
MOS Scales
Stepped Temperament
Stepped temperament is the name I give to the 2.3.5.9.10.11.13.29.31-limit temperament with a generator of a flat 5/4 that tempers out 967458816/847425747, 16325867520/13841287201, 823543/629856, etc. Obviously, this is a high complexity temperament, though the good thing is that you have two major thirds that you can choose from; a 12c sharp third (appears after 15 generators) or a 15c flat third (the generator). I tried to demonstrate this fact in the limit, though I have a feeling there's a more accurate way of writing it. A similar occurrence happens in pseudo-semaphore, where the 3rd harmonic has 2 different mappings.
Another thing you may notice is the size of the limit; this is the largest limit that I've seen as of 4/8/2020. Considering I'm only sticking under 31, this is still a sizable limit.
Using a scale tree, you can find EDOs that have this generator, starting with 13 & 16EDO. There's a zigzag pattern starting with 13 & 42EDO, though if you want a more linear pattern, there's one between 13 and 29EDO.
Interval Chain
| 258.28 | 629.59 | 1000.89 | 172.19 | 543.49 | 914.79 | 86.09 | 457.40 | 828.70 | 0.0 | 371.30 | 742.60 | 1113.91 | 285.21 | 656.51 | 1027.81 | 199.11 | 570.41 | 941.72 |
| 22/19 -4c | 36/25 | 16/9 +5c | 32/29 | 11/8 -8c | 22/13 | 20/19 | 13/10 | 8/5 +15c | 1/1 | 5/4 -15c | 20/13 | 19/10 | 13/11 +4c | 16/11 +8c | 29/16 | 9/8 -5c | 25/18 | 19/11 +4c |
MOS Scales
3L4s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|
| 0.0 | ||||||
| 285.21 | ||||||
| 371.30 | ||||||
| 656.51 | ||||||
| 742.60 | ||||||
| 1027.81 | ||||||
| 1113.91 |
L = 285.21 (4x)
s = 86.09 (-3x)
c = 199.11 (7x)
3L7s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|---|
| 0.0 | |||||||||
| 199.11 | |||||||||
| 285.21 | |||||||||
| 371.30 | |||||||||
| 570.41 | |||||||||
| 656.51 | |||||||||
| 742.60 | |||||||||
| 941.72 | |||||||||
| 1027.81 | |||||||||
| 1113.91 |
L = 199.11 (7x)
s = 86.09 (-3x)
c = 113.02 (10x)
3L10s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.0 | ||||||||||||
| 113.02 | ||||||||||||
| 199.11 | ||||||||||||
| 285.21 | ||||||||||||
| 371.30 | ||||||||||||
| 484.32 | ||||||||||||
| 570.41 | ||||||||||||
| 656.51 | ||||||||||||
| 742.60 | ||||||||||||
| 855.62 | ||||||||||||
| 941.72 | ||||||||||||
| 1027.81 | ||||||||||||
| 1113.91 |
L = 113.02 (10x)
s = 86.09 (-3x)
c = 26.92 (13x)
Sothuyo Temperament
Sothuyo temperament is the name I give to the 2.3.5.13.17-limit temperament with a generator of a slightly sharp 13/10 that tempers out 170/169, 6250000000/5931980229, etc. The name for this temperament comes from the colour notation name of 170/169, which is 17o3u2yb1.
Using a scale tree, you can find EDOs that support this generator, starting with 8 and 13EDO. There's a zigzag pattern between 13 and 21EDO.
Interval Chain
| 387.88 | 846.76 | 105.63 | 564.51 | 1023.38 | 282.25 | 741.13 | 0.0 | 458.87 | 917.75 | 176.62 | 635.49 | 1094.37 | 353.24 | 812.12 |
| 5/4 | 18/11 -6c | 16/15 -6c | 18/13 | 9/5 +5c | 20/17 | 20/13 -5c | 1/1 | 13/10 +5c | 17/10 | 10/9 -5c | 13/9 | 15/8 +6c | 11/9 +6c | 8/5 |
MOS Scales
3L2s
| 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| 0.0 | ||||
| 176.62 | ||||
| 458.87 | ||||
| 635.49 | ||||
| 917.75 |
L = 282.25 (-2x)
s = 176.62 (3x)
c = 105.63 (-5x)
5L3s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|
| 0.0 | |||||||
| 176.62 | |||||||
| 353.24 | |||||||
| 458.87 | |||||||
| 635.49 | |||||||
| 812.12 | |||||||
| 917.75 | |||||||
| 1094.37 |
L = 176.62 (3x)
s = 105.63 (-5x)
c = 70.99 (8x)
5L8s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.0 | ||||||||||||
| 70.99 | ||||||||||||
| 176.62 | ||||||||||||
| 247.610 | ||||||||||||
| 353.24 | ||||||||||||
| 458.87 | ||||||||||||
| 529.86 | ||||||||||||
| 635.49 | 706.48 | |||||||||||
| 812.12 | ||||||||||||
| 917.75 | ||||||||||||
| 988.74 | ||||||||||||
| 1094.37 |
L = 105.63 (-5x)
s = 70.99 (8x)
c = 34.64258 (-13x)
Ocean Temperament
Ocean temperament is the name I give to the 13-limit temperament with a generator of a slightly sharp 6/5 that tempers out 100/99, 875/864, 21875/21384, etc. Two generators give 16/11, three give 7/4, and five give 14/11.
Using a scale tree, you can find EDOs that support this generator, starting with 11 and 15EDO. Using my slightly better knowledge about zigzags in scale trees, the zigzag of EDOs start with 11-15-26.
On a side note, I believe I have found a better way to find new generators; usually, I would type something like 927.927364 into Scale Workshop and pray to whatever god there is that the MOS scales are good. The problem with this method is that these would usually give very small s steps (sometimes around 3 cents or so) or the generator is Orwell. How I found this temperament is focusing on EDO steps (something like 52\107); this is slightly better for me, as there's a bit more of a good sense as to if it will cause small s steps or not.
Interval Chain
| 131.63 | 455.68 | 779.74 | 1103.79 | 227.84 | 551.89 | 875.95 | 0.0 | 324.05 | 648.11 | 972.16 | 96.21 | 420.26 | 744.32 | 1068.37 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 14/13 +4c | 13/10 | 11/7 | 17/9 +3c | 8/7 -3c | 11/8 | 5/3 -8c | 1/1 | 6/5 +8c | 16/11 | 7/4 +3c | 18/17 -3c | 14/11 | 20/13 | 13/7 -4c |
Eigenmonzos
| 7/4 | 322.94196882304163 |
| 14/11 | 323.50159282087367 |
| 3/2 | 323.64441181561097 |
| 13/8 | 324.0527661769311 |
| 5/4 | 324.1446071165522 |
| 16/11 | 324.3410288176217 |
| 9/8 | 325.48875021634683 |
MOS Scales
(note; these scales would be better with equal generators up & down [for example, the first scale could be 3|3 in Modal UDP Notation])
4L3s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|
| 0.0 | ||||||
| 96.21 | ||||||
| 324.05 | ||||||
| 420.26 | ||||||
| 648.11 | ||||||
| 744.32 | ||||||
| 972.16 |
L = 227.84 (-3x)
s = 96.21 (4x)
c = 131.63 (-7x)
4L7s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.0 | ||||||||||
| 96.21 | ||||||||||
| 192.42 | ||||||||||
| 324.05 | ||||||||||
| 420.26 | ||||||||||
| 516.48 | ||||||||||
| 648.11 | ||||||||||
| 744.32 | ||||||||||
| 840.53 | ||||||||||
| 972.16 | ||||||||||
| 1068.37 |
L = 131.63 (-7x)
s = 96.21 (4x)
c = 35.42 (-11x)
4L11s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.0 | ||||||||||||||
| 96.21 | ||||||||||||||
| 192.42 | ||||||||||||||
| 288.63 | ||||||||||||||
| 324.05 | ||||||||||||||
| 420.26 | ||||||||||||||
| 516.48 | ||||||||||||||
| 612.69 | ||||||||||||||
| 648.11 | ||||||||||||||
| 744.32 | ||||||||||||||
| 840.53 | ||||||||||||||
| 936.74 | ||||||||||||||
| 972.16 | ||||||||||||||
| 1068.37 | ||||||||||||||
| 1164.58 |
L = 96.21 (4x)
s = 35.42 (-11x)
c = 61.79 (-15x)
Worell Temperament
Worell temperament is the name I give to the 11-limit temperament with a generator of a slightly flat 7/6 that tempers out 343/324, 117649/116640, 9058973/8957952, etc. Six generators give 5/4, seven generators give 16/11, ten generators give 8/7, and eleven generators give 4/3. It is named worell temperament because of the perceived similarity to orwell, except for higher complexity for its primes; for example, orwell takes three generators to get to 5/4, while worell takes twice as many generators.
Using a scale tree, you can find EDOs that support this generator, starting with 9 & 14EDO. Admittedly, I don't enjoy 14EDO's approximation of 7/6, but the website I'm using can only go up to 19 notes.
Its not a temperament that I'm too proud of, mainly from the fact I realized while writing this that this was already a generator for halthird temperament. Also, the L:s ratio gets a bit wonky at the 14-note scale.
Interval Chain
| 815.640 | 1079.700 | 143.760 | 407.820 | 671.880 | 935.940 | 0.0 | 264.060 | 528.120 | 792.180 | 1056.240 | 120.300 | 384.360 |
| 8/5 | 28/15 | 12/11 -7c | 81/64 | 40/27 -9c | 12/7 | 1/1 | 7/6 | 27/20 +9c | 128/64 | 11/6 +7c | 15/14 | 5/4 |
Eigenmonzos
| 40/27 | 259.77564436566377 |
| 7/4 | 263.1174093530875 |
| 3/2 | 263.45863628496477 |
| 81/64 | 264.05999884615005 |
| 11/8 | 264.09743680503476 |
| 5/4 | 264.38561897747246 |
| 7/6 | 266.87090560373764 |
MOS Scales
4L1s
| 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| 0.0 | ||||
| 264.060 | ||||
| 528.120 | ||||
| 792.180 | ||||
| 1056.240 |
L = 264.060 (1x)
s = 143.760 (-4x)
c = 120.300 (5x)
4L5s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|
| 0.0 | ||||||||
| 120.300 | ||||||||
| 264.060 | ||||||||
| 384.360 | ||||||||
| 528.120 | ||||||||
| 648.420 | ||||||||
| 792.180 | ||||||||
| 912.480 | ||||||||
| 1056.240 |
L = 143.760 (-4x)
s = 120.300 (5x)
c = 23.460 (-9x)
9L5s
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.0 | |||||||||||||
| 120.300 | |||||||||||||
| 240.600 | |||||||||||||
| 264.060 | |||||||||||||
| 384.360 | |||||||||||||
| 504.660 | |||||||||||||
| 528.120 | |||||||||||||
| 648.420 | |||||||||||||
| 768.720 | |||||||||||||
| 792.180 | |||||||||||||
| 912.480 | |||||||||||||
| 1032.780 | |||||||||||||
| 1056.240 | |||||||||||||
| 1176.540 |
L = 120.300 (5x)
s = 23.460 (-9x)
c = 96.960 (14x)