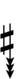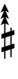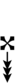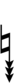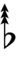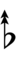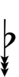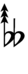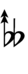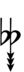42edo
| ← 41edo | 42edo | 43edo → |
42 equal divisions of the octave (abbreviated 42edo or 42ed2), also called 42-tone equal temperament (42tet) or 42 equal temperament (42et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 42 equal parts of about 28.6 ¢ each. Each step represents a frequency ratio of 21/42, or the 42nd root of 2.
Theory
42edo has a patent val fifth (the step of which is not from 7edo, this being a first for edos of the form 7n) and a third both over 12 cents sharp, using the same 400-cent interval to represent 5/4 as does 12edo, which means it tempers out 128/125. In the 7-limit, it tempers out 64/63 and 126/125, making it a tuning supporting the augene temperament.
42edo is on the optimal ET sequence of the eugene, joan, lemba, neutron, qeema, seville, sevond, skateboard, tritikleismic and vines temperaments.
42edo is a diatonic edo because its 5th falls between 4\7 = 686 ¢ and 3\5 = 720 ¢. 42edo is one of the most difficult diatonic edos to notate, because no other diatonic edo's fifth is as sharp (see 47edo for the opposite extreme).
Odd harmonics
While not an accurate tuning on the full 7-limit, 42edo does an excellent job on the 2.9.15.7.33.39 2*42 subgroup, having the same tuning on it as does 84edo. On this subgroup 42 has the same commas as 84.
| Harmonic | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 | 21 | 23 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +12.3 | +13.7 | +2.6 | -3.9 | -8.5 | -12.0 | -2.6 | +9.3 | -11.8 | -13.6 | +0.3 |
| Relative (%) | +43.2 | +47.9 | +9.1 | -13.7 | -29.6 | -41.8 | -8.9 | +32.7 | -41.3 | -47.7 | +1.0 | |
| Steps (reduced) |
67 (25) |
98 (14) |
118 (34) |
133 (7) |
145 (19) |
155 (29) |
164 (38) |
172 (4) |
178 (10) |
184 (16) |
190 (22) | |
Subsets and supersets
Since 42 factors into 2 × 3 × 7, 42edo contains subset edos 2, 3, 6, 7, 14, and 21.
Intervals
| # | Cents | Ups and downs notation | ||
|---|---|---|---|---|
| 0 | 0.0 | P1 | perfect unison | D |
| 1 | 28.6 | ^1, m2 | up unison, minor 2nd | ^D, Eb |
| 2 | 57.1 | ^^1, ^m2 | dup 1sn, upminor 2nd | ^^D, ^Eb |
| 3 | 85.7 | ^^m2 | dupminor 2nd | ^^Eb |
| 4 | 114.3 | ^3m | trupminor 2nd | ^3Eb |
| 5 | 143.9 | v3M | trudmajor 2nd | v3E |
| 6 | 171.4 | vvM2 | dudmajor 2nd | vvE |
| 7 | 200.0 | vM2 | downmajor 2nd | vE |
| 8 | 228.6 | M2 | major 2nd | E |
| 9 | 257.1 | m3 | minor 3rd | F |
| 10 | 285.7 | ^m3 | upminor 3rd | ^F |
| 11 | 314.3 | ^^m3 | dupminor 3rd | ^^F |
| 12 | 342.9 | ^3m3 | trupminor 3rd | ^3F |
| 13 | 371.4 | v3M3 | trudmajor 3rd | v3F# |
| 14 | 400.0 | vvM3 | dudmajor 3rd | vvF# |
| 15 | 428.6 | vM3 | downmajor 3rd | vF# |
| 16 | 457.1 | M3, v4 | major 3rd, down 4th | F#, vG |
| 17 | 485.7 | P4 | perfect 4th | G |
| 18 | 514.3 | ^4 | up 4th | ^G |
| 19 | 543.9 | ^^4 | dup 4th | ^^G |
| 20 | 571.4 | ^34, ^^d5 | trup 4th, dupdim 5th | ^3G, ^^Ab |
| 21 | 600.0 | v3A4, ^3d5 | trudaug 4th, trupdim 5th | v3G#, ^3Ab |
| 22 | 628.6 | vvA4, v35 | dudaug 4th, trud 5th | vvG#, v3A |
| 23 | 657.1 | vv5 | dud 5th | vvA |
| 24 | 685.7 | v5 | down 5th | vA |
| 25 | 714.3 | P5 | perfect 5th | A |
| 26 | 742.9 | ^5, m6 | up 5th, minor 6th | ^A, Bb |
| 27 | 771.4 | ^m6 | upminor 6th | ^Bb |
| 28 | 800.0 | ^^m6 | dupminor 6th | ^^Bb |
| 29 | 828.6 | ^3m6 | trupminor 6th | ^3Bb |
| 30 | 857.1 | v3M6 | trudmajor 6th | v3B |
| 31 | 885.7 | vvM6 | dudmajor 6th | vvB |
| 32 | 914.3 | vM6 | downmajor 6th | vB |
| 33 | 942.9 | M6 | major 6th | B |
| 34 | 971.4 | m7 | minor 7th | C |
| 35 | 1000.0 | ^m7 | upminor 7th | ^C |
| 36 | 1028.6 | ^^m7 | dupminor 7th | ^^C |
| 37 | 1057.1 | ^3m7 | trupminor 7th | ^3C |
| 38 | 1085.7 | v3M7 | trudmajor 7th | v3C# |
| 39 | 1114.3 | vvM7 | dudmajor 7th | vvC# |
| 40 | 1142.9 | vM7 | downmajor 7th | vC# |
| 41 | 1171.4 | M7, v8 | major 7th, down 8ve | C#, vD |
| 42 | 1200.0 | P8 | perfect 8ve | D |
Chords can be named using ups and downs as C upminor, D downmajor seven, etc. See Ups and downs notation #Chords and chord progressions.
Notation
Ups and downs notation
Assuming the natural notes form a chain of fifths, the major 2nd is 8 edosteps and the minor 2nd is only one. The naturals create a 5edo-like scale, with two of the notes inflected by a comma-sized edostep:
D * * * * * * * E F * * * * * * * G * * * * * * * A * * * * * * * B C * * * * * * * D
D♯ is next to E. The notation requires ups and downs with three arrows, and if chords are to be spelled correctly four or more arrows may be required in certain cases. For example, a 1/1 – 5/4 – 3/2 – 9/5 chord with a root on the edostep midway between G and A would be written either as v3G♯–v5B♯ – v3D♯ – vF♯ or as ^3A♭ – ^C – ^3E♭ – ^5G♭. This is a dud dup-seven chord, written either as v3G♯vv,^^7 or as ^3A♭vv,^^7.
In this table, dup is equivalent to quidsharp, trup is equivalent to quudsharp, trudsharp is equivalent to quup, dudsharp is equivalent to quip, etc.
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |
||||||||||||||
| Flat symbol |
Alternatively, sharps and flats with arrows borrowed from Helmholtz–Ellis notation can be used:
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |
|||||||||||||||||
| Flat symbol |
Sagittal notation
Best fifth notation
This notation uses the same sagittal sequence as 35b.
Evo flavor
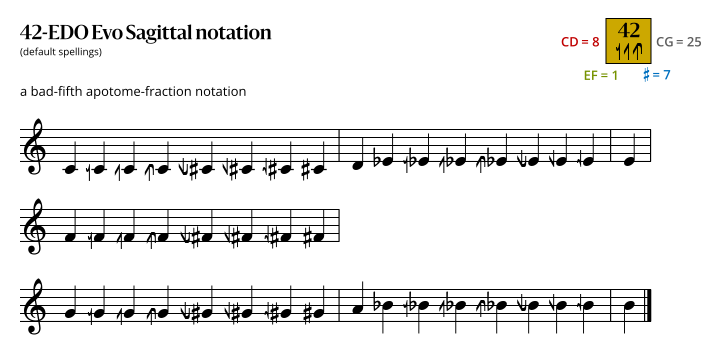
Revo flavor
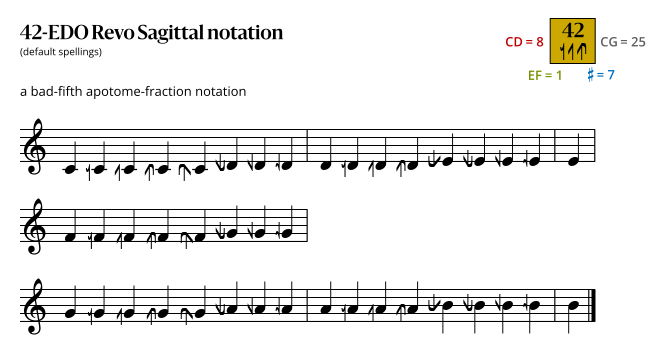
Second-best fifth notation
This notation uses the same sagittal sequence as 47edo, and is a superset of the notations for edos 21, 14, and 7.

Approximation to JI
The following tables show how 15-odd-limit intervals are represented in 42edo. Prime harmonics are in bold; inconsistent intervals are in italics.
| Interval and complement | Error (abs, ¢) | Error (rel, %) |
|---|---|---|
| 1/1, 2/1 | 0.000 | 0.0 |
| 5/3, 6/5 | 1.356 | 4.7 |
| 15/8, 16/15 | 2.554 | 8.9 |
| 7/4, 8/7 | 2.603 | 9.1 |
| 13/10, 20/13 | 2.929 | 10.3 |
| 13/11, 22/13 | 3.495 | 12.2 |
| 9/8, 16/9 | 3.910 | 13.7 |
| 13/12, 24/13 | 4.284 | 15.0 |
| 11/9, 18/11 | 4.551 | 15.9 |
| 15/14, 28/15 | 5.157 | 18.0 |
| 15/11, 22/15 | 5.906 | 20.7 |
| 11/10, 20/11 | 6.424 | 22.5 |
| 9/7, 14/9 | 6.513 | 22.8 |
| 11/6, 12/11 | 7.780 | 27.2 |
| 13/9, 18/13 | 8.046 | 28.2 |
| 11/8, 16/11 | 8.461 | 29.6 |
| 15/13, 26/15 | 9.402 | 32.9 |
| 7/6, 12/7 | 9.728 | 34.0 |
| 9/5, 10/9 | 10.975 | 38.4 |
| 11/7, 14/11 | 11.063 | 38.7 |
| 7/5, 10/7 | 11.084 | 38.8 |
| 13/8, 16/13 | 11.956 | 41.8 |
| 3/2, 4/3 | 12.331 | 43.2 |
| 5/4, 8/5 | 13.686 | 47.9 |
| 13/7, 14/13 | 14.013 | 49.0 |
| Interval and complement | Error (abs, ¢) | Error (rel, %) |
|---|---|---|
| 1/1, 2/1 | 0.000 | 0.0 |
| 5/3, 6/5 | 1.356 | 4.7 |
| 7/4, 8/7 | 2.603 | 9.1 |
| 13/11, 22/13 | 3.495 | 12.2 |
| 11/8, 16/11 | 8.461 | 29.6 |
| 7/6, 12/7 | 9.728 | 34.0 |
| 9/5, 10/9 | 10.975 | 38.4 |
| 11/7, 14/11 | 11.063 | 38.7 |
| 7/5, 10/7 | 11.084 | 38.8 |
| 13/8, 16/13 | 11.956 | 41.8 |
| 3/2, 4/3 | 12.331 | 43.2 |
| 5/4, 8/5 | 13.686 | 47.9 |
| 13/7, 14/13 | 14.559 | 51.0 |
| 11/6, 12/11 | 20.792 | 72.8 |
| 9/7, 14/9 | 22.059 | 77.2 |
| 11/10, 20/11 | 22.147 | 77.5 |
| 15/14, 28/15 | 23.414 | 82.0 |
| 13/12, 24/13 | 24.287 | 85.0 |
| 9/8, 16/9 | 24.661 | 86.3 |
| 13/10, 20/13 | 25.643 | 89.7 |
| 15/8, 16/15 | 26.017 | 91.1 |
| 11/9, 18/11 | 33.122 | 115.9 |
| 15/11, 22/15 | 34.478 | 120.7 |
| 13/9, 18/13 | 36.618 | 128.2 |
| 15/13, 26/15 | 37.973 | 132.9 |
Regular temperament properties
| Subgroup | Comma list | Mapping | Optimal 8ve stretch (¢) |
Tuning error | |
|---|---|---|---|---|---|
| Absolute (¢) | Relative (%) | ||||
| 2.3 | [67 -42⟩ | [⟨42 67]] | −3.89 | 3.88 | 13.57 |
| 2.3.5 | 128/125, 5000000/4782969 | [⟨42 67 98]] | −4.55 | 3.30 | 11.55 |
| 2.3.5.7 | 64/63, 126/125, 6860/6561 | [⟨42 67 98 118]] | −3.65 | 3.26 | 11.42 |
Octave stretch or compression
42edo’s inaccurate 3rd and 5th harmonics can be greatly improved through stretching or compressing octaves. Both approaches work about equally well but in opposite directions, giving two quite different flavors of tuning to play with.
What follows is a comparison of stretched- and compressed-octave 42edo tunings.
- Octave size: 1206.3 ¢
Stretching the octave of 42edo by around 6 ¢ results in improved primes 3, 5 and 13, but worse primes 2, 7 and 11. This approximates all harmonics up to 16 within 13.3 ¢. The tuning 108ed6 does this. So does the tuning 97ed5 whose octave differs by only 0.1 ¢.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +6.3 | -6.3 | +12.6 | -0.3 | +0.0 | -8.4 | -9.8 | -12.6 | +6.0 | +13.3 | +6.3 |
| Relative (%) | +22.0 | -22.0 | +44.0 | -1.0 | +0.0 | -29.2 | -34.0 | -44.0 | +21.0 | +46.5 | +22.0 | |
| Steps (reduced) |
42 (42) |
66 (66) |
84 (84) |
97 (97) |
108 (0) |
117 (9) |
125 (17) |
132 (24) |
139 (31) |
145 (37) |
150 (42) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +11.4 | -2.1 | -6.6 | -3.5 | +6.5 | -6.3 | -13.8 | +12.3 | +14.0 | -9.1 | +0.1 | +12.6 |
| Relative (%) | +39.5 | -7.2 | -23.0 | -12.0 | +22.5 | -22.0 | -47.9 | +42.9 | +48.9 | -31.6 | +0.5 | +44.0 | |
| Steps (reduced) |
155 (47) |
159 (51) |
163 (55) |
167 (59) |
171 (63) |
174 (66) |
177 (69) |
181 (73) |
184 (76) |
186 (78) |
189 (81) |
192 (84) | |
- Step size: 28.689 ¢, octave size: 1204.9 ¢
Stretching the octave of 42edo by around 5 ¢ results in improved primes 3, 5 and 13, but worse primes 2 and 11. This approximates all harmonics up to 16 within 13.9 ¢. The tuning 189zpi does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +4.9 | -8.5 | +9.9 | -3.5 | -3.5 | -12.2 | -13.9 | +11.7 | +1.5 | +8.6 | +1.4 |
| Relative (%) | +17.2 | -29.6 | +34.4 | -12.1 | -12.3 | -42.6 | -48.4 | +40.9 | +5.1 | +29.9 | +4.9 | |
| Step | 42 | 66 | 84 | 97 | 108 | 117 | 125 | 133 | 139 | 145 | 150 | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +6.3 | -7.3 | -12.0 | -8.9 | +0.9 | -12.0 | +9.1 | +6.4 | +8.0 | +13.5 | -6.1 | +6.3 |
| Relative (%) | +21.8 | -25.4 | -41.7 | -31.2 | +3.0 | -41.9 | +31.8 | +22.3 | +27.9 | +47.1 | -21.1 | +22.1 | |
| Step | 155 | 159 | 163 | 167 | 171 | 174 | 178 | 181 | 184 | 187 | 189 | 192 | |
- Octave size: 1204.5 ¢
Stretcing the octave of 42edo by around 4.5 ¢ results in improved primes 3, 5, 11 and 13, but worse primes 2 and 7. This approximates all harmonics up to 16 within 13.6 ¢. The tuning 150ed12 does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +4.5 | -9.1 | +9.1 | -4.4 | -4.5 | -13.3 | +13.6 | +10.5 | +0.2 | +7.2 | +0.0 |
| Relative (%) | +15.9 | -31.7 | +31.7 | -15.3 | -15.9 | -46.4 | +47.6 | +36.6 | +0.6 | +25.2 | +0.0 | |
| Steps (reduced) |
42 (42) |
66 (66) |
84 (84) |
97 (97) |
108 (108) |
117 (117) |
126 (126) |
133 (133) |
139 (139) |
145 (145) |
150 (0) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +4.8 | -8.8 | -13.5 | -10.5 | -0.7 | -13.6 | +7.5 | +4.7 | +6.3 | +11.8 | -7.8 | +4.5 |
| Relative (%) | +16.8 | -30.5 | -47.0 | -36.6 | -2.5 | -47.6 | +26.1 | +16.4 | +21.9 | +41.1 | -27.2 | +15.9 | |
| Steps (reduced) |
155 (5) |
159 (9) |
163 (13) |
167 (17) |
171 (21) |
174 (24) |
178 (28) |
181 (31) |
184 (34) |
187 (37) |
189 (39) |
192 (42) | |
- Octave size: 1202.5 ¢
Stretching the octave of 42edo by around 2.5 ¢ results in improved primes 5, 11 and 13, but worse primes 2 and 7. This approximates all harmonics up to 16 within 11.9 ¢. The tuning 145ed11 does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +2.5 | -12.4 | +4.9 | -9.2 | -9.9 | +9.5 | +7.4 | +3.9 | -6.8 | +0.0 | -7.5 |
| Relative (%) | +8.6 | -43.3 | +17.1 | -32.2 | -34.7 | +33.1 | +25.7 | +13.4 | -23.7 | +0.0 | -26.2 | |
| Steps (reduced) |
42 (42) |
66 (66) |
84 (84) |
97 (97) |
108 (108) |
118 (118) |
126 (126) |
133 (133) |
139 (139) |
145 (0) |
150 (5) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -2.9 | +11.9 | +7.0 | +9.8 | -9.3 | +6.3 | -1.4 | -4.3 | -2.9 | +2.5 | +11.4 | -5.0 |
| Relative (%) | -10.2 | +41.7 | +24.5 | +34.2 | -32.4 | +22.0 | -4.9 | -15.1 | -10.1 | +8.6 | +39.8 | -17.6 | |
| Steps (reduced) |
155 (10) |
160 (15) |
164 (19) |
168 (23) |
171 (26) |
175 (30) |
178 (33) |
181 (36) |
184 (39) |
187 (42) |
190 (45) |
192 (47) | |
- 42edo
- Step size: 28.571 ¢, octave size: 1200.0 ¢
Pure-octaves 42edo approximates all harmonics up to 16 within 13.7 ¢. The tuning 190zpi is almost exactly the same as pure-octaves 42edo, its octave differing by less than 0.05 ¢.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +0.0 | +12.3 | +0.0 | +13.7 | +12.3 | +2.6 | +0.0 | -3.9 | +13.7 | -8.5 | +12.3 |
| Relative (%) | +0.0 | +43.2 | +0.0 | +47.9 | +43.2 | +9.1 | +0.0 | -13.7 | +47.9 | -29.6 | +43.2 | |
| Steps (reduced) |
42 (0) |
67 (25) |
84 (0) |
98 (14) |
109 (25) |
118 (34) |
126 (0) |
133 (7) |
140 (14) |
145 (19) |
151 (25) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -12.0 | +2.6 | -2.6 | +0.0 | +9.3 | -3.9 | -11.8 | +13.7 | -13.6 | -8.5 | +0.3 | +12.3 |
| Relative (%) | -41.8 | +9.1 | -8.9 | +0.0 | +32.7 | -13.7 | -41.3 | +47.9 | -47.7 | -29.6 | +1.0 | +43.2 | |
| Steps (reduced) |
155 (29) |
160 (34) |
164 (38) |
168 (0) |
172 (4) |
175 (7) |
178 (10) |
182 (14) |
184 (16) |
187 (19) |
190 (22) |
193 (25) | |
- Step size: Octave size: 1199.1 ¢
Compressing the octave of 42edo by around 1 ¢ results in improved primes 3, 5 and 7, but worse primes 2, 11 and 13. This approximates all harmonics up to 16 within 13.2 ¢. The tuning 118ed7 does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -0.9 | +10.9 | -1.9 | +11.5 | +9.9 | +0.0 | -2.8 | -6.8 | +10.6 | -11.7 | +9.0 |
| Relative (%) | -3.2 | +38.0 | -6.5 | +40.4 | +34.8 | +0.0 | -9.7 | -24.0 | +37.1 | -40.8 | +31.5 | |
| Steps (reduced) |
42 (42) |
67 (67) |
84 (84) |
98 (98) |
109 (109) |
118 (0) |
126 (8) |
133 (15) |
140 (22) |
145 (27) |
151 (33) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +13.2 | -0.9 | -6.2 | -3.7 | +5.5 | -7.8 | +12.8 | +9.7 | +10.9 | -12.6 | -3.9 | +8.1 |
| Relative (%) | +46.1 | -3.2 | -21.6 | -13.0 | +19.4 | -27.2 | +44.9 | +33.9 | +38.0 | -44.1 | -13.6 | +28.3 | |
| Steps (reduced) |
156 (38) |
160 (42) |
164 (46) |
168 (50) |
172 (54) |
175 (57) |
179 (61) |
182 (64) |
185 (67) |
187 (69) |
190 (72) |
193 (75) | |
- Step size: 28.534 ¢, octave size: 1198.4 ¢
Compressing the octave of 42edo by around 1.5 ¢ results in improved primes 3, 5, 7 and 13, but worse primes 2 and 11. This approximates all harmonics up to 16 within 13.9 ¢. Its 13-limit WE tuning and 13-limit TE tuning both do this.
Of the tunings discussed in this section, 13-limit WE and TE are the only ones to approximate all harmonics up to 10 within 10 cents, making them a good all-round choice.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -1.6 | +9.8 | -3.1 | +10.0 | +8.3 | -1.8 | -4.7 | -8.9 | +8.4 | -13.9 | +6.7 |
| Relative (%) | -5.5 | +34.4 | -11.0 | +35.1 | +28.9 | -6.4 | -16.5 | -31.1 | +29.6 | -48.7 | +23.4 | |
| Step | 42 | 67 | 84 | 98 | 109 | 118 | 126 | 133 | 140 | 145 | 151 | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +10.8 | -3.4 | -8.7 | -6.3 | +2.9 | -10.5 | +10.1 | +6.9 | +8.0 | +13.1 | -6.8 | +5.1 |
| Relative (%) | +37.8 | -11.9 | -30.5 | -22.0 | +10.1 | -36.7 | +35.3 | +24.1 | +28.1 | +45.8 | -23.9 | +17.9 | |
| Step | 156 | 160 | 164 | 168 | 172 | 175 | 179 | 182 | 185 | 188 | 190 | 193 | |
- Step size: Octave size: 1196.6 ¢
Compressing the octave of 42edo by around 3.5 ¢ results in improved primes 3, 5, 11 and 13, but worse primes 2 and 7. This approximates all harmonics up to 16 within 13.7 ¢. The tuning 151ed12 does this. So do the 7-limit WE and TE tunings of 42et, whose octaves are within 0.3 ¢ of 151ed12.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -3.4 | +6.9 | -6.9 | +5.7 | +3.4 | -7.0 | -10.3 | +13.7 | +2.3 | +8.2 | +0.0 |
| Relative (%) | -12.0 | +24.1 | -24.1 | +19.9 | +12.0 | -24.7 | -36.1 | +48.2 | +7.9 | +28.7 | +0.0 | |
| Steps (reduced) |
42 (42) |
67 (67) |
84 (84) |
98 (98) |
109 (109) |
118 (118) |
126 (126) |
134 (134) |
140 (140) |
146 (146) |
151 (0) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +3.9 | -10.5 | +12.5 | -13.7 | -4.7 | +10.3 | +2.2 | -1.2 | -0.2 | +4.8 | +13.3 | -3.4 |
| Relative (%) | +13.6 | -36.7 | +44.0 | -48.2 | -16.6 | +36.1 | +7.6 | -4.1 | -0.6 | +16.7 | +46.6 | -12.0 | |
| Steps (reduced) |
156 (5) |
160 (9) |
165 (14) |
168 (17) |
172 (21) |
176 (25) |
179 (28) |
182 (31) |
185 (34) |
188 (37) |
191 (40) |
193 (42) | |
- Octave size: 1195.2 ¢
Compressing the octave of 42edo by around 5 ¢ results in improved primes 3, 5, 11 and 13, but worse primes 2 and 7. This approximates all harmonics up to 16 within 14.2 ¢. The tuning 109ed6 does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -4.8 | +4.8 | -9.5 | +2.6 | +0.0 | -10.7 | +14.2 | +9.5 | -2.2 | +3.6 | -4.8 |
| Relative (%) | -16.7 | +16.7 | -33.4 | +9.1 | +0.0 | -37.8 | +49.9 | +33.4 | -7.6 | +12.6 | -16.7 | |
| Steps (reduced) |
42 (42) |
67 (67) |
84 (84) |
98 (98) |
109 (0) |
118 (9) |
127 (18) |
134 (25) |
140 (31) |
146 (37) |
151 (42) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -1.0 | +13.0 | +7.4 | +9.5 | -10.1 | +4.8 | -3.5 | -6.9 | -6.0 | -1.2 | +7.3 | -9.5 |
| Relative (%) | -3.6 | +45.5 | +25.8 | +33.2 | -35.6 | +16.7 | -12.2 | -24.3 | -21.1 | -4.1 | +25.5 | -33.4 | |
| Steps (reduced) |
156 (47) |
161 (52) |
165 (56) |
169 (60) |
172 (63) |
176 (67) |
179 (70) |
182 (73) |
185 (76) |
188 (79) |
191 (82) |
193 (84) | |
- Step size: 28.444 ¢, octave size: 1194.6 ¢
Compressing the octave of 42edo by around 5.5 ¢ results in improved primes 3, 5, 11 and 13, but worse primes 2 and 7. This approximates all harmonics up to 16 within 12.4 ¢. The tuning 191zpi does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -5.4 | +3.8 | -10.7 | +1.2 | -1.6 | -12.4 | +12.4 | +7.6 | -4.2 | +1.5 | -6.9 |
| Relative (%) | -18.8 | +13.3 | -37.6 | +4.2 | -5.5 | -43.7 | +43.6 | +26.7 | -14.6 | +5.3 | -24.3 | |
| Step | 42 | 67 | 84 | 98 | 109 | 118 | 127 | 134 | 140 | 146 | 151 | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -3.3 | +10.7 | +5.0 | +7.0 | -12.6 | +2.2 | -6.0 | -9.5 | -8.6 | -3.8 | +4.5 | -12.3 |
| Relative (%) | -11.5 | +37.5 | +17.5 | +24.7 | -44.3 | +7.9 | -21.2 | -33.4 | -30.4 | -13.5 | +15.9 | -43.1 | |
| Step | 156 | 161 | 165 | 169 | 172 | 176 | 179 | 182 | 185 | 188 | 191 | 193 | |
- Step size: 28.387 ¢, octave size: 1192.3 ¢
Compressing the octave of 42edo by around 7.5 ¢ results in improved primes 3, 5 and 11, but worse primes 2 and 7. This approximates all harmonics up to 16 within 12.9 ¢. The tuning 67edt does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -7.7 | +0.0 | +12.9 | -4.3 | -7.7 | +9.3 | +5.2 | +0.0 | -12.1 | -6.8 | +12.9 |
| Relative (%) | -27.2 | +0.0 | +45.5 | -15.3 | -27.2 | +32.7 | +18.3 | +0.0 | -42.6 | -23.8 | +45.5 | |
| Steps (reduced) |
42 (42) |
67 (0) |
85 (18) |
98 (31) |
109 (42) |
119 (52) |
127 (60) |
134 (0) |
140 (6) |
146 (12) |
152 (18) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -12.1 | +1.5 | -4.3 | -2.5 | +6.1 | -7.7 | +12.2 | +8.6 | +9.3 | +13.9 | -6.3 | +5.2 |
| Relative (%) | -42.6 | +5.4 | -15.3 | -8.9 | +21.4 | -27.2 | +43.0 | +30.2 | +32.7 | +49.0 | -22.1 | +18.3 | |
| Steps (reduced) |
156 (22) |
161 (27) |
165 (31) |
169 (35) |
173 (39) |
176 (42) |
180 (46) |
183 (49) |
186 (52) |
189 (55) |
191 (57) |
194 (60) | |
Scales
- Eugene/Tritikleismic[9]: 3 8 3 3 8 3 3 8 3
- Eugene/Tritikleismic[15]: 3 3 2 3 3 3 3 2 3 3 3 3 2 3 3
- Lemba[16]: 3 2 3 2 3 3 2 3 3 2 3 2 3 3 2 3
- Qeema/Skateboard[15]: 2 5 2 2 2 5 2 2 2 5 2 2 2 5 2
- Qeema/Skateboard[19]: 2 2 3 2 2 2 2 3 2 2 2 3 2 2 2 2 3 2 2
- Seville/Sevond[14] 1st mode: 1 5 1 5 1 5 1 5 1 5 1 5 1 5
- Seville/Sevond[14] 2nd mode: 5 1 5 1 5 1 5 1 5 1 5 1 5 1
- Seville/Sevond[21]: 1 4 1 1 4 1 1 4 1 1 4 1 1 4 1 1 4 1 1 4
- Subsets of MOS scales
(Names used are idiosyncratic.)
- Eugene/Tritikleismic[9]
- Groovy aeolian pentatonic: 11 6 8 3 14
- Otonal mixolydian pentatonic: 14 3 8 11 6
- Pseudo-equipentatonic: 11 6 8 6 11
- Septimal melodic minor pentatonic: 8 3 14 14 3
- Septimal Picardy pentatonic: 8 6 11 3 14
- Undecimal lydian-aeolian pentatonic: 8 14 3 11 6
- Yokai pentatonic: 3 14 8 3 14
- Approximations of gamelan scales
- 5-tone pelog: 4 5 15 3 15
- 7-tone pelog: 4 5 9 6 3 10 5
- 5-tone slendro: 8 9 8 9 8
Instruments
Lumatone
Skip fretting
Skip fretting system 42 3 11: One way to play 42edo on a 14edo guitar is to tune the strings 11\42, or approximately a just 6/5, apart. All examples on this page are for 7-string guitar.
- Prime intervals
1/1: string 2 open
2/1: string 5 fret 3
3/2: string 4 fret 1 and string 7 fret 4
5/4: string 3 fret 1
7/4: string 1 fret 1 and string 4 fret 4
11/8: string 7 fret 2
13/8: string 3 fret 6
17/16: string 1 fret 5
19/16: string 1 fret 7
23/16: string 4 open and string 7 fret 3
29/16: string 5 fret 1
31/16: string 1 fret 3 and string 4 fret 6
- Chords
Minor 7th: 100123X
Music
Modern renderings
- "Ricercar a 3" from The Musical Offering, BWV 1079 (1747) – rendered by Claudi Meneghin (2024)
- White Christmas - 42edo reimagining by Todd Harrop (2024)
21st century
- improv 42edo (2023)
- Improvisation in 42edo (2023), transcribed by Stephen Weigel (2024)
- Circulating and Traversing (2024) - see the composer’s notes
- Through the Dark (2024) - uses mostly Augene[15] with some chromaticism
- Glory of Them (2024)