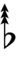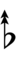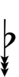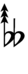84edo

From Xenharmonic Wiki
Jump to navigation Jump to search
← 83edo84edo85edo →
Prime factorization 22 × 3 × 7
Step size 14.2857¢ 
Fifth 49\84 (700¢) (→7\12)
Semitones (A1:m2) 7:7 (100¢ : 100¢)
Consistency limit 9
Distinct consistency limit 9

84 equal divisions of the octave (abbreviated 84edo or 84ed2), also called 84-tone equal temperament (84tet) or 84 equal temperament (84et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 84 equal parts of about 14.3 ¢ each. Each step represents a frequency ratio of 21/84, or the 84th root of 2.

Theory

84 = 7 × 12, and 84edo shares the perfect fifth with 12edo, tempering out the Pythagorean comma in its patent val. In the 5-limit it tempers out the sensipent comma; in the 7-limit 225/224, 1728/1715, 2430/2401, 6144/6125, supporting orwell, compton, and sensei.

84edo is where the orwell temperament takes its name from, since the generator of 7/6 is equal to 19 steps of the edo, referencing the book 1984. Orwell in 84edo comes in two varieties – the 84e val ⟨84 133 195 236 290], supporting the original orwell, and its patent val ⟨84 133 195 236 291] supporting newspeak. 84edo orwell offers mos scales of size 9, 13, 22, and 31, of which the 31-note scale is the maximal evenness scale.

It has fairly good approximation to higher prime harmonics such as 13, 19, 23, 29, and 31. In fact, it is consistent to the no-11 no-17 25-odd-limit. In the 13-limit it is the optimal patent val for the rank-5 temperament tempering out 144/143.

Prime harmonics

Approximation of prime harmonics in 84edo
Harmonic 2 3 5 7 11 13 17 19 23 29 31 37
Error Absolute (¢) +0.00 -1.96 -0.60 +2.60 +5.82 +2.33 -4.96 +2.49 +0.30 -1.01 -2.18 +5.80
Relative (%) +0.0 -13.7 -4.2 +18.2 +40.8 +16.3 -34.7 +17.4 +2.1 -7.0 -15.2 +40.6
Steps
(reduced) 		84
(0) 		133
(49) 		195
(27) 		236
(68) 		291
(39) 		311
(59) 		343
(7) 		357
(21) 		380
(44) 		408
(72) 		416
(80) 		438
(18)
Approximation of prime harmonics in 84edo (continued)
Harmonic 41 43 47 53 59 61 67 71 73 79 83 89
Error Absolute (¢) -0.49 +2.77 +5.92 -2.08 -2.03 -2.60 +6.41 +6.02 +0.78 +6.89 +7.10 +0.55
Relative (%) -3.4 +19.4 +41.5 -14.5 -14.2 -18.2 +44.9 +42.1 +5.5 +48.2 +49.7 +3.8
Steps
(reduced) 		450
(30) 		456
(36) 		467
(47) 		481
(61) 		494
(74) 		498
(78) 		510
(6) 		517
(13) 		520
(16) 		530
(26) 		536
(32) 		544
(40)

Subsets and supersets

84 is a largely composite number. Since 84 factors as 22 × 3 × 7, 84edo has subset edos 2, 3, 4, 6, 7, 12, 14, 21, 28, 42. Being a small multiple of 28, it tempers out the oquatonic comma, which maps 5/4 to 9\28.

Intervals

# Cents Approximate Ratios* Ups and Downs Notation
0 0.000 1/1 Perfect 1sn P1 D
1 14.286 81/80, 105/104, 126/125, 169/168, 196/195 Up 1sn ^1 ^D
2 28.571 50/49, 64/63, 65/64, 91/90 Dup 1sn ^^1 ^^D
3 42.857 36/35, 40/39, 46/45, 49/48 Trup 1sn ^^^1 ^^^D
4 57.143 27/26 Trudminor 2nd vvvm2 vvvEb
5 71.429 24/23, 25/24, 26/25, 28/27 Dudminor 2nd vvm2 vvEb
6 85.714 20/19, 21/20 Downminor 2nd vm2 vEb
7 100.000 19/18 Minor 2nd m2 Eb
8 114.286 15/14, 16/15 Upminor 2nd ^m2 ^Eb
9 128.571 14/13 Dupminor 2nd ^^m2 ^^Eb
10 142.857 13/12 Trupminor 2nd ^^^m2 ^^^Eb
11 157.143 23/21 Trudmajor 2nd vvvM2 vvvE
12 171.429 21/19 Dudmajor 2nd vvM2 vvE
13 185.714 10/9 Downmajor 2nd vM2 vE
14 200.000 9/8 Major 2nd M2 E
15 214.286 26/23 Upmajor 2nd ^M2 ^E
16 228.571 8/7 Dupmajor 2nd ^^M2 ^^E
17 242.857 15/13, 23/20 Trupmajor 2nd ^^^M2 ^^^E
18 257.143 52/45 Trudminor 3rd vvvm3 vvvF
19 271.429 7/6 Dudminor 3rd vvm2 vvF
20 285.714 45/38, 46/39 Downminor 3rd vm3 vF
21 300.000 19/16, 25/21, 32/27 Minor 3rd m3 F
22 314.286 6/5 Upminor 3rd ^m3 ^F
23 328.571 23/19 Dupminor 3rd ^^m3 ^^F
24 342.857 28/23, 39/32 Trupminor 3rd ^^^m3 ^^^F
25 357.143 16/13 Trudmajor 3rd vvvM3 vvvF#
26 371.429 26/21 Dudmajor 3rd vvM3 vvF#
27 385.714 5/4 Downmajor 3rd vM3 vF#
28 400.000 24/19 Major 3rd M3 F#
29 414.286 19/15 Upmajor 3rd ^M3 ^F#
30 428.571 9/7, 23/18, 32/25 Dupmajor 3rd ^^M3 ^^F#
31 442.857 84/65 Trupmajor 3rd ^^^M3 ^^^F#
32 457.143 13/10, 30/23 Trud 4th vvv4 vvvG
33 471.429 21/16 Dud 4th vv4 vvG
34 485.714 65/49 Down 4th v4 vG
35 500.000 4/3 Perfect 4th P4 G
36 514.286 27/20 Up 4th ^4 ^G
37 528.571 19/14 Dup 4th ^^4 ^^G
38 542.857 26/19 Trup 4th ^^^4 ^^^G
39 557.143 18/13 Trudaug 4th vvvA4 vvvG#
40 571.429 25/18, 32/23 Dudaug 4th vvA4 vvG#
41 585.714 7/5 Downaug 4th vA4 vG#
42 600.000 27/19, 38/27 Aug 4th, Dim 5th A4, d5 G#, Ab
43 614.286 10/7 Updim 5th ^d5 ^Ab
44 628.571 23/16, 36/25 Dupdim 5th ^^d5 ^^Ab
45 642.857 13/9 Trupdim 5th ^^^d5 ^^^Ab
46 657.143 19/13 Trud 5th vvv5 vvvA
47 671.429 28/19 Dud 5th vv5 vvA
48 685.714 40/27 Down 5th v5 vA
49 700.000 3/2 Perfect 5th P5 A
50 714.286 98/65 Up 5th ^5 ^A
51 728.571 32/21 Dup 5th ^^5 ^^A
52 742.857 20/13, 23/15 Trup 5th ^^^5 ^^^A
53 757.143 65/42 Trudminor 6th vvvm6 vvvBb
54 771.429 14/9, 25/16, 36/23 Dudminor 6th vvm6 vvBb
55 785.714 30/19 Downminor 6th vm6 vBb
56 800.000 19/12 Minor 6th m6 Bb
57 814.286 8/5 Upminor 6th ^m6 ^Bb
58 828.571 21/13 Dupminor 6th ^^m6 ^^Bb
59 842.857 13/8 Trupminor 6th ^^^m6 ^^^Bb
60 857.143 23/14, 64/39 Trudmajor 6th vvvM6 vvvB
61 871.429 38/23 Dudmajor 6th vvM6 vvB
62 885.714 5/3 Downmajor 6th vM6 vB
63 900.000 32/19, 27/16, 42/25 Major 6th M6 B
64 914.286 39/23, 76/45 Upmajor 6th ^M6 ^B
65 928.571 12/7 Dupmajor 6th ^^M6 ^^B
66 942.857 45/26 Trupmajor 6th ^^^M6 ^^^B
67 957.143 26/15, 40/23 Trudminor 7th vvvm7 vvvC
68 971.429 7/4 Dudminor 7th vvm7 vvC
69 985.714 23/13 Downminor 7th vm7 vC
70 1000.000 16/9 Minor 7th m7 C
71 1014.286 9/5 Upminor 7th ^m7 ^C
72 1028.571 38/21 Dupminor 7th ^^m7 ^^C
73 1042.857 42/23 Trupminor 7th ^^^m7 ^^^C
74 1057.143 24/13 Trudmajor 7th vvvM7 vvvC#
75 1071.429 13/7 Dudmajor 7th vvM7 vvC#
76 1085.714 15/8, 28/15 Downmajor 7th vM7 vC#
77 1100.000 36/19 Major 7th M7 C#
78 1114.286 19/10, 40/21 Upmajor 7th ^M7 ^C#
79 1128.571 23/12, 25/13, 27/14, 48/25 Dupmajor 7th ^^M7 ^^C#
80 1142.857 52/27 Trupmajor 7th ^^^M7 ^^^C#
81 1157.143 35/18, 39/20, 96/49 Trud 8ve vvv8 vvvD
82 1171.429 45/23, 49/25, 63/32, 128/65, 180/91 Dud 8ve vv8 vvD
83 1185.714 125/63, 160/81, 195/98, 336/169 Down 8ve v8 vD
84 1200.000 2/1 Perfect 8ve P8 D

* as a 2.3.5.7.13.19.23-subgroup temperament

Notation

4L 5s (gramitonic) notation

The notation of Orwell[9]. Notes are denoted as LsLsLsLss = JKLMNOPQRJ, and raising and lowering by a chroma (L − s), 3 steps in this instance, is denoted by & "amp" and @ "at".

# Cents Note Name Associated Ratio
0 0.0 J Perfect 0-gramstep 1/1
8 114.3 K@ Minor 1-gramstep 15/14~16/15
11 157.1 K Major 1-gramstep 11/10~12/11
16 228.6 L@ Diminished 2-gramstep 8/7
19 271.4 L Perfect 2-gramstep 7/6
27 385.7 M@ Minor 3-gramstep 5/4
30 428.6 M Major 3-gramstep 9/7
35 500.0 N@ Minor 4-gramstep 4/3
38 542.9 N Major 4-gramstep 11/8~15/11
46 657.1 O@ Minor 5-gramstep 16/11~22/15
49 700.0 O Major 5-gramstep 3/2
54 771.4 P@ Minor 6-gramstep 14/9
57 814.3 P Major 6-gramstep 8/5
65 928.6 Q@ Perfect 7-gramstep 12/7
68 971.4 Q Augmented 7-gramstep 7/4
73 1042.9 R@ Minor 8-gramstep 11/6~20/11
76 1085.7 R Major 8-gramstep 15/8~28/15
84 1200.0 J Perfect 9-gramstep 2/1

Regular temperament properties

Subgroup Comma List Mapping Optimal
8ve Stretch (¢) 		Tuning Error
Absolute (¢) Relative (%)
2.3.5 78732/78125, 531441/524288 [⟨84 133 195]] +0.498 0.531 3.72
2.3.5.7 225/224, 1728/1715, 78732/78125 [⟨84 133 195 236]] +0.141 0.769 5.39
2.3.5.7.13 225/224, 351/350, 640/637, 1701/1690 [⟨84 133 195 236 311]] -0.013 0.754 5.28
2.3.5.7.11 225/224, 441/440, 1344/1331, 1728/1715 [⟨84 133 195 236 291]] (84) -0.225 1.003 7.02
2.3.5.7.11.13 144/143, 225/224, 351/350, 441/440, 975/968 [⟨84 133 195 236 291 311]] (84) -0.292 0.928 6.50
2.3.5.7.11 99/98, 121/120, 176/175, 78732/78125 [⟨84 133 195 236 290]] (84e) +0.601 1.151 8.05
2.3.5.7.11.13 99/98, 121/120, 176/175, 275/273, 1701/1690 [⟨84 133 195 236 290 311]] (84e) +0.396 1.146 8.02

Rank-2 temperaments

Periods
per 8ve 		Generator* Cents* Associated
Ratio* 		Temperaments
1 19\84 271.43 7/6 Orwell (84e) / newspeak (84)
1 25\84 357.14 768/625 Dodifo
1 27\84 385.71 5/4 Mutt
1 31\84 442.86 125/81 Sensei
1 41\84 585.71 7/5 Merman
2 5\84 71.43 25/24 Narayana
2 11\84 157.14 35/32 Bison
2 13\84 185.71 10/9 Secant
3 11\84 157.14 35/32 Nessafof
7 5\84 500.00
(14.29) 		4/3
(81/80) 		Absurdity
12 27\84
(1\84) 		385.71
(14.29) 		5/4
(126/125) 		Compton
21 41\84
(1\84) 		585.71
(14.29) 		91875/65536
(126/125) 		Akjayland
28 49\84
(1\84) 		500.00
(14.29) 		4/3
(105/104) 		Oquatonic

* octave-reduced form, reduced to the first half-octave, and minimal form in parentheses if it is distinct

Scales

MOS

Brightest mode is listed.

Other

Music

John Cage
Eliora
JUMBLE
Retrieved from "https://en.xen.wiki/index.php?title=84edo&oldid=146593"