Parapyth
Parapyth, also known as parapythagorean, is the rank-3 temperament tempering out 352/351 and 364/363 in the 2.3.7.11.13 subgroup.
Inspired by George Secor's 29-tone high tolerance temperament, parapyth was found by Margo Schulter in 2002, and it continued to be developed as part of her neoclassical tuning theory (NTT), although a regular temperament perspective is as viable.
In the early prototype, there was only a single chain of fifths, tuned slightly sharp such that:
- The minor third (−3 fifths) is 13/11, tempering out 352/351;
- The major third (+4 fifths) hits 14/11, tempering out 896/891;
- The augmented unison (+7 fifths) hits 14/13, tempering out 28672/28431.
This temperament is now known as pepperoni. Parapyth encapsulates pepperoni and adds a 28/27 ~ 33/32 spacer interval such that harmonics 7, 11, and 13 are all made available simply by using two chains of fifths.
See Pentacircle clan#Parapyth for technical data.
Interval lattice

|
| In CTE tuning and lattice basis {~2, ~3, ~7/4} |
|
| In MET-24 tuning and lattice basis {~2, ~3, ~33/32} |
These diagrams differ by lattice bases and tunings. The first diagram is generated by {~2, ~3, ~7/4}, corresponding to the octave-reduced form of the mapping, and tuned to the 2.3.7.11.13 subgroup CTE tuning. The second diagram shows the preferred settings in Margo Schulter's neoclassical tuning theory, where it is generated by {~2, ~3, ~33/32}, and tuned to MET-24.
Scales
- Parapyth12 – 12-tone Fokker block in 2.3.7.11.13 TOP tuning
- Pepperoni7 – 7-tone single chain of fifths in 271edo tuning
- Pepperoni12 – 12-tone single chain of fifths in 271edo tuning
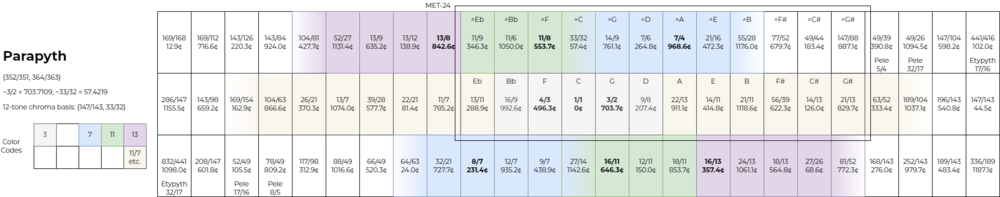
- MET-24 – 24-tone double chain of fifths in 2048edo tuning
Tunings
The most important tuning for parapyth is that given by MET-24 (milder extended temperament):
- ~2/1 = 1200.000 ¢, ~3/2 = 703.711 ¢, ~33/32 = 57.422 ¢.
Another tuning derives from a 24-tone subset of George Secor's 29-HTT, thus a "24-HTT":
- ~2/1 = 1200.000 ¢, ~3/2 = 703.579 ¢, ~33/32 = 58.090 ¢.
The fifth is in the 9th-secorian-comma tuning, which makes the augmented second of 63/52 pure. This fifth leads to an equal 3.247-cent error in 9/8 and 14/13 (63/52 = (9/8)⋅(14/13)) and thus a possible minimax tuning for the no-5 13-odd-limit. The minor third is extremely close to just 13/11, only off by 1/3 harmonisma. The spacer is determined such that 7/4 is pure.
Yet another possible tuning is that given by Peppermint-24:
- ~2/1 = 1200.000 ¢, ~3/2 = 704.096 ¢, ~33/32 = 58.680 ¢.
The fifth leads to step ratio φ for the chromatic scale and the spacer tunes the 7/6 pure.
Edo tunings
The parapyth edos below 311 that are not contorted in 2.3.7.11.13 are 17, 22, 24, 29, 41, 46, 58, 63, 65, 80, 87, 104, 109, 121, 128, 133, 145, 150, 167, 172, 184, 191, 196, 213, 230, 232, 237, 254, 259, 271, 278, 283, and 295.
87edo is special for being the smallest "strict parapyth edo" (tempers out 352/351 and 364/363 and maps all of 121/120, 144/143, and 169/168 positively, meeting Margo Schulter's criterion for "middle parapyth in the strict sense"). The following are strict parapyth edos below 311 that are not contorted in the 13-limit: 87, 104, 121, 128, 133, 145, 150, 167, 184, 191, 196, 208, 213, 230, 232, 237, 254, 259, 271, 278, 283, 295. (Note: 208edo is contorted in 2.3.7.11.13 subgroup but not in the full 13-limit.)
If we instead mean "parapyth" to refer to etypyth – its most elegant extension to the no-5's 17-limit (so we ignore S10 and S11) – then the minimal strict etypyth (a.k.a. 17-limit parapyth) is 46edo, although this requires accepting its 21/17 as standing in for ~16/13 and ~26/21, corresponding roughly to (the octave complement of) acoustic phi so that stacking this interval gives a ~17:21:26:32 chord. The benefit of taking this no-5's interpretation is you do not deal with any conceptual issues arising from an out-of-tune 15/13 in 46edo, but you could deal with this alternately by interpreting simply only in the 13-odd-limit adding odds 17, 21 and 23, which highlights that a benefit of 46edo is a fairly accurate 23/16 in the usual parapyth mapping of a tritone (C–F♯), tempering out (23/16)/(9/8)3 = 736/729. Alternatively, if you want a more accurate 9/7, 7/6, 13/11, 104edo is an excellent etypyth tuning. 104edo is a dual-5 system that supports both the sensamagic (104) and pele (104c) mappings of 5, so that the combined 25/16 is very accurate (tempered together with the 81/52 (C–vG♯), distinguished from 11/7 (C–A♭) and 14/9 (C–^G) simultaneously). Pele may be preferable as a default due to it observing S10 and S11. Sensamagic has the capacity to observe them too, but in the specific case of 104edo it tempers out S10.
See also
- Leapday – a rank-2 reduction of parapyth with additional extensions for approximating harmonics 17 and 23