User talk:Aura
Getting Started
Okay, so I'm new here, and I'm new to editing wikis in general, so I need to learn some of the ropes around here. Also, I do have some ideas for interval names and notations, building off of both the SHEFKHED interval naming system and ups and downs notation, and I would like to discuss this. I mean, seeing as I've taken a shine to 159edo, I need a better way of notating this kind of stuff in my music- something that still manages to be diatonic-based... --Aura (talk) 19:41, 31 August 2020 (UTC)
- Howdy here! If you want help with editing the wiki, there's a Conventions page on the left side of the wiki that you can refer to, though as a relatively new editor myself, its pretty easy to get the hang of things if you just look at what other people do while editing. On the topic of notation, I'd be happy to talk about it (and perhaps about 159EDO as well!). --CritDeathX (talk) 02:26, 1 September 2020 (UTC)
- I'm glad to see that someone has noticed what I'm doing! For the record, I do have distinct names for intervals like 11/8 and 16/11. I call the 11/8 interval the "paramajor fourth" and the 16/11 interval the "paraminor fifth" in part as a reference to this [1]. Similarly, I call 128/99 the "paraminor fourth" and 99/64 the "paramajor fifth". For the record, I do use "parasuper" and "parasub" as prefixes not only for the alteration of perfect primes and perfect octaves by 33/32, but also for the augmentation of major intervals and the dimunition of minor intervals by 33/32. Because the dimunition of a major interval by 33/32 does not result in the same interval as does the augmentation of a minor interval by 33/32, especially in those equal divisions of the octave where 243/242 is not tempered out, I use the term "greater neutral" to refer to dimunition of a major interval by 33/32, and the term "lesser neutral" to refer to the augmentation of a minor interval by 33/32. Do note that I use the Pythagorian chain of fifths as a base. --Aura (talk) 02:51, 1 September 2020 (UTC)
- Okay, I like the sound of this so far. I assume you use super/sub and major/minor for 7- & 5-limit intervals respectively, yes? --CritDeathX (talk) 03:32, 1 September 2020 (UTC)
- Yes, I do. However, this raises the question of what to do for intervals like 256/225, which naturally occurs between the seventh and second scale degrees in the just versions of the Greater Neapolitan and Lesser Neapolitan scales- otherwise known as the Neapolitan Major and Neapolitan Minor scales respectively. --Aura (talk) 03:44, 1 September 2020 (UTC)
- Okay... I have an idea... So, I'm looking at this page [[2]], as well as this page [[3]], and I notice that there's more than one "minor third" and more than one "major third". The same is true of intervals such as supermajor thirds and subminor thirds- particularly for equal divisions of the octave where the septimal kleisma is not tempered out, such as in 159edo. With that in mind, I'm thinking we should disambiguate between different intervals in the same general range. We can build directly off of the SHEFKHED interval naming system for the basics, though with the difference that any Pythagorean interval other than the Perfect Prime, the Perfect Octave, the Perfect Fifth and the Perfect Fourth with an odd limit of 243 or less should gain the explicit label of "Diatonic"- this lends itself to names such as "Diatonic Major Sixth" for 27/16. Following along this same line of thinking for 5-limit intervals, we can similarly build off of the SHEFKHED interval naming system and explicitly label both 5/4 and 8/5, as well as intervals connected to them by a chain of Perfect Fifths "Diatonic"- assuming the odd limit for said interval is 45 or less. Among the end results of this are that 5/3 is labeled the "Classic Diatonic Major Sixth". I'm currently thinking that certain other 5-limit intervals should also gain the label "Classic" such as 25/16 or even 25/24... --Aura (talk) 06:58, 1 September 2020 (UTC)
- Hello and thx for contributing your ideas! This topic is of my interest and I actually opened a conversation on our FB group on how we may call most 5-limit intervals. To summarize, some would use "pental" for 5-limit intervals, some others would default to simplest ratios in the group and add definitives when needed, but the solution most convincing to me is to call any Pythagorean intervals "Pythagorean" and any 5-limit intervals "classic" (sometimes "grave/acute" for high-odd-limit intervals), though to distinguish 25/24 from 135/128 this needs further disambiguation. I'd also refrain from a meantone-centrist view, where "aug" and "dim" are sometimes abused e.g. "aug sixth" for 7/4, which is only true in meantone. FloraC (talk) 08:24, 1 September 2020 (UTC)
- For the record, I'm doing this with 159edo in mind, and this is not a meantone temperament as the syntonic comma is not tempered out. I'm not keen on using too many numeric descriptors like "pental" or "septimal" or even "undecimal" for this particular idea, as at the end of the day, my goal is to build off of the SHEFKHED interval naming system for EDOs up to 160edo. I should also point out that not all Pythagorean intervals are Diatonic intervals- only those with an odd limit of 243 or less, therefore, I'm thinking that "Diatonic" is the label that ought to be privileged over "Pythagorean". On a semi-related note, my preferred major scale consists of the intervals 1/1, 9/8, 5/4, 4/3, 3/2, 27/16, 15/8, and 2/1, and I do in fact build directly off of this scale for my diatonic chords- yes, the grave fifth occurs between the sixth and the third, and for me, this serves to amplify the diatonic functions of the VIm chord, as this kind of tuning says "we're not done yet", especially in deceptive cadences. --Aura (talk) 15:39, 1 September 2020 (UTC)
- While I'm on this whole topic of Diatonic intervals, I should mention that I prefer the notes of all my scales to connect directly to the tonic by means of the intervals between the tonic and the other notes in the scale having a power of two in the numerator and or the denominator- that said, I still recognize that 6/5 doesn't meet this criteria when this interval occurs between the I and the IIIm scale degrees, and thus, my preferred minor scale consists of the intervals 1/1, 9/8, 77/64, 4/3, 3/2, 8/5, 16/9, and 2/1. It is for this reason- along with the fact that the 7-limit finds frequent use among barbershop quartets and the like as accidentals in otherwise diatonic keys- that I would classify 11/8, 16/11, 7/4, and 8/7 as "Paradiatonic" intervals. --Aura (talk) 16:09, 1 September 2020 (UTC)
- Now, back to this discussion of notation and interval naming... Some will undoubtedly ask where this process of coming up with labels for scale steps of differing edos should stop, and I have an answer for that as well. There is a step-size limit at play in which the step size should be greater than 7 cents. This is because at a step size of 7 cents, the distance halfway between steps is 3.5 cents, which, from what I'm gathering, is below the average just noticeable difference between pitches. At step sizes of 7 cents and smaller, the steps will begin to bleed into one another and become indistinguishable from one another to even the best trained ears. Thus, any edo with a step size of 7 cents or less is ineligible for this kind of extensive process of labeling different step sizes. --Aura (talk) 16:51, 1 September 2020 (UTC)
- I can anticipate that some may object that I should draw the line for defining edo steps at something more substantial like 13.5 cents, but I while concur that an edo step size between 7.5 and 13.5 cents is not viable in the traditional musical sense as a step between consecutive notes, I do notice that it does have a usage as a comma pump, and therefore, it has musical value as an edo step size for purposes of modulation, especially for modulating Jacob-Collier-style between keys. --Aura (talk) 17:49, 1 September 2020 (UTC)
- One of the problems I have with notation that doesn't take these kinds of kleismatic differences into account is that without such distinctions, it's hard to determine which notes should have which tunings in order to accomplish a seamless modulation between keys on different circles of fifths, and I've honestly found that to be a significant problem with transcriptions of Jacob Collier's rendition of In the Bleak Midwinter in particular. --Aura (talk) 18:33, 1 September 2020 (UTC)
- Wow that was a long read! I'd just like to remind, with 159edo you can think of pitch categories and you don't have to pick fixed pitches for each category, unlike 12edo where you don't have much choice. For example, the 3rd note can vary from 5/4 to 9/7 in different occasions. AFAIK Jacob does his stuff mostly in terms of JI, so I'd think this way too in this case. As for where to draw the edo stopping line, my answer would be 6 cents for human audience (cuz there's an edo around that size that's become my latest favorite :)). FloraC (talk) 06:02, 2 September 2020 (UTC)
- Sorry about that! I had a lot to say, and I still have a lot to say. For the record, I define a pitch class as consisting of a given pitch plus all multiples and divisions of that pitch by powers of two, so in 159edo, that's 159 different pitch classes available to work with. Also, I'd greatly prefer to keep the number of pitch classes limited for sections of my songs that remain in a particular key so that I don't have to do as much work in tuning them, not to mention that I've grown to like the idea of chords with different diatonic functions having different tunings- something that inevitably results from limiting your pitch class selection, for better or for worse. It is true that I'll change things up for purposes of modulation and when otherwise using accidentals, but nevertheless, while working in any one particular key, I'll generally keep using the same set of fixed pitches. --Aura (talk) 10:08, 2 September 2020 (UTC)
- Just to throw this out there, I classify prime-limits based on their function relative to the tonic in tonal music. The 2-limit is the "Pitch Class Prime"- tying into my aforementioned definition of "Pitch Class". The 3-limit and 5-limit are classified as the "Diatonic Primes" because of their key functions in diatonic and just chromatic music. The 7-limit, 11-limit and 13-limit are classified as "Paradiatonic Primes" due to their relative ease (and, in the case of the 7-limit, frequency) of use as accidentals in otherwise diatonic keys, and, due to the fact that these relatively low primes can create intervals that can be readily used as substitutions for diatonic intervals- again tying into comments I made before about the interval 77/64 in particular. The 17-limit and 19-limit are classified as "Quasidiatonic Primes" owing to the most basic intervals in these families having striking similarities to diatonic intervals, but with greater complexity. Finally, the 23-limit, 29-limit, and 31-limit are classified as "Quasiparadiatonic Primes", a mouthful of a name that I've given them on account of these primes either having striking similarities to paradiatonic intervals, or being able to create intervals that can find use as substitutions for paradiatonic intervals, albeit with greater complexity. I also have a distinct classification for primes between 37 and 1021, and another for primes beyond 1021, but the names of these classes ties into a whole different topic. --Aura (talk) 18:52, 2 September 2020 (UTC)
- Okay, so in accordance with Sam's suggestion, I'm renaming the 23-limit, 29-limit, and 31-limit the "Pseudodiatonic Primes", since these primes are not diatonic by any stretch, yet they can still serve as substitutes for the paradiatonic primes in a pinch. Any further thoughts? --Aura (talk) 22:26, 3 September 2020 (UTC)
- Wow that was a long read! I'd just like to remind, with 159edo you can think of pitch categories and you don't have to pick fixed pitches for each category, unlike 12edo where you don't have much choice. For example, the 3rd note can vary from 5/4 to 9/7 in different occasions. AFAIK Jacob does his stuff mostly in terms of JI, so I'd think this way too in this case. As for where to draw the edo stopping line, my answer would be 6 cents for human audience (cuz there's an edo around that size that's become my latest favorite :)). FloraC (talk) 06:02, 2 September 2020 (UTC)
- Hello and thx for contributing your ideas! This topic is of my interest and I actually opened a conversation on our FB group on how we may call most 5-limit intervals. To summarize, some would use "pental" for 5-limit intervals, some others would default to simplest ratios in the group and add definitives when needed, but the solution most convincing to me is to call any Pythagorean intervals "Pythagorean" and any 5-limit intervals "classic" (sometimes "grave/acute" for high-odd-limit intervals), though to distinguish 25/24 from 135/128 this needs further disambiguation. I'd also refrain from a meantone-centrist view, where "aug" and "dim" are sometimes abused e.g. "aug sixth" for 7/4, which is only true in meantone. FloraC (talk) 08:24, 1 September 2020 (UTC)
- Okay, I like the sound of this so far. I assume you use super/sub and major/minor for 7- & 5-limit intervals respectively, yes? --CritDeathX (talk) 03:32, 1 September 2020 (UTC)
- I'm glad to see that someone has noticed what I'm doing! For the record, I do have distinct names for intervals like 11/8 and 16/11. I call the 11/8 interval the "paramajor fourth" and the 16/11 interval the "paraminor fifth" in part as a reference to this [1]. Similarly, I call 128/99 the "paraminor fourth" and 99/64 the "paramajor fifth". For the record, I do use "parasuper" and "parasub" as prefixes not only for the alteration of perfect primes and perfect octaves by 33/32, but also for the augmentation of major intervals and the dimunition of minor intervals by 33/32. Because the dimunition of a major interval by 33/32 does not result in the same interval as does the augmentation of a minor interval by 33/32, especially in those equal divisions of the octave where 243/242 is not tempered out, I use the term "greater neutral" to refer to dimunition of a major interval by 33/32, and the term "lesser neutral" to refer to the augmentation of a minor interval by 33/32. Do note that I use the Pythagorian chain of fifths as a base. --Aura (talk) 02:51, 1 September 2020 (UTC)
links again
You should try [[File:Anticipation.mp3]] in the wiki text which appears as:
- BTW: interesting piece!
Best regards --Xenwolf (talk) 16:06, 4 September 2020 (UTC)
Quartismic Temperament
Howdy there, Aura! I decided to try and leave this for you to check out while you have the idea of a quartismic temperament in mind.
11-limit Mapping:
1 1 2 2 2
0 13 7 18 33
-9?
The temperament finder gives me different (very complex) mappings, so I'm not going to worry about that. Also something interesting to note is that there's two types of fifths, as shown in the scale above. Anyways, have fun! --CritDeathX (talk) 13:44, 8 September 2020 (UTC)
Oh, I just noticed that you had a page for quartismic temperaments. Hopefully this temperament's interesting enough to list on there! --CritDeathX (talk) 14:01, 8 September 2020 (UTC)
I found another temperament! 2.3.5.9.19 Mapping:
1 0 1 2 2
0 -16 15 2 14
Scale Tree Diagram: http://www.microtonalsoftware.com/scale-tree.html?left=12&right=11&rr=1200&ioi=106.71461627796054
I should mention there is an 11 in this, but its way high up. Have fun with this too! --CritDeathX (talk) 15:08, 8 September 2020 (UTC)
- Okay, I have to admit that I don't quite know what I'm looking at for any of the links you've sent, or even how to read the mappings that you've posted. It is true that I can play the scales on the keyboard, however, a number of the other facets of what you've shown are flying over my head at the moment- not that I don't want to actually get this data linked to the page on quartismic temperaments, as I'm absolutely sure this data is valuable. --Aura (talk) 15:19, 8 September 2020 (UTC)
- The mappings show how many generators & octaves take to get to a certain interval. For example, in the first temperament I found, our 5/4 can be found after going 7 generators up, and then to get to 5/1, we go two octaves up. The scale tree diagram shows related generators based on certain EDOs. In case you're confused more, the generators I've used are in the titles of the scales in Scale Workshop. --CritDeathX (talk) 15:38, 8 September 2020 (UTC)
Preview feature if the wiki
Hi Aura,
I just want to draw your attention to the fact that there is a preview function when editing pages. Using it regularly will save you from having to make some small changes unnecessarily. It's the [Show preview] button right to the [Save changes] button below the edit memo.
Best regards --Xenwolf (talk) 05:49, 9 September 2020 (UTC)
- Thanks. Unfortunately, I seem to be quite prone to missing words or punctuation marks only to see the need for corrections later... --Aura (talk) 05:50, 9 September 2020 (UTC)
- I should also point out that if you go through the records of my edits, you'll find I'm prone to second-guessing myself in terms of how I try to express stuff... --Aura (talk) 06:14, 9 September 2020 (UTC)
- Never mind. I know exactly what you're talking about. 🙂
- I also see (sorry only now) that you are using the visual editor, so the preview is already incorporated in editing. I personally like the wikitext editor which separates editing and preview (like here on talk pages). Lots of minor updates make it harder to keep track even of a work in progress (especially for discussions, where others may be already starting to answer) or take longer time for organizers of this wiki to patrol the recent changes page (for example as to detect vandalism attempts).
- Another thought: since this is a wiki it's not necessary to immediately fix every tiny error you make all the time. Improving your edits could as well be a good starting point for your fellow users here in the wiki. 🙂
- Best regards --Xenwolf (talk) 11:57, 9 September 2020 (UTC)
Great idea
I'm very interested in learning about your Ideas of Consonance. Maybe this can help me figure out my own ideas about Consonance/Dissonance. --Xenwolf (talk) 19:36, 11 September 2020 (UTC)
undecimal subminor second, undecimal supermajor seventh
May I invite you to discuss the topic(s) under Talk:33/32#undecimal subminor second? --13:09, 18 September 2020 (UTC)
Feature request to Diatonic Function Map
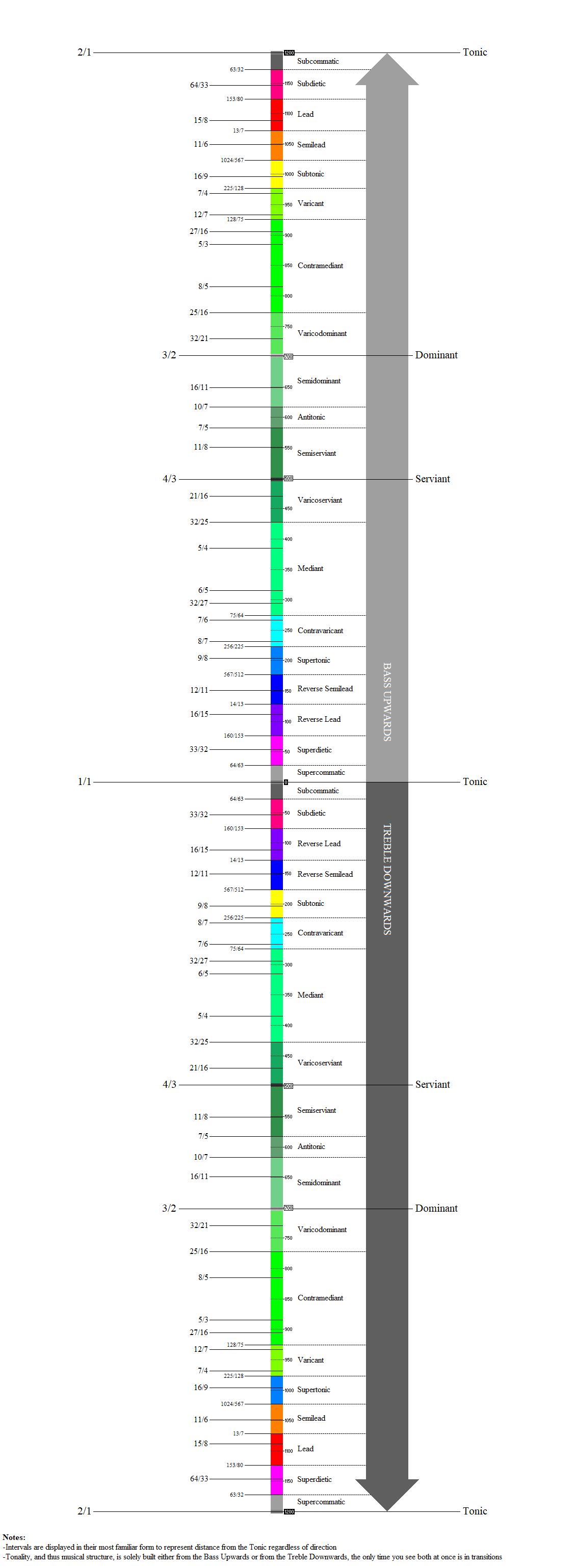
I think an important improvement to File:New Diatonic Function Map.png would be to add a cents scale somewhere. I just tried to do it myself but it turned out as too hard. I hope it's not a big issue to you... --Xenwolf (talk) 22:02, 21 September 2020 (UTC)
- Would be great, but I'm not sure how to do that... I mean, it's already a little less than one cent per pixel as is in terms of its overall size- it wouldn't be feasible to add markers for all 1200 cents... --Aura (talk) 02:12, 22 September 2020 (UTC)
- I think 50-cent steps would absolutely suffice, maybe even 100-cent steps. It's for the reason that you don't have to look up intervals or calculate from ratio. And furthermore you can see at a glance that it is a logarithmic diagram. This scale could as well have bigger and smaller ticks and maybe only some numbers like 0, 300, 600, 900, 1200 (if numbers at all). I'm currently not able to decide what would look best... --Xenwolf (talk) 05:00, 22 September 2020 (UTC)
- Alright, here's the latest version with the cents markers added...
- I think it's more useful now. I still have to figure out what all it may tell me...
- I'm also surprised how large the 50-cent steps appear. Sadly we have no image map feature active in this wiki. I also plan to add a graph for the interval categories. --Xenwolf (talk) 17:23, 22 September 2020 (UTC)
- I reckon you might want to make pages for all of the listed intervals on the chart if they don't exist already, and gather their sizes in cents. Once that's done, you can go through the Gallery of Just Intervals page manually and catalog the interval categories you see as well as calculate where they fall on this chart. Don't forget to also check the SHEFKHED interval names page, as it could also be of help for classifying intervals that are not listed on the chart. --Aura (talk) 17:46, 22 September 2020 (UTC)
- Thanks for this enlightening reading (the SHEFKHED page). Now the meaning of these "confusing" labels
P, M, m, A, dgets so obvious! It's really hard to see it if you are not an English native speaker (and even harder to recognize what you don't see); in German for example they would readR, G, k, Ü, v(R: rein, G: groß, k: klein, Ü: übermäßig, v: vermindert). --Xenwolf (talk) 18:11, 22 September 2020 (UTC)
- Thanks for this enlightening reading (the SHEFKHED page). Now the meaning of these "confusing" labels
- Just to make things easier, I can tell you that aside from this chart and the resources I've created, everything else here on this Wiki assumes Bass-Up tonality, so you should only focus on where intervals fall relative to the upper half of the chart for now. My predictions for the results of going through the aforementioned process is that in the Superdietic region, you should find lots of primes and seconds interspersed with one another whereas this is less common in the adjacent regions, likewise, the Subdietic region should host a similar interspersing of sevenths and octaves. In the Contravaricant region, you should expect to find seconds and thirds interspersed with one another, with a corresponding interspersing of sixth and sevenths in the Varicant region. In the Varicoserviant region, you should expect to find thirds and fourths interspersed with one another, with a corresponding interspersing of fifths and sixths in the Varicodominant region. Finally in the Antitonic region, there's a mixture of fourths and fifths. Aside from these specific regions, most of the other intervals are pretty straightforward in terms of what to expect- for example, primes in the Supercommatic region, seconds in the Reverse Lead, Reverse Semilead and Supertonic regions, thirds in the Mediant region, fourths in the Serviant and Semiserviant regions, Fifths in the Semidominant and Dominant regions, Sixths in the Contramediant region, Sevenths in the Subtonic, Semilead, and Lead regions, and octaves in the Subcommatic region. While I do expect there to be outliers, they should be relatively few in number. --Aura (talk) 18:03, 22 September 2020 (UTC)
tri vs. tre
The English one, two, three is uno, due, tre in Italian. Because classic music language is mostly (derived from) Italian, both is right. --Xenwolf (talk) 12:45, 22 September 2020 (UTC)
- Ah... Still, I assume "tridecimal" is the form we ought to use- for consistency's sake. --Aura (talk) 14:26, 22 September 2020 (UTC)
programming language of choice
What programming language do you usually use (if you use one at all)? I ask because I plan to enrich the cent page with some snippets. --Xenwolf (talk) 09:50, 19 October 2020 (UTC)
- Truth be told I don't really know how to program, so I guess the answer is "none of them"... --Aura (talk) 12:14, 19 October 2020 (UTC)
Harmony in Folly of a Drunk
(Continued from User talk:Xenwolf#The Song that Started It All for Me.)
Thanks for the practice piece! So now I can say the harmony is indeed very novel for me, I don’t seem to anticipate its exposition at all. --Arseniiv (talk) 14:27, 1 November 2020 (UTC)
- Is that a good thing or a bad thing? All I know is that most of the people I've talked to seem to actually like the harmony and the surprise modulation. --Aura (talk) 14:40, 1 November 2020 (UTC)
Harmonize categories of interval pages
Hi Aura, we are interested in your opinion about Categories of interval pages, thanks in advance for taking the time. --Xenwolf (talk) 21:19, 8 November 2020 (UTC)
Reply to: Introduction
Please see new reply from SA (SAKryukov). Thank you for your suggestions, but it is still inconvenient, as the channel of communication is based on two different pages, "talk" pages of each of the two, and you probably receive system notifications only if someone edits your "talk" page, and also this is somewhat intrusive. I suggest to support the chat only on one page (in this case, mine), but I can write non-permanent note used just to notify you got a reply. (I removed my previous message as redundant, duplicating the text on (my "talk" page).
- Hi SAKryukov, welcome to the xenharmonic wiki. As to mark a reply as such, just indent it by
:(one more colon than the text you reply to) at the beginning of the line and sign it at the end. This can be done by typing--~~~~(2 hyphens and 4 tildes), which will be automatically transformed at the time you save into your linked username (+ talk page) and a timestamp. (Also the preview does the transformation but not persistently.) --Xenwolf (talk) 14:59, 24 November 2020 (UTC)
Windows and Chrome Version
For Windows version, use the Hotkey [⊞]+[R] then type in winver and press [OK]. For Chrome, open menu (☰) → Help → About Google Chrome (see Image in How-To Geek article). --Xenwolf (talk) 19:54, 24 November 2020 (UTC)
Internal links
Just a short reminder: there is no need for underscores instead of spaces for internal links. See following examples:
- 159edo_notation
- _159edo_notation_
- __159edo___notation__
- 159edo notation
- 159edo notation
- 159edo notation
the last three options differ only in the wiki markup
But honestly, which way looks most natural to you?
The revolutionary idea behind wikis was to support internal linking in the best possible way. MediaWiki, the software that keeps Wikipedia running, and our wiki, tries to go one step further and links other sites in a very convenient way. For example, it doesn't matter if you write the first letter of an article in lower or upper case, so Cent and cent mean the same target page.
This link to the article about interval size measures (it's actually interval size measure) shows another feature: the characters you add directly after the closing ]] are integrated in the link title. This is the reason why Wikipedia articles (as well as most articles in our Wiki) are in singular form.
So much for today about the simplicity of internal linking, I hope my digression did not bore you. --Xenwolf (talk) 20:24, 24 November 2020 (UTC)
Centralized description for Space Tour
I saw that you now have to do the same correction twice. To overcome this, please have look at this option: By placing the following line
{{File:Space Tour.mp3/Description}}
into both pages (File:Space Tour.mp3 and User:Aura) instead of the actual description and the EDO time table, you will get the contents of File:Space Tour.mp3/Description included (without the part above the horizontal rule). See User:Xenwolf/SandBox#Include File:Space Tour.mp3/Description for a live demo. --Xenwolf (talk) 16:31, 4 December 2020 (UTC)
- Hint: Don't include the nowiki tags when copying. Sorry if this is a bit confusing. On my SandBox page, I start with the explanation what to do, only then I do it. --Xenwolf (talk) 19:07, 4 December 2020 (UTC)
Interval test
The file:Interval Test.mp3 seems not very helpful in making the virtual fundamental audible, at least from my perspective. What frequencies are you using? What wave type? --Xenwolf (talk) 21:22, 6 December 2020 (UTC)
- I'm replicating the intervals with Musescore 3's sine wave instrument as close as I can, but unfortunately, I don't have equipment that can replicate the intervals exactly. I don't know the exact frequencies in Hz, but I do know that the scientific names of the pitches I'm using are C7 and A7, with the A7 differing in relationship to the standard C7. --Aura (talk) 21:28, 6 December 2020 (UTC)
- For the record, I do seem to be hearing harmonics of the virtual fundamental for the 27/16 interval- and these harmonics are consistent with the virtual fundamental being a C3- but I don't seem to be hearing the virtual fundamental itself... --Aura (talk) 21:34, 6 December 2020 (UTC)
- Hmm... if it is wider, then that would mean I accidentally made a typo when tweaking the A for the second interval in the file- lowering the A by 15.65 cents instead. My bad. My main concern however, is whether or not the fundamental of the 27/16 can be heard at all. I mean, I'm hearing what sounds like the virtual fundamental's 10th and 11th harmonics... --Aura (talk) 22:53, 6 December 2020 (UTC)
- That makes sense. I'd wager that 81/80 does have a kind of leading-tone property, but it's a really tense kind of tense and harmonically-disconnected leading-tone property as opposed to the more lax and harmonically-connected leading-tone property of an interval like 16/15. --Aura (talk) 23:19, 6 December 2020 (UTC)
- Another strange thing I found out is that, according to Wikipedia's article on the subject, apparently not everyone can hear the virtual fundamental. -Aura (talk) 22:05, 6 December 2020 (UTC)
- Once I added E7-13.69c and G7+1.96c, and turned the volume up, I started hearing the C3 fundamental for the chord featuring the 27/16 interval, and an F3 fundamental for the chord featuring the 5/3 interval- they both have a lousy quality though because of the errors in tuning. --Aura (talk) 23:36, 6 December 2020 (UTC)
Reduce comma tables on EDO pages
Please have a look at Xenharmonic Wiki: Things to do #Comma tables in EDO_pages. Thanks --Xenwolf (talk) 09:10, 11 January 2021 (UTC)
Aura's Diatonic Scales: naming fix
Dawson, I think the mode naming for the Mixolydian scale needs to be fixed: the adjective “Myxic” should be replaced with “Myxolydic”. This way, this adjective will be consistent with your naming schema, in particular, with “Lydic”. — SA, Sunday 2021 February 14, 17:49 UTC
- Actually "Myxic" comes from "Myxian", which is actually a contracted form of "Mixolydian", as used in the coinage of mode names like "Lydomyxian" and "Myxaeolian", see Wikipedia's Article on the Jazz Scale. The reason for this use is that "Mixolydian" is too long for use in forming other mode names, so a shortened form was created for this purpose. Given this etymology, we can assume that "Myxic" and "Mixolydic" are perfect synonyms. --Aura (talk) 18:31, 14 February 2021 (UTC)
- “Myxolydic” then, not “Mixolydic” :-). Okay, maybe you have the reason. In all cases, I strongly suggest using only “Myxolydic” in the Microtonal Playground application, where it is much better to see consistent names, as they all come together. I've designed a way to represent all 49 modes in one window; you will see 7 main modes per each scale (Ionian for Ionian scale, Doric for Doric, etc.), and can dynamically switch to any other of 7 modes by Ctrl+Click, with proper changes in titles, key marks. and transposition to the new tonic. It's going to be quite playable. I think we need to discuss all this. — SA, Sunday 2021 February 14, 19:22 UTC
- Also, I would strongly suggest you think about your idea of using any shortened form of anything. In part, this is non-computer thinking, where we have to write and type anything manually. In case of even a little hardness, we don't do this anymore, right? We need to think exclusively about readability, not about writing. If some word is too long, you need to understand perfectly: where exactly it may not fit? can it be confused with some other word? If you cannot answer such questions, rethink shortening, maybe it can only make things worse. Well, just an idea to share... — SA, Sunday 2021 February 14, 19:29 UTC
- For the record, I do think that “Mixolydic” works as an adjective in describing the modes of Mixolydian, however, my reservations have more to do with when the diatonic mode names are eventually combined with each other in order to name and describe non-diatonic modes, which I do eventually plan on covering at some point. If we use "mixolydic" as the 'only' adjective, we'd get "lydomixolydic", and "mixolydaeolic"- which are rather unwieldy, even in spoken form, not to mention that the instance of "lyd-" in "mixolydaeolic" makes it sound like the mode in question is a cross between Lydian, Mixolydian and Aeolian rather than just a cross between Mixolydian and Aeolian. I guess you can say that the shortened forms are more useful as combining forms on account of issues like this. As long as we consider that caveat, I can indeed change "Myxic" to the more obvious "Mixolydic". Does that make sense? --Aura (talk) 19:52, 14 February 2021 (UTC)
- I should also mention one final thing- the reason why the "i" in the original shortened form "Mixic" was changed to "y" to make "Myxic" is so that we don't confuse "Myxic" with something pertaining to mixing or mixtures. Therefore, in order to avoid multiple levels of confusion in combining forms, "Mixolydian" has the shortened form "Myxian" for usage as a base combining form. I hope this makes more sense. --Aura (talk) 20:12, 14 February 2021 (UTC)
- Thank you for correcting me. Sure, should be “Mixolydic”. I produced “Myxolydic” under the influence of “Myxic”, as I can see it now. :-) — SA, Sunday 2021 February 14, 22:05 UTC
- For the record, “so we don't confuse” is not a sufficient reason for modifying a letter while creating neologisms. New words also tend to follow cultural tradition, not just convenience or something, so it's always better not to break this principle. — SA, Sunday 2021 February 14, 22:10 UTC
- True, but if the Wikipedia article on the Jazz scale is any indication, there does seem to be a bit of an emerging tradition to use "myx-" and "myxian" as the shortened combining forms of "Mixolydian", as in "Lydomyxian" and "Myxaeolian". So in effect, that's ultimately the fault of whoever created the words "Lydomyxian" and "Myxaeolian" in the first place, and I can tell you that that was someone else's idea. --Aura (talk) 22:42, 14 February 2021 (UTC)
- Fair enough — this is a known kind of flexibility in English. My note was rather on the general approach to creating neologisms. — SA, Monday 2021 February 15, 15:47 UTC
- By the way, on your page, I found a striking example of English i-y flexibility: “Dystopia”. A good name for composition, by the way. It's really “dystopia” but compare — “disorientation”, with extremely close functions of dys-/dis-... — SA, Monday 2021 February 15, 18:47 UTC
Comma name inconsistency
So you named 1701/1700 the "palingenesis comma" and "palingenesia" with adjective "palingenic". Earlier you named 19712/19683 the "symbiosma" and "symbiotic comma" with adjective "symbiotic". That's an instance of inconsistency, considering both are English words derived from Greek and with the ending -sis.
My suggestion:
- comma name: palingenesis/symbiosis or palingenetic/symbiotic comma.
- temperament/chord name: palingenetic/symbiotic temperament/chords.
What do you think? FloraC (talk) 07:50, 15 February 2021 (UTC)
- Ah. Part of the problem was that I was looking at the wrong adjective for "palingenesis". As to the comma names, I think that both of your suggested options are viable and should both be valid. I'll fix the names accordingly.
- Let's not shy away from analysing the names, since I reckon it not preferable for a comma to have lots of aliases. At least it's good to have a single-word canonical form so as to ease categorization on this wiki. The "-tic comma" and "-tic chords" pair seems most convincing. It's how most other comma names that don't end in -ma are used in this context. The "-sis" (without "comma") is reminiscent of "diesis", so syntactically those are viable as well, but semantically questionable (they're plain English words that already have meanings). The "-sis comma" version makes more semantic sense but less syntactic sense, cuz "diesis" clearly doesn't take "comma". The "-ma" version, I'm not quite convinced of. If you check other such commas, most of them are either (1) Greek words originally ending in -ma e.g. limma, schisma, (2) Germanic or Arabic words e.g. grossma, rastma, and (3) proper nouns e.g. orwellisma. That's my analysis. Anyway I'm moving palingenic chords > palingenetic chords, and symbiosma > symbiotic comma.
- You may not by sure if going for 27-odd-limit is a good idea, but I sure think it is, because I do indeed go for 27/16 rather than 5/3 for the Major Sixth in my own compositions when I use Diatonic scales. That said, I do see plenty of articles getting generous additions as opposed to being entirely rewritten- as if the 27-odd-limit is simply taking things to the next level for more advanced chords. --Aura (talk) 15:56, 16 February 2021 (UTC)
Probably defective media file
I noticed that the .mp3 file in this section is pretty much noise, not what you intended to demonstrate. Please check it up.
By the way, do you know if other media files are supported on this site the same way? These days, the most usable Web standard is Opus, and it provides the best combination of compression, quality, and latency. MP3 is not open source and generally is one of the worst standards ever designed. It was rendered obsolete more than a decade ago. It can be clearly seen in the Web video. (I don't mean some industries such as car audio, notorious with their media retards.) Anyway, I know who I can ask... — SA, Monday 2021 February 15, 18:16 UTC
Space Tour, Welcome to Dystopia, and 159edo
Hey Aura, I just listened to your two 159edo compositions, “Space Tour” and “Welcome to Dystopia”, and I thought you would like to hear some feedback. I think both of your compositions are incredible and I can’t imagine how long it must have taken you to detune everything by hand. I also have a few questions. First of all, what is the approximation of 159edo that you use and how is it different from 159edo itself? Second of all, how did you decide which EDOs “Space Tour” would mimic at different parts of the song? Userminusone (talk) 00:44, 19 February 2021 (UTC)
- The approximation of 159edo that I use differs from 159edo itself by fractions of a cent. The reason it's only an approximation is because I can only specify tuning to within two decimal places in MuseScore- the main program I use to compose and generate sound- as opposed to being able to straight-up divide the octave into 159 equal parts as would be required to have true 159edo. As for how I decided what EDOs to mimic, well, some were easy- like 12edo, 24edo and 53edo, while most of the others were inspired by the comments of others concerning said EDOs in the Table of EDO impressions. --Aura (talk) 02:25, 19 February 2021 (UTC)
- Thanks for your reply. I think it's interesting that you feel the need to specify that you aren't writing in true 159edo just because MuseScore has a limitation to its retuning capabilities that most likely nobody will notice. I also write microtonal music in MuseScore, which means that my compositions are also tuned very slightly differently from true EDOs. Personally, I don't think that the slight discrepancies that come from the retuning limitations of various music making software and hardware have to be accounted for. In fact, I think it would be better for these discrepancies to be ignored if they are only a few hundredths of a cent wide, as is the case for your compositions. Do you have any additional thoughts on this matter? --Userminusone (talk) 03:10, 19 February 2021 (UTC)
- It maybe true that these discrepancies won't be noticed by listeners, but the fact that I'm the one doing the tuning and cent-size look ups for various intervals means that I would feel as if I were being somewhat disingenuous to the more strict analysts who use computers for their analysis if I didn't add that disclaimer. I hope this makes some degree of sense. --Aura (talk) 03:17, 19 February 2021 (UTC)
MuseScore 159edo retuning plugin...
Hey Aura, I’ve been thinking about how a 159edo retuning plugin for MuseScore could be made, so I want to explain the main issue and offer 3 potential solutions. This block of text comes directly from the n-tet retuning plugin README. “A maximum of 3 arrows are allowed on each accidental, as MuseScore currently does not provide accidentals with more than 3 arrows. Due to these limitations, and with the help of quartertone accidentals, the plugin can only handle EDOs with a sharpness rating of up to 8.” The main problem here is that 159edo has a sharpness rating of 15 (one sharp equals 15 steps), meaning that there would have to be support for septuple up and septuple down accidentals in MuseScore for each note in 159edo to be accessible. (Assuming usage of ups and downs notation without lifts and drops). Here are my three solutions. The first solution is to come up with a notation system for 159edo using accidentals that can be found in MuseScore’s advanced palate. Then, a fork of euwbah’s n-tet retuning plugin would have to be made which could map all of the accidentals to edostep offsets. (Sharp equals 15 steps, triple up natural equals 3 steps, etc) The second solution is to use ups and downs notation from 53edo combined with “+” and “-“ symbols to indicate 1 step offsets for 159edo. For this to be effective, the plugins which raise and lower a note by one edostep (these plugins are included with the n-tet retuning plugin) would have to be modified to add and remove the “+” and “-“ symbols as necessary as well as changing the accidental and the pitch offset. The third solution is to use polychromatic notation for 53edo and to use the up and down arrow accidentals to access the notes in 159edo. Again, the pitch up and pitch down plugins would have to be modified. This time, these plugins would have to change the note head color as well as changing the accidental and the pitch offset. I would like to know which option you think is best for making 159edo compositions in MuseScore. In addition, since the programming language for MuseScore (Qt) is a bit too confusing for me to make anything meaningful in, you’ll have to ask FloraC to do the actual coding. I might be able to help a little bit, but not much. --Userminusone (talk) 18:34, 19 February 2021 (UTC)
- Is it possible that there's a fourth solution? I mean, I already have a planned set of accidentals that seems to work, but they seem to need refining in terms of their design. In addition to the traditional natural, sharp, flat, double sharp and double-flat accidentals there are two new sets of quartertone accidentals, and an interesting set of tree-like arrows.
- The above image shows the new quartertone accidentals, while the image below shows the remainder of the new accidentals.

- top row from left to right:
- rastma wide
- biyatisma wide
- syntonic wide
- syntonic + rastma wide
- syntonic + byatisma wide
- double syntonic wide
- bottom row from left to right:
- rastma narrow
- biyatisma narrow
- syntonic narrow
- syntonic + rastma narrow
- syntonic + byatisma narrow
- double syntonic narrow
- I hope the above accidentals make sense. I mean, I'm trying to make my approach to this whole thing as clean and straighforward as possible. --Aura (talk) 19:45, 19 February 2021 (UTC)
- It seems like you’re thinking of using custom accidentals with MuseScore. As far as I know, MuseScore has absolutely no support for importing custom accidentals, but I could be wrong. Even if MuseScore could support custom accidentals, I don’t think the custom accidentals could be utilized in a plugin because the plugin would have to be able to recognize these new accidentals with internal accidental codes. Since I know almost nothing about Qt, the programming language used to make plugins in MuseScore, you’ll have to double check with FloraC if your fourth solution is possible/feasible. --Userminusone (talk) 20:05, 19 February 2021 (UTC)
- For now, while custom accidentals cannot be supported in MuseScore, wouldn't you rather have the detuning done automatically by a modified version of the n-tet retuning plugin rather than you having to input all the cent offsets yourself?
- This image shows all of the microtonal accidentals currently in MuseScore. I'm sure at least some of them can temporarily suit your needs. --Userminusone (talk) 21:08, 19 February 2021 (UTC)
- I'm willing to wait for MuseScore 4 for this considering that that version is currently in the works, in part because some of the accidentals that could otherwise be the most useful have been found to present legibility issues. However, I don't think it will hurt to at least get the ball rolling on this one. With that, do you have the time and or resources to help me finalize the designs of these custom accidentals of mine? Once that's done, we can talk to Euwbah and others about how to implement these in MuseScore 4. --Aura (talk) 21:14, 19 February 2021 (UTC)
- Well, I do have some time this weekend, but I wouldn’t say I have the resources to assist with making custom accidentals. I’m not an artist and I don’t have any kind of glyph editing programs or other art making programs. I can give feedback on your custom accidentals every now and then but I can’t help directly with the accidental making process. My main reason for asking you about this plugin is to give you a way of composing in 159edo without having to worry about retuning the notes manually. That’s why I think it would be better for you to have a temporary solution that doesn’t require any custom accidentals whatsoever. --Userminusone (talk) 21:42, 19 February 2021 (UTC)
New Comma
Hey Aura, can you give me your thoughts on the somewhat esoteric comma that I called the "Goldis" comma? I particularly would like to know if you have a better name for it and if you can think of higher limit extensions to the temperament. (The link to the page is on my user page) --Userminusone (talk) 21:58, 18 March 2021 (UTC)
A potentially useful thing I came up with...
Hey Aura, I have another thing that I would like you to check out and give me your thoughts on. It might be more up your alley as it is based on Just Intonation. It's called the Averiant. --Userminusone (talk) 22:46, 25 July 2021 (UTC)
- I checked it out and I'm not sure what to make of it at the moment. --Aura (talk) 14:43, 26 July 2021 (UTC)
- That's understandable. One thing I didn't mention, though, is that the Averiant could be useful for finding new commas. As an example, the comma 128/125 can be derived by taking the (1/3)averiant between 2/1 and 1/1 to get 5/4, and then equating 5/4 with 1/3 of an octave (or equivalently, equating 125/64 with an octave). 128/125 is quite a large comma but my point is that this approach could theoretically be used to find much smaller commas. --Userminusone (talk) 21:31, 26 July 2021 (UTC)
- Sorry for the late reply, but to answer your question, one thing you could do is start with three simple ratios and find the averiantal percentage of the middle ratio relative to the two outer ratios, which you can then use to derive the comma. As an example, I could calculate the (1/1 - 5/4)averiantal percentage of 8/7, which is 3/5. To derive a comma from this, I would equate (8/7)^5 with (5/4)^3, resulting in the rainy comma. --Userminusone (talk) 20:25, 1 August 2021 (UTC)
Please remember to link new edo pages from the parent page
Please remember to add a link in the EDO page when you create new edo pages. FloraC (talk) 09:08, 23 December 2022 (UTC)
Where is Folly of a Drunk?
Where is it now? I love it very much! Can I listen to it and refer to friends?
I recently listened to all I found on your pages, like it.
Thank you.
—SA
- Hey! It's been a while since we last talked! I'll have to reestablish Folly of a Drunk on here, but I'm not in the position to do that right now. Besides, you might also want so hear Kite's arrangement. --Aura (talk) 18:54, 15 July 2023 (UTC)
- Thank you for your reply. I recently wrote to you on Youtube page “How Many Notes Are There? The Theory of Quarter Tones” (not very good one). I have a lot of new development, including Microtonal Fabric. It's too early to discuss now. But I must say that Microtonal Fabric is permanently used in Brainin School of Music for a pretty long time now. Brainin reported that little children master feeling micro tones wonderfully well and show unmatched level of musical intellect. Professional musicians usually fail to solve the problems well solved by those little students… --SA (talk) 13 July 2023
- Where is that Kite's arrangement? Yes, I would like to hear it. Will you give me a link? --SA (talk) 13 July 2023
- Here you go: https://youtu.be/fOZiX7f7t8Q?t=806 --Fredg999 (talk) 00:15, 14 September 2023 (UTC)
Temperament(s) for Diatonicized Chromaticism?
I have been listening to the music of Ivan Wyschnegradsky (whose page needs expansion) and looking up how to put 11L 2s scale that he called "Diatonicized Chromaticism" into one or more temperaments. My thoughts on this in part can be found at Musical Mad Science Musings on Diatonicized Chromaticism (although this needs updating and filling in. Searching for relevant intervals and commas has led me to The Nexus and to the conclusion that you would be the person to talk to about this. Added: Lucius Chiaraviglio (talk) 15:14, 28 March 2025 (UTC)
- Sorry I didn't get to this sooner. Oddly enough, while I'm not terribly familiar with many aspects of this scale, I do know that 159edo supports it, with the large step at 13\159 and the small step at 8\159. It should be noted that 13\159 represents 128/121, while the small step represents 28/27- the latter is tempered together with 729/704 because the symbiotic comma is tempered out. --Aura (talk) 02:36, 6 April 2025 (UTC)
- Thanks -- I had also noticed that 159edo supports this scale, although I didn't track down what the steps temper to.
- I took some time out from trying to figure out the temperament for 11L 2s to trying to figure out the temperament for the next higher analog of this scale to serve as a basic scale for a multiple of 12edo: 17L 2s, for which 36edo implements the basic version of this scale. (I have made a start at this under what I linked above, on the same page.) I don't know whether Ivan Wyschnegradsky had this scale in mind when he was composing the few compositions he wrote in 36edo (I have yet to see a score for any of these compositions, and none of the YouTube videos of them are of the scrolling score type), but I was struck by how similar it sounded to his 24edo compositions (although with noticeably smaller steps), so I suspect that he might have had something of the sort in mind. (Unfortunately I haven't seen any of his writings about this, although one of the videos of his 24 Preludes has a substantial excerpt about working with 11L 2s in 24edo, linked from my user sub-page that I linked above.)
- Surprisingly, the going has been easier for 19L 2s than for 11L 2s, despite having had longer to work on figuring out a temperament for 11L 2s. So maybe figuring this out for 19L 2s will teach me how to do it for 11L 2s, although working with the 11th harmonic seems to be just inherently weird.
- Lucius Chiaraviglio (talk) 06:52, 6 April 2025 (UTC)
- Yes, working with the 11/8 paramajor fourth is inherently weird- it takes either the 16/15 diatonic semitone or the 5/4 major third to set it up, and it really seems to like being a chord root in a 1/1-25/22-14/11-3/2 chord. Have you tried working with this stuff? --Aura (talk) 11:29, 6 April 2025 (UTC)
- I haven't worked with it myself, but I am trying to gain an understanding. Obviously it isn't easy — Ivan Wyschnegradsky and a very few others figured it out, but only a very few others. But you gave me an idea of things to test, with the 1/1-25/22-14/11-3/2 chord. Although keep in mind that in 24edo (which Ivan Wyschnegradsky used most of the time, along with Alois Hába and a few compositions of Charles Ives), 14/11 maps down to the same as 5/4 (8\24) due to 24edo's badly flat 7th harmonic, while 25/22 maps not to the closest interval 9/8 as 4\24, but up to the same as 22/19 as 5\24, because its 5th harmonic is sharp (but not sharp enough for mapping as 25 instead of 5^2). But then again, I don't know whether Alois Hába or Charles Ives (ore much more recently, Scott Crothers) ever used 11L 2s as opposed to other ways of using 24edo; 11L 2s may be unique to Ivan Wyschnegradsky. Added: Lucius Chiaraviglio (talk) 19:44, 6 April 2025 (UTC) Last modified: Lucius Chiaraviglio (talk) 00:34, 7 April 2025 (UTC)
- Oddly enough, my experiments with how to handle the 11/8 paramajor fourth relative to the tonic have been largely carried out in 159edo, but I must say that even in 24edo, that 11/8 wants to go to either 3/2 relative to the tonic, or, to 4/3 relative to the tonic- at least in terms of chord roots. It should be noted that the 11/8 seems to literally bring in its own set of related accidentals relative to the key you're in- I've already hinted at 25/16 being dragged in, and the same is true with the 7/4, which, in this capacity, acts like an ultramajor sixth relative to the tonic. Naturally, the 33/32 will likely be dragged in as well, but what I didn't tell you is that 75/64 wants to rear its head in scalar motion on a tIV chord- the only two default scale degrees relative to the tonic that can be used with a tIV chord are the 5/4, and the 15/8. Otherwise, if you use an 11/8, it has to either be part of a 1/1-11/8-3/2 suspension on the tonic, or as part of a 1/1-5/4-3/2-55/32 built on the 8/5 relative to the tonic. There are other chords I know, such as the 1/1-11/8-15/8 built on the tonic, or the 1/1-11/8-7/4 built on the tonic, but these tend to be harder to set up and follow up properly. --Aura (talk) 02:50, 7 April 2025 (UTC)
- Keep in mind that 159edo is going to be a LOT different from the low to mid (and even upper-mid) double digits EDOs. You get literally a lot finer control over how your chords sound. Of course, the downside is that you need an instrument (or group thereof) that can play that many pitches (or you need to be REALLY GOOD on a continuous-pitch instrument). Another thing I noticed is that in 11L 2s it is hard to choose a mode that lets you hit common 5-limit intervals without going off-scale with accidentals. (I need to see how that plays out with 7-limit intervals in an EDO that supports the 7-limit better than 24edo, like 37edo or 59edo or 61edo, although I noticed that if you go all the way afield to 50edo or 46edo, this scale shoots itself in the foot by not mapping intervals of ANY mode to 3/2 or 4/3, which map differently than in 11L 2s EDOs closer to 24EDO in the tuning spectrum. Not sure yet, but I think 17L 2s operating in in the 2.3.7... subgroup may have less of that problem.) Lucius Chiaraviglio (talk) 08:03, 7 April 2025 (UTC)
- I know that 159edo is different to many of the smaller EDOs that you pointed out- there's a reason I chose to specialize in that particular tuning system. Furthermore I should mention that a 53edo-based instrument can have a few extra buttons added so that one may divide the step of 53edo into three- think the Neod, which is mentioned on the 159edo page. Of course, I think it pays to let people hear what 159edo is capable of by means of writing music for that tuning system in MuseScore Studio 4 or later and post the results to YouTube- that way, people might just be inspired to build the instruments necessary for live performance. --Aura (talk) 12:26, 7 April 2025 (UTC)
- On another note, I'm not sure how 11L 2s fares in 159edo in terms of what it can reach, and while it is important to note that 11L 2s can't often hit common 5-limit intervals in as of itself, I should think that detempered versions of that MOS, as well as the MOS itself, could still be useful in 159edo, as that will expand my capabilities. --Aura (talk) 12:26, 7 April 2025 (UTC)
- I saw that Neod video a while back, although I should probably give it another go. I should look at 11L 2s in 159edo once I get back to working on a temperament (or probably clan of temperaments) for that scale. In an EDO that big, you might well want to add some strategic accidentals to the scale. And I have been impressed by a subset of works I have seen written in MuseScore 4 — the capabilities of MuseScore (including synthesizing instrument imitations that actually sound decent) seem to have greatly improved in the last few years. Lucius Chiaraviglio (talk) 23:30, 7 April 2025 (UTC)
- I'll have to check them out (actually listened to 1 already, but quite a while ago, before I started thinking much about this).
- Meanwhile, I thought it would be useful to have a table of odd harmonics of the various EDOs supporting 17L 2s to make it easier to track stability of mapping, and I plan to do this for 11L 2s as well (which so far I had been doing this for by way of manual lookup of each EDO, which is time-consuming). Right now it is actually a collection of tables, because Template:Harmonics in equal doesn't have a way to string them together and doesn't have a way to set a uniform column width. Any idea how to fix this, other than manually creating a table with values copied from these? In the process of doing this, I have noticed an interesting comparison: 11L 2s has a very well-behaved 11th harmonic (it has to, since 11/8 and 16/11 is the generator pair), but doesn't seem to have well-behaved low harmonics; but in contrast, 17L 2s doesn't have a simple generator (23/16 is too flat by a few cents, and 13/9 is too sharp, and has multiple odd harmonics/subharmonics in it anyway), and the harmonics of the compound ratios that could be used as generators for it are NOT well-behaved, except that the 3rd harmonic is amazingly well-behaved, only flipping to a different mapping at 112edo and higher EDO values (and the 23rd harmonic would be well-behaved if only it wasn't flat of the actual generator — it would be an excellent generator for 21L 2s or the soft end of 2L 19s).
- Lucius Chiaraviglio (talk) 20:12, 8 April 2025 (UTC)
- I finished doing the above for 11L 2s. Note that 11/8 (the dark generator, and thereby the bright generator 16/11) remains stable throughout the entire currently posted 11L 2s table &emdash; the worst relative error is -34.8%, at 127edo, so your favorite 159edo still easily fits in. Right now I strung out the odd harmonics to high values in case I need these for composite ratios, which seem likely to be needed for 17L 2s, but seem like they may not be needed for 11L 2s (but better leave them in for now until I am sure they are not needed). Lucius Chiaraviglio (talk) 22:54, 9 April 2025 (UTC)
- Good catch of typo -- fixed this. Also rechecked the rest, but didn't find any more. Lucius Chiaraviglio (talk) 05:30, 10 April 2025 (UTC)
- Scrolling through these table groups, I started noticing interesting things, like how even though the 11th harmonic is the only one with stable mapping all the way through 11L 2s, some of the others have stable mapping in sections, like the 3rd harmonic has stable mapping in the middle section but is all over the place in both the hard and soft ends, but the 9th harmonic actually does okay in the hard end, as does the 17th harmonic (both of these get to be all over the place in the soft end), and the 5th and 13th harmonics have stable mapping in the soft end as long as the EDO values are not too large.
- In partial contrast, with 17L 2s, the harmonic/subharmonics of the generator have unstable mapping (because no simple ratio with a reasonable sized numerator and denominator fits into this zone), but the 3rd harmonic is nearly rock-solid (and 112b is a respectable if overly-complex quarter-comma meantone approximation), although presumably its mapping would break if I put in the rest of the right-most column of the MOS spectrum table. And there the 5th harmonic seems very much usable in the soft end of the scale tuning spectrum as long as the EDO sizes don't get too large (and even then, sometimes it is still okay), which looks to me like enabling a 2.3.5.23 meantone extension; it goes all over the place in the hard end, but there the 25th harmonic shines and is rock-solid as long as you don't go harder than 36edo (basic), and the 13th harmonic just barely misses being rock-solid in this zone (just barely breaks on 125edo, for which 125f would be not bad). Although those harmonics would also appear less solid if I included the rest of the MOS tuning spectrum.
- Lucius Chiaraviglio (talk) 07:28, 10 April 2025 (UTC)
In reply to your previous comment, I should mention that I'd like to know where ~3/2 is relative to the 11L 2s of 159edo. I imagine it's rather far along the generator sequence. At the same time, I'm wondering what sorts of chords you can actually get from 11L 2s in 159edo. --Aura (talk) 16:53, 10 April 2025 (UTC)
- This is further up on my page than the tables of odd harmonics: For the fifths in the basic 11L 2s (Wyschnegradsky) diatonicized chromatic scale (the version that 24edo yields), the 11/8-span of a patent fifth is a stack of 10 intervals of 11/8, octave-reduced. I haven't yet done this calculation for 159edo — it isn't too far off from basic, but it has small enough increments that attempting to use the same 11/8-span gives the b val fifth instead of the patent fifth. 00:07, 11 April 2025 (UTC)
- Did this for 159edo — the 16/11-span of 3/2 is 51. And it doesn't even work for the next EDO up or down from the last column of the tuning spectrum table of 11L 2s (135edo or 146edo, respectively) — the 3rd harmonic mapping is too unstable for EDO sizes that large in this region. Also, 51 is so many iterations of the generator that it goes well outside of the 11L 2s scale. Added: Lucius Chiaraviglio (talk) 05:53, 11 April 2025 (UTC) Last Modified: Lucius Chiaraviglio (talk) 06:52, 11 April 2025 (UTC)
- Fascinating. I guess that means we need to look into chords with 94\159 fifths, which actually approximate 128/85 rather than 3/2. These chords clearly cannot be pure 5-limit either, but that's okay. Given that you mention 2.3.5.23 meantime, I'm now wondering if we can cobble together something for 11L 2s based on the ~128/85 archagall fifth. To start on this front, what are the modes of 11L 2s that contain ~128/85 in 159edo? --Aura (talk) 10:40, 11 April 2025 (UTC)
- I need to do the modes for 11L 2s with respect to coverage of standard diatonic intervals anyway, but that's going to take a bit of time, so stay tuned. The 128/85 b fifth is going to stick out like a sore thumb in the midst of the much more accurate other intervals that 159edo has, though, so maybe it would be better to try to come up with a decent MODMOS derived from 11L 2s for 159edo? In the meantime, I figured out which equal temperaments this might apply to, since they get their fifth from 51 stacked and octave-reduced 16/11 generators, leading up to 159edo: 37b, 61, 98, 159 (have not yet tried to extend beyond 159edo to see how far you can get before the fifth mapping breaks again). Note that for 61edo, 51 stacked and octave-reduced 16/11 generators gives the same note as 10 stacked and octave-reduced 11/8 generators. I also included 37b to show the small endpoint of the series (and if you really want to go weird, include 24b); judging by the pattern, the next member of the series would be 257 (not yet sure of wart, if any). Graham Breed's temperament finder lists some more members of the series without warts, going up into the thousands, but doesn't give the temperament a name beyond 159 & 98, and I don't see 61edo listed in the Nexus, Nexus clan, or Nexus family, although 159edo comes up several times (maybe one of those temperaments would be better, although they probably go from the fifth to the 11/8 rather than the other way around, and would not necessarily support 11L 2s). Its enormous complexity is presumably the reason it never got a name; I don't know the specifics for computing badness, but I am going to stick my neck out and guess that this temperament would have huge badness despite its high accuracy.
- As for 2.3.*.23, the 23rd harmonic mapping is pretty unstable in the zone of 11L 2s — it gets better mapping stability in 17L 2s, although this region has its generators sharp enough relative to 23/16 that larger EDO sizes often flip to the next flatter approximation, so the generator for that needs to be something between 23/16 and 13/9.
- Lucius Chiaraviglio (talk) 15:21, 11 April 2025 (UTC)
- The modes of 11L 2s that enable reaching 4/3 and/or 3/2 within-scale are not very many. If you want to get both, you have to use sLLLLLsLLLLLL (no other mode gets both). If you want to get 3/2 but can live without 4/3 (more common than the opposite), then you can also use LsLLLLLsLLLLL or sLLLLLLsLLLLL. If you use LLLLLLsLLLLLs or LLLLLsLLLLLLs or LLLLLsLLLLLsL you can get 4/3 but not 3/2. In EDOs with fine enough steps to distinguish 128/85(?) from 3/2, this instead gets you 128/85(?) (or 85/64(?) instead of 4/3). So if we want a MODMOS for 159edo that gets us back to 3/2 (and maybe 4/3) we need a second generator that gets tempered to be the same as 11/8 or 16/11 in the coarser EDOs supporting 11L 2s but distinguished in the finer ones. Not sure yet what that would be, and not sure yet whether 128/85 is the best slightly-inflated fifth substitute to use for this purpose. Lucius Chiaraviglio (talk) 00:02, 12 April 2025 (UTC)
- Well, the ~128/85 fifth of 159edo is indeed useful in its own right as an imitation of the kind of thing you see in 22edo. Perhaps we can play to the strengths of that type of system where two ~128/85 intervals octave-reduced add up to ~17/15, and two instances of ~17/15 add up to ~9/7- yes, that is how 159edo works since both 22edo and 159edo support archagall temperament. Not only that but the ~128/85 interval is 159edo's best approximation of 17edo's fifth, so we could also shoot for 11L 2s harmonies that evoke the kind of stuff found in 17edo and it's multiples. The point is that if the ~11/8 and ~16/11 intervals count as ambisonances (basically, they're both consonances and dissonances at the same time) for our intents and purposes, as I know they do for mine, then we can afford to play with some of the less accurate intervals in our 11L 2s harmonies- assuming we play our cards right. --Aura (talk) 04:28, 12 April 2025 (UTC)
- Mavka a.k.a. archagallismic looks sort of like what we want, although that seems like more dimensions than we need, and I don't know how you would map that many dimensions onto any kind of keyboard, even before considering the size. (But maybe that could be trimmed down to a rank-3 temperament that is different from Archgallic, so as to get the 11/8 in there?) I see that its multiple generators include ~3, and it includes 150edo as a patent val, so you can get both 128/85 and 3/2. Lucius Chiaraviglio (talk) 08:25, 12 April 2025 (UTC)
- If I can map 159edo onto an isomorphic keyboard layout using tertiaschis temperament, albeit by squashing the hexagons, then we can work together to map a lower-dimensional temperament that relates to archagallic temperament. --Aura (talk) 09:39, 12 April 2025 (UTC)
- Tertiaschis looks interesting. Meanwhile, I determined that the Rastma (243/242) is NOT the right interval for differentiating 159edo from 61edo (lower on the series I noted above) — 159edo maps it correctly to 1 increment, but 61edo not only doesn't temper it out, but inflates it to 2 increments, while 98edo inverts it. Lucius Chiaraviglio (talk) 18:16, 12 April 2025 (UTC)
- Should have tried the Char comma/chroma (256/255) first. This is tempered out in 24edo, 37edo, 61edo, and 98edo, but correctly maps to 1 increment of 159edo; it is exactly the amount by which an archagall fifth exceeds a normal fifth. Lucius Chiaraviglio (talk) 10:13, 13 April 2025 (UTC)
- That's the idea. Although other parts of the 11L 2s tuning spectrum may need alternate syntonic comma/chroma analogs, just like some parts of the 5L 2s tuning spectrum are best served by 64/63 instead of 81/80. (And sorry about the typo in the 256/255 page.) Lucius Chiaraviglio (talk) 21:12, 13 April 2025 (UTC)
- Checking in to let you know I didn't forget about this. In addition, I've been coming to the conclusion that while 17L 2s seems to fit with temperaments that proceed along at least part of the vertical axis of the corresponding tuning spectrum table, 11L 2s requires temperaments that proceed along the horizontal axis of the corresponding tuning spectrum table. Lucius Chiaraviglio (talk) 01:49, 21 April 2025 (UTC)
- Also, 256/255 currently has an edit war going on about whether its names should include "char comma", from our point of view, "char comma" would have been nice for enabling "char chroma" in tuning systems that use it as an interval instead of tempering it out. I thought about putting this in the associated discussion page, but the edit war is going on with apparently no inclination to put any further discussion there (although plenty of old discussion about naming exists there).
- Separately, work on 17L 2s is looking like it might give me a decent 2.3.5.13.23 meantone extension (but still need to check 13th harmonic mapping stability to be sure).
- Lucius Chiaraviglio (talk) 10:20, 21 April 2025 (UTC)
- For 17L 2s, had to go for a 2.3.5.23.53 meantone extension to get the soft half of the spectrum — the 13th harmonic mapping just wasn't stable enough. Now that I've got that done, I wonder if giving the very high harmonics another look might turn up something similar for 11L 2s? Bonus points if it works accurately enough to include an EDO as large as 159edo (for the 17L 2 meantone extension, 146edo was too big to fit without an accuracy-degrading 'c' wart as well as an 'i' wart). Lucius Chiaraviglio (talk) 10:13, 22 April 2025 (UTC)
- On second thought, what turned out to be necessary for (at least the soft half of) 17L 2s might not help for 11L 2s, because the former didn't have a stable generator fraction within range, whereas the latter does &mbdash; hence the same extraordinary measures might not do much for it. But it can't hurt to check. Lucius Chiaraviglio (talk) 11:28, 22 April 2025 (UTC)
- Sorry about not responding for a while, I had stuff to do, but I was reading what you were saying, and I've been checking in on harmonies that you can get from instances of ~85/64 and ~128/85. It seems that in 159edo, starting on your ~17/16, you can build an approximation of a 1/1-25/22-128/85-320/187 suspension easily, and you can use this as an unexpected option for something resembling a Neapolitan chord. Not sure how this chord fits with 11L 2s however- it probably doesn't. --Aura (talk) 16:24, 24 April 2025 (UTC)
- 11L 2s won't include 25/22 (29\159, which is 3\159 too high) or 320/187 (123\159, which is also 3\159 too high — I just checked to make sure it maps correctly, although 200/117 is a lot more accurate). I was thinking maybe a MODMOS derived from 11L 2s would do it, but for that to work, one would have to be too high and the other too low. So it would have to be made with accidentals. Of course, 159edo has a load of other scales, so maybe one of these might do the job without accidentals. Lucius Chiaraviglio (talk) 18:10, 24 April 2025 (UTC)
- I don't know if this is a good temperament for 11L 2s, but I stumbled upon Tritricot while looking up stuff for temperamental generator of temperaments supporting 17L 2s: All listed *-limits of Tritricot list 159edo as their first optimal tuning. (And even if Tritricot isn't optimal for 11L 2s, it WILL work by hook or by crook, since all EDOs listed in the optimal tuning sequence are ≥159edo, and 159edo is already >143edo, which is the largest EDO that DOESN'T support 11L 2s, being to 11L 2s what 35edo is to 5L 2s. But I have yet to check whether you have to use some awful number of generators to get 11/8 or 16/11. Although at that high an EDO size, a MODmos based upon 11L 2s is likely to be better anyway, and for that you're going to want at least a secondary generator.) Lucius Chiaraviglio (talk) 05:05, 28 April 2025 (UTC)
Notability guidelines review
I drafted up a new proposal for the notability guidelines over at User:Sintel/Notability guidelines. You can use the talk page there to let us know what you think about them, especially in context of the discussion on the previous proposal wrt some of the pages you created.
Thank you! – Sintel🎏 (talk) 23:46, 7 May 2025 (UTC)
- Note for future readers: this conversation moved to User talk:Sintel/Notability guidelines from this point. --BudjarnLambeth (talk) 02:41, 8 May 2025 (UTC)