11edo
| ← 10edo | 11edo | 12edo → |
(semiconvergent)
11 equal divisions of the octave (abbreviated 11edo or 11ed2), also called 11-tone equal temperament (11tet) or 11 equal temperament (11et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 11 equal parts of about 109 ¢ each. Each step represents a frequency ratio of 21/11, or the 11th root of 2.
Theory
| Harmonic | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 | 21 | 23 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -47.4 | +50.0 | +13.0 | +14.3 | -5.9 | +32.2 | +2.6 | +4.1 | +29.8 | -34.4 | +26.3 |
| Relative (%) | -43.5 | +45.9 | +11.9 | +13.1 | -5.4 | +29.5 | +2.4 | +3.8 | +27.3 | -31.5 | +24.1 | |
| Steps (reduced) |
17 (6) |
26 (4) |
31 (9) |
35 (2) |
38 (5) |
41 (8) |
43 (10) |
45 (1) |
47 (3) |
48 (4) |
50 (6) | |
Compared to 12edo, the intervals of 11edo are stretched:
- The "minor second" at 109.09 cents, functions melodically very much like the 100-cent minor second of 12edo.
- The "major second" at 218.18 cents, works in a similar fashion to the 200-cent major second of 12edo, but as a major ninth, it may sound less concordant. Its inversion, at 981.82 cents, can function as a "bluesy" seventh relative to 12edo's 1000-cent interval, although it is still about 13 cents away from 7/4.
- The "minor third" at 327.27 cents, is rather sharp and encroaching upon "neutral third".
- The "major third" at 436.36 cents, is quite sharp, and closer to the supermajor third of frequency ratio 9/7 than the simpler third of 5/4.
- The "perfect fourth" at 545.45 cents, does not sound like a perfect fourth at all, and passes more easily as the 11/8 superfourth than the simpler perfect fourth of 4/3.
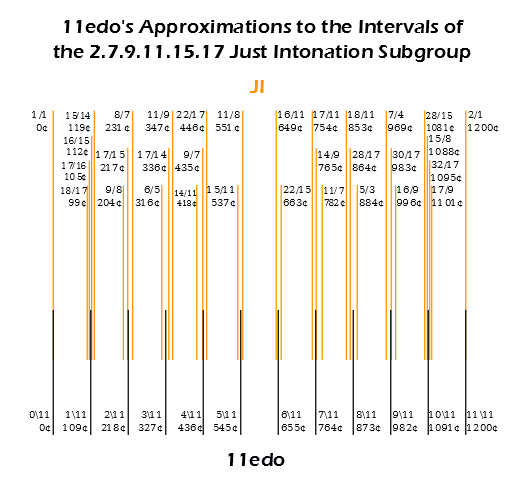
11edo does not approximate many small prime harmonics well, only providing good approximations to 7/4 and 11/8. However, 11edo can be treated as a subset of 22edo, and take 22edo's 6/5, 9/7, and 16/15 via direct approximation.
11edo provides the same tuning on the 2*11 subgroup 2.9.15.7.11.17 as does 22edo, and on this subgroup it tempers out the same commas as 22. Also on this subgroup there is an approximation of the 8:9:11:14:15:16:17 chord and its subchords. Though the error is rather large, this does provide 11 with a variety of chords approximating JI chords.
11edo has a good approximation of 9/7, hence one natural approach to harmony in 11edo is to generate chords from stacks of this interval. Incidentally, correcting the tuning of 9/7 to just tuning and stacking this interval has the beneficial side effect of also improving the tuning of the 17th harmonic to almost exactly just intonation, with an error of only 0.3 cents. It may therefore be worth considering this JI tuning as an alternative to 11edo.
Being less than twelve, 11edo maps easily to the standard keyboard. The suggested mapping disregards the Ab/G# key, leaving Orgone[7] on the whites. The superfluous Ab can be made a note of 22edo, a tuning known as "elevenplus".
Notation
Ups and downs notation
11edo can be notated using ups and downs. Conventional notation, including the staff, note names, relative notation, etc. can be used in two ways. The first preserves the melodic meaning of sharp/flat, major/minor and aug/dim, in that sharp is higher pitched than flat, and major/aug is wider than minor/dim. The disadvantage to this approach is that conventional interval arithmetic no longer works. e.g. M2 + M2 isn't M3, and D + M2 isn't E. Chord names are different because C - E - G isn't P1 - M3 - P5.
The second approach preserves the harmonic meaning of sharp/flat, major/minor and aug/dim, in that the former is always further fifthwards on the chain of fifths than the latter. Sharp is lower in pitch than flat, and major/aug is narrower than minor/dim. While this approach may seem bizarre at first, interval arithmetic and chord names work as usual. Furthermore, conventional 12edo music can be directly translated to 11edo "on the fly".
The 11edo solfege in the table is derived from 22edo solfege.
| # | Cents | Solfege | Approximate Ratios* | Up/down notation with major wider than minor |
Up/down notation with major narrower than minor |
Smitonic (3rd-gen) notation |
TDW Machine notation |
Pseudo-Diatonic Category | Audio | ||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0.00 | do | 1/1 | P1 | A | P1 | A | A | Q, P# | Unison | |
| 1 | 109.09 | ra | 15/14, 16/15, 17/16, 18/17 | ^1, m2 | ^A, B | ^1, M2 | ^A, B | A#, Bb | Q#, Rb | Minor second | |
| 2 | 218.18 | re | 8/7, 9/8, 17/15 | ~2, m3 | ^B, Cb | ~2, M3 | ^B, C# | B | R | Major second | |
| 3 | 327.27 | me | 6/5, 11/9, 17/14 | M2, ~3 | B#, vC | m2, ~3 | Bb, vC | C | R#, Sb | Minor third | |
| 4 | 436.36 | mo | 9/7, 14/11, 22/17 | M3, v4 | C, vD | m3, v4 | C, vD | C#, Db | S | Major third/Minor fourth | |
| 5 | 545.45 | fu | 11/8, 15/11 | P4, v5 | D, vE | P4, v5 | D, vE | D | S#, Tb | Major fourth | |
| 6 | 654.55 | su | 16/11, 22/15 | ^4, P5 | ^D, E | ^4, P5 | ^D, E | D#, Eb | T | Minor fifth | |
| 7 | 763.64 | lo | 14/9, 11/7, 17/11 | ^5, m6 | ^E, Fb | ^5, M6 | ^E, F# | E | T#, Ub | Major fifth/Minor sixth | |
| 8 | 872.73 | la | 5/3, 18/11, 28/17 | ~6, m7 | vF, Gb | ~6, M7 | vF, G# | F | U | Major sixth | |
| 9 | 981.82 | ta | 7/4, 16/9, 30/17 | M6, ~7 | F, vG | m6, ~7 | F, vG | F#, Gb | U#, Pb | Minor seventh | |
| 10 | 1090.91 | ti | 15/8, 17/9, 28/15, 32/17 | M7, v8 | G, vAv | m7, v8 | G, vAv | G | P, Qb | Major seventh | |
| 11 | 1200.00 | do | 2/1 | P8 | A | P8 | A | A | Q, P# | Octave | |
* in 2.7.9.11.15.17 subgroup
The ups and downs notations above are heptatonic systems generated by 5ths (~3/2). Alternative notations include pentatonic 5th-generated, octatonic 5th-generated, nonatonic 5th-generated, heptatonic 3rd-generated, and hexatonic 2nd-generated.
Pentatonic 5th-generated: D * * E G * * A C * * D (Sensoid generator = wide 3/2 = 7\11 = perfect 5thoid)
D - ^D/Eb - D#/vE - E - G - ^G/Ab - G#/vA - A - C - ^C/Db - C#/vD - D
P1 - ^1/ms3 - A1/~s3 - Ms3 - P4d - ^4d/d5d - A4d/v5d - P5d - ms7 - ~s7/d8d - Ms7/v8d - P8d (s = sub-, d = -oid)
pentatonic genchain of fifths: ...Cb - Gb - Db - Ab - Eb - C - G - D - A - E - C# - G# - D# - A# - E#...
pentatonic genchain of fifths: ...ds3 - ds7 - d4d - d8d - d5d - ms3 - ms7 - P4d - P1 - P5d - Ms3 - Ms7 - A4d - A1 - A5d - As3 - As7... (s = sub-, d = -oid)
Octatonic 5th-generated: A B * C D E * F G * H A (Sensoid generator = wide 3/2 = 7\11 = perfect 6th)
A - B - B#/Cb - C - D - E - E#/Fb - F - G - G#/Hb - H - A
P1 - m2 - M2/m3 - M3 - P4 - m5 - M5 - P6 - m7 - M7/m8 - M8 - P9
octatonic genchain of sixths: ...Db - Ab - Fb - Cb - Hb - E - B - G - D - A - F - C - H - E# - B# - G# - D# - A#...
octatonic genchain of sixths: ...d7 - d4 - d9 - d6 - m3 - m8 - m5 - m2 - m7 - P4 - P1 - P6 - M3 - M8 - M5 - M2 - M7 - A4 - A1 - A6 - A3...
Nonatonic 5th-generated: A B * C D E F G * H J A (Joanatonic generator = narrow 3/2 = 6\11 = perfect 6th)
A - B - B#/Cb - C - D - E - F - G - G#/Hb - H - J - A
P1 - m2 - M2/m3 - M3/m4 - M4 - P5 - P6 - m7 - M7/m8 - M8/m9 - M9 - P10
nonotonic genchain of sixths: ...E# - A# - F# - B# - G# - C - H - D - J - E - A - F - B - G - Cb - Hb - Db - Jb - Eb...
nonotonic genchain of sixths: ...M2 - M7 - M3 - M8 - M4 - M9 - P5 - P1 - P6 - m2 - m7 - m3 - m8 - m4 - m9...
Heptatonic 3rd-generated: D * E F * G A * B C * D (Smitonic generator = 3\11 = perfect 3rd)
D - D#/Eb - E - F - F#/Gb - G - A - A#/Bb - B - C - C#/Db - D
P1 - m2 - M2 - P3 - m4 - M4 - m5 - M5 - P6 - m7 - M7 - P8
genchain of thirds: ...E# - G# - B# - D# - F# - A# - C# - E - G - B - D - F - A - C - Eb - Gb - Bb - Db - Fb - Ab - Cb...
genchain of thirds: ...M5 - M7 - M2 - M4 - P6 - P1 - P3 - m5 - m7 - m2 - m4 - d6...
Hexatonic 2nd-generated: R * S * T * U * P Q * R (Machinoid generator = 2\11 = perfect 2nd)
R - R#/Sb - S - S#/Tb - T - T#/Ub - U - U#/Pb - P - Q - Q#/Rb - R
P1 - A1/d2 - P2 - m3 - M3 - m4 - M4 - m5 - M5 - P6 - A6/d7 - P7
genchain of seconds: ... - Qb - Rb - Sb - Tb - Ub - Pb - Q - R - S - T - U - P - Q# - R# - S# - T# - U# - P#...
genchain of seconds: ... - m3 - m4 - m5 - P6 - P1 - P2 - M3 - M4 - M5 - A6 - A1...
Sagittal notation
This notation is a subset of the notations for EDOs 22, 44, and 66.
Evo flavor
Revo flavor

Regular temperament properties
Uniform maps
| Min. size | Max. size | Wart notation | Map |
|---|---|---|---|
| 10.6744 | 10.8399 | 11cdeef | ⟨11 17 25 30 37 40] |
| 10.8399 | 10.8643 | 11cdf | ⟨11 17 25 30 38 40] |
| 10.8643 | 10.9446 | 11cf | ⟨11 17 25 31 38 40] |
| 10.9446 | 10.9823 | 11c | ⟨11 17 25 31 38 41] |
| 10.9823 | 11.0413 | 11 | ⟨11 17 26 31 38 41] |
| 11.0413 | 11.1290 | 11b | ⟨11 18 26 31 38 41] |
| 11.1290 | 11.2149 | 11be | ⟨11 18 26 31 39 41] |
Commas
11et tempers out the following commas. This assumes val ⟨11 17 26 31 38 41].
| Prime limit |
Ratio[note 1] | Monzo | Cents | Color name | Name(s) |
|---|---|---|---|---|---|
| 3 | 177147/131072 | [-17 11⟩ | 521.50 | sasawa 3rd | Pythagorean augmented third |
| 5 | 135/128 | [-7 3 1⟩ | 92.18 | Layobi | Major chroma |
| 5 | (16 digits) | [-25 7 6⟩ | 31.57 | Lala-tribiyo | Ampersand comma |
| 5 | (42 digits) | [-68 18 17⟩ | 2.52 | Quinla-seyo | Vavoom comma |
| 7 | (18 digits) | [-10 7 8 -7⟩ | 22.41 | Lasepru-aquadbiyo | Blackjackisma |
| 7 | 1029/1024 | [-10 1 0 3⟩ | 8.43 | Latrizo | Gamelisma |
| 7 | 225/224 | [-5 2 2 -1⟩ | 7.71 | Ruyoyo | Marvel comma |
| 7 | 16875/16807 | [0 3 4 -5⟩ | 6.99 | Quinru-aquadyo | Mirkwai comma |
| 7 | 2401/2400 | [-5 -1 -2 4⟩ | 0.72 | Bizozogu | Breedsma |
| 11 | 121/120 | [-3 -1 -1 0 2⟩ | 14.37 | Lologu | Biyatisma |
| 11 | 65536/65219 | [16 0 0 -2 -3⟩ | 8.39 | Satrilu-aruru | Orgonisma |
Approximation to JI
| Harmonic | 8 | 9 | 11 | 14 | 16 | ||||
|---|---|---|---|---|---|---|---|---|---|
| JI interval from 1/1 | 1/1 = 0 cents | 9/8 = 204 | 11/8 = 551 | 7/4 = 969 | 2/1 = 1200 | ||||
| Nearest 11edo interval | 0\11 = 0¢ | 2\11 = 218¢ | 5\11 = 545 | 9\11 = 982 | 11\11 = 1200 | ||||
| Difference | 0 | +14¢ | -6¢ | +13¢ | 0¢ | ||||
| JI interval between | 9:8 = 204¢ | 11:9 = 347 | 14:11 = 418 | 8:7 = 231 | |||||
| Nearest 11edo interval | 2\11 = 218¢ | 3\11 = 327 | 4\11 = 436 | 2\11 = 218 | |||||
| Difference | +14¢ | -20¢ | +18¢ | -13¢ |
11edo also may be considered a 2.7.9.11.15.17 subgroup temperament. See diagram:
Scales
MOS scales
Although 11edo has one fewer interval in the octave than 12edo, in terms of moment-of-symmetry scales, it offers a great deal more variety. This is because 11 is a prime number, while 12 is composite. Cycles of 2\11 (two degrees of 11edo), 3\11, 4\11 and 5\11 produce scales which do not repeat at the octave until all 11 intervals have been included.
Instruments
11edo ukulele
Ensembles
In February 2011, Oddmusic U-C, as part of its Microtonal Design Seminar, generated a 7-piece ensemble for playing music in 11edo. Instrumentation: autotuner, cümbüş, electronic keyboard, kalimba, retrofretted guitar, tuned bottles, udderbot. Recordings forthcoming.
Lumatone
There is a Lumatone mapping for 11edo.
Introductory Materials
Music
- See also: Category:11edo tracks
Strict 11edo
Modern renderings
- Bluin' The Black Keys (1926) – rendered by Francium (2024)
20th century
- First Piece Ever[dead link] (1970) — apparently the first piece ever written for 11edo.
- "The Turquoise Dabo Girl", from Xentonality (1997)
21st century
- Scatter Brain (2024)
- The Stuffed Ones (2004) – 4-piece suite (details)
- "Goopy" · "Ellie" · "Ziggy" · "Towelbear"
- Hyperimprovisations Nuggetwarp (2009)
- The City Sleeps, A Madrigal (2011) – play | SoundCloud
- Olive Flamenco (2019)
- "Tostadosto" from The Decatonic Album (2024) – Spotify | Bandcamp | YouTube
- "Sleep Slope" from XenRhythms (2024) – Spotify | Bandcamp | YouTube
- Cool My Head (2010)
- Orange Clips on Sausages (2004) – play | SoundClick
- Blue Gel (2004) – play | SoundClick
- conversation is (2010) – play | SoundClick
- Like Parker 3 (2019)
- Like 40s music (2022)
- From The Equal-Tempered Keyboard (1999–2022)
- "Prelude in 11ET"
- "Adagio in 11ET" – Bandcamp | SoundCloud[dead link]
- "Invention in 11ET" – Bandcamp | SoundCloud[dead link]
- Divertimento in 11 tone Orgone (2021) – sheet music | YouTube – orgone in 11edo tuning
- Sylvian Moon Dance (2021) – audio | sheet music | YouTube – orgone in 11edo tuning
- Ocean of the Necrophages (2021) – orgone in 11edo tuning
- Piano: audio | sheet music | YouTube
- Strings: audio | sheet music
- Ghost Bridge (2020)
- Micropiece in 11edo (2020)
- Prelude & Fugue in 11edo, in Four Parts, for Recorder, Organ, Cello (2022)
- George Secor · 11EDO improvisation (1971) (2022)
- Fire Memes (with Anthony "Pomp" Pompliano) – Machine[6] in 11edo tuning
- Theory of Creation – Machine[6] in 11edo tuning
- "Search Party" from No Fun House (2025) – Spotify | Bandcamp | YouTube
- Cursed Star (2024)
- "Overcoming", from Edolian (2020)
- 33322 (2024)
- Angkor Wat, September 1066 (2004)
- "Longwayaway People", from Rhythm and Xen (2015)
- "Make a Dream", from Rhythm and Xen (2015)
- Jaunt (2012) – play | YouTube
- Counterpoint in 11EDO[dead link]
- Jeffrey Dahmer Cooks at 11EDO (2011)
- The Metamorphosis of Gregor (2011)
- Eleven Birds (2012) – blog | play
- The Execution of 12 Equal[dead link]
- "10. 11 / octave", from Comets Over Flatland (2007)
- Icicle Caverns (2010) (score)
- 11 TET Hernya (2020)
- YG_A (2022)
Unequal Derivatives of 11edo
- 11 Tone March (2024)
Videos
- The Stuffed Ones: Goopy, Ziggy, Ellie, Towelbear by zipzappoozoo
- 11-equal Improvisation, Mike Battaglia - youtube
See also
- 11edo Zine — There is an 11edo Zine! As far as we know, 11edo is the first xenharmonic tuning system to have its own zine.
Notes
- ↑ Ratios longer than 10 digits are presented by placeholders with informative hints