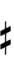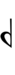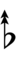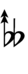44edo
| ← 43edo | 44edo | 45edo → |
44 equal divisions of the octave (abbreviated 44edo or 44ed2), also called 44-tone equal temperament (44tet) or 44 equal temperament (44et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 44 equal parts of about 27.3 ¢ each. Each step represents a frequency ratio of 21/44, or the 44th root of 2.
Theory
44edo is a double of 22edo, to which it adds the ratios of 13, 19, and 23. While not the most accurate 2.3.5.7.11 tuning, 22edo is certainly a relatively compact one, and it's natural to extend it this way. The most practically useful of these additions is easily the 13th harmonic with its neutral intervals, but the 17th, 19th, and 23rd are not to be dismissed.
It is on the optimal ET sequence for 7-, 11- and 13-limit nautilus temperament, for 11-limit spell temperament, and for 13-limit cantrip temperament. In the 13-limit it supplies the optimal patent val for vigin temperament.
The 2*44 subgroup of 44edo is 2.9.5.21.11.13.17.19.23, on which 44 tempers out the same commas as the patent val for 88edo.
Harmonics
| Harmonic | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 | 21 | 23 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +7.1 | -4.5 | +13.0 | -13.0 | -5.9 | +4.9 | +2.6 | +4.1 | +2.5 | -7.1 | -1.0 |
| Relative (%) | +26.2 | -16.5 | +47.6 | -47.7 | -21.5 | +18.1 | +9.7 | +15.2 | +9.1 | -26.2 | -3.7 | |
| Steps (reduced) |
70 (26) |
102 (14) |
124 (36) |
139 (7) |
152 (20) |
163 (31) |
172 (40) |
180 (4) |
187 (11) |
193 (17) |
199 (23) | |
Subsets and supersets
44edo has subsets 2, 4, 11, 22.
One step of 44edo is very close (only 0.0086 cents sharp) to 64/63 (the septimal comma). Ruthenium temperament realizes this proximity through a regular temperament perspective, and it is supported by a large number of edos which are a multiple of 44 - for example 1012edo, 1848edo, and 2684edo.
Intervals
In 44edo, sharps and flats alter pitch by 6 EDOsteps. This means intervals can be notated with half sharps and half flats equal to 3 EDOsteps, in addition to ups and downs. The table below uses only sharps, flats, and ups and downs. When translating music from 22edo to 44edo, single ups and downs simply become double ups and downs (vEb in 22edo would be vvEb in 44edo).
| Degrees | Cents | Ups and downs notation | ||
|---|---|---|---|---|
| 0 | 0.000 | Perfect 1sn | P1 | D |
| 1 | 27.273 | Up 1sn | ^1 | ^D |
| 2 | 54.545 | Minor 2nd | m2 | Eb |
| 3 | 81.818 | Upminor 2nd | ^m2 | ^Eb |
| 4 | 109.091 | Dupminor 2nd, Downmid 2nd | ^^m2, v~2 | ^^Eb |
| 5 | 136.364 | Mid 2nd | ~2 | vvvE, ^^^Eb |
| 6 | 163.636 | Dudmajor 2nd, Upmid 2nd | vvM2, ^~2 | vvE |
| 7 | 190.909 | Downmajor 2nd | vM2 | vE |
| 8 | 218.182 | Major 2nd | M2 | E |
| 9 | 245.455 | Upmajor 2nd, Downminor 3rd | ^M2, vm3 | ^E, vF |
| 10 | 272.727 | Minor 3rd | m3 | F |
| 11 | 300.000 | Upminor 3rd | ^m3 | ^F |
| 12 | 327.273 | Dupminor 3rd, Downmid 3rd | ^^m3, v~3 | ^^F |
| 13 | 354.545 | Mid 3rd | ~3 | ^^^F, vvvF# |
| 14 | 381.818 | Dudmajor 3rd, Upmid 3rd | vvM3, ^~3 | vvF# |
| 15 | 409.091 | Downmajor 3rd | vM3 | vF# |
| 16 | 436.364 | Major 3rd | M3 | F# |
| 17 | 463.636 | Upmajor 3rd, Down 4th | ^M3, v4 | ^F#, vG |
| 18 | 490.909 | Perfect 4th | P4 | G |
| 19 | 518.182 | Up 4th | ^4 | ^G |
| 20 | 545.455 | Dup 4th, Downmid 4th, Dim 5th | ^^4, v~4, d5 | Ab, ^^G |
| 21 | 572.727 | Mid 4th, Updim 5th | ~4, ^d5 | ^^^G, vvvG# |
| 22 | 600.000 | Upmid 4th, Downmid 5th | ^~4, v~5 | vvG#, ^^Ab |
| 23 | 627.273 | Downaug 4th, Mid 5th | vA4, ~5 | vvvA, ^^^Ab |
| 24 | 654.545 | Aug 4th, Upmid 5th, Dud 5th | A4, ^~5, vv5 | G#, vvA |
| 25 | 681.818 | Down 5th | v5 | vA |
| 26 | 709.091 | Perfect 5th | P5 | A |
| 27 | 736.364 | Up 5th, Downminor 6th | ^5, vm6 | ^A, vBb |
| 28 | 763.636 | Minor 6th | m6 | Bb |
| 29 | 790.909 | Upminor 6th | ^m6 | ^Bb |
| 30 | 818.182 | Dupminor 6th, Downmid 6th | ^^m6, v~6 | ^^Bb |
| 31 | 845.455 | Mid 6th | ~6 | ^^^Bb, vvvB |
| 32 | 872.727 | Dudmajor 6th, Upmid 6th | vvM6, ^~6 | vvB |
| 33 | 900.000 | Downmajor 6th | vM6 | vB |
| 34 | 927.273 | Major 6th | M6 | B |
| 35 | 954.545 | Upmajor 6th, Downminor 7th | ^M6, vm7 | ^B, vC |
| 36 | 981.818 | Minor 7th | m7 | C |
| 37 | 1009.091 | Upminor 7th | ^m7 | ^C |
| 38 | 1036.364 | Dupminor 7th, Downmid 7th | ^^m7, v~7 | ^^C |
| 39 | 1063.636 | Mid 7th | ~7 | ^^^C, vvvC# |
| 40 | 1090.909 | Dudmajor 7th, Upmid 7th | vvM7, ^~7 | vvC# |
| 41 | 1118.182 | Downmajor 7th | vM7 | vC# |
| 42 | 1145.455 | Major 7th | M7 | C# |
| 43 | 1172.727 | Upmajor 7th, Down 8ve | ^M7, v8 | ^C#, vD |
| 44 | 1200.000 | Perfect 8ve | P8 | D |
Notation
Ups and downs notation
44edo can be notated with ups and downs, spoken as up, dup, trup, dudsharp, downsharp, sharp, upsharp etc. and down, dud, trud, dupflat etc.
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |||||||||||||
| Flat symbol |
Half-sharps and half-flats can be used to avoid triple arrows:
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |||||||||||||
| Flat symbol |
Alternative ups and downs have sharps and flats with arrows borrowed from extended Helmholtz–Ellis notation:
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| Flat symbol | |
|
|
|
|
|
|
|
|
|
|
|
|
|
If double arrows are not desirable, arrows can be attached to quarter-tone accidentals:
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |
|
|
|
|
|
|
|
|
|
|
|
| |
| Flat symbol | |
|
|
|
|
|
|
|
|
|
|
|
|
Sagittal notation
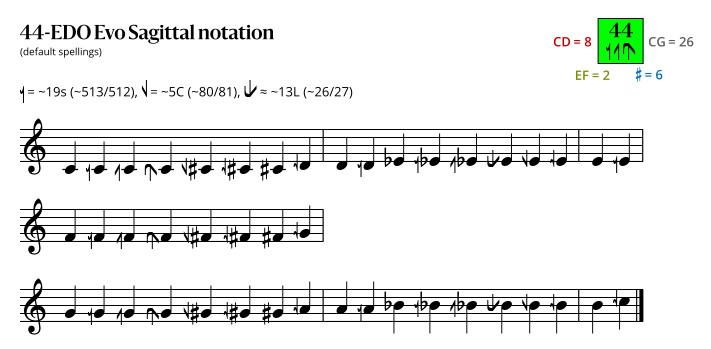
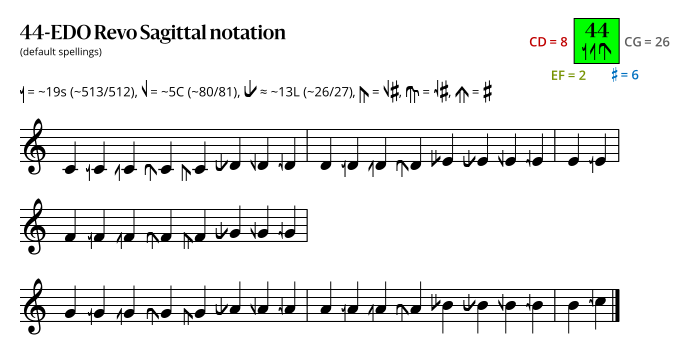
This notation uses the same sagittal sequence as EDOs 23b, 30, and 37, and is a superset of the notations for EDOs 22 and 11.
Evo flavor
Revo flavor
Evo-SZ flavor
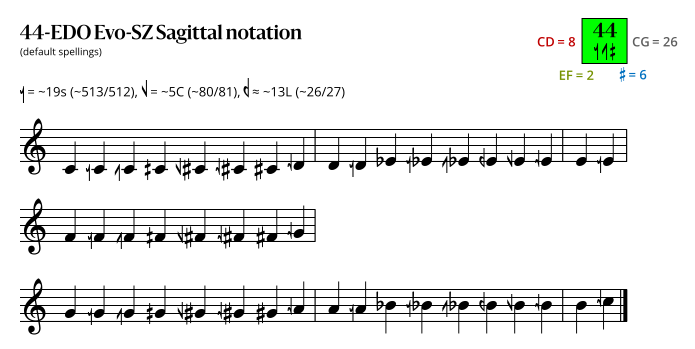
In the diagrams above, a sagittal symbol followed by an equals sign (=) means that the following comma is the symbol's primary comma (the comma it exactly represents in JI), while an approximately equals sign (≈) means it is a secondary comma (a comma it approximately represents in JI). In both cases the symbol exactly represents the tempered version of the comma in this EDO.
Regular temperament properties
Rank-2 temperaments
| Periods per 8ve |
Generator* | Cents* | Associated ratio* |
Temperaments |
|---|---|---|---|---|
| 1 | 3\44 | 81.82 | 22/21 | Nautilus |
| 1 | 7\44 | 190.91 | 9/8 | Spell/cantrip |
| 1 | 9\44 | 245.46 | 15/13 | Immunity |
| 1 | 13\44 | 354.55 | 11/9 | Ringo |
| 1 | 15\44 | 409.09 | 5/4 | Hocus |
| 2 | 3\44 | 81.82 | 22/21 | Harry |
| 4 | 4\44 | 109.09 | 16/15 | Bidia |
| 11 | 2\44 | 54.55 | 33/32 | Hendecatonic |
* Octave-reduced form, reduced to the first half-octave
Scales
- Evacuated planet[idiosyncratic term] (approximated from 66afdo): 5 13 8 12 6
- Approximations of gamelan scales:
- 5-tone pelog: 4 6 15 4 15
- 7-tone pelog: 4 6 9 6 4 10 5
- 5-tone slendro: 9 9 8 9 9
Instrument layouts
Music
- Improvisation in 44edo - composed and played by Bryan Deister (May 2023), transcribed by Stephen Weigel (Sept 2024)
- Leaning Dream - Pizza Tower - adapted into 44edo by Bryan Deister (July 2024)
- 44edo improvisation - Bryan Deister (Oct 2024)