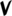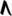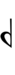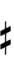45edo
| ← 44edo | 45edo | 46edo → |
45 equal divisions of the octave (abbreviated 45edo or 45ed2), also called 45-tone equal temperament (45tet) or 45 equal temperament (45et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 45 equal parts of about 26.7 ¢ each. Each step represents a frequency ratio of 21/45, or the 45th root of 2.
Theory
45edo effectively has two approximate major thirds, each almost equally far from just, but as the flat one is slightly closer, it qualifies as a meantone temperament, forming a good approximation to 2/5-comma meantone. It is a flat-tending system in the 7-limit, with 3, 5, and 7 all flat, but the 11 is sharp.
It provides the optimal patent val for flattone temperament, 7-limit rank-3 avicennmic temperament tempering out 525/512, the 11-limit calliope temperament tempering out 45/44 and 81/80, and the rank-4 temperament tempering out 45/44. It tempers out 81/80, 3125/3087, 525/512, 875/864 and 45/44. It is also the unique equal temperament tuning whose patent val tempers out both the syntonic comma and the ennealimma.
45edo tempers out the quartisma and provides an excellent tuning for the 2.7/3.33 subgroup direct quartismic temperament, in which it approximates the 33/32 quartertone with 2 steps and 7/6 with 10 steps. A bit more broadly, it maps the 2.17.25.27.33.63.65 subgroup to great precision; this is the part of the 17-limit shared with 270edo.
Otherwise, it can be treated as a 2.5/3.7/3 subgroup system (borrowing 5/3 from 15edo and 7/3 from 9edo) and is a good tuning for gariberttet, defined by tempering out 3125/3087 in this subgroup, approximating 2/5-comma gariberttet.
Odd harmonics
| Harmonic | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 | 21 | 23 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -8.6 | -13.0 | -8.8 | +9.4 | +8.7 | +12.8 | +5.1 | +1.7 | -4.2 | +9.2 | +11.7 |
| Relative (%) | -32.3 | -48.7 | -33.1 | +35.3 | +32.6 | +48.0 | +19.0 | +6.4 | -15.7 | +34.6 | +44.0 | |
| Steps (reduced) |
71 (26) |
104 (14) |
126 (36) |
143 (8) |
156 (21) |
167 (32) |
176 (41) |
184 (4) |
191 (11) |
198 (18) |
204 (24) | |
Intervals
| Step # | ET | Just (JI) | Error (ET−JI) |
Ups and downs notation | ||||
|---|---|---|---|---|---|---|---|---|
| Cents | Interval | Cents | ||||||
| 0 | 0.000 | 1/1 | 0.000 | 0.000 | Perfect Unison | P1 | D | |
| 1 | 26.666 | 65/64 | 26.841 | -0.174 | Up unison | ^1 | ^D | |
| 2 | 53.333 | 33/32 | 53.273 | 0.060 | Augmented Unison | A1 | D# | |
| 3 | 80.000 | 22/21 | 80.537 | -0.537 | Diminished 2nd | d2 | Ebb | |
| 4 | 106.666 | 17/16 | 104.955 | 1.711 | Downminor 2nd | vm2 | vEb | |
| 5 | 133.333 | 27/25 | 133.238 | 0.095 | Minor 2nd | m2 | Eb | |
| 6 | 160.000 | 11/10 | 165.004 | -5.004 | Mid 2nd | ~2 | vE | |
| 7 | 186.666 | 10/9 | 182.404 | 4.262 | Major 2nd | M2 | E | |
| 8 | 213.333 | 9/8 | 203.910 | 9.423 | Upmajor 2nd | ^M2 | ^E | |
| 9 | 240.000 | 8/7 | 231.174 | 8.826 | Augmented 2nd | A2 | E# | |
| 10 | 266.666 | 7/6 | 266.871 | -0.205 | Diminished 3rd | d3 | Fb | |
| 11 | 293.333 | 32/27 | 294.135 | -0.802 | Downminor 3rd | vm3 | vF | |
| 12 | 320.000 | 6/5 | 315.641 | 4.359 | Minor 3rd | m3 | F | |
| 13 | 346.666 | 11/9 | 347.408 | -0.741 | Mid 3rd | ~3 | ^F | |
| 14 | 373.333 | 5/4 | 386.314 | -12.980 | Major 3rd | M3 | F# | |
| 15 | 400.000 | 63/50 | 400.108 | -0.108 | Upmajor 3rd | ^M3 | ^F# | |
| 16 | 426.666 | 9/7 | 435.084 | -8.418 | Augmented 3rd | A3 | Fx | |
| 17 | 453.333 | 13/10 | 454.294 | -0.961 | Diminished 4th | d4 | Gb | |
| 18 | 480.000 | 21/16 | 470.781 | 9.219 | Down 4th | v4 | vG | |
| 19 | 506.666 | 4/3 | 498.045 | 8.622 | Perfect 4th | P4 | G | |
| 20 | 533.333 | 49/36 | 533.742 | -0.409 | Up 4th or Mid 4th | ^4, ~4 | ^G | |
| 21 | 560.000 | 18/13 | 563.382 | -3.382 | Augmented 4th | A4 | G# | |
| 22 | 586.666 | 7/5 | 582.512 | 4.155 | Upaugmented 4th | ^A4 | ^G# | |
| 23 | 613.333 | 10/7 | 617.488 | -4.155 | Downdiminshed 5th | vd5 | vAb | |
| 24 | 640.000 | 13/9 | 636.618 | 3.382 | Diminished 5th | d5 | Ab | |
| 25 | 666.666 | 72/49 | 666.258 | 0.409 | Down 5th or Mid 5th | v5, ~5 | vA | |
| 26 | 693.333 | 3/2 | 701.955 | -8.622 | Perfect 5th | P5 | A | |
| 27 | 720.000 | 32/21 | 729.219 | -9.219 | Up 5th | ^5 | ^A | |
| 28 | 746.666 | 20/13 | 745.786 | 0.961 | Augmented 5th | A5 | A# | |
| 29 | 773.333 | 14/9 | 764.916 | 8.418 | Diminished 6th | d6 | Bbb | |
| 30 | 800.000 | 100/63 | 799.892 | 0.108 | Downminor 6th | vm6 | vBb | |
| 31 | 826.666 | 8/5 | 813.686 | 12.980 | Minor 6th | m6 | Bb | |
| 32 | 853.333 | 18/11 | 852.592 | 0.741 | Mid 6th | ~6 | vB | |
| 33 | 880.000 | 5/3 | 884.359 | -4.359 | Major 6th | M6 | B | |
| 34 | 906.666 | 27/16 | 905.865 | 0.802 | Upmajor 6th | ^M6 | ^B | |
| 35 | 933.333 | 12/7 | 933.129 | 0.205 | Augmented 6th | A6 | B# | |
| 36 | 960.000 | 7/4 | 968.826 | -8.826 | Diminished 7th | d7 | Cb | |
| 37 | 986.666 | 16/9 | 996.089 | -9.423 | Downminor 7th | vm7 | vC | |
| 38 | 1013.333 | 9/5 | 1017.596 | -4.262 | Minor 7th | m7 | C | |
| 39 | 1040.000 | 20/11 | 1034.996 | 5.004 | Mid 7th | ~7 | ^C | |
| 40 | 1066.666 | 50/27 | 1066.762 | -0.095 | Major 7th | M7 | C# | |
| 41 | 1093.333 | 32/17 | 1095.044 | -1.711 | Upmajor 7th | ^M7 | ^C# | |
| 42 | 1120.000 | 21/11 | 1119.463 | 0.537 | Augmented 7th | A7 | Cx | |
| 43 | 1146.666 | 64/33 | 1146.727 | -0.060 | Diminished 8ve | d8 | Db | |
| 44 | 1173.333 | 128/65 | 1173.158 | 0.174 | Down 8ve | v8 | vD | |
| 45 | 1200.000 | 2/1 | 1200.000 | 0.000 | Perfect Octave | P8 | D | |
Notation
Ups and Downs notation
Spoken as up, sharp, upsharp, etc. Note that up can be respelled as downsharp.
| Step offset | −4 | −3 | −2 | −1 | 0 | +1 | +2 | +3 | +4 |
|---|---|---|---|---|---|---|---|---|---|
| Symbol | |
|
|
|
|
|
|
|
|
Quarter-tone notation
Since a sharp raises by two steps, quarter-tone accidentals can also be used.
| Step offset | −4 | −3 | −2 | −1 | 0 | +1 | +2 | +3 | +4 |
|---|---|---|---|---|---|---|---|---|---|
| Symbol | |
|
|
|
|
|
|
|
|
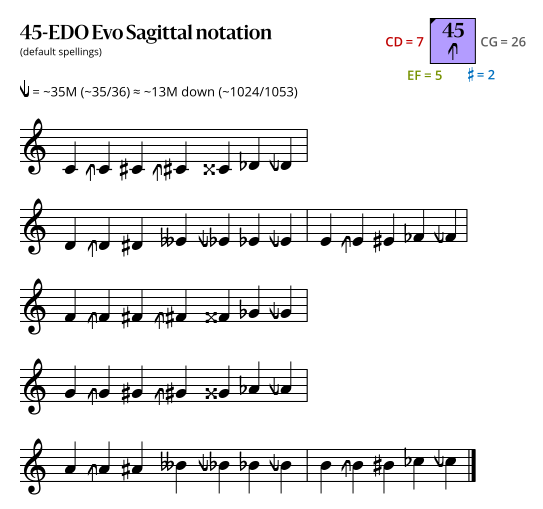
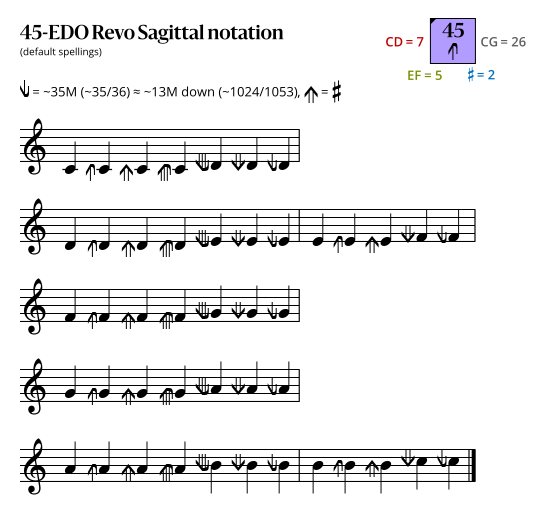
Sagittal notation
This notation uses the same sagittal sequence as EDOs 52 and 59b.
Evo flavor
Revo flavor
Evo-SZ flavor
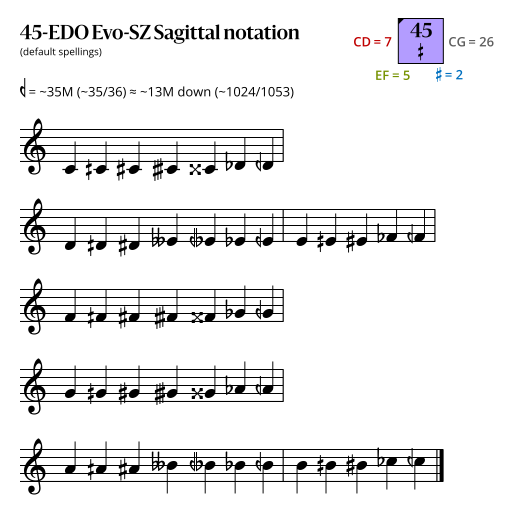
Because it contains no Sagittal symbols, this Evo-SZ Sagittal notation is also a Stein-Zimmerman notation.
In the following diagrams, a sagittal symbol followed by an equals sign (=) means that the following comma is the symbol's primary comma (the comma it exactly represents in JI), while an approximately equals sign (≈) means it is a secondary comma (a comma it approximately represents in JI). In both cases the symbol exactly represents the tempered version of the comma in this EDO.
Regular temperament properties
Commas
This is a partial list of the commas that 45et tempers out with its patent val, ⟨45 71 104 126 143 156 167].
| Prime limit |
Ratio[note 1] | Monzo | Cents | Color name | Name(s) |
|---|---|---|---|---|---|
| 5 | 81/80 | [-4 4 -1⟩ | 21.51 | Gu | Syntonic comma, Didymus comma, meantone comma |
| 5 | (26 digits) | [1 -27 18⟩ | 0.86 | Satritribiyo | Ennealimma |
| 7 | 16807/16384 | [-14 0 0 5⟩ | 44.13 | Laquinzo | Cloudy comma |
| 7 | 525/512 | [-9 1 2 1⟩ | 43.41 | Lazoyoyo | Avicennma, Avicenna's enharmonic diesis |
| 7 | 875/864 | [-5 -3 3 1⟩ | 21.90 | Zotrigu | Keema |
| 7 | 3125/3087 | [0 -2 5 -3⟩ | 21.18 | Triru-aquinyo | Gariboh comma |
| 7 | (16 digits) | [-11 -9 0 9⟩ | 1.84 | Tritrizo | Septimal ennealimma |
| 7 | 4375/4374 | [-1 -7 4 1⟩ | 0.40 | Zoquadyo | Ragisma |
| 11 | 45/44 | [-2 2 1 0 -1⟩ | 38.91 | Luyo | Undecimal 1/5-tone |
| 11 | 385/384 | [-7 -1 1 1 1⟩ | 4.50 | Lozoyo | Keenanisma |
| 11 | (18 digits) | [24 -6 0 1 -5⟩ | 0.51 | Saquinlu-azo | Quartisma |
- ↑ Ratios longer than 10 digits are presented by placeholders with informative hints.
Octave stretch and compression
45edo's approximations of 3/1, 5/1, 7/1, 11/1 and 13/1 and 17/1 are all improved by an stretched-octave version of 45edo, such as 116ed6. The trade-off is a slightly worse 2/1.
The tuning 183ed17 may be used for this purpose too, it improves 3/1, 5/1, 7/1, 11/1, 13/1 and 17/1, but at the cost of a noticeably worse 2/1 than 116ed6.
What follows is a comparison of compressed- and stretched-octave 45edo tunings.
- Step size: 26.550 ¢, octave size: 1194.8 ¢
Compressing the octave of 45edo by around 5 ¢ results in improved primes 5 and 7, but worse primes 2, 3, 11 and 13. This approximates all harmonics up to 16 within 11.1 ¢. The tuning 209zpi does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -5.2 | +9.6 | -10.5 | +1.4 | +4.4 | +3.0 | +10.8 | -7.3 | -3.8 | -9.5 | -0.9 |
| Relative (%) | -19.8 | +36.3 | -39.5 | +5.4 | +16.6 | +11.4 | +40.7 | -27.3 | -14.4 | -35.8 | -3.2 | |
| Step | 45 | 72 | 90 | 105 | 117 | 127 | 136 | 143 | 150 | 156 | 162 | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -6.7 | -2.2 | +11.1 | +5.6 | +6.8 | -12.5 | +0.1 | -9.1 | +12.7 | +11.8 | -12.1 | -6.1 |
| Relative (%) | -25.2 | -8.4 | +41.7 | +20.9 | +25.6 | -47.1 | +0.3 | -34.1 | +47.7 | +44.4 | -45.5 | -23.0 | |
| Step | 167 | 172 | 177 | 181 | 185 | 188 | 192 | 195 | 199 | 202 | 204 | 207 | |
- 45edo
- Step size: 26.667 ¢, octave size: 1200.0 ¢
Pure-octaves 45edo approximates all harmonics up to 16 within 13.0 ¢.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +0.0 | -8.6 | +0.0 | -13.0 | -8.6 | -8.8 | +0.0 | +9.4 | -13.0 | +8.7 | -8.6 |
| Relative (%) | +0.0 | -32.3 | +0.0 | -48.7 | -32.3 | -33.1 | +0.0 | +35.3 | -48.7 | +32.6 | -32.3 | |
| Steps (reduced) |
45 (0) |
71 (26) |
90 (0) |
104 (14) |
116 (26) |
126 (36) |
135 (0) |
143 (8) |
149 (14) |
156 (21) |
161 (26) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +12.8 | -8.8 | +5.1 | +0.0 | +1.7 | +9.4 | -4.2 | -13.0 | +9.2 | +8.7 | +11.7 | -8.6 |
| Relative (%) | +48.0 | -33.1 | +19.0 | +0.0 | +6.4 | +35.3 | -15.7 | -48.7 | +34.6 | +32.6 | +44.0 | -32.3 | |
| Steps (reduced) |
167 (32) |
171 (36) |
176 (41) |
180 (0) |
184 (4) |
188 (8) |
191 (11) |
194 (14) |
198 (18) |
201 (21) |
204 (24) |
206 (26) | |
- Step size: 26.695 ¢, octave size: 1201.3 ¢
Stretching the octave of 45edo by around 1 ¢ results in improved primes 3, 5, 7 and 13, but worse primes 2 and 11. This approximates all harmonics up to 16 within 13.2 ¢. Its 13-limit WE tuning and 13-limit TE tuning both do this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +1.3 | -6.6 | +2.5 | -10.0 | -5.3 | -5.3 | +3.8 | -13.2 | -8.8 | +13.1 | -4.1 |
| Relative (%) | +4.8 | -24.8 | +9.6 | -37.6 | -20.0 | -19.7 | +14.3 | -49.5 | -32.8 | +49.1 | -15.2 | |
| Step | 45 | 71 | 90 | 104 | 116 | 126 | 135 | 142 | 149 | 156 | 161 | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -9.2 | -4.0 | +10.1 | +5.1 | +6.9 | -11.9 | +1.2 | -7.5 | -11.9 | -12.3 | -9.2 | -2.8 |
| Relative (%) | -34.3 | -14.9 | +37.7 | +19.1 | +25.9 | -44.7 | +4.6 | -28.0 | -44.4 | -46.1 | -34.4 | -10.4 | |
| Step | 166 | 171 | 176 | 180 | 184 | 187 | 191 | 194 | 197 | 200 | 203 | 206 | |
- Step size: Octave size: 1202.4 ¢
Stretching the octave of 45edo by around 2.5 ¢ results in improved primes 3, 5, 7 and 13, but worse primes 2 and 11. This approximates all harmonics up to 16 within 12.2 ¢. The tuning 161ed12 does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +2.4 | -4.8 | +4.8 | -7.4 | -2.4 | -2.1 | +7.2 | -9.6 | -5.0 | -9.7 | +0.0 |
| Relative (%) | +9.0 | -18.0 | +18.0 | -27.7 | -9.0 | -7.8 | +27.1 | -36.1 | -18.7 | -36.2 | +0.0 | |
| Steps (reduced) |
45 (45) |
71 (71) |
90 (90) |
104 (104) |
116 (116) |
126 (126) |
135 (135) |
142 (142) |
149 (149) |
155 (155) |
161 (0) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -5.0 | +0.3 | -12.2 | +9.6 | +11.6 | -7.2 | +6.0 | -2.6 | -6.9 | -7.3 | -4.1 | +2.4 |
| Relative (%) | -18.6 | +1.2 | -45.8 | +36.1 | +43.3 | -27.1 | +22.6 | -9.7 | -25.8 | -27.2 | -15.2 | +9.0 | |
| Steps (reduced) |
166 (5) |
171 (10) |
175 (14) |
180 (19) |
184 (23) |
187 (26) |
191 (30) |
194 (33) |
197 (36) |
200 (39) |
203 (42) |
206 (45) | |
- Step size: Octave size: 1203.3 ¢
Stretching the octave of 45edo by around 3 ¢ results in improved primes 3, 5, 7, 11 and 13, but a worse prime 2. This approximates all harmonics up to 16 within 13.4 ¢. The tuning 116ed6 does this. So does 126ed7 whose octave is identical within 0.1 ¢.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +3.3 | -3.3 | +6.7 | -5.3 | +0.0 | +0.5 | +10.0 | -6.7 | -1.9 | -6.5 | +3.3 |
| Relative (%) | +12.5 | -12.5 | +25.0 | -19.6 | +0.0 | +2.0 | +37.5 | -25.0 | -7.1 | -24.2 | +12.5 | |
| Steps (reduced) |
45 (45) |
71 (71) |
90 (90) |
104 (104) |
116 (0) |
126 (10) |
135 (19) |
142 (26) |
149 (33) |
155 (39) |
161 (45) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -1.5 | +3.9 | -8.6 | -13.4 | -11.4 | -3.3 | +10.0 | +1.4 | -2.8 | -3.1 | +0.1 | +6.7 |
| Relative (%) | -5.7 | +14.5 | -32.1 | -50.0 | -42.5 | -12.5 | +37.5 | +5.4 | -10.5 | -11.7 | +0.5 | +25.0 | |
| Steps (reduced) |
166 (50) |
171 (55) |
175 (59) |
179 (63) |
183 (67) |
187 (71) |
191 (75) |
194 (78) |
197 (81) |
200 (84) |
203 (87) |
206 (90) | |
- Step size: 26.745 ¢, octave size: 1203.5 ¢
Stretching the octave of 45edo by around 3.5 ¢ results in improved primes 3, 5, 7, 11 and 13, but a worse prime 2. This approximates all harmonics up to 16 within 12.6 ¢. Its 7-limit WE tuning and 7-limit TE tuning both do this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +3.5 | -3.1 | +7.1 | -4.8 | +0.5 | +1.0 | +10.6 | -6.1 | -1.3 | -5.8 | +4.0 |
| Relative (%) | +13.2 | -11.4 | +26.4 | -18.1 | +1.7 | +3.9 | +39.5 | -22.9 | -4.9 | -21.8 | +14.9 | |
| Step | 45 | 71 | 90 | 104 | 116 | 126 | 135 | 142 | 149 | 155 | 161 | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -0.9 | +4.6 | -7.9 | -12.6 | -10.6 | -2.6 | +10.8 | +2.2 | -2.0 | -2.3 | +1.0 | +7.5 |
| Relative (%) | -3.2 | +17.1 | -29.5 | -47.3 | -39.7 | -9.7 | +40.3 | +8.3 | -7.5 | -8.7 | +3.6 | +28.1 | |
| Step | 166 | 171 | 175 | 179 | 183 | 187 | 191 | 194 | 197 | 200 | 203 | 206 | |
- Step size: 26.762 ¢, octave size: 1204.3 ¢
Stretching the octave of 45edo by around 4 ¢ results in improved primes 3, 5, 7, 11 and 13, but a worse prime 2. This approximates all harmonics up to 16 within 12.9 ¢. The tuning 207zpi does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +4.3 | -1.9 | +8.6 | -3.1 | +2.4 | +3.2 | +12.9 | -3.7 | +1.2 | -3.2 | +6.7 |
| Relative (%) | +16.0 | -6.9 | +32.1 | -11.5 | +9.1 | +11.9 | +48.1 | -13.8 | +4.6 | -12.0 | +25.1 | |
| Step | 45 | 71 | 90 | 104 | 116 | 126 | 135 | 142 | 149 | 155 | 161 | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +2.0 | +7.5 | -4.9 | -9.6 | -7.5 | +0.6 | -12.7 | +5.5 | +1.3 | +1.1 | +4.4 | +11.0 |
| Relative (%) | +7.3 | +27.9 | -18.4 | -35.9 | -28.1 | +2.2 | -47.6 | +20.6 | +5.0 | +4.0 | +16.5 | +41.2 | |
| Step | 166 | 171 | 175 | 179 | 183 | 187 | 190 | 194 | 197 | 200 | 203 | 206 | |
- Step size: 26.788 ¢, octave size: 1205.5 ¢
Stretching the octave of 45edo by around 5.5 ¢ results in improved primes 3, 5, 7, 11 and 13, but a worse prime 2. This approximates all harmonics up to 16 within 11.9 ¢. The tuning 71edt does this. So do the tunings 104ed5 and 155ed11 whose octave is identical within 0.3 ¢.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +5.5 | +0.0 | +10.9 | -0.4 | +5.5 | +6.5 | -10.4 | +0.0 | +5.1 | +0.8 | +10.9 |
| Relative (%) | +20.4 | +0.0 | +40.8 | -1.3 | +20.4 | +24.2 | -38.8 | +0.0 | +19.1 | +3.1 | +40.8 | |
| Steps (reduced) |
45 (45) |
71 (0) |
90 (19) |
104 (33) |
116 (45) |
126 (55) |
134 (63) |
142 (0) |
149 (7) |
155 (13) |
161 (19) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +6.3 | +11.9 | -0.4 | -4.9 | -2.7 | +5.5 | -7.8 | +10.6 | +6.5 | +6.3 | +9.7 | -10.4 |
| Relative (%) | +23.5 | +44.6 | -1.3 | -18.4 | -10.2 | +20.4 | -29.0 | +39.5 | +24.2 | +23.5 | +36.2 | -38.8 | |
| Steps (reduced) |
166 (24) |
171 (29) |
175 (33) |
179 (37) |
183 (41) |
187 (45) |
190 (48) |
194 (52) |
197 (55) |
200 (58) |
203 (61) |
205 (63) | |
- Octave size: 1206.1 ¢
Stretching the octave of 45edo by around 6 ¢ results in improved primes 3, 5, 7, 11, 13 and 17, but a worse prime 2. This approximates all harmonics up to 16 within 13.3 ¢. The tuning 183ed17 does this.
| Harmonic | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +6.1 | +1.1 | +12.3 | +1.2 | +7.2 | +8.4 | -8.4 | +2.1 | +7.3 | +3.2 | +13.3 |
| Relative (%) | +22.9 | +4.0 | +45.8 | +4.5 | +26.9 | +31.2 | -31.3 | +7.9 | +27.4 | +11.8 | +49.7 | |
| Steps (reduced) |
45 (45) |
71 (71) |
90 (90) |
104 (104) |
116 (116) |
126 (126) |
134 (134) |
142 (142) |
149 (149) |
155 (155) |
161 (161) | |
| Harmonic | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +8.8 | -12.3 | +2.3 | -2.3 | +0.0 | +8.3 | -4.9 | -13.3 | +9.4 | +9.3 | +12.7 | -7.3 |
| Relative (%) | +32.7 | -45.9 | +8.4 | -8.4 | +0.0 | +30.8 | -18.4 | -49.7 | +35.1 | +34.7 | +47.5 | -27.4 | |
| Steps (reduced) |
166 (166) |
170 (170) |
175 (175) |
179 (179) |
183 (0) |
187 (4) |
190 (7) |
193 (10) |
197 (14) |
200 (17) |
203 (20) |
205 (22) | |
Instruments
Lumatone
See Lumatone mapping for 45edo
Music
- Fishbowl (2023)
- Archipelago Arpeggio (2024)
- Fallen Angel (2024)
- Solar Guardian (2024)