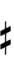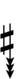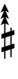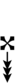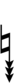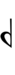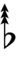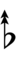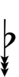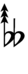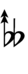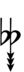89edo
| ← 88edo | 89edo | 90edo → |
89 equal divisions of the octave (abbreviated 89edo or 89ed2), also called 89-tone equal temperament (89tet) or 89 equal temperament (89et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 89 equal parts of about 13.5 ¢ each. Each step represents a frequency ratio of 21/89, or the 89th root of 2.
Theory
89edo has a harmonic 3 less than a cent flat and a harmonic 5 less than five cents sharp, with a 7 two cents sharp and an 11 1.5 cents sharp. It thus delivers reasonably good 11-limit harmony and very good no-fives harmony along with the very useful approximations represented by its commas. On a related note, a notable characteristic of this edo is that it is the lowest in a series of four consecutive edos to temper out quartisma.
It tempers out 32805/32768 (schisma) in the 5-limit; 126/125, 1728/1715, and 2401/2400 in the 7-limit; and 176/175, 243/242, 441/440 and 540/539 in the 11-limit. It is an especially good tuning for the myna temperament, both in the 7-limit, tempering out 126/125 and 1728/1715, and in the 11-limit, where 176/175 is tempered out also. It is likewise a good tuning for the rank-3 temperament thrush, tempering out 126/125 and 176/175.
The 13-limit is a little tricky as 13 is tuned distinctly flat, tempering out 169/168, 364/363, 729/728, 832/825, and 1287/1280. 13/10 and 15/13 are particularly out of tune in this system, each being about 9 cents off. The alternative 89f val fixes that but tunes 13/8 much sharper, conflating it with 18/11. It tempers out 144/143, 196/195, 351/350, and 352/351 instead, and supports 13-limit myna and thrush. However, 58edo is a better tuning for those purposes.
The 17 and 19 are tuned fairly well, making it consistent to the no-13 21-odd-limit. The equal temperament tempers out 256/255 and 561/560 in the 17-limit; and 171/170, 361/360, 513/512, and 1216/1215 in the 19-limit.
89edo is the 11th in the Fibonacci sequence, which means its 55th step approximates logarithmic φ (i.e. 1200(φ − 1) ¢ within a fraction of a cent.
Prime harmonics
| Harmonic | 2 | 3 | 5 | 7 | 11 | 13 | 17 | 19 | 23 | 29 | 31 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +0.00 | -0.83 | +4.70 | +1.96 | +1.49 | -4.57 | +2.91 | -0.88 | +5.43 | -4.86 | +1.03 |
| Relative (%) | +0.0 | -6.2 | +34.8 | +14.5 | +11.1 | -33.9 | +21.6 | -6.6 | +40.3 | -36.0 | +7.7 | |
| Steps (reduced) |
89 (0) |
141 (52) |
207 (29) |
250 (72) |
308 (41) |
329 (62) |
364 (8) |
378 (22) |
403 (47) |
432 (76) |
441 (85) | |
Subsets and supersets
89edo is the 24th prime edo, following 83edo and before 97edo.
Intervals
| Steps | Cents | Approximate ratios | Ups and downs notation |
|---|---|---|---|
| 0 | 0 | 1/1 | D |
| 1 | 13.5 | ^D, ^^E♭♭ | |
| 2 | 27 | ^^D, ^3E♭♭ | |
| 3 | 40.4 | 41/40, 42/41 | ^3D, v4E♭ |
| 4 | 53.9 | 31/30, 32/31, 33/32, 34/33 | ^4D, v3E♭ |
| 5 | 67.4 | 27/26 | v3D♯, vvE♭ |
| 6 | 80.9 | 22/21 | vvD♯, vE♭ |
| 7 | 94.4 | 19/18, 37/35 | vD♯, E♭ |
| 8 | 107.9 | 33/31 | D♯, ^E♭ |
| 9 | 121.3 | 15/14, 29/27 | ^D♯, ^^E♭ |
| 10 | 134.8 | 40/37 | ^^D♯, ^3E♭ |
| 11 | 148.3 | 12/11, 37/34 | ^3D♯, v4E |
| 12 | 161.8 | 34/31 | ^4D♯, v3E |
| 13 | 175.3 | 21/19, 31/28, 41/37 | v3D𝄪, vvE |
| 14 | 188.8 | 29/26 | vvD𝄪, vE |
| 15 | 202.2 | 9/8 | E |
| 16 | 215.7 | 17/15 | ^E, ^^F♭ |
| 17 | 229.2 | 8/7 | ^^E, ^3F♭ |
| 18 | 242.7 | 23/20, 38/33 | ^3E, v4F |
| 19 | 256.2 | 22/19, 36/31 | ^4E, v3F |
| 20 | 269.7 | 7/6 | v3E♯, vvF |
| 21 | 283.1 | 20/17, 33/28 | vvE♯, vF |
| 22 | 296.6 | 19/16, 32/27 | F |
| 23 | 310.1 | ^F, ^^G♭♭ | |
| 24 | 323.6 | 41/34 | ^^F, ^3G♭♭ |
| 25 | 337.1 | 17/14 | ^3F, v4G♭ |
| 26 | 350.6 | 38/31 | ^4F, v3G♭ |
| 27 | 364 | 21/17, 37/30 | v3F♯, vvG♭ |
| 28 | 377.5 | 41/33 | vvF♯, vG♭ |
| 29 | 391 | vF♯, G♭ | |
| 30 | 404.5 | 24/19 | F♯, ^G♭ |
| 31 | 418 | 14/11 | ^F♯, ^^G♭ |
| 32 | 431.5 | 41/32 | ^^F♯, ^3G♭ |
| 33 | 444.9 | 22/17, 31/24 | ^3F♯, v4G |
| 34 | 458.4 | 30/23 | ^4F♯, v3G |
| 35 | 471.9 | 21/16 | v3F𝄪, vvG |
| 36 | 485.4 | 41/31 | vvF𝄪, vG |
| 37 | 498.9 | 4/3 | G |
| 38 | 512.4 | 39/29 | ^G, ^^A♭♭ |
| 39 | 525.8 | 23/17, 42/31 | ^^G, ^3A♭♭ |
| 40 | 539.3 | 15/11, 41/30 | ^3G, v4A♭ |
| 41 | 552.8 | 11/8 | ^4G, v3A♭ |
| 42 | 566.3 | v3G♯, vvA♭ | |
| 43 | 579.8 | 7/5 | vvG♯, vA♭ |
| 44 | 593.3 | 31/22, 38/27 | vG♯, A♭ |
| 45 | 606.7 | 27/19 | G♯, ^A♭ |
| 46 | 620.2 | 10/7 | ^G♯, ^^A♭ |
| 47 | 633.7 | ^^G♯, ^3A♭ | |
| 48 | 647.2 | 16/11 | ^3G♯, v4A |
| 49 | 660.7 | 22/15, 41/28 | ^4G♯, v3A |
| 50 | 674.2 | 31/21, 34/23 | v3G𝄪, vvA |
| 51 | 687.6 | vvG𝄪, vA | |
| 52 | 701.1 | 3/2 | A |
| 53 | 714.6 | ^A, ^^B♭♭ | |
| 54 | 728.1 | 32/21, 35/23 | ^^A, ^3B♭♭ |
| 55 | 741.6 | 23/15 | ^3A, v4B♭ |
| 56 | 755.1 | 17/11 | ^4A, v3B♭ |
| 57 | 768.5 | v3A♯, vvB♭ | |
| 58 | 782 | 11/7 | vvA♯, vB♭ |
| 59 | 795.5 | 19/12 | vA♯, B♭ |
| 60 | 809 | A♯, ^B♭ | |
| 61 | 822.5 | 37/23 | ^A♯, ^^B♭ |
| 62 | 836 | 34/21 | ^^A♯, ^3B♭ |
| 63 | 849.4 | 31/19 | ^3A♯, v4B |
| 64 | 862.9 | 28/17 | ^4A♯, v3B |
| 65 | 876.4 | v3A𝄪, vvB | |
| 66 | 889.9 | vvA𝄪, vB | |
| 67 | 903.4 | 27/16, 32/19 | B |
| 68 | 916.9 | 17/10 | ^B, ^^C♭ |
| 69 | 930.3 | 12/7 | ^^B, ^3C♭ |
| 70 | 943.8 | 19/11, 31/18 | ^3B, v4C |
| 71 | 957.3 | 33/19, 40/23 | ^4B, v3C |
| 72 | 970.8 | 7/4 | v3B♯, vvC |
| 73 | 984.3 | 30/17 | vvB♯, vC |
| 74 | 997.8 | 16/9 | C |
| 75 | 1011.2 | ^C, ^^D♭♭ | |
| 76 | 1024.7 | 38/21 | ^^C, ^3D♭♭ |
| 77 | 1038.2 | 31/17 | ^3C, v4D♭ |
| 78 | 1051.7 | 11/6 | ^4C, v3D♭ |
| 79 | 1065.2 | 37/20 | v3C♯, vvD♭ |
| 80 | 1078.7 | 28/15, 41/22 | vvC♯, vD♭ |
| 81 | 1092.1 | vC♯, D♭ | |
| 82 | 1105.6 | 36/19 | C♯, ^D♭ |
| 83 | 1119.1 | 21/11 | ^C♯, ^^D♭ |
| 84 | 1132.6 | ^^C♯, ^3D♭ | |
| 85 | 1146.1 | 31/16, 33/17 | ^3C♯, v4D |
| 86 | 1159.6 | 41/21 | ^4C♯, v3D |
| 87 | 1173 | v3C𝄪, vvD | |
| 88 | 1186.5 | vvC𝄪, vD | |
| 89 | 1200 | 2/1 | D |
Notation
Ups and downs notation
89edo can be notated using ups and downs notation using Helmholtz–Ellis accidentals:
| Step Offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp Symbol |  |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
| Flat Symbol |  |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
Approximation to JI
Zeta peak index
| Tuning | Strength | Closest edo | Integer limit | ||||||
|---|---|---|---|---|---|---|---|---|---|
| ZPI | Steps per octave | Step size (cents) | Height | Integral | Gap | Edo | Octave (cents) | Consistent | Distinct |
| 497zpi | 89.0229355804124 | 13.4796723133902 | 7.567368 | 1.124501 | 16.042570 | 89edo | 1199.69083589172 | 12 | 12 |
Regular temperament properties
| Subgroup | Comma list | Mapping | Optimal 8ve stretch (¢) |
Tuning error | |
|---|---|---|---|---|---|
| Absolute (¢) | Relative (%) | ||||
| 2.3 | [-141 89⟩ | [⟨89 141]] | +0.262 | 0.262 | 1.95 |
| 2.3.5 | 32805/32768, 10077696/9765625 | [⟨89 141 207]] | −0.500 | 1.098 | 8.15 |
| 2.3.5.7 | 126/125, 1728/1715, 32805/32768 | [⟨89 141 207 250]] | −0.550 | 0.955 | 7.08 |
| 2.3.5.7.11 | 126/125, 176/175, 243/242, 16384/16335 | [⟨89 141 207 250 308]] | −0.526 | 0.855 | 6.35 |
Rank-2 temperaments
| Periods per 8ve |
Generator* | Cents* | Associated ratio* |
Temperaments |
|---|---|---|---|---|
| 1 | 13\89 | 175.28 | 72/65 | Sesquiquartififths / sesquart |
| 1 | 21\89 | 283.15 | 13/11 | Neominor |
| 1 | 23\89 | 310.11 | 6/5 | Myna |
| 1 | 29\89 | 391.01 | 5/4 | Amigo |
| 1 | 37\89 | 498.87 | 4/3 | Grackle |
* Octave-reduced form, reduced to the first half-octave, and minimal form in parentheses if distinct
Scales
Music
- Singing Golden Myna (2022) – myna[11] in 89edo