User talk:FloraC/Archive 2020

This page is an archive of past discussions. Please do not edit the contents of this page. If you wish to start a new discussion or revive an old one, please do so on the current talk page.

Thanks

Thanks for correcting all my mistakes on the supermajor chord's ratio! TallKite (talk) 06:54, 30 April 2020 (UTC)

Question about VisualEdit

Hello FloraC,
as I see, you made part of your edits using the visual editor. Do you remember if it was enabled by default or did you need to enable it yourself in Preferences? (I didn't see this option when I was active before pausing more than a year. I tried it now, but disabled it again: it breaks one of my favorite access keys (v) for the [Show changes] button.)
BTW: thanks for all your gardening work! 🙂
--Xenwolf (talk) 11:31, 8 June 2020 (UTC)

It's enabled by default. I play with another wiki project where there's no such a feature, and I was impressed when I found it here! FloraC (talk) 15:53, 8 June 2020 (UTC)

I see. Is there a way to access advances formatting features like the new table column classes? I tried to find it, but wasn't successful. --Xenwolf (talk) 18:25, 8 June 2020 (UTC)

The feature about alignment is probably completely missing. FloraC (talk) 18:41, 8 June 2020 (UTC)

Difference between clan and family

Hi FloraC,
Do you maybe know the difference between clan and family? Today I found both in Sensamagic. --Xenwolf (talk) 08:59, 9 June 2020 (UTC)

As far as I understand, family: a single comma is tempered out; clan: more commas. Maybe I'm not the best one to ask. I'm just a beginner anyway. FloraC (talk) 11:54, 9 June 2020 (UTC)

Thanks so far, I let you know if I know more... --Xenwolf (talk) 13:14, 9 June 2020 (UTC)

Text alignment "left-9" fixed

Hi FloraC,
The issue you found is fixed now. Apart from the amount of extra work it has certainly created for you, I think it (Approximate Ratios in column 3) looks even better in this form than before.
--Xenwolf (talk) 07:03, 12 June 2020 (UTC)

FJS name

Hi FloraC,
Do you have a good reference for this naming scheme? I'd plead to add a link to it in the template.
--Xenwolf (talk) 19:50, 23 July 2020 (UTC)

Ah, only now I see you already did exactly that. --Xenwolf (talk) 19:53, 23 July 2020 (UTC)

You answered my long-year badness question

... by adding this important clarification. Thanks a lot. --Xenwolf (talk) 11:48, 24 July 2020 (UTC)

Yes that confused me too until I really tried to reproduce them. FloraC (talk) 12:14, 24 July 2020 (UTC)

Quartismic Temperaments

Hey, Flora, remember the computer-generated lists of quartismic EDOs you showed me and Inthar on Inthar's user page? Well, come to find out that the computer missed 44edo on both counts, yet, when I checked it by performing the procedure documented on the monzo page to test for the quartisma, I got "0" as a result, making 44edo a quartismic temperament. I should point out that judging by the degree of relative error for the 7th harmonic in 44edo, I doubt that 88edo will make the cut. So, I'm thinking we should combine our strategies for finding quartismic temperaments and double-check our findings with the monzo test. --Aura (talk) 18:32, 8 September 2020 (UTC)

The algorithm doesn't miss anything. It's a sequence of edos with progressively lower TE error. 44edo is contorted in the 11-limit, meaning that it's the same as 22, which is outperformed by 24, so it doesn't show up. FloraC (talk) 04:39, 9 September 2020 (UTC)

If the algorithm doesn't miss anything, then I'd like to know why the different runs don't all get the same EDOs... --Aura (talk) 01:09, 11 September 2020 (UTC)

The first result is on no-5 11-limit (2.3.7.11) basis. The second full 11-limit (2.3.5.7.11). FloraC (talk) 02:01, 11 September 2020 (UTC)

I should also point out that when I tried this same test on 46edo, I got "-1" as a result despite 46edo seeming to have the telltale signs of being a quartismic temperament- Talk about inconsistency. For the record, Inthar and I both thought that 46edo was one of these quartismic temperaments- but we were all wrong. --Aura (talk) 21:20, 8 September 2020 (UTC)

No it's zero. You can also verify that by simply looking at the interval table. FloraC (talk) 04:39, 9 September 2020 (UTC)

I must point out that after double-checking and correcting an error in my calculations, I've reestablished that 46edo actually does temper out the quartisma. However, judging from from this calculation, there are still EDOs like 23edo that at first glance appear to temper out the quartisma but nevertheless actually fail the monzo test. --Aura (talk) 05:17, 9 September 2020 (UTC)

Okay, I need help to redefine the quartismic temperament properly. After stumbling across this site, I'm now trying to re-gather my bearings. All I know is that the Altierran temperaments are a specific type of quartismic temperament that tempers out the schisma as well as the quartisma... --Aura (talk) 02:18, 11 September 2020 (UTC)

Did you get my wiki email?

... maybe you dropped it as spam? Best regards --Xenwolf (talk) 11:15, 10 September 2020 (UTC)

Yes and I replied using the same wiki mail function. Seems you don't receive it. I'll try again. FloraC (talk) 11:35, 10 September 2020 (UTC)

Should work this time. FloraC (talk) 11:37, 10 September 2020 (UTC)

Yes, received it now (for the first time). --Xenwolf (talk) 12:51, 10 September 2020 (UTC)

159edo Interval Chart

Hey, Flora, I saw the conversation on the discussion page for Table of 159edo intervals. I hope you like what you see now. I also hope that this chart isn't made shorter after I finish my work- I really want others to see both members of any given pair octave compliments- especially when the more obscure intervals are involved... --Aura (talk) 03:29, 17 September 2020 (UTC)

That looks very neat. And it need not be shortened to half-octave since it's not in the main edo page, where I reckon spacing is a matter. FloraC (talk) 03:45, 17 September 2020 (UTC)

undecimal subminor second, undecimal supermajor seventh

May I invite you to discuss the topic(s) under Talk:33/32#undecimal subminor second? --13:09, 18 September 2020 (UTC)

FJS help

Hi FloraC,
you seem to have a solid understanding of the Functional Just System.
Would you please help me to get started with filling in the FJS name parameter] on interval pages?
--Xenwolf (talk) 20:27, 18 September 2020 (UTC)

Sure. And to answer the question when an interval starts with P, M, m, A, or d, an interval in FJS is interpreted as Pythagorean tuning offset by some commas. So if the Pythagorean note is major, it is M, if the Pythagorean note is minor, it is m, and so on. In that regard it's the same as Helmholtz-Ellis so you might first get started from that. FloraC (talk) 05:50, 19 September 2020 (UTC)

Diatonic and Paradiatonic Functionality Chart

Hey Flora, I recently made a new version of the Musical Function Chart that I referenced in out discussion on 33/32. Would you mind looking over this? I hope this version is better than the one I initially referenced in the discussion.

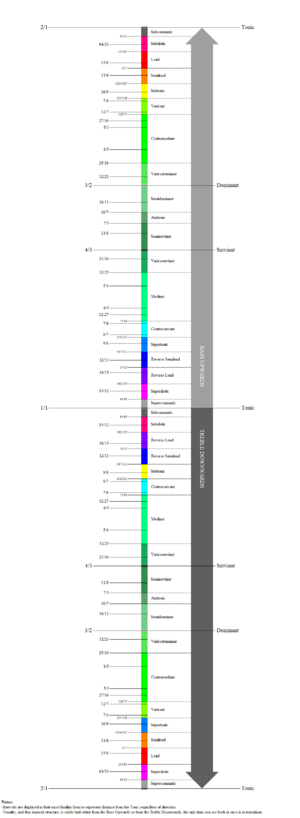
As you can see, 33/32 and its octave compliment 64/33 both appear in regions designated "Superdietic" and "Subdietic". Both "Superdietic" and "Subdietic" are related to "diesis" on account of a diesis- according to one definition- being the smallest usable melodic interval. I know I've found that 33/32 is definitely large enough to be a melodic interval in its own right. However, I also can't help but notice the fact that intervals in both the Superdietic region and the Subdietic region tend to have multiple functions- that is, depending on both the direction of a tonality's construction and the structure of a given chord, they tend to alternate either between primes and seconds or between sevenths and octaves. For instance, while 33/32 functions as a prime in a 22:26:33 triad built on the octave reduced 11th harmonic, it functions as a second in a 28:33:42 triad built on the octave reduced 7th harmonic if 7/4 is interpreted as a type of seventh, as it forms the interval 33/28- a type of minor third- with the iteration of the 7th harmonic directly below it. I also notice that in 159edo, 33/32 is located further away from the perfect unison than the unison-second as depicted in SHEFKHED interval names- thus qualifying it for designation as a second, even though it is a perfect fifth above 11/8. I do note that 11/8 forms a similar ratio with 7/6. As you can see from the chart, both 8/7 and 7/6 fall into a region designated "Contravaricant", indicating the high likelihood for intervals in this region to act as either seconds or thirds, yet, while 11/8 could rightly be analyzed as a superdiminished fifth, it more commonly functions a fourth relative to the Tonic- particularly outside of Blues music... --Aura (talk) 11:12, 19 September 2020 (UTC)

Optimal Patent Vals for various Quartismic Temperaments

Hey Flora, I see from your recent edits that you appear to know how to find the optimal patent vals for different temperaments... Could you help me find the optimal patent vals for the various Quartismic temperaments? Oh, and could you clean up the EDO lists on the Quartismic family page while you're at it? Thanks. --Aura (talk) 11:33, 3 October 2020 (UTC)

The sequence I originally showed you was that of progressively lower TE error. The last entry is the optimal patent val. FloraC (talk) 12:01, 3 October 2020 (UTC)

That was back when there was only one type of Quartismic temperament, and now we have a whole family of them... --Aura (talk) 12:04, 3 October 2020 (UTC)

I'll look into them when time permits. Now I'm focusing on my own construction, and I'd appreciate it if you help to come up with names. FloraC (talk) 12:18, 3 October 2020 (UTC)

What sorts of names do you want me to come up with? --Aura (talk) 17:03, 3 October 2020 (UTC)

Temperaments. In particular, one that tempers out 896/891 & 472392/471625, an 11-limit extension to canou family. Maybe we talk about it at Talk:Canou family. FloraC (talk) 18:58, 3 October 2020 (UTC)

159edo notation systems

Hey, Flora, what do you think of how this conversation has been going since you last commented on it? --Aura (talk) 16:49, 15 October 2020 (UTC)

spaced interwiki syntax

Your suggestion to add a space after the interwiki prefix for style reasons absolutely makes sense to me. I already added it in Help:Editing #Interwiki links which also announces google searches being available in the same syntax. --Xenwolf (talk) 17:59, 15 October 2020 (UTC)

My Own System

Hey, Flora, I'm busy trying to create my own music system, and also explain how it's different from the Hunt System. I would appreciate feedback on what I have written so far on this. Thanks! --Aura (talk) 12:31, 19 October 2020 (UTC)

I read it, but I prefer not judging it for now. Look, I don't have that 24edo-background as you do; I haven't worked substantially with 11-limit (I'm most experienced with 19edo). My "ideas of tonality" is also under construction – I might take a deeper look at yours after finishing mine. FloraC (talk) 13:08, 19 October 2020 (UTC)

Ah. That makes sense. ---Aura (talk) 08:38, 22 October 2020 (UTC)-Aura (talk) 18:27, 19 October 2020 (UTC)

just approximation tables

When I added a table to 73edo to reflect its mapping to prime (or primary) intervals, I decided to add the approximating steps as well, and decided to stop at 23. It's very similar to the tables you usually add to edo pages, there I found examples with more or less than these columns. What do you think is a reasonable set? Besides your criticism concerning the FJS, wouldn't it be useful to add the fifth span to these tables? (I've to confess that I'm still not able to calculate them) --Xenwolf (talk) 20:27, 19 October 2020 (UTC)

The fifthspan is included for example in 17edo, 19edo, or 53edo. --Xenwolf (talk) 04:16, 20 October 2020 (UTC)

Tbh I don't add the prime limits with specific criteria. I do if I see the prime limit potentially usable in that edo. Neither have I got any formula for fifthspan. FloraC (talk) 05:16, 20 October 2020 (UTC)

Another Comma

Hey Flora, I've recently discovered that 19712/19683 is the sum of the quartisma and the nexuma- two commas that I've named- and I see you have this comma on as one of the commas tempered out in some of the various members of the Canou family. I think we need to come up with a name for 19712/19683 now. I mean, I think this will help bring our projects together in a way. --Aura (talk) 15:43, 21 October 2020 (UTC)

I can confirm the nexus comma is indeed an important one in 2.3.11 subgroup. I'm working on projective tuning spaces and hopefully that'll allow a graphical approach to both rastma and nexus comma. Btw I prefer "nexus comma" to "nexuma".

I have to admit "nexuma" is still a pretty cool name- if you know how to pronounce it. That said, I'm definitely keeping "nexus comma" as one of the official names. --Aura (talk) 08:38, 22 October 2020 (UTC)

I also noticed 19712/19683 is a good extension to canou temperament. It identifies 11/8 by 2187/2048 over 9/7. It's tempered out in vishnu, newt, kwai, supers, guiron and amity so I guess it may be called "vishnewt". FloraC (talk) 07:08, 22 October 2020 (UTC)

"Vishnewt" is a funny comma name. However, I'm thinking this comma should have other names for the sake of those who don't want to be left with lingering giggling fits. --Aura (talk) 08:38, 22 October 2020 (UTC)

I finally decided to call 19712/19683 the "symbiotic comma" due to it being tempered out in in vishnu, newt, kwai, supers, guiron and amity, as well as both it being a good extension to canou temperament and effectively linking our projects together. I hope you like the name... --Aura (talk) 04:32, 23 November 2020 (UTC)

That was an inspiring name. The part I don't like is that I feel somewhat obliged to inform people in the FB group again. FloraC (talk) 06:50, 23 November 2020 (UTC)

space around arguments

... is what most templates explicitly support. I like help editors and reviewers (see diff view) to separate contents from frames, just like in table cells, so I added trimming to templates like Monzo and EDOs. I observe that you generally do not put spaces around template arguments. Maybe there is a good reason for this, then please tell me so I understand. But if you don't care, I would find it very relaxing if you could join my argument. 🙂 --Xenwolf (talk) 12:05, 11 November 2020 (UTC)

Well, I don't really care. FloraC (talk) 12:11, 11 November 2020 (UTC)

Great, thanks. 🙂 --Xenwolf (talk) 15:02, 11 November 2020 (UTC)

Thanks a lot

.. for fixing all my errors! That really is an alarming error rate that I have delivered. I must have done too much at once. BTW: Do you happen to know anything about Active Server Pages? --Xenwolf (talk) 09:44, 13 November 2020 (UTC)

I hope you're going well. I have no clue about Active Server Pages. FloraC (talk) 11:25, 13 November 2020 (UTC)

families and clans as categories

I sometimes think of breaking apart the collections of temperaments in favor to building categories of pages each dedicated to a single temperament. This way, it would be possible to have some temperaments belong to different collections. You are seemingly more familiar with the temperaments, what do you think about my idea? --Xenwolf (talk) 20:28, 20 November 2020 (UTC)

I'm not sure about the detail. Currently some temperaments has got their own dedicated pages (such as meantone), and some not. Meanwhile the "data" are in collection pages (such as meantone family). Do you mean each entry of "data" in the collection pages should be moved to their respective dedicated pages, and only links should remain there? FloraC (talk) 08:09, 21 November 2020 (UTC)

I have the impression that these pages are not really readable and linking to entries in it is harder than it could be. But I'm not absolutely sure if dedicated pages for temperaments has no downsides. What would be left for family and clan pages then? I'm really not an expert concerning the content, but the kind of page we have now seems to "fight" with the wiki concept. Maybe it would help to open a broader discussion about this? In the todo corner? --Xenwolf (talk) 11:37, 21 November 2020 (UTC)

Eventually, most temperaments should have their dedicated pages. That's the only thing I'm sure about. We absolutely should open a broader discussion for such considerable changes. FloraC (talk) 12:03, 21 November 2020 (UTC)

Thanks for sharing your thoughts about this. --Xenwolf (talk) 13:00, 21 November 2020 (UTC)

Name for 169/162

Hey, Flora, I was messing around, and I've discovered that the 13-limit is really good for trientone (third-tone) intervals in the same way that the 11-limit is really good for quartertone intervals- seriously, the simplest combination of trientone intervals adding up to 9/8 is basically 27/26-27/26-169/162, with 27/26 acting as the parachromatic interval and 169/162 acting as the paradiatonic interval. So now, I'm looking for a name for 169/162... do you have any ideas? --Aura (talk) 21:53, 23 November 2020 (UTC)

That's not how I approach just intonation. I can use three different but much simpler third tones to reach an exact whole tone: 9/8 = (27/26)*(26/25)*(25/24). Alternatively I might want to temper out 2197/2187 = (9/8)/(27/26)³, or even {325/324, 625/624}, which equates all three.

But as I see 169/162 is the difference between 13/9 and 18/13, it can be the "tridecimal tritonic third tone". FloraC (talk) 06:40, 24 November 2020 (UTC)

Makes sense. However, I do in fact approach just intonation from a classical-minded semi-artistic standpoint. --Aura (talk) 07:01, 24 November 2020 (UTC)

Another comma

Hey, Flora, I found another comma- 200704/200475- which marks the difference between a stack of two 135/112 and a single 16/11... I can confirm that this comma is tempered out in 159edo, and for some reason, I can't help but think it should be called the "reef comma" as it is similar to the island comma in that it splits a pretty important utonal interval in half when tempered out... What do you think? --Aura (talk) 03:48, 29 November 2020 (UTC)

Hmmm, I'm yet to see how 135/112 is a useful interval by itself. FloraC (talk) 07:54, 29 November 2020 (UTC)

Funny enough, 135/112 makes for an excellent third for when you wish to use 16/11 as your outside interval. Sure, the result sounds something like a xenharmonic diminished triad, but judging from my experience with music, I'd say there are times when that sort of thing is exactly what you want. --Aura (talk) 17:44, 29 November 2020 (UTC)

dev wiki

Hello Flora, did you already know that this wiki has three siblings: de, es, and dev? In dev, there is Scribunto+Lua running right now; maybe you are interested in co-evaluating if this option should be adapted for production. See mw:Extension:Scribunto/Lua_reference_manual and dev: Module:Hello for a start. --Xenwolf (talk) 09:33, 7 December 2020 (UTC)

I'll take a look, but tbh I'm not familiar with lua. I'll write to you if I got findings. FloraC (talk) 15:21, 7 December 2020 (UTC)

Now it's even more interesting, here I test a module that generates prime approximation tables for EDOs (module link in heading), the task that's not really possible with bare templates, see Test (template link in heading). --Xenwolf (talk) 00:22, 9 December 2020 (UTC)

BTW: Lua is quite new for me, too. But already now I find it much easier to write (and read) than templates. :) --Xenwolf (talk) 00:22, 9 December 2020 (UTC)

Cool. I've been hoping to see it in the edo pages. FloraC (talk) 10:08, 9 December 2020 (UTC)

Also the EDO (alias ET) template could be heavily enhanced by modules. It's really fun to see all template difficulties melting away this way. --Xenwolf (talk) 10:41, 9 December 2020 (UTC)

Concerning "tone ratio" (or maybe better "semitone ratio", or "tonal partition"): indeed its m2:A1. Would you agree that it is only applicable if the fifth is between 3\5 and 4\7 in size? This is because m2 := 3*P8-4*P5 and A1 := 7*P5-4*P8 and this way we can deduce it by knowing the number of steps of the fifth in that EDO (P5\EDO). --Xenwolf (talk) 10:41, 9 December 2020 (UTC)

1. I added a parameter called "Semitones" and it appears as "Semitones (A1:m2)". I haven't implemented it in any edo pages. I'm fine with it changed to "Semitones (m2:A1)". The neologism seems unnecessary now.
2. I disagree on the contraint from non-positive values. It's artificial. FloraC (talk) 11:13, 9 December 2020 (UTC)

∎

Well, but negative-size semitones are also not quite natural (pun intended) ;-) TBH, I personally have no problems with negative values for musical intervals. Concerning the neologism I found out that tonal properties could be the right word. I'm not willing to push this into the template but for me (with a Classic/Baroque/Jazz background) it absolutely makes sense. --Xenwolf (talk) 11:48, 9 December 2020 (UTC)

I'd really like someone joining evaluating Lua on dev. If it can be safely activated here, we will have much less to do with checking tables for correctness and precision consistency, so a good investment of time. --Xenwolf (talk) 11:48, 9 December 2020 (UTC)

meaning of todo

Hi Flora, you added a todo text I don't understand. Can you please elaborate this a bit further? Thanks :) --Xenwolf (talk) 08:25, 10 December 2020 (UTC)

The EDO lists in the temperament pages needs explanation. A guideline for which edo is included and how to generate the list should also be there. FloraC (talk) 08:29, 10 December 2020 (UTC)

I see. Should we start a project for that or do you have enough expertise to give reasonable criteria? --Xenwolf (talk) 09:17, 10 December 2020 (UTC)

It's a sequence of progressively lower tuning error, most probably TE error based on what I've seen (despite that there are sometimes outliers). FloraC (talk) 09:31, 10 December 2020 (UTC)

As you can see, the optimal patent val page gives some practical guide, so it should be clear. FloraC (talk) 09:41, 10 December 2020 (UTC)

So we should probably be more canonical in these temperament sections (I'm already thinking of another template, but if I recall right, we already talked about this idea); it also becomes clear that my new habit of writing the EDO list according to the pattern {{EDOs|legend=1| ... }} falls obviously short. Much better would be to link the leading "EDOs: " before the actual list with an understandable explanation of the background (including rules).

Oh, there's a parameter "legend"? I didn't notice the update. FloraC (talk) 11:42, 10 December 2020 (UTC)

I saw that basically only you are using the template (besides me), and since I also observed that you usually observe the recent changes, I thought that there was no need to tell you about the change. I also was not totally satisfied with it, I'm still wondering if another parameter name would be better or if adding a "shell template" (like "EDOs2", with the parameter set by default) would ease it's usage in the temperament sections, but all the names I thought of seemed unsatisfying... --Xenwolf (talk) 12:25, 10 December 2020 (UTC)

So, what about starting an infobox or infotable for temperaments, a template covering all the aspects? I mean, the content of these sections are highly formalized anyway (although not consistently formatted).

Relative error page needs work

See the discussion tab for Relative_error --TallKite (talk) 06:44, 11 December 2020 (UTC)

6250/6237

So apparently, someone found a comma, 6250/6237, and didn't name it. Thus, when Xenwolf was working on the 72edo page, he and I both tried to find the name of 6250/6237, but we couldn't find a name for it. So, we decided to try and come up with a name for it. After running the numbers through Wolfram Alpha, I found that 6250/6237 is tempered out by both 53edo and 159edo despite their differing patent vals for both 7 and 11. Upon further calculations, I found that 6250/6237 is only slightly smaller than Mercator's comma at 3.60472 cents, and that when this comma is tempered out, 160/77- which is the difference between 5/2 and 77/64- is equated with a stack of four 6/5 minor thirds, and a stack of five 5/4 major thirds is equated with a stack consisting of an octave plus 81/64 plus 77/64. Moreover, it appears that 6250/6237 is also the difference between the keenanisma and the kleisma, as well as the difference between the keenanisma and the undecimal hemifourths comma (160083/160000; needs a better name). Furthermore, I've found that 6250/6237 is also the difference between the amity comma and the symbiotic comma, as well as the sum of the lehmerisma and the wizardharry comma (4000/3993). Do you have any thoughts about what to name this comma? --Aura (talk) 18:28, 23 December 2020 (UTC)

Its color name is Luruquinyo. Edos 12, 15, 19 and 34 all temper it out. --TallKite (talk) 05:56, 24 December 2020 (UTC)

The color name is good, but I'd sooner have another name for it as well. --Aura (talk) 05:58, 24 December 2020 (UTC)

Haven't been able to find any sense with this comma. But to point out a fact, it can't be simultaneously the difference between the keenanisma and the kleisma and between the keenanisma and the undecimal hemifourths comma. You might want to check that. FloraC (talk) 06:02, 24 December 2020 (UTC)

I did. If the kleisma is 15625/15552, and the undecimal hemifourths comma is 160083/160000, then do note that (15625/15552)/(160083/160000) is equal to (6250/6237)^2. Perhaps I said it in the wrong fashion, but the math doesn't lie. The keenanisma is halfway between the kleisma and the undecimal hemifourths comma- (15625/15552)/(6250/6237) = (385/384), and (385/384)/(6250/6237) = (160083/160000). --Aura (talk) 06:07, 24 December 2020 (UTC)

Ok that makes sense. FloraC (talk) 06:16, 24 December 2020 (UTC)

So, does that clear things up for you? --Aura (talk) 06:18, 24 December 2020 (UTC)

Yep. FloraC (talk) 06:25, 24 December 2020 (UTC)

Since 6250/6237 is tempered out in Octoid, Tritiklesmic, Catakleismic, Quadritikleismic, Compton, Unthirds, Amity, Alphaquarter, Hanuman, and Enlil, and, since this same comma forms the interval between the kleisma and the keenanisma and the interval between the keenanisma and the undecimal hemifourths comma, perhaps we should call this comma the "liganellus comma" or the "liganelluma". Both of these names come from a compound of Latin "ligans" and Latin "anellus", as "ligans" means "uniting" and "anellus" refers to a little ring, including the sort which forms an element in a chain. --Aura (talk) 08:16, 25 December 2020 (UTC)

So far none of the findings about this comma really impresses me, so I'm fine with whatever name it gets. But I don't like you concatenating Latin words with -ma like that. I told you I don't like nexuma. That's because the u isn't part of the root. Simply nexus and liganellus are way better. FloraC (talk) 11:00, 25 December 2020 (UTC)

Actually, "nexum" is the accusative form of "nexus", and "anellum" is the accusative form of "anellus". Also, the reason I attach the "-ma" to the "u" to make an "-uma" type suffix is precisely because there are some cases where I need a vowel in order to attach the "-ma" suffix, and let's face it, "nexusima", which was the other option I was considering, is actually worse than "nexuma". Plus, in the English word "nexus", as in a number of other English nouns derived from Latin, the "u" is already embedded in the word, and it's not a productive suffix outside of taxonomy. Did I mention that I'm a native English speaker and that "sm" clusters are sometimes prone to becoming pronounced like "spm"? This is even true for words like "small"- which I'm liable to pronounce as [ˈspmɑɫ]- and I don't want to end up pronouncing "nexma" as [ˈnæ̠kspmə]. --Aura (talk) 14:08, 25 December 2020 (UTC)

Hmm, since the "-us" in "nexus" is not part of the stem in Latin, would "nexisma" be a good replacement for "nexuma"? --Aura (talk) 21:58, 25 December 2020 (UTC)

But if you're fine with nexisma then you should be fine with nexma as well. FloraC (talk) 07:30, 27 December 2020 (UTC)

Not so fast. Instances of "s" that are stuck between two stop sounds, like what happens when "nexma" is pronounced as [ˈnæ̠kspmə], make it sound almost like there ought to be an extra syllable near the "s" as the lack of such a syllable strikes me as less than ideal in terms of syllable structure. In contrast, "nexisma", which is likely to be pronounced as [næ̠kˈse̽zbmə], actually does have the extra vowel, so you don't have a case of an "s" stuck between two stop sounds. --Aura (talk) 12:36, 27 December 2020 (UTC)

625/624

Hey, Flora, I was just telling Xenwolf about how I found another comma that seems to need a name- 625/624. This comma seems to be like the liganellus comma in that it binds well-known temperaments together- in this case, Catakleismic, Tritikleismic, Quadritikleismic, Octoid, Benediction, Sqrtphi, Ekadash, Countercata, Widefourth, Portentious, Yajna, Enlil, Hanuman, Marvel and Agni. Since this comma seems to be used so much, so on one hand, I could see the name reflecting its usage in extensions. On the other hand, I do see that tempering out this comma not only equates 25/24 with 26/25, splitting 13/12 in half and going part of the way towards splitting the 9/8 whole tone into three (the other comma needing to be tempered in order to finish the job being 676/675), but also equates 39/32 with the interval that results from stacking four 5/4 major thirds then octave-reducing. With all this in mind, perhaps we should call this comma the "vinctrias comma". Any thoughts? --Aura (talk) 23:34, 26 December 2020 (UTC)

Never mind, it Looks like 625/624 is already called the "tunbarsma". Talk about an embarassing case of "open mouth and insert foot". I still like the name "vinctrias" though, so perhaps that name can be given to the temperament that tempers out 625/624 and 676/675. Sorry about this disaster of a thread. --Aura (talk) 23:58, 26 December 2020 (UTC)

Yeah, and just to remind you, all 13-limit superparticular commas have been named. FloraC (talk) 07:30, 27 December 2020 (UTC)

Right. However, it doesn't look like all 17-limit superparticular commas have been named though, so should I try naming some of those? --Aura (talk) 09:37, 27 December 2020 (UTC)

Try as you may, it makes more sense if you have some interesting theories or actual uses about them. So far even the named ones are barely explored: we haven't got pages for commas that are as easy to approach as 256/255, 289/288, and 513/512. FloraC (talk) 11:15, 27 December 2020 (UTC)