385/384
| Ratio | 385/384 |
| Factorization | 2-7 × 3-1 × 5 × 7 × 11 |
| Monzo | [-7 -1 1 1 1⟩ |
| Size in cents | 4.5025618¢ |
| Names | keenanisma, undecimal kleisma |
| Color name | 1ozy1, lozoyo 1sn, Lozoyo comma |
| FJS name | [math]\text{P1}^{5,7,11}[/math] |
| Special properties | superparticular, reduced |
| Tenney height (log2 nd) | 17.1737 |
| Weil height (log2 max(n, d)) | 17.1774 |
| Wilson height (sopfr(nd)) | 40 |
| Harmonic entropy (Shannon, [math]\sqrt{nd}[/math]) |
~1.38718 bits |
| Comma size | small |
| S-expression | S33 × S34 × S35 |
| open this interval in xen-calc | |
The keenanisma or undecimal kleisma is the 11-limit comma 385/384 = [-7 -1 1 1 1⟩ of 4.503 cents. It is both the interval that separates 77/64 and 6/5, and, the sum of the schisma and the symbiotic comma. Tempering it out leads to a temperament of the 11-limit rank-4 keenanismic family.
In addition to equating 77/64 and 6/5, tempering out the keenanisma equates 48/35 with 11/8, 35/24 with 16/11, and 12/11 with 35/32, which are 7-limit intervals of low complexity, lying across from 1/1 in the hexanies 8/7-6/5-48/35-8/5-12/7-2 and 7/6-5/4-35/24-5/3-7/4-2. Hence keenanismic tempering allows the hexany to be viewed as containing some 11-limit harmony. The hexany is a fundamental construct in the 3D lattice of 7-limit pitch classes, the "deep holes" of the lattice as opposed to the "holes" represented by major and minor tetrads, and in terms of the cubic lattice of 7-limit tetrads, the otonal tetrad with root 11 (or 11/8) is represented by [-2 0 0]: 1-6/5-48/35-12/7-2. In terms of 7-limit chord relationships, this complexity is as low as possible for an 11-limit projection comma, equaling the [0 1 -1] of 56/55 and less than the other alternatives. Since keenanismic temperament is also quite accurate, this singles it out as being of special interest.
Edos with patent vals tempering out the keenansima include 15, 19, 22, 31, 34, 37, 41, 53, 68, 72, 118, 159, 190, 212 and 284.
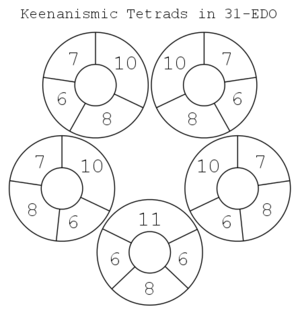
Characteristic of keenanismic tempering are the keenanismic tetrads, 385/384-tempered versions of 1-5/4-3/2-12/7, 1-5/4-10/7-12/7, 1-6/5-3/2-7/4, 1-5/4-16/11-7/4, and 1-14/11-16/11-7/4. These are essentially tempered dyadic chords, where every interval of the chord is a keenanismic tempered version of an interval of the 11-odd-limit tonality diamond, and hence regarded as an 11-odd-limit consonance.
Name
Originally this comma was recommended by Paul Erlich to be named "Keenan's kleisma", after Dave Keenan, due to "it figur[ing] particularly heavily in his many postings about microtemperament"[1]. Dave himself initially resisted this eponymous naming, recommending a more descriptive name[2]. And so undecimal kleisma was adopted[3], and to this day, undecimal kleisma is a name in the Stichting Huygens-Fokker records[4].
The history of the name "keenanisma" is less clear. It is possible that "Keenan's kleisma" remained in use, and eventually was altered to "keenanisma" following a pattern used for many commas named for people. Another possibility is that when a temperament based on this comma was being named, "undecimal kleisma" was seen as unfit to base the name upon, and so Keenan's name was referenced instead, leading to "keenanismic", and then later "keenanisma" was formed from that.
See also
- Keenanismic chords
- Keenanismic family, the rank-4 temperament family where it is tempered out
- Keenanismic temperaments, a collection of rank-3 temperaments where it is tempered out
- Small comma
- List of superparticular intervals