Miracle
Miracle is a regular temperament discovered by George Secor in 1974 which has as a generator an interval, called a secor (after George), that serves as both 15/14 and 16/15 semitones.
Miracle is an exceptionally efficient linear temperament which is a member of both the marvel temperaments, by tempering out 225/224, and the gamelismic clan, by tempering out 1029/1024. It is quite accurate, with TOP error only 0.63 cents/octave, meaning intervals of the 11-odd-limit tonality diamond are represented with only one or two cents of error. Yet it is also very low-complexity (efficient), as evidenced by the high density of 11-odd-limit ratios in the #Interval chain. At least one inversion of every interval in the 11-odd-limit tonality diamond is represented within 22 secors of the starting value.
Some temperaments have 11/9 as a "neutral third", meaning it is exactly half of a 3/2 (tempering out 243/242), and other temperaments (→ Gamelismic clan) have 8/7 as exactly a third of 3/2. Miracle is distinguished by doing both of these things at the same time, so 3/2 is divided into six equal parts. This is in fact the generator of miracle temperament, called a secor, and it represents both 16/15 and 15/14.
Miracle can also be thought of as a cluster temperament with 10 clusters of notes in an octave. The small chroma interval between adjacent notes in each cluster is very versatile, representing 45/44 ~ 49/48 ~ 50/49 ~ 55/54 ~ 56/55 ~ 64/63 all tempered together.
In terms of 13-limit extensions, it is discussed in Miracle extensions. See Gamelismic clan #Miracle for technical data.
Interval chain
In the following table, odd harmonics and subharmonics 1–21 are labeled in bold.
| # | Cents* | Approximate ratios |
|---|---|---|
| 0 | 0.0 | 1/1 |
| 1 | 116.6 | 15/14, 16/15 |
| 2 | 233.3 | 8/7 |
| 3 | 349.9 | 11/9 |
| 4 | 466.6 | 21/16 |
| 5 | 583.2 | 7/5 |
| 6 | 699.9 | 3/2 |
| 7 | 816.5 | 8/5 |
| 8 | 933.2 | 12/7 |
| 9 | 1049.8 | 11/6 |
| 10 | 1166.5 | 49/25, 55/28, 63/32, 88/45, 96/49, 108/55 |
| 11 | 83.1 | 21/20, 22/21 |
| 12 | 199.8 | 9/8 |
| 13 | 316.4 | 6/5 |
| 14 | 433.1 | 9/7 |
| 15 | 549.7 | 11/8 |
| 16 | 666.3 | 22/15 |
| 17 | 783.0 | 11/7 |
| 18 | 899.6 | 27/16, 42/25 |
| 19 | 1016.3 | 9/5 |
| 20 | 1132.9 | 27/14, 48/25 |
| 21 | 49.6 | 33/32, 36/35 |
| 22 | 166.2 | 11/10 |
| 23 | 282.9 | 33/28 |
| 24 | 399.5 | 44/35 |
| 25 | 516.2 | 27/20 |
| 26 | 632.8 | 36/25 |
| 27 | 749.5 | 54/35, 77/50 |
| 28 | 866.1 | 33/20 |
| 29 | 982.8 | 44/25 |
| 30 | 1099.4 | 66/35 |
| 31 | 16.1 | 81/80, 99/98, 121/120 |
* In 11-limit CWE tuning, octave reduced
Chords
Scales
- Mos scales
- Miracle[10] – 72edo tuning
- Blackjack (miracle[21]) – 72edo tuning
- Blackwoo
- Transversal scales
- Others
- Mir1 – 6-tone scale, 72edo tuning
- Mir2 – 6-tone scale, 72edo tuning
- Miracle 8 – 8-tone scale, 72edo tuning
- Miracle 12 – 12-tone scale, 72edo tuning
- Miracle 12a – 12-tone scale, 72edo tuning
- Miracle 24hi – 24-tone scale, 72edo tuning
- Miracle 24lo – 24-tone scale, 72edo tuning
Tunings
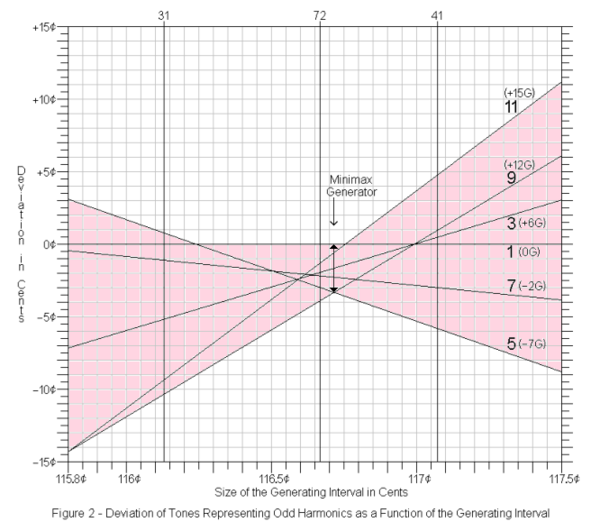
Displayed on the right is a chart of the tuning spectrum of miracle by how the odd harmonics up to 11 are tuned, showing the minimax generator, i.e. the secor.
Prime-optimized tunings
| Euclidean | ||
|---|---|---|
| Unskewed | Skewed | |
| Equilateral | CEE: ~15/14 = 116.516¢ | CSEE: ~15/14 = 116.561¢ |
| Tenney | CTE: ~15/14 = 116.677¢ | CWE: ~15/14 = 116.676¢ |
| Benedetti, Wilson |
CBE: ~15/14 = 116.730¢ | CSBE: ~15/14 = 116.714¢ |
| Euclidean | ||
|---|---|---|
| Unskewed | Skewed | |
| Equilateral | CEE: ~15/14 = 116.687¢ | CSEE: ~15/14 = 116.630¢ |
| Tenney | CTE: ~15/14 = 116.711¢ | CWE: ~15/14 = 116.647¢ |
| Benedetti, Wilson |
CBE: ~15/14 = 116.736¢ | CSBE: ~15/14 = 116.677¢ |
Tuning spectrum
| Edo generator |
Eigenmonzo (unchanged-interval) |
Generator (¢) | Comments |
|---|---|---|---|
| 15/8 | 111.731 | ||
| 2\21 | 114.286 | Lower bound of 7-odd-limit diamond monotone | |
| 7/4 | 115.587 | ||
| 11/9 | 115.803 | ||
| 3\31 | 116.129 | Lower bound of 9- and 11-odd-limit, 11-limit 15- and 21-odd-limit diamond monotone | |
| 5/4 | 116.241 | ||
| 21/11 | 116.412 | ||
| 15/11 | 116.441 | ||
| 7/5 | 116.502 | ||
| 10\103 | 116.505 | ||
| [0 -27 25 5⟩ | 116.573 | 7-odd-limit least squares | |
| [0 -19 20⟩ | 116.578 | 5-odd-limit least squares | |
| 5/3 | 116.588 | 5- and 7-odd-limit minimax | |
| 11/10 | 116.591 | ||
| 11/6 | 116.596 | ||
| 11/7 | 116.617 | ||
| 7/6 | 116.641 | ||
| 7\72 | 116.667 | ||
| [0 17 -11 -6 11⟩ | 116.672 | 11-odd-limit least squares | |
| 9/5 | 116.716 | 9- and 11-odd-limit minimax, Secor's definition of secor | |
| [0 117 -44 -19⟩ | 116.721 | 9-odd-limit least squares | |
| 11/8 | 116.755 | ||
| 21/20 | 116.770 | ||
| 9/7 | 116.792 | ||
| 11\113 | 116.814 | ||
| 3/2 | 116.993 | ||
| 4\41 | 117.073 | Upper bound of 11-odd-limit, 11-limit 15- and 21-odd-limit diamond monotone | |
| 21/16 | 117.695 | ||
| 15/14 | 119.443 | ||
| 1\10 | 120.000 | Upper bound of 7- and 9-odd-limit diamond monotone |
Music
- Realm of Possibility (2021) – Miracle[31] with a 116.72-cent generator and 1200.53-cent octave
- Blackjack (2001) – play | SoundClick – Blackjack (Miracle[21])
- Blacklight (2002) – play | SoundClick – Blackjack (Miracle[21])
- Black and Jill (2003) – Blackjack (Miracle[21])
- Soprano version – play | SoundClick
- Udderbot version
- Inner Voices (2005) – Blackjack (Miracle[21])
- Transpian (2006) – Blackjack (Miracle[21])
- microproj (2007) – Blackjack (Miracle[21])