Didacus
| Didacus |
176/175, 1375/1372 (2.5.7.11)
(11-odd limit) 4.13 ¢
(11-odd limit) 19 notes
Didacus is a temperament of the 2.5.7 subgroup, tempering out 3136/3125, the hemimean comma, such that two intervals of 7/5 reach the same point as three intervals of 5/4; the generator is therefore (7/5)/(5/4) = 28/25, two of which stack to 5/4 and three of which stack to 7/5, meaning that the 4:5:7 chord is "locked" to (0 2 5) in terms of logarithmic size and generator steps. It presents one of the most efficient traversals of the no-threes subgroup, especially considering that some tunings of didacus extend neatly to 11 and 13 (as explained below).
31edo is a very good tuning of didacus, with its generator 5\31 (which is the "mean tone" of 31edo); but 25edo, 37edo, and 68edo among others are good tunings as well. As this generator tends to be slightly less than 1/6 of the octave, MOS scales of didacus tend to consist of 6 long intervals interspersed by sequences of diesis-sized steps (representing 50/49~128/125), therefore bearing similar properties to those of slendric.
It also has a simple extension to prime 11 - undecimal didacus, by tempering out 176/175, the valinorsma, so that (5/4)2 is equated to 11/7 and 9 generators stack to 11/4; prime 13 can be found by tempering out 640/637, equating 16/13 to 49/40, and thereby putting the 13th harmonic 8 generators down. Beyond tridecimal didacus, further extensions to primes 17 and 19, known as roulette and mediantone, are also possible, sharing in common the interpretation of the generator as 19/17.
As for prime 3, while didacus has as a weak extension, among others, septimal meantone (didacus is every other step of septimal meantone, and has an interpretation such that the generator represents 9/8~10/9, known as isra), strong extensions that include 3 are rather complex. Hemithirds (25 & 31) tempers out 1029/1024 to find the fifth at 3/2 ~ (8/7)3, and therefore the 3rd harmonic 15 generators down; and hemiwürschmidt (31 & 37) tempers out 2401/2400 so that (5/4)8 is equated to 6/1, finding the 3rd harmonic 16 generators up (and as described for the page for 5-limit würschmidt, there is also a free extension to find 23/1 at 28 generators). These two mappings intersect in 31edo, though the latter hews closer to the optimal range for undecimal didacus specifically.
For technical data, see Hemimean clan #Didacus.
Theory
Interval chain
In the following table, odd harmonics and subharmonics 1–35 are labeled in bold.
| # | Cents* | Approximate ratios | |||
|---|---|---|---|---|---|
| 2.5.7 intervals | Intervals of extensions | ||||
| Tridecimal didacus | Hemithirds | Hemiwürschmidt | |||
| 0 | 0.0 | 1/1 | |||
| 1 | 194.4 | 28/25, 125/112 | 49/44, 55/49 | ||
| 2 | 388.9 | 5/4 | 44/35 | 144/115 | |
| 3 | 583.3 | 7/5 | 128/91 | ||
| 4 | 777.7 | 25/16 | 11/7 | 36/23 | |
| 5 | 972.1 | 7/4 | 44/25, 160/91 | 184/105 | |
| 6 | 1166.6 | 49/25, 125/64 | 55/28, 128/65 | 96/49, 45/23 | |
| 7 | 161.0 | 35/32 | 11/10, 100/91 | 23/21, 126/115 | |
| 8 | 355.4 | 49/40 | 16/13 | 128/105 | 60/49, 92/75 |
| 9 | 549.9 | 175/128 | 11/8 | 48/35, 63/46, 115/84 | |
| 10 | 744.3 | 49/32 | 20/13, 77/50 | 32/21 | 23/15, 75/49 |
| 11 | 938.7 | 55/32, 112/65 | 128/75 | 12/7 | |
| 12 | 1133.1 | 25/13, 77/40 | 40/21 | 23/12, 48/25 | |
| 13 | 127.6 | 14/13 | 16/15 | 15/14 | |
| 14 | 322.0 | 77/64, 110/91 | 25/21 | 6/5 | |
| 15 | 516.4 | 35/26, 88/65 | 4/3 | 75/56 | |
| 16 | 710.8 | 98/65 | 112/75 | 3/2 | |
| 17 | 905.3 | 22/13 | 5/3 | 42/25 | |
| 18 | 1099.7 | 49/26 | 28/15 | 15/8 | |
| 19 | 94.1 | 55/52 | 25/24 | 21/20 | |
* In CWE undecimal didacus
The hexatonic framework
The 2.5.7 subgroup can be crudely approximated by 6edo, which is itself technically a didacus tuning as 5/4 spans 2 steps and 7/5 spans 3. Every other didacus tuning is essentially a dietic inflection of this basic hexatonic structure. Therefore, the intervals of didacus can be organized according to how many steps of 6edo, or equivalently the 6-note MOS, they correspond to. They can be labeled "wholetone", "ditone", "tritone", etc., and inflected so that "minor" intervals are those just below a step of 6edo, and "major" intervals are just above, whereas the unison, octave, and generators can be labeled "perfect" instead. Below are the intervals within 10 generators of the unison in undecimal CEE tuning.
| Steps of 6edo | Unison | Wholetone | Ditone | Tritone | Tetratone | Pentatone | Hexatone |
|---|---|---|---|---|---|---|---|
| "Augmented" interval | 33.89 | 228.24 | 456.49 | 650.84 | 845.19 | 1039.54 | |
| JI intervals represented | 50/49, 56/55, 65/64 | 8/7, 25/22 | 13/10, 64/49 | 16/11 | 13/8 | 20/11, 64/35 | |
| "Major" interval | 422.60 | 616.95 | 811.30 | ||||
| JI intervals represented | 14/11, 32/25 | 10/7 | 8/5, 35/22 | ||||
| "Perfect" interval | 0.00 | 194.35 | 1005.65 | 1200.00 | |||
| JI intervals represented | 1/1 | 28/25 | 25/14 | 2/1 | |||
| "Minor" interval | 388.70 | 583.05 | 777.40 | ||||
| JI intervals represented | 5/4, 44/35 | 7/5 | 11/7, 25/16 | ||||
| "Diminished" interval | 160.46 | 354.81 | 549.16 | 743.51 | 971.76 | 1166.11 | |
| JI intervals represented | 11/10, 35/32 | 16/13, 49/40 | 11/8 | 20/13, 49/32 | 7/4, 44/25 | 49/25, 55/28 |
Similarly to tertian harmony in diatonic and chain of fifths-based systems, a system of harmony for didacus can be constructed based on these hexatonic categories. The fundamental chord, 4:5:7:8, splits the hexatone into intervals of 2, 3, and 1 tones respectively, so that 4:5:7 is a tritone stacked atop a ditone, 5:7:8 is a wholetone stacked atop a tritone, and 7:8:10 is a ditone stacked atop a wholetone. We can then take these chords' complements to achieve the other permutations of 1, 2, and 3. Notably, other chords one may want to use, such as 8:11:14, also fit into this format; 8:11:14 is a ditone stacked atop a tritone, and in that fashion much can be obtained from creating different harmonies from inflections of hexatonic interval categories.
Isomorphism with Sirius
One of the more peculiar properties of the Didacus temperament is its relationship with the 3.5.7 subgroup temperament Sirius. Sirius tempers out 3125/3087 which is the difference between 5/3 stacked twice and 7/5 stacked thrice, so that 5/3 spans three generators (representing (5/3)/(7/5) = 25/21) and 7/5 spans two. Therefore 7/3, the tritave-reduced harmonic 7, is split into 5 equal parts, 3 of which represent 5/3, the tritave-reduced harmonic 5, and we can see that the 3:5:7 chord in Sirius and 4:5:7 chord in Didacus are isomorphic to each other's complement.
Even more interestingly, Sirius also has 6L 1s and 6L 7s MOS scales, which have the same shape as the 7- and 13-note MOSes of Didacus, and a descendant 19-note MOS scale. While the cardinalities of scales diverge between the temperaments after 19 notes, a quite analogous hexatonic picture to the previous section can be constructed for Sirius, and in sharing this structure despite the massive stretch between octaves and tritaves, Didacus and Sirius provide a unique avenue for transferring consonant octave-repeating no-threes harmony into consonant tritave-repeating no-twos harmony.
Tunings
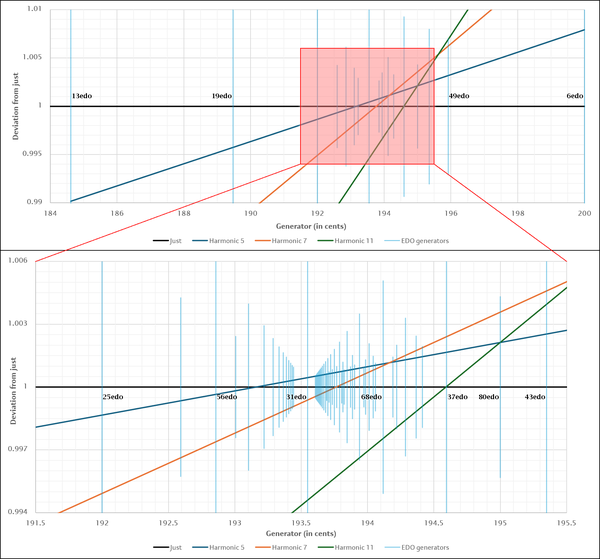
Optimized tunings
| Weight-skew\Order | Euclidean | |
|---|---|---|
| Constrained | Destretched | |
| Tenney | (2.5.7) CTE: ~28/25 = 193.650¢ | (2.5.7) POTE: ~28/25 = 193.772¢ |
| Equilateral | (2.5.7) CEE: ~28/25 = 193.681¢ (12/29-comma) | |
| Tenney | (2.5.7.11) CTE: ~28/25 = 194.246¢ | (2.5.7.11) POTE: ~28/25 = 194.556¢ |
| Optimized chord | Generator value | Polynomial | Further notes |
|---|---|---|---|
| 4:5:7 (+1 +2) | ~28/25 = 197.346 | g5 − 3g2 + 2 = 0 | Close to -1/5-comma |
| 5:7:8 (+2 +1) | ~28/25 = 193.829 | g5 − g2 − 4 = 0 | Close to 37/95-comma |
| 7:8:10 (+1 +2) | ~28/25 = 193.630 | g5 + g2 − 3 = 0 | Close to 33/80-comma |
| 8:11:14 (+1 +1) | ~28/25 = 195.043 | g9 − g5 − 1 = 0 | |
| 11:14:16 (+3 +2) | ~28/25 = 192.698 | g9 − 5g5 + 6 = 0 |
Tuning spectrum
The below tuning spectrum assumes undecimal didacus.
| EDO generator |
Eigenmonzo (unchanged interval)* |
Generator (¢) | Extension | Comments |
|---|---|---|---|---|
| 1\7 | 171.429 | 7dee val, lower bound of (2.5.7) 7-odd-limit diamond monotone | ||
| 2\13 | 184.615 | 13e val, lower bound of (2.5.7.11) 11-odd-limit diamond monotone | ||
| 3\19 | 189.474 | ↓ Spell (49/48) | 19e val | |
| 125/112 | 190.115 | Full-comma | ||
| 7\44 | 190.909 | 44dee val | ||
| 4\25 | 192.000 | ↑ Spell ↓ Hemithirds (1029/1024) |
||
| 13\81 | 192.593 | 81ee val | ||
| 9\56 | 192.857 | 56e val | ||
| 14\87 | 193.103 | 87e val | ||
| 5/4 | 193.157 | 1/2-comma, lower bound of (2.5.7.11) 7- and 11-odd-limit diamond tradeoff | ||
| 19\118 | 193.220 | 118ee val | ||
| 5\31 | 193.548 | ↑ Hemithirds ↓ Hemiwurschmidt (2401/2400) |
||
| 35/32 | 193.591 | 3/7-comma | ||
| 7/4 | 193.765 | 2/5-comma | ||
| 26\161 | 193.789 | 161e val | ||
| 21\130 | 193.846 | 130e val | ||
| 49/40 | 193.917 | 3/8-comma | ||
| 16\99 | 193.939 | |||
| 27\167 | 194.012 | 167e val | ||
| 11\68 | 194.118 | |||
| 7/5 | 194.171 | 1/3-comma, upper bound of (2.5.7) 7-odd-limit diamond tradeoff | ||
| 17\105 | 194.286 | |||
| 23\142 | 194.366 | |||
| 11/8 | 194.591 | |||
| 6\37 | 194.595 | ↑ Hemiwurschmidt | ||
| 196/125 | 194.678 | 1/4-comma | ||
| 19\117 | 194.872 | 117d val | ||
| 13\80 | 195.000 | |||
| 11/10 | 195.001 | |||
| 7\43 | 195.349 | |||
| 11/7 | 195.623 | Upper bound of (2.5.7.11) 11-odd-limit diamond tradeoff | ||
| 8\49 | 195.918 | |||
| 28/25 | 196.198 | Untempered tuning | ||
| 9\55 | 196.364 | 55de val | ||
| 1\6 | 200.000 | Upper bound of (no-threes) 7- and 11-odd-limit diamond monotone |
* Besides the octave
Other tunings
- DKW (2.5.7): ~2 = 1200.000, ~28/25 = 194.061