Kleismic
| Kleismic; hanson; cata |
325/324, 625/624 (2.3.5.13)
((2.3.5.13) 15-odd limit) 2.35 ¢
((2.3.5.13) 15-odd limit) 34 notes
Kleismic, alternatively called hanson in the 5-limit, is a rank-2 temperament and parent of the kleismic family, generated by a classical minor third (6/5), six of which stacked are equated to the perfect twelfth (3/1), and thereby characterized by the vanishing of the kleisma (ratio: 15625/15552, monzo: [-6 -5 6⟩).
Another useful interpretation of the kleisma as a comma is that it makes the classical chromatic semitone, 25/24, into a third-tone by equating three of this interval to 9/8. As 27/24 = 27/26 × 26/25 × 25/24, it is natural to equate 25/24 to 26/25 and 27/26 as well, thereby tempering out the tunbarsma (S25 = (25/24)/(26/25) = 625/624) and the marveltwin comma (S25 × S26 = (25/24)/(27/26) = 325/324 = S10/S12) respectively, and resulting in a low-complexity but high-accuracy extension to the 2.3.5.13 subgroup sometimes known as cata. From S25 × S26 and S25 we can see that S26 = (26/25)/(27/26) = 676/675 = S13/S15 = (16/12)/(15/13)2 is also tempered out, meaning 4/3 is split into two 15/13's and thus 3/1 (from 22/(4/3)) is split into two 26/15's (from 2/(15/13)). From 325/324 = S10/S12 = (13/9)/(12/10)2 we can see that 13/9 is split into two 6/5's, so that it's equated with 36/25; the consequence of this is that the chain of generators naturally gives us hemitwelfths at 3 generator steps of a slightly sharpened ~6/5 because of (6/5)2 × 6/5 ~ 13/9 × 6/5 = 26/15 being half of 3/1 as discussed.
Extensions with prime 7 include catakleismic (which adds 225/224, finding 7 at 22 generators up), countercata (which adds 5120/5103, finding 7 at 31 generators down), metakleismic (which adds 179200/177147, finding 7 at 56 generators up), keemun (which adds 49/48, finding 7 at 3 generators up), anakleismic (which adds 2240/2187, finding 7 at 37 generators up), and catalan (which adds 64/63, finding 7 at 12 generators down). Of these, catakleismic can perhaps be considered the canonical extension, as it makes an intuitive further equivalence of 25/24~26/25~27/26 to 28/27 (by tempering out the square superparticular S27 in addition to S25 and S26), and can be defined independently in the 7-limit by tempering out 225/224 and 4375/4374. However, countercata is well-tuned closer to the optimal range of kleismic (between 53edo and 87edo), especially that of 2.3.5.13 cata, and naturally emerges in that context, identifying 64/63 with 65/64 by tempering out 4096/4095. Catakleismic and countercata merge in 53edo, as the former finds 7 at 22 generators up while the latter finds it at 31 generators down (22 + 31 = 53).
Most of these extensions can also incorporate prime 11 (and thereby reach the full 13-limit) by tempering out 385/384, equating the ~6/5 generator to 77/64. This works well since the optimal tunings of cata's ~6/5 are usually intermediate between just 6/5 (just flat of 19edo) and 77/64 (just sharp of 15edo).
For technical data, see Kleismic family #Kleismic a.k.a. hanson.
Interval chain
In the following table, odd harmonics 1–15 are labeled in bold.
| # | Cents* | Approximate ratios |
|---|---|---|
| 0 | 0.0 | 1/1 |
| 1 | 317.1 | 6/5, 65/54 |
| 2 | 634.2 | 13/9, 36/25 |
| 3 | 950.3 | 26/15, 45/26 |
| 4 | 68.4 | 25/24, 26/25, 27/26 |
| 5 | 385.6 | 5/4, 81/65 |
| 6 | 702.7 | 3/2 |
| 7 | 1019.8 | 9/5, 65/36 |
| 8 | 136.9 | 13/12, 27/25 |
| 9 | 454.0 | 13/10 |
| 10 | 771.1 | 25/16, 39/25, 81/52 |
| 11 | 1088.2 | 15/8 |
| 12 | 205.3 | 9/8 |
| 13 | 522.4 | 27/20, 65/48 |
| 14 | 839.6 | 13/8, 81/50 |
| 15 | 1156.7 | 39/20 |
| 16 | 273.8 | 75/64 |
| 17 | 590.9 | 45/32 |
| 18 | 908.0 | 27/16 |
| 19 | 25.1 | 65/64, 81/80 |
* In 2.3.5.13-subgroup CTE tuning
Tunings
Optimized tunings
| Weight-skew\Order | Euclidean | |
|---|---|---|
| Constrained | Destretched | |
| Tenney | (2.3.5) CTE: ~6/5 = 317.0308¢ | (2.3.5) POTE: ~6/5 = 317.007¢ |
| Equilateral | (2.3.5) CEE: ~6/5 = 317.1033¢
(11/61-kleisma) | |
| Tenney | (2.3.5.13) CTE: ~6/5 = 317.1110¢ | (2.3.5.13) POTE: ~6/5 = 317.0756¢ |
| Optimized chord | Generator value | Polynomial | Further notes |
|---|---|---|---|
| 3:4:5 (+1 +1) | ~6/5 = 317.1496 | g6 + 2g5 − 8 = 0 | 1 – 3 – 5 equal-beating tuning, close to 8/43-kleisma |
| 4:5:6 (+1 +1) | ~6/5 = 317.9593 | g6 − 2g5 + 2 = 0 | 1 – 3 – 5 equal-beating tuning, close to 2/7-kleisma |
| 10:12:15 (+2 +3) | ~6/5 = 317.6675 | g6 − 5g + 3 = 0 | Close to 1/4-kleisma |
| 9:13:15 (+2 +1) | ~6/5 = 317.5679 | 3g3 + 4g − 10 = 0 | Close to 13/36-marveltwin comma |
| 13:15:18 (+2 +3) | ~6/5 = 317.0010 | 3g3 − g − 4 = 0 | Close to 13/51-marveltwin comma |
Tuning spectrum
| EDO generator |
Eigenmonzo (unchanged interval)* |
Generator (¢) | Comments |
|---|---|---|---|
| 6/5 | 315.6413 | Untempered tuning, lower bound of 5-odd-limit diamond tradeoff | |
| 5\19 | 315.7895 | Lower bound of 2.3.5.13-subgroup 15-odd-limit diamond monotone | |
| 27/26 | 316.3343 | 1/4-tunbarsma | |
| 29\110 | 316.3636 | 110ff val | |
| 24\91 | 316.4835 | 91f val | |
| 27/25 | 316.6547 | 1/8-kleisma | |
| 19\72 | 316.6667 | ||
| 9/5 | 316.7995 | 1/7-kleisma | |
| 33\125 | 316.8000 | 125f val | |
| 26/25 | 316.9750 | 1/4-marveltwin comma | |
| 14\53 | 316.9811 | ||
| 3/2 | 316.9925 | 1/6-kleisma; 5- and 9-odd-limit minimax tuning | |
| 65\246 | 317.0732 | ||
| 51\193 | 317.0984 | ||
| 15/8 | 317.1153 | 2/11-kleisma | |
| 13/10 | 317.1349 | 13- and 15-odd-limit minimax tuning | |
| 37\140 | 317.1429 | ||
| 13/8 | 317.1805 | ||
| 60\227 | 317.1807 | ||
| 23\87 | 317.2414 | ||
| 5/4 | 317.2627 | 1/5-kleisma, upper bound of 5-odd-limit diamond tradeoff | |
| 13/12 | 317.3216 | ||
| 32\121 | 317.3554 | ||
| 41\155 | 317.4194 | ||
| 15/13 | 317.4197 | 1/3-marveltwin comma | |
| 9\34 | 317.6471 | ||
| 25/24 | 317.6681 | 1/4-kleisma, virtually DR 10:12:15 | |
| 22\83 | 318.0723 | 83f val | |
| 13/9 | 318.3088 | 1/2-marveltwin comma, upper bound of 2.3.5.13-subgroup 15-odd-limit diamond tradeoff | |
| 125/72 | 318.3437 | 1/3-kleisma | |
| 13\49 | 318.3673 | 49f val | |
| 625/432 | 319.6949 | 1/2-kleisma | |
| 4\15 | 320.0000 | Upper bound of 2.3.5.13-subgroup 15-odd-limit diamond monotone |
* Besides the octave
Other tunings
- DKW (2.3.5): ~2 = 1\1, ~6/5 = 317.1983
Scales
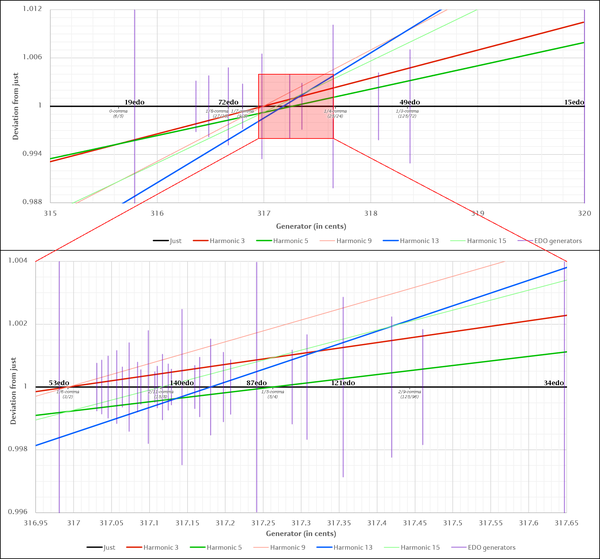
Images
A chart of the tuning spectrum of hanson and cata, showing the offsets of odd harmonics 3, 5, 9, 13, and 15, as a function of the generator; all EDO tunings are shown with vertical lines whose length indicates the EDO's tolerance, i.e. half of its step size in either direction of just, and some small EDOs supporting the temperament are labeled. Comma fractions with corresponding eigenmonzos also labeled.