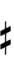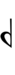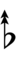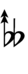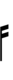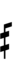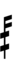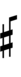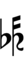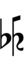51edo

From Xenharmonic Wiki
Jump to navigation Jump to search
← 50edo 51edo 52edo →
Prime factorization 3 × 17
Step size 23.5294 ¢ 
Fifth 30\51 (705.882 ¢) (→ 10\17)
Semitones (A1:m2) 6:3 (141.2 ¢ : 70.59 ¢)
Consistency limit 3
Distinct consistency limit 3

51 equal divisions of the octave (abbreviated 51edo or 51ed2), also called 51-tone equal temperament (51tet) or 51 equal temperament (51et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 51 equal parts of about 23.5 ¢ each. Each step represents a frequency ratio of 21/51, or the 51st root of 2.

Theory

Since 51 = 3 × 17, 51edo shares its fifth with 17edo. Compared to other multiples of 17edo, notably 34edo and 68edo, 51edo's harmonic inventory seems lacking, getting few harmonics very well considering its step size. However, it does possess excellent approximations of 11/10 and 21/16, only about 0.3 cents off in each case.

Using the patent val, 51et tempers out 250/243 in the 5-limit, 225/224 and 2401/2400 in the 7-limit, and 55/54 and 100/99 in the 11-limit. It is the optimal patent val for sonic, the rank-3 temperament tempering out 55/54, 100/99, and 250/243, and also for the rank-4 temperament tempering out 55/54. It provides an alternative tuning to 22edo for porcupine, with a nice fifth but a rather flat major third, and the optimal patent val for the 7- and 11-limit porky temperament, which is sonic plus 225/224. 51 contains an archeotonic 6L 1s scale based on repetitions of 8\51, creating a scale with a whole-tone-like drive towards the tonic through the 17edo semitone at the top.

Alternatively, using the 51c val ⟨51 81 119 143], the 5/4 is mapped to 1\3 (400 cents), supporting augmented. In the 7-limit it tempers out 245/243 and supports hemiaug and rodan. The 51cd val ⟨51 81 119 144] takes the same 7/4 from 17edo, and supports augene.

51edo's step is the closest direct approximation to the Pythagorean comma by edo steps, though that comma itself is mapped to a different interval.

Odd harmonics

Approximation of prime harmonics in 51edo
Harmonic 2 3 5 7 11 13 17 19 23 29 31
Error Absolute (¢) +0.0 +3.9 -9.8 -4.1 -10.1 +6.5 -10.8 +8.4 +7.0 +5.7 +7.9
Relative (%) +0.0 +16.7 -41.8 -17.5 -43.1 +27.8 -46.1 +35.6 +29.8 +24.3 +33.6
Steps
(reduced) 		51
(0) 		81
(30) 		118
(16) 		143
(41) 		176
(23) 		189
(36) 		208
(4) 		217
(13) 		231
(27) 		248
(44) 		253
(49)

Subsets and supersets

51edo contains 3edo and 17edo as subsets.

One of the very powerful (but very complex) supersets of 51edo is 612edo, which divides each step of 51edo into 12 equal parts, for which the name "skisma" has been proposed.

Intervals

# Cents Ups and downs notation
0 0.0 Perfect 1sn P1 D
1 23.5 Up 1sn ^1 ^D
2 47.1 Downminor 2nd vm2 vEb
3 70.6 Minor 2nd m2 Eb
4 94.1 Upminor 2nd ^m2 ^Eb
5 117.6 Downmid 2nd v~2 ^^Eb
6 141.2 Mid 2nd ~2 vvvE, ^^^Eb
7 164.7 Upmid 2nd ^~2 vvE
8 188.2 Downmajor 2nd vM2 vE
9 211.8 Major 2nd M2 E
10 235.3 Upmajor 2nd ^M2 ^E
11 258.8 Downminor 3rd vm3 vF
12 282.4 Minor 3rd m3 F
13 305.9 Upminor 3rd ^m3 ^F
14 329.4 Downmid 3rd v~3 ^^F
15 352.9 Mid 3rd ~3 ^^^F, vvvF#
16 376.5 Upmid 3rd ^~3 vvF#
17 400.0 Downmajor 3rd vM3 vF#
18 423.5 Major 3rd M3 F#
19 447.1 Upmajor 3rd ^M3 ^F#
20 470.6 Down 4th v4 vG
21 494.1 Perfect 4th P4 G
22 517.6 Up 4th ^4 ^G
23 541.2 Downdim 5th vd5 vAb
24 564.7 Dim 5th d5 Ab
25 588.2 Updim 5th ^d5 ^Ab
26 611.8 Downaug 4th vA4 vG#
27 635.3 Aug 4th A4 G#
28 658.8 Upaug 4th ^A4 ^G#
29 682.4 Down 5th v5 vA
30 705.9 Perfect 5th P5 A
31 729.4 Up 5th ^5 ^A
32 752.9 Downminor 6th vm6 vBb
33 776.5 Minor 6th m6 Bb
34 800.0 Upminor 6th ^m6 ^Bb
35 823.5 Downmid 6th v~6 ^^Bb
36 847.1 Mid 6th ~6 vvvB, ^^^Bb
37 870.6 Upmid 6th ^~6 vvB
38 894.1 Downmajor 6th vM6 vB
39 917.6 Major 6th M6 B
40 941.2 Upmajor 6th ^M6 ^B
41 964.7 Downminor 7th vm7 vC
42 988.2 Minor 7th m7 C
43 1011.8 Upminor 7th ^m7 ^C
44 1035.3 Downmid 7th v~7 ^^C
45 1058.8 Mid 7th ~7 ^^^C, vvvC#
46 1082.4 Upmid 7th ^~7 vvC#
47 1105.9 Downmajor 7th vM7 vC#
48 1129.4 Major 7th M7 C#
49 1152.9 Upmajor 7th ^M7 ^C#
50 1176.5 Down 8ve v8 vD
51 1200.0 Perfect 8ve P8 D

Notation

Ups and downs notation

51edo can be notated with ups and downs, spoken as up, dup, trup, dudsharp, downsharp, sharp, upsharp etc. and down, dud, trud, dupflat etc.

Step offset 0 1 2 3 4 5 6 7 8 9 10 11 12
Sharp symbol
         
             
Flat symbol          
             

Half-sharps and half-flats can be used to avoid triple arrows:

Step offset 0 1 2 3 4 5 6 7 8 9 10 11 12
Sharp symbol
 
    
    
    
Flat symbol  
    
    
    

In 51edo, a combination of quarter tone accidentals and arrow accidentals from Helmholtz–Ellis notation can be used.

Step offset 0 1 2 3 4 5 6 7 8 9 10 11 12 13 14
Sharp symbol  
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
Flat symbol  
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 

If double arrows are not desirable, then arrows can be attached to quarter-tone accidentals:

Step offset 0 1 2 3 4 5 6 7 8 9 10 11 12 13
Sharp symbol
 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
Flat symbol  
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 

Ivan Wyschnegradsky's notation

Since a sharp raises by six steps, Wyschnegradsky accidentals borrowed from 72edo can also be used:

Step offset 0 1 2 3 4 5 6 7 8 9 10 11 12 13
Sharp symbol
 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 
  
 		 
Flat symbol  
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 		 
 

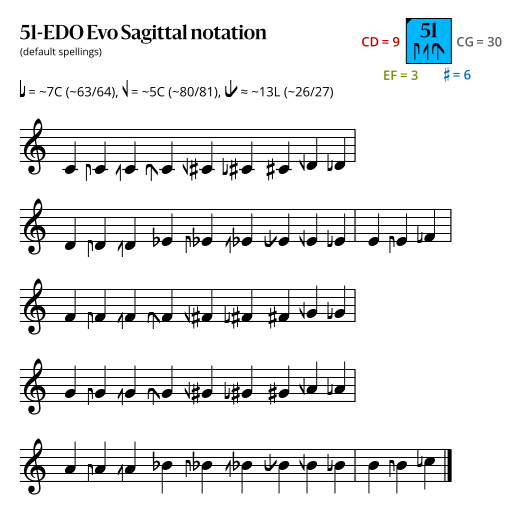
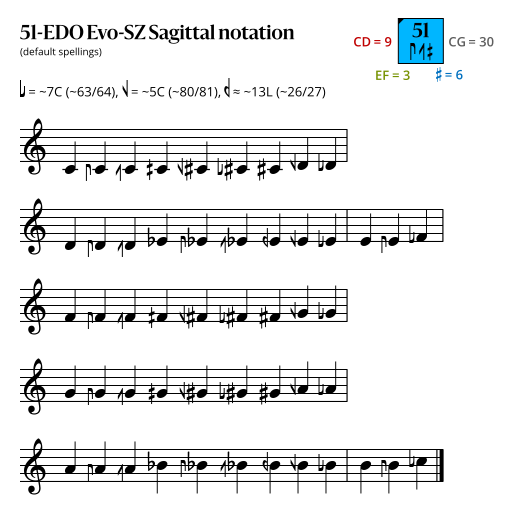
Sagittal notation

In the following diagrams, a sagittal symbol followed by an equals sign (=) means that the following comma is the symbol's primary comma (the comma it exactly represents in JI), while an approximately equals sign (≈) means it is a secondary comma (a comma it approximately represents in JI). In both cases the symbol exactly represents the tempered version of the comma in this edo.

Evo flavor

Sagittal notationPeriodic table of EDOs with sagittal notation64/6381/8027/26

Revo flavor

Sagittal notationPeriodic table of EDOs with sagittal notation64/6381/8027/26

Evo-SZ flavor

Sagittal notationPeriodic table of EDOs with sagittal notation64/6381/8027/26

Regular temperament properties

Subgroup Comma list Mapping Optimal
8ve stretch (¢) 		Tuning error
Absolute (¢) Relative (%)
2.3.7 1029/1024, [17 -16 3⟩ [⟨51 81 143]] −0.339 1.63 6.92
2.3.5 128/125, [-13 17 -6⟩ [⟨51 81 119]] (51c) −2.789 2.41 10.3
2.3.5 250/243, 34171875/33554432 [⟨51 81 118]] (51) +0.581 2.77 11.8

Rank-2 temperaments

Table of rank-2 temperaments by generator
Periods
per 8ve 		Generator* Cents* Associated
ratio* 		Temperament
1 5\51 117.6 15/14 Miracle (51e, out of tune) / oracle (51)
1 7\51 164.7 11/10 Porky (51)
1 10\51 235.3 8/7 Rodan (51cf…, out of tune) / aerodino (51ce)
1 5\51 541.2 15/11 Necromanteion (51ce)
3 19\51
(2\51) 		447.1
(47.1) 		9/7
(36/35) 		Hemiaug (51ce)
3 21\51
(4\51) 		494.1
(94.1) 		4/3
(16/15) 		Augmented (51c)

* Octave-reduced form, reduced to the first half-octave, and minimal form in parentheses if distinct

Scales

  • Porky[7], Palace: 7 7 7 9 7 7 7
  • UFO scale (inflected MOS of Teefs[19]): 2 2 4 1 2 2 2 4 2 5 2 4 4 2 2 1 4 2 2
  • Cosmic scale (subset of UFO scale): 21 9 4 9 8

Instruments

Lumatone
See Lumatone mapping for 51edo.

Music

Bryan Deister
Frédéric Gagné
James Mulvale (FASTFAST)
Ray Perlner
  • Fugue (2023) – for organ in 51edo Porcupine[7] ssssssL "Pandian"
Retrieved from "https://en.xen.wiki/index.php?title=51edo&oldid=195004"