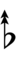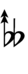74edo
| ← 73edo | 74edo | 75edo → |
74 equal divisions of the octave (abbreviated 74edo or 74ed2), also called 74-tone equal temperament (74tet) or 74 equal temperament (74et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 74 equal parts of about 16.2 ¢ each. Each step represents a frequency ratio of 21/74, or the 74th root of 2.
Theory
74edo is most notable as a meantone tuning, tempering out 81/80 in the 5-limit; 126/125 and 225/224 in the 7-limit; 99/98, 176/175 and 441/440 in the 11-limit; and 144/143 and 847/845 in the 13-limit. Discarding 847/845 from that gives the 13-limit meantone extension grosstone, for which 74edo gives the optimal patent val; and discarding 144/143 gives semimeantone, a 13-limit 62 & 74 temperament with half-octave period and two parallel tracks of meantone.
74edo tunes harmonic 11 only 1/30 of a cent sharp, and 13 2.7 cents sharp, making it a distinctly interesting choice for higher-limit meantone.
Odd harmonics
| Harmonic | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 | 21 | 23 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -4.66 | +2.88 | +4.15 | +6.90 | +0.03 | +2.72 | -1.78 | -7.66 | -5.62 | -0.51 | +4.16 |
| Relative (%) | -28.7 | +17.7 | +25.6 | +42.6 | +0.2 | +16.7 | -11.0 | -47.2 | -34.7 | -3.1 | +25.6 | |
| Steps (reduced) |
117 (43) |
172 (24) |
208 (60) |
235 (13) |
256 (34) |
274 (52) |
289 (67) |
302 (6) |
314 (18) |
325 (29) |
335 (39) | |
Subsets and supersets
Since 74 factors into 2 × 37, 74edo contains 2edo and 37edo as its subsets.
Intervals
| Steps | Cents | Approximate ratios | Ups and downs notation |
|---|---|---|---|
| 0 | 0 | 1/1 | D |
| 1 | 16.2 | ^D, vE♭♭ | |
| 2 | 32.4 | ^^D, E♭♭ | |
| 3 | 48.6 | 34/33, 37/36, 38/37 | vvD♯, ^E♭♭ |
| 4 | 64.9 | 26/25 | vD♯, ^^E♭♭ |
| 5 | 81.1 | 21/20, 22/21 | D♯, vvE♭ |
| 6 | 97.3 | 18/17 | ^D♯, vE♭ |
| 7 | 113.5 | 16/15 | ^^D♯, E♭ |
| 8 | 129.7 | 14/13 | vvD𝄪, ^E♭ |
| 9 | 145.9 | 25/23, 37/34 | vD𝄪, ^^E♭ |
| 10 | 162.2 | 11/10 | D𝄪, vvE |
| 11 | 178.4 | 31/28 | ^D𝄪, vE |
| 12 | 194.6 | 19/17, 28/25, 37/33 | E |
| 13 | 210.8 | 26/23, 35/31 | ^E, vF♭ |
| 14 | 227 | 33/29 | ^^E, F♭ |
| 15 | 243.2 | 23/20, 38/33 | vvE♯, ^F♭ |
| 16 | 259.5 | vE♯, ^^F♭ | |
| 17 | 275.7 | 34/29 | E♯, vvF |
| 18 | 291.9 | 13/11 | ^E♯, vF |
| 19 | 308.1 | F | |
| 20 | 324.3 | 29/24 | ^F, vG♭♭ |
| 21 | 340.5 | 28/23 | ^^F, G♭♭ |
| 22 | 356.8 | 16/13 | vvF♯, ^G♭♭ |
| 23 | 373 | 26/21, 31/25, 36/29 | vF♯, ^^G♭♭ |
| 24 | 389.2 | 5/4 | F♯, vvG♭ |
| 25 | 405.4 | 24/19 | ^F♯, vG♭ |
| 26 | 421.6 | 37/29 | ^^F♯, G♭ |
| 27 | 437.8 | vvF𝄪, ^G♭ | |
| 28 | 454.1 | 13/10 | vF𝄪, ^^G♭ |
| 29 | 470.3 | 21/16, 38/29 | F𝄪, vvG |
| 30 | 486.5 | ^F𝄪, vG | |
| 31 | 502.7 | G | |
| 32 | 518.9 | 31/23 | ^G, vA♭♭ |
| 33 | 535.1 | 15/11 | ^^G, A♭♭ |
| 34 | 551.4 | 11/8 | vvG♯, ^A♭♭ |
| 35 | 567.6 | vG♯, ^^A♭♭ | |
| 36 | 583.8 | 7/5 | G♯, vvA♭ |
| 37 | 600 | 17/12, 24/17 | ^G♯, vA♭ |
| 38 | 616.2 | 10/7 | ^^G♯, A♭ |
| 39 | 632.4 | vvG𝄪, ^A♭ | |
| 40 | 648.6 | 16/11 | vG𝄪, ^^A♭ |
| 41 | 664.9 | 22/15 | G𝄪, vvA |
| 42 | 681.1 | ^G𝄪, vA | |
| 43 | 697.3 | A | |
| 44 | 713.5 | ^A, vB♭♭ | |
| 45 | 729.7 | 29/19, 32/21, 35/23 | ^^A, B♭♭ |
| 46 | 745.9 | 20/13, 37/24 | vvA♯, ^B♭♭ |
| 47 | 762.2 | 31/20 | vA♯, ^^B♭♭ |
| 48 | 778.4 | A♯, vvB♭ | |
| 49 | 794.6 | 19/12 | ^A♯, vB♭ |
| 50 | 810.8 | 8/5 | ^^A♯, B♭ |
| 51 | 827 | 21/13, 29/18 | vvA𝄪, ^B♭ |
| 52 | 843.2 | 13/8 | vA𝄪, ^^B♭ |
| 53 | 859.5 | 23/14 | A𝄪, vvB |
| 54 | 875.7 | ^A𝄪, vB | |
| 55 | 891.9 | B | |
| 56 | 908.1 | 22/13 | ^B, vC♭ |
| 57 | 924.3 | 29/17 | ^^B, C♭ |
| 58 | 940.5 | vvB♯, ^C♭ | |
| 59 | 956.8 | 33/19 | vB♯, ^^C♭ |
| 60 | 973 | B♯, vvC | |
| 61 | 989.2 | 23/13 | ^B♯, vC |
| 62 | 1005.4 | 25/14, 34/19 | C |
| 63 | 1021.6 | ^C, vD♭♭ | |
| 64 | 1037.8 | 20/11 | ^^C, D♭♭ |
| 65 | 1054.1 | vvC♯, ^D♭♭ | |
| 66 | 1070.3 | 13/7 | vC♯, ^^D♭♭ |
| 67 | 1086.5 | 15/8 | C♯, vvD♭ |
| 68 | 1102.7 | 17/9 | ^C♯, vD♭ |
| 69 | 1118.9 | 21/11 | ^^C♯, D♭ |
| 70 | 1135.1 | 25/13 | vvC𝄪, ^D♭ |
| 71 | 1151.4 | 33/17, 37/19 | vC𝄪, ^^D♭ |
| 72 | 1167.6 | C𝄪, vvD | |
| 73 | 1183.8 | ^C𝄪, vD | |
| 74 | 1200 | 2/1 | D |
Notation
Ups and downs notation
32edo can be notated with ups and downs, spoken as up, dup, dudsharp, downsharp, sharp, upsharp etc. and down, dud, dupflat etc. Note that dudsharp is equivalent to trup (triple-up) and dupflat is equivalent to trud (triple-down).
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol |  |
|
|
 
|
|
|
|
|
|
|
|
|
|
| Flat symbol |
|
|
|
|
|
|
|
|
|
|
|
|
Another notation uses alternative ups and downs. It uses sharps and flats with arrows, borrowed from extended Helmholtz–Ellis notation:
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |
 |
 |
 |
 |
|
 |
 |
 |
 |
|
 |
 |
| Flat symbol |  |
 |
 |
 |
|
 |
 |
 |
 |
|
 |
 |
Sagittal notation
Evo flavor
Revo flavor
In the diagrams above, a sagittal symbol followed by an equals sign (=) means that the following comma is the symbol's primary comma (the comma it exactly represents in JI), while an approximately equals sign (≈) means it is a secondary comma (a comma it approximately represents in JI). In both cases the symbol exactly represents the tempered version of the comma in this EDO.
Music
Modern renderings
- Maple Leaf Rag (1899) – rendered by Francium (2024)
- Maple Leaf Rag (1899) – arranged for harpsichord and rendered by Claudi Meneghin (2024)