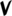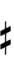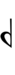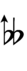69edo

← 68edo

69edo

70edo →

Prime factorization	3 × 23
Step size	17.3913¢
Fifth	40\69 (695.652¢)
Semitones (A1:m2)	4:7 (69.57¢ : 121.7¢)
Dual sharp fifth	41\69 (713.043¢)
Dual flat fifth	40\69 (695.652¢)
Dual major 2nd	12\69 (208.696¢) (→4\23)
Consistency limit	5
Distinct consistency limit	5

69 equal divisions of the octave (abbreviated 69edo or 69ed2), also called 69-tone equal temperament (69tet) or 69 equal temperament (69et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 69 equal parts of about 17.4 ¢ each. Each step represents a frequency ratio of 2^1/69, or the 69th root of 2. Nice.

Theory

69edo has been called "the love-child of 23edo and quarter-comma meantone". As a meantone system, it is on the flat side, with a fifth of 695.652 cents. Such a fifth is closer to 2/7-comma meantone than 1/4-comma, and is nearly identical to that of "Synch-Meantone", or Wilson's equal beating meantone, wherein the perfect fifth and the major third beat at equal rates. Therefore 69edo can be treated as a closed system of Synch-Meantone for most purposes.

69edo offers two kinds of meantone 12-tone scales. One is the raw meantone scale, which has a 7:4 step ratio, and other is period-3 lithium scale, which has a 6:5 step ratio and stems from a temperament tempering out 3125/3087 along with 81/80. It should be noted that while the lithium scale has a meantone fifth, it produces a tcherepnin scale instead of traditional diatonic.

In the 7-limit it is a mohajira system, tempering out 6144/6125, but not a septimal meantone system, as 126/125 maps to one step. In the 11-limit it tempers out 99/98, and supports the 31&69 variant of mohajira, identical to the standard 11-limit mohajira in 31edo but not in 69.

The concoctic scale for 69edo is 22\69, and the corresponding rank two temperament is 22 & 69, defined by tempering out the [-41, 1, 17⟩ comma in the 5-limit.

Odd harmonics

Approximation of odd harmonics in 69edo

Harmonic		3	5	7	9	11	13	15	17	19	21	23
Error	Absolute (¢)	-6.30	-3.71	+5.09	+4.79	+5.20	-5.75	+7.38	-0.61	-1.86	-1.22	-2.19
	Relative (%)	-36.2	-21.3	+29.3	+27.5	+29.9	-33.0	+42.5	-3.5	-10.7	-7.0	-12.6
Steps (reduced)		109 (40)	160 (22)	194 (56)	219 (12)	239 (32)	255 (48)	270 (63)	282 (6)	293 (17)	303 (27)	312 (36)

Intervals

Steps	Cents	Approximate ratios	Ups and downs notation (Dual flat fifth 40\69)	Ups and downs notation (Dual sharp fifth 41\69)
0	0	1/1	D	D
1	17.391		^D, vvE♭♭	^D, vE♭
2	34.783		^^D, vE♭♭	^^D, E♭
3	52.174	32/31, 33/32, 34/33, 35/34	^3D, E♭♭	^3D, v10E
4	69.565	25/24, 26/25	D♯, v3E♭	^4D, v9E
5	86.957	20/19, 21/20	^D♯, vvE♭	^5D, v8E
6	104.348	17/16, 35/33	^^D♯, vE♭	^6D, v7E
7	121.739		^3D♯, E♭	^7D, v6E
8	139.13	13/12	D𝄪, v3E	^8D, v5E
9	156.522	23/21, 34/31, 35/32	^D𝄪, vvE	^9D, v4E
10	173.913	21/19, 31/28, 32/29	^^D𝄪, vE	^10D, v3E
11	191.304	19/17, 29/26	E	D♯, vvE
12	208.696	26/23, 35/31	^E, vvF♭	^D♯, vE
13	226.087	33/29	^^E, vF♭	E
14	243.478	23/20	^3E, F♭	^E, vF
15	260.87		E♯, v3F	F
16	278.261	20/17, 34/29	^E♯, vvF	^F, vG♭
17	295.652	19/16	^^E♯, vF	^^F, G♭
18	313.043	6/5	F	^3F, v10G
19	330.435	23/19, 29/24	^F, vvG♭♭	^4F, v9G
20	347.826		^^F, vG♭♭	^5F, v8G
21	365.217	21/17, 37/30	^3F, G♭♭	^6F, v7G
22	382.609	5/4	F♯, v3G♭	^7F, v6G
23	400	29/23	^F♯, vvG♭	^8F, v5G
24	417.391	14/11	^^F♯, vG♭	^9F, v4G
25	434.783		^3F♯, G♭	^10F, v3G
26	452.174	13/10	F𝄪, v3G	F♯, vvG
27	469.565	21/16	^F𝄪, vvG	^F♯, vG
28	486.957		^^F𝄪, vG	G
29	504.348		G	^G, vA♭
30	521.739	23/17	^G, vvA♭♭	^^G, A♭
31	539.13		^^G, vA♭♭	^3G, v10A
32	556.522	29/21	^3G, A♭♭	^4G, v9A
33	573.913	32/23	G♯, v3A♭	^5G, v8A
34	591.304	31/22	^G♯, vvA♭	^6G, v7A
35	608.696	37/26	^^G♯, vA♭	^7G, v6A
36	626.087	23/16, 33/23	^3G♯, A♭	^8G, v5A
37	643.478	29/20	G𝄪, v3A	^9G, v4A
38	660.87		^G𝄪, vvA	^10G, v3A
39	678.261	34/23, 37/25	^^G𝄪, vA	G♯, vvA
40	695.652		A	^G♯, vA
41	713.043		^A, vvB♭♭	A
42	730.435	29/19, 32/21, 35/23	^^A, vB♭♭	^A, vB♭
43	747.826	20/13, 37/24	^3A, B♭♭	^^A, B♭
44	765.217		A♯, v3B♭	^3A, v10B
45	782.609	11/7	^A♯, vvB♭	^4A, v9B
46	800	35/22	^^A♯, vB♭	^5A, v8B
47	817.391	8/5	^3A♯, B♭	^6A, v7B
48	834.783	34/21	A𝄪, v3B	^7A, v6B
49	852.174		^A𝄪, vvB	^8A, v5B
50	869.565	33/20	^^A𝄪, vB	^9A, v4B
51	886.957	5/3	B	^10A, v3B
52	904.348	32/19	^B, vvC♭	A♯, vvB
53	921.739	17/10, 29/17	^^B, vC♭	^A♯, vB
54	939.13		^3B, C♭	B
55	956.522	33/19	B♯, v3C	^B, vC
56	973.913		^B♯, vvC	C
57	991.304	23/13	^^B♯, vC	^C, vD♭
58	1008.696	34/19	C	^^C, D♭
59	1026.087	29/16	^C, vvD♭♭	^3C, v10D
60	1043.478	31/17	^^C, vD♭♭	^4C, v9D
61	1060.87	24/13, 35/19	^3C, D♭♭	^5C, v8D
62	1078.261		C♯, v3D♭	^6C, v7D
63	1095.652	32/17	^C♯, vvD♭	^7C, v6D
64	1113.043	19/10	^^C♯, vD♭	^8C, v5D
65	1130.435	25/13	^3C♯, D♭	^9C, v4D
66	1147.826	31/16, 33/17	C𝄪, v3D	^10C, v3D
67	1165.217		^C𝄪, vvD	C♯, vvD
68	1182.609		^^C𝄪, vD	^C♯, vD
69	1200	2/1	D	D

Proposed names

Degree	Carmen's naming system	Cents	Approximate Ratios*	Error (abs, ¢)
0	Natural Unison, 1	0.000	1/1	0.000
1	Ptolemy's comma	17.391	100/99	-0.008
2	Jubilisma, lesser septimal sixth tone	34.783	50/49, 101/99	-0.193, 0.157
3	lesser septendecimal quartertone, _____	52.174	34/33, 101/98	0.491, -0.028
4	_____	69.565	76/73	-0.158
5	Small undevicesimal semitone	86.957	20/19	-1.844
6	Large septendecimal semitone	104.348	17/16	-0.608
7	Septimal diatonic semitone	121.739	15/14	2.296
8	Tridecimal neutral second	139.130	13/12	0.558
9	Vicesimotertial neutral second	156.522	23/21	-0.972
10	Undevicesimal large neutral second, undevicesimal whole tone	173.913	21/19	0.645
11	Quasi-meantone	191.304	19/17	-1.253
12	Whole tone	208.696	9/8	4.786
13	Septimal whole tone	226.087	8/7	-5.087
14	Vicesimotertial semifourth	243.478	23/20	1.518
15	Subminor third, undetricesimal subminor third	260.870	7/6, 29/25	-6.001, 3.920
16	Vicesimotertial subminor third	278.261	27/23	0.670
17	Pythagorean minor third	295.652	32/27	1.517
18	Classic minor third	313.043	6/5	-2.598
19	Vicesimotertial supraminor third	330.435	23/19	-0.327
20	Undecimal neutral third	347.826	11/9	0.418
21	Septendecimal submajor third	365.217	21/17	-0.608
22	Classic major third	382.609	5/4	-3.705
23	Undetricesimal major third, Septendecimal major third	400.000	29/23, 34/27	-1.303, 0.910
24	Undecimal major third	417.391	14/11	-0.117
25	Supermajor third	434.783	9/7	-0.301
26	Barbados third	452.174	13/10	-2.040
27	Septimal sub-fourth	469.565	21/16	-1.216
28	_____	486.957	53/40	-0.234
29	Just perfect fourth	504.348	4/3	6.303
30	Vicesimotertial acute fourth	521.739	23/17	-1.580
31	Undecimal augmented fourth	539.130	15/11	2.180
32	Undecimal superfourth, undetricesimal superfourth	556.522	11/8, 29/21	5.204, -2.275
33	Narrow tritone, classic augmented fourth	573.913	7/5, 25/18	-8.600, 5.196
34	_____	591.304	31/22	-2.413
35	High tritone, undevicesimal tritone	608.696	10/7, 27/19	-8.792, 0.344
36	_____	626.087	33/23	1.088
37	Undetricesimal tritone	643.478	29/20	0.215
38	Undevicesimal diminished fifth, undecimal diminished fifth	660.870	19/13, 22/15	3.884, -2.180
39	Vicesimotertial grave fifth, _____	678.261	34/23, 37/25	1.580, -0.456
40	Just perfect fifth	695.652	3/2	-6.303
41	_____	713.043	80/53	0.234
42	Super-fifth, undetricesimal super-fifth	730.435	32/21, 29/19	1.216, -1.630
43	Septendecimal subminor sixth	747.826	17/11	-5.811
44	Subminor sixth	765.217	14/9	0.301
45	Undecimal minor sixth	782.609	11/7	0.117
46	Septendecimal subminor sixth	800.000	27/17	-0.910
47	Classic minor sixth	817.391	8/5	3.705
48	Septendecimal supraminor sixth	834.783	34/21	0.608
49	Undecimal neutral sixth	852.174	18/11	-0.418
50	Vicesimotertial submajor sixth	869.565	38/23	0.327
51	Classic major sixth	886.957	5/3	2.598
52	Pythagorean major sixth	904.348	27/16	-1.517
53	Septendecimal major sixth, undetricesimal major sixth	921.739	17/10, 29/17	3.097, -2.883
54	Supermajor sixth, undetricesimal supermajor sixth	939.130	12/7, 50/29	6.001, -3.920
55	Vicesimotertial supermajor sixth	956.522	40/23	-1.518
56	Harmonic seventh	973.913	7/4	5.087
57	Pythagorean minor seventh	991.304	16/9	-4.786
58	Quasi-meantone minor seventh	1008.696	34/19	1.253
59	Minor neutral undevicesimal seventh	1026.087	38/21	-0.645
60	Vicesimotertial neutral seventh	1043.478	42/23	0.972
61	Tridecimal neutral seventh	1060.870	24/13	-0.558
62	Septimal diatonic major seventh	1078.261	28/15	-2.296
63	Small septendecimal major seventh	1095.652	32/17	0.608
64	Small undevicesimal semitone	1113.043	20/19	1.844
65	_____	1130.435	73/38	0.158
66	Septendecimal supermajor seventh	1147.826	33/17	-0.491
67	_____	1165.217	49/25	-0.193
68	_____	1182.609	99/50	0.008
69	Octave, 8	1200.000	2/1	0.000

*some simpler ratios listed

Regular temperament properties

Subgroup	Comma List	Mapping	Optimal 8ve Stretch (¢)	Tuning Error
				Absolute (¢)	Relative (%)
2.3	[-109 69⟩	[⟨69 109]]	+1.99	1.99	11.43
2.3.5	81/80, [-41 1 17⟩	[⟨69 109 160]]	+1.86	1.64	9.40
2.3.5.7	81/80, 126/125, 4117715/3981312	[⟨69 109 160 193]] (69d)	+2.49	1.79	10.28
2.3.5.7	81/80, 3125/3087, 6144/6125	[⟨69 109 160 194]] (69)	+0.94	2.13	12.23

Rank 2 temperaments

Periods per 8ve	Generator	Temperaments
1	2\69	Gammy (69de)
1	19\69	Rarity
1	20\69	Mohaha (69e)
1	22\69	Caleb (69) marveltri (69)
1	29\69	Meantone (69d)
3	5\69	Ogene (69bceef)
3	6\69	August (7-limit, 69cdd) Lithium (69)
3	9\69	Nessafof (69e)

Scales

• Supermajor[11], 3L 8s – 6 6 6 7 6 6 6 7 6 6 7
• Meantone[7], 5L 2s (gen = 40\69) – 11 11 7 11 11 11 7
• Meantone[12], 7L 5s (gen = 40\69) – 7 4 7 4 7 4 7 7 4 7 4 7
• Lithium[9], 3L 6s – 11 6 6 11 6 6 11 6 6
• Lithium[12], 9L 3s – 5 6 6 6 5 6 6 6 5 6 6 6

Music

Eliora

• Hypergiant Sakura (2021)

Francium

• 69 hours before (2023)