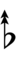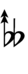67edo
| ← 66edo | 67edo | 68edo → |
67 equal divisions of the octave (abbreviated 67edo or 67ed2), also called 67-tone equal temperament (67tet) or 67 equal temperament (67et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 67 equal parts of about 17.9 ¢ each. Each step represents a frequency ratio of 21/67, or the 67th root of 2.
Theory
67edo tempers out 81/80, supporting meantone, with a tuning which is slightly sharp of 1/6-comma (the tuning favored by Mozart and contemporaries, though they suggested the flatter and composite 55edo as an approximation). It is indistinguishable from 4⁄25 = 0.16-comma meantone. In the 7-limit the patent val tempers out 1029/1024 and 1728/1715, so that it supports mothra. In the 11-limit it tempers out 176/175 and 540/539, supporting mosura, an alternative 11-limit mothra. In the 13-limit it tempers out 144/143 and 196/195, supporting 13-limit mosura. It tempers out the orgonisma, and on the 2.7.11 subgroup it supports the orgone temperament.
It is a promising tuning which has, as many relatively large equal temperaments do, a variety of tonal resources: it is the second edo after 26edo to have both meantone and an orgone temperament. It has relatively good approximations of the 3rd, 7th, 11th, 13th, 15th, 17th harmonics, although the 5th, 9th, and 19th as well as certain higher ones are workable as well. 33 + 34 can be used to construct this temperament explaining some of its properties. It does well on the 2.3.7.11.13.17.23.31.37.41 subgroup.
Prime harmonics
| Harmonic | 2 | 3 | 5 | 7 | 11 | 13 | 17 | 19 | 23 | 29 | 31 | 37 | 41 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | +0.00 | -3.45 | +7.72 | -1.66 | +3.91 | +1.26 | +2.51 | +6.96 | -1.41 | -8.68 | +1.23 | -0.60 | +0.79 |
| Relative (%) | +0.0 | -19.2 | +43.1 | -9.3 | +21.8 | +7.1 | +14.0 | +38.9 | -7.9 | -48.5 | +6.9 | -3.3 | +4.4 | |
| Steps (reduced) |
67 (0) |
106 (39) |
156 (22) |
188 (54) |
232 (31) |
248 (47) |
274 (6) |
285 (17) |
303 (35) |
325 (57) |
332 (64) |
349 (14) |
359 (24) | |
Subsets and supersets
67edo is the 19th prime edo, following 61edo and before 71edo.
Intervals
| Steps | Cents | Approximate ratios | Ups and downs notation |
|---|---|---|---|
| 0 | 0 | 1/1 | D |
| 1 | 17.9 | ^D, E♭♭ | |
| 2 | 35.8 | ^^D, ^E♭♭ | |
| 3 | 53.7 | 31/30, 32/31, 33/32, 34/33, 35/34 | vvD♯, ^^E♭♭ |
| 4 | 71.6 | 24/23 | vD♯, vvE♭ |
| 5 | 89.6 | 20/19 | D♯, vE♭ |
| 6 | 107.5 | 17/16, 33/31 | ^D♯, E♭ |
| 7 | 125.4 | 14/13, 29/27 | ^^D♯, ^E♭ |
| 8 | 143.3 | vvD𝄪, ^^E♭ | |
| 9 | 161.2 | 11/10, 23/21, 34/31 | vD𝄪, vvE |
| 10 | 179.1 | 31/28 | D𝄪, vE |
| 11 | 197 | E | |
| 12 | 214.9 | 17/15, 26/23 | ^E, F♭ |
| 13 | 232.8 | 8/7 | ^^E, ^F♭ |
| 14 | 250.7 | 15/13, 22/19 | vvE♯, ^^F♭ |
| 15 | 268.7 | 7/6 | vE♯, vvF |
| 16 | 286.6 | 13/11, 33/28 | E♯, vF |
| 17 | 304.5 | 31/26 | F |
| 18 | 322.4 | ^F, G♭♭ | |
| 19 | 340.3 | 28/23 | ^^F, ^G♭♭ |
| 20 | 358.2 | 16/13 | vvF♯, ^^G♭♭ |
| 21 | 376.1 | 36/29 | vF♯, vvG♭ |
| 22 | 394 | F♯, vG♭ | |
| 23 | 411.9 | 19/15, 33/26 | ^F♯, G♭ |
| 24 | 429.9 | ^^F♯, ^G♭ | |
| 25 | 447.8 | 22/17 | vvF𝄪, ^^G♭ |
| 26 | 465.7 | 17/13 | vF𝄪, vvG |
| 27 | 483.6 | F𝄪, vG | |
| 28 | 501.5 | 4/3 | G |
| 29 | 519.4 | 23/17, 31/23 | ^G, A♭♭ |
| 30 | 537.3 | 15/11 | ^^G, ^A♭♭ |
| 31 | 555.2 | 11/8, 29/21 | vvG♯, ^^A♭♭ |
| 32 | 573.1 | 32/23 | vG♯, vvA♭ |
| 33 | 591 | 31/22 | G♯, vA♭ |
| 34 | 609 | ^G♯, A♭ | |
| 35 | 626.9 | 23/16, 33/23 | ^^G♯, ^A♭ |
| 36 | 644.8 | 16/11 | vvG𝄪, ^^A♭ |
| 37 | 662.7 | 22/15 | vG𝄪, vvA |
| 38 | 680.6 | 34/23 | G𝄪, vA |
| 39 | 698.5 | 3/2 | A |
| 40 | 716.4 | ^A, B♭♭ | |
| 41 | 734.3 | 26/17 | ^^A, ^B♭♭ |
| 42 | 752.2 | 17/11 | vvA♯, ^^B♭♭ |
| 43 | 770.1 | vA♯, vvB♭ | |
| 44 | 788.1 | 30/19 | A♯, vB♭ |
| 45 | 806 | 35/22 | ^A♯, B♭ |
| 46 | 823.9 | 29/18 | ^^A♯, ^B♭ |
| 47 | 841.8 | 13/8 | vvA𝄪, ^^B♭ |
| 48 | 859.7 | 23/14 | vA𝄪, vvB |
| 49 | 877.6 | A𝄪, vB | |
| 50 | 895.5 | B | |
| 51 | 913.4 | 22/13 | ^B, C♭ |
| 52 | 931.3 | 12/7 | ^^B, ^C♭ |
| 53 | 949.3 | 19/11, 26/15 | vvB♯, ^^C♭ |
| 54 | 967.2 | 7/4 | vB♯, vvC |
| 55 | 985.1 | 23/13, 30/17 | B♯, vC |
| 56 | 1003 | C | |
| 57 | 1020.9 | ^C, D♭♭ | |
| 58 | 1038.8 | 20/11, 31/17 | ^^C, ^D♭♭ |
| 59 | 1056.7 | 35/19 | vvC♯, ^^D♭♭ |
| 60 | 1074.6 | 13/7 | vC♯, vvD♭ |
| 61 | 1092.5 | 32/17 | C♯, vD♭ |
| 62 | 1110.4 | 19/10 | ^C♯, D♭ |
| 63 | 1128.4 | 23/12 | ^^C♯, ^D♭ |
| 64 | 1146.3 | 31/16, 33/17 | vvC𝄪, ^^D♭ |
| 65 | 1164.2 | vC𝄪, vvD | |
| 66 | 1182.1 | C𝄪, vD | |
| 67 | 1200 | 2/1 | D |
Notation
Ups and downs notation
67edo can be notated with ups and downs, spoken as up, dup, dudsharp, downsharp, sharp, upsharp etc. and down, dud, dupflat etc. Note that dudsharp is equivalent to trup (triple-up) and dupflat is equivalent to trud (triple-down).
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |||||||||||||
| Flat symbol |
Another notation uses alternative ups and downs. Here, this can be done using sharps and flats with arrows, borrowed from extended Helmholtz–Ellis notation:
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |||||||||||||
| Flat symbol |
Sagittal notation
Evo flavor

Revo flavor

In the diagrams above, a sagittal symbol followed by an equals sign (=) means that the following comma is the symbol's primary comma (the comma it exactly represents in JI), while an approximately equals sign (≈) means it is a secondary comma (a comma it approximately represents in JI). In both cases the symbol exactly represents the tempered version of the comma in this EDO.
Scales
Mos scales
- Meantone[5]: 11 11 17 11 17
- Meantone[7]: 11 11 6 11 11 11 6
- Barbados[5], Bustling Docks (original/default tuning): 14 14 11 14 14
- Barbados[9]: 11 3 11 3 11 3 11 3 11
Modmos scales
- Cavernous (original/default tuning): 14 14 11 21 7
- Formicarium (original/default tuning): 14 7 18 14 14
- Negri Blues (original/default tuning): 14 14 3 8 14 14
- Negri Blues Septatonic (original/default tuning): 14 14 3 8 11 3 14
- Negri Blues Octatonic (original/default tuning): 7 14 7 11 7 11 3 7
- Understory (original/default tuning): 14 7 18 7 21
- Meantone Ionian Pentatonic: 22 6 11 22 6
- Meantone Minor Melodic: 11 6 11 11 11 11 6
- Meantone Minor Harmonic: 11 6 11 11 6 16 6
- Meantone Minor Hexatonic: 11 6 11 11 17 11
- Meantone Dorian Harmonic: 11 6 16 6 11 6 11
- Meantone Mixolydian Pentatonic: 22 6 11 17 11
- Meantone Phrygian Dominant: 6 16 6 11 6 11 11
- Meantone Phrygian Dominant Hexatonic: 6 16 6 11 6 22
- Meantone Phrygian Dominant Pentatonic: 22 6 11 6 22
- Meantone Phrygian Pentatonic: 6 11 22 6 22
- Meantone Double Harmonic: 6 16 6 11 6 16 6
Blues scales
- Lost spirit (approximated from 31edo): 17 11 6 5 13 4 11
- Blackened skies (approximated from 72edo): 18 10 5 6 5 18 5
- Blues Aeolian Hexatonic: 17 11 6 5 6 22
- Blues Aeolian Pentatonic I: 17 11 11 6 22
- Blues Aeolian Pentatonic II: 17 22 6 11 11
- Blues Bright Double Harmonic: 6 16 6 11 6 11 6 5
- Blues Dark Double Harmonic: 11 6 11 6 5 6 16 6
- Blues Dorian Hexatonic: 17 11 11 11 6 11
- Blues Dorian Pentatonic: 17 22 11 6 11
- Blues Dorian Septatonic: 17 11 6 5 11 6 11
- Blues Harmonic Hexatonic: 11 6 11 11 22 6
- Blues Harmonic Septatonic: 17 11 6 5 6 11 5 6
- Blues Leading: 17 11 6 5 17 6 5
- Blues Minor: 17 11 6 5 17 11
- Blues Minor Maj7: 17 11 6 5 22 6
- Blues Pentachordal: 11 6 11 5 6 28
- Greyed Skies (approximated from 91edo): 17 11 5 6 6 17 5
- Akebono I: 11 6 11 11 17
- Augmented: 17 6 16 6 16 6
- Dominant Pentatonic: 11 11 17 17 11
- Hirajoshi: 11 6 12 6 22
- Javanese Pentachordal: 6 11 17 4 29
Others
- Approximation of Pelog lima: 6 10 22 7 22
- Arcade (approximated from 32afdo): 22 4 13 15 13
- Cosmic (approximated from 32afdo): 29 10 6 11 11
- Mechanical (approximated from 16afdo): 17 5 17 15 13
- Moonbeam (approximated from 16afdo): 11 6 12 22 6
- Springwater (approximated from 8afdo): 11 11 17 15 13
- Volcanic (approximated from 16afdo): 6 16 17 15 13
- Deja Vu (approximated from 101afdo): 18 21 6 12 10
- Freeway (approximated from 6afdo): 15 12 11 11 9 8
- Mushroom (approximated from 30afdo): 15 12 11 4 24
- Underpass (approximated from 10afdo): 18 21 12 6 10
- Sourgummy (approximated from 51afdo): 14 12 14 14 13
- Bubblegum/Cola (approximated from 60afdo/99afdo): 14 13 13 13 14
- Spearmint/Whitechocolate (approximated from 62afdo/90afdo): 13 14 13 14 13
- Lemonade (approximated from 79afdo): 14 13 13 14 13
- Candycorn (approximated from 91afdo): 11 12 11 10 12 11
- Trailmix (approximated from 97afdo): 11 11 11 12 11 11
- Liquorice (approximated from 101afdo): 11 11 12 10 12 11
- Apple Mint (approximated from 80afdo): 9 11 9 9 10 9 10
Instruments
Music
- Beginning of a piece in 67 tone (2011) [dead link]