28edo
| ← 27edo | 28edo | 29edo → |
28 equal divisions of the octave (abbreviated 28edo or 28ed2), also called 28-tone equal temperament (28tet) or 28 equal temperament (28et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 28 equal parts of about 42.9 ¢ each. Each step represents a frequency ratio of 21/28, or the 28th root of 2.
Theory
| Harmonic | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 | 21 | 23 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -16.2 | -0.6 | +16.9 | +10.4 | +5.8 | +16.6 | -16.8 | -19.2 | +2.5 | +0.6 | +14.6 |
| Relative (%) | -37.9 | -1.4 | +39.4 | +24.2 | +13.6 | +38.8 | -39.3 | -44.9 | +5.8 | +1.5 | +34.0 | |
| Steps (reduced) |
44 (16) |
65 (9) |
79 (23) |
89 (5) |
97 (13) |
104 (20) |
109 (25) |
114 (2) |
119 (7) |
123 (11) |
127 (15) | |
| Harmonic | 25 | 27 | 29 | 31 | 33 | 35 | 37 | 39 | 41 | 43 | 45 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -1.2 | -5.9 | -1.0 | +12.1 | -10.4 | +16.3 | +5.8 | +0.4 | -0.5 | +2.8 | +9.8 |
| Relative (%) | -2.8 | -13.7 | -2.3 | +28.3 | -24.3 | +38.0 | +13.5 | +0.9 | -1.1 | +6.5 | +22.8 | |
| Steps (reduced) |
130 (18) |
133 (21) |
136 (24) |
139 (27) |
141 (1) |
144 (4) |
146 (6) |
148 (8) |
150 (10) |
152 (12) |
154 (14) | |
28edo is a multiple of both 7edo and 14edo (and of course 2edo and 4edo). It shares three intervals with 12edo: the 300 cent minor third, the 600 cent tritone, and the 900 cent major sixth. Thus it tempers out the greater diesis 648:625. It does not however temper out the 128:125 lesser diesis, as 28 is not divisible by 3. It has the same perfect fourth and fifth as 7edo. It also has decent approximations of several septimal intervals, of which 9/7 and its inversion 14/9 are also found in 14edo. Its approximation to 5/4 is unusually good for an edo of this size, being the next convergent to log25 after 3edo.
28edo can approximate the 7-limit subgroup 2.27.5.21 quite well, and on this subgroup it has the same commas and tunings as 84edo. The temperament corresponding to orwell temperament now has a major third as generator, though as before 225/224, 1728/1715 and 6144/6125 are tempered out. The 225/224-tempered version of the augmented triad has a very low complexity, so many of them appear in the MOS scales for this temperament, which have sizes 7, 10, 13, 16, 19, 22, 25.
Another subgroup for which 28edo works quite well is 2.5.11.19.21.27.29.39.41.
28edo is the 2nd perfect number EDO.
Intervals
The following table compares it to potentially useful nearby just intervals.
| Step # | ET (e) | Just (j) | Delta (e-j) |
Ups and downs notation | |||
|---|---|---|---|---|---|---|---|
| Cents | Interval | Cents | |||||
| 0 | 0.00 | 1/1 | 0.00 | 0.00 | unison | 1 | D |
| 1 | 42.86 | 41/40 | 42.74 | 0.12 | up-unison | ^1 | ^D |
| 2 | 85.71 | 21/20 | 84.47 | 1.24 | dup 1sn, dud 2nd | ^^1, vv2 | ^^D, vvE |
| 3 | 128.57 | 14/13 | 128.30 | 0.27 | down 2nd | v2 | vE |
| 4 | 171.43 | 11/10 | 165.00 | 6.43 | 2nd | 2 | E |
| 5 | 214.29 | 17/15 | 216.69 | -2.40 | up 2nd | ^2 | ^E |
| 6 | 257.14 | 7/6 | 266.87 | -9.73 | dup 2nd, dud 3rd | ^^2, vv3 | ^^E, vvF |
| 7 | 300.00 | 6/5 | 315.64 | -15.64 | down 3rd | v3 | vF |
| 8 | 342.86 | 11/9 | 347.41 | -4.55 | 3rd | 3 | F |
| 9 | 385.71 | 5/4 | 386.31 | -0.60 | up 3rd | ^3 | ^F |
| 10 | 428.57 | 9/7 | 435.08 | -6.51 | dup 3rd, dud 4th | ^^3, vv4 | ^^F, vvG |
| 11 | 471.43 | 21/16 | 470.78 | 0.65 | down 4th | v4 | vG |
| 12 | 514.29 | 4/3 | 498.04 | 16.25 | 4th | 4 | G |
| 13 | 557.14 | 11/8 | 551.32 | 5.82 | up 4th | ^4 | ^G |
| 14 | 600.00 | 7/5 | 582.51 | 17.49 | dup 4th, dud 5th | ^^4, vv5 | ^^G, vvA |
| 15 | 642.86 | 16/11 | 648.68 | -5.82 | down 5th | v5 | vA |
| 16 | 685.71 | 3/2 | 701.96 | -16.25 | 5th | 5 | A |
| 17 | 728.57 | 32/21 | 729.22 | -0.65 | up 5th | ^5 | ^A |
| 18 | 771.43 | 14/9 | 764.92 | 6.51 | dup 5th, dud 6th | ^^5, vv6 | ^^A, vvB |
| 19 | 814.29 | 8/5 | 813.68 | 0.61 | down 6th | v6 | vB |
| 20 | 857.14 | 18/11 | 852.59 | 4.55 | 6th | 6 | B |
| 21 | 900.00 | 5/3 | 884.36 | 15.64 | up 6th | ^6 | ^B |
| 22 | 942.86 | 12/7 | 933.13 | 9.73 | dup 6th, dud 7th | ^^6, vv7 | ^^B, vvC |
| 23 | 985.71 | 30/17 | 983.31 | 2.40 | down 7th | v7 | vC |
| 24 | 1028.57 | 20/11 | 1035.00 | -6.43 | 7th | 7 | C |
| 25 | 1071.42 | 13/7 | 1071.70 | -0.27 | up 7th | ^7 | ^C |
| 26 | 1114.29 | 40/21 | 1115.53 | -1.24 | dup 7th, dud 8ve | ^^7, vv8 | ^^C, vvD |
| 27 | 1157.14 | 80/41 | 1157.26 | -0.12 | down 8ve | v8 | vD |
| 28 | 1200.00 | 2/1 | 1200.00 | 0.00 | 8ve | 8 | D |
Notation
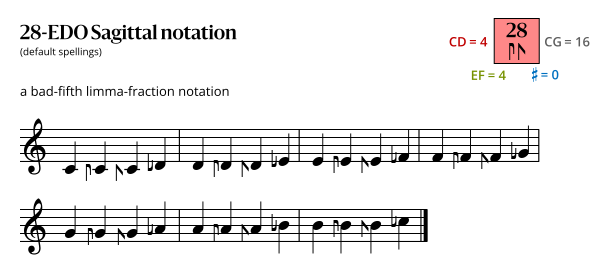
Sagittal notation
This notation uses the same sagittal sequence as EDOs 23 and 33, and is a superset of the notations for EDOs 14 and 7.
Chord names
Ups and downs can be used to name 28edo chords. Because every interval is perfect, the quality can be omitted, and the words major, minor, augmented and diminished are never used.
- 0-8-16 = C E G = C = C or C perfect
- 0-7-16 = C vE G = Cv = C down
- 0-9-16 = C ^E G = C^ = C up
- 0-8-15 = C E vG = C(v5) = C down-five
- 0-9-17 = C ^E ^G = C^(^5) = C up up-five
- 0-8-16-24 = C E G B = C7 = C seven
- 0-8-16-23 = C E G vB = C,v7 = C add down-seven
- 0-7-16-24 = C vE G B = Cv,7 = C down add seven
- 0-7-16-23 = C vE G vB = Cv7 = C down-seven
For a more complete list, see Ups and downs notation #Chord names in other EDOs.
Regular temperament properties
Rank-2 temperaments
| Periods per 8ve |
Generator | Temperaments |
|---|---|---|
| 1 | 1\28 | |
| 1 | 3\28 | Negri |
| 1 | 5\28 | Machine |
| 1 | 9\28 | Worschmidt |
| 1 | 11\28 | A-team |
| 1 | 13\28 | Thuja |
| 2 | 1\28 | |
| 2 | 3\28 | Octokaidecal |
| 2 | 5\28 | Antikythera |
| 4 | 1\28 | |
| 4 | 2\28 | Demolished |
| 4 | 3\28 | |
| 7 | 1\28 | Whitewood |
| 14 | 1\28 |
Commas
28et tempers out the following commas. This assumes the val ⟨28 44 65 79 97 104].
| Prime limit |
Ratio[1] | Monzo | Cents | Color name | Name(s) |
|---|---|---|---|---|---|
| 3 | 2187/2048 | [-11 7⟩ | 113.69 | Lawa | Apotome |
| 5 | 648/625 | [3 4 -4⟩ | 62.57 | Quadgu | Diminished comma, major diesis |
| 5 | 16875/16384 | [-14 3 4⟩ | 51.12 | Laquadyo | Negri comma, double augmentation diesis |
| 5 | (12 digits) | [17 1 -8⟩ | 11.45 | Saquadbigu | Würschmidt comma |
| 7 | 36/35 | [2 2 -1 -1⟩ | 48.77 | Rugu | Mint comma, septimal quartertone |
| 7 | 50/49 | [1 0 2 -2⟩ | 34.98 | Biruyo | Jubilisma, tritonic diesis |
| 7 | 3125/3087 | [0 -2 5 -3⟩ | 21.18 | Triru-aquinyo | Gariboh comma |
| 7 | 126/125 | [1 2 -3 1⟩ | 13.79 | Zotrigu | Starling comma, septimal semicomma |
| 7 | 65625/65536 | [-16 1 5 1⟩ | 2.35 | Lazoquinyo | Horwell comma |
| 7 | (30 digits) | [47 -7 -7 -7⟩ | 0.34 | Trisa-seprugu | Akjaysma |
| 11 | 176/175 | [4 0 -2 -1 1⟩ | 9.86 | Lorugugu | Valinorsma |
| 11 | 441/440 | [-3 2 -1 2 -1⟩ | 3.93 | Luzozogu | Werckisma |
| 11 | 4000/3993 | [5 -1 3 0 -3⟩ | 3.03 | Triluyo | Wizardharry comma |
- ↑ Ratios longer than 10 digits are presented by placeholders with informative hints
Scales
28edo is particularly well suited to Whitewood in the same way that 15edo is for Blackwood, as it has one third that is heavily tempered, but in a familiar way shared with 12edo, while the other one is significantly closer to just. (Contrast with 20 & 21edo, where one third remains the same as in 12, and the other is pushed further away, making the overall sound considerably more xenharmonic) This makes the 5ths more out of tune, but in a useful way, as you can stack major and minor thirds indefinitely until they repeat 4 octaves and 14 notes up, producing one of the largest nonrepeating harmonious chords possible in an edo this low. This produces mode of symmetry scales with two different modes and 4 different keys, making it equally easy to establish any chord in the scale as the root and modulate between them.
- Whitewood Major [14] 13131313131313
- Whitewood Minor [14] 31313131313131
- Whitewood Major [21] 121121121121121121121
- Whitewood Minor [21] 211211211211211211211
- Whitewood Diminished [21] 112112112112112112112
- (Whitewood neutral is also theoretically possible, stacking neutral or subminor & supermajor thirds, but in practice that works out as 22222222222222, or 14edo, so it doesn't count as a 28edo scale)
If you're looking for something a little more xenharmonic sounding, but still relatively low in badness, Negri [9] & [10] function very similarly to Porcupine [7] & [8] in 15edo, further cementing the idea that 28 is to 15 what 29 is to 12 - a similar structure, but with tempering errors in the opposite direction and slightly greater complexity. Both have a decent selection of familiar major and minor chords clustered towards one end of the scale, while the way they modulate between one-another and the melodies they form is quite alien to people used to the diatonic scale. Most of the compositional notes on the Porcupine page can also be applied here.
- Negri [9] 333343333
- Negri [10] 3333333331
- Negri [19] 2121212121212121211
However, unlike 15, 28 is complex enough to do recognisable approximations of various diatonic scales and their modes, although they will sound noticeably out of tune and it's obviously not the best method of using the temperament.
- Diatonic Major [7] 5434552
- Diatonic Minor [7] 5254345
- Diatonic Naive Major [7] 4534543
- Diatonic Naive Minor [7] 4354345
- Diatonic Major [10] 3243432322
- Diatonic Minor [10] 3223243432
- Diatonic Major [12] 322232232322
- Diatonic Minor [12] 322322232232
- Diatonic Major [16] 2122221222122122
- Diatonic Minor [16] 2122212222122212
- Harmonic Minor [7] 5254372
- Harmonic Major [7] 5434372
- Harmonic Minor [8] 52543522, 52543432
- Harmonic Major [8] 54343522, 54343432
- Harmonic Minor [10] 3223243432
- Harmonic Minor [11] 32232433222
- Harmonic Major [9] 324343432
- Harmonic Major [10] 3243433222
- Harmonic Minor [12] 322322232232, 322322233222
- Harmonic Major [12] 322232232232, 322232233222
- Harmonic Minor [16] 2122212222122212, 212221222212121222
- Harmonic Major [16] 2122221222122212, 212221222212121222
- Melodic Minor [7] 5254552
- Melodic Major [7] 5434345
- Melodic Minor [11] 32232432322
- Melodic Major [9] 324343432
- Diasem (Right-handed) 414434143
- Diasem (Left-handed) 441434143
- Melodic Minor [12] 322322232322
- Melodic Major [12] 322232232232
- Melodic Minor [16] 2122212222122122
- Melodic Major [16] 2122221222122212
Interestingly, as it has a near perfect 21/16, 28edo can also generate Oneirotonic scales (see 13edo) by stacking it's 11th degree, and they actually sound better in this temperament.
- Oneirotonic [5] 65656
- Oneirotonic [8] 55155151
- Oneirotonic [13] 4141141411411
- Oneirotonic [18] 311311131131113111
- Pathological Oneirotonic [23] 21112111121112111121111
- machine5
- machine6
- machine11
- machine17
Instruments
28edo can be played on the Lumatone. See Lumatone mapping for 28edo.
28edo can also be played on a 14edo guitar with very little effort. See User:MisterShafXen/Skip fretting system 28 2 3.
Music
- minuet in 28edo (2025)
- G.27 Variations in 28edo (2023)
- Happy Birthday Canon, 5-in-1 Canon in 28edo
- Canon on Twinkle Twinkle Little Star, for Baroque Oboe & Viola (2023) – (for Organ)
- Edolian - Machinery (2020)