Gallery of omnitetrachordal scales
An (incomplete) list of omnitetrachordal (OTC) scales.
Scales with two step sizes
These are mostly tempered (irrational) scales. For a few patterns (usually where the generator is close to a perfect 4/3 or 3/2), a Pythagorean (3-limit JI) version with only two step sizes is possible.
In some cases, multiple OTC patterns with the same number of large and small steps exist.
Follow the links for more detailed info on each scale!
3 tones
2L+s (the simplest possible OTC scale; s=9/8, L=4/3)
4 tones
(no OTC scales possible)
5 tones
2L+3s - sLsLs (MOS)
3L+2s - LsLsL (MOS)
6 tones
2L+4s - LsssLs
7 tones
2L+5s - sLsssLs (MOS)
5L+2s - LsLLLsL (MOS)
8 tones
2L+6s - LssssLss
9 tones
2L+7s - LsssssLss
10 tones
2L+8s - LsssssLsss
3L+7s - LsssLsLsss
5L+5s - LsLsLsLsLs (MOS)
7L+3s - sLLLsLLLsL
8L+2s - LLsLLLsLLL
11 tones
2L+9s - LssssssLsss
12 tones
2L+10s - LsssLsssssss (4+4+4), sssLssssLsss (5+2+5)
5L+7s - ssLsLssLsLsL (MOS)
7L+5s - LLsLsLLsLsLs (MOS)
10L+2s - LLLsLLLLsLLL
13 tones
2L+11s - LsssssssLssss
7L+6s - sLLLssLssLLLs
14 tones
2L+12s - LssssssssLssss
5L+9s - sssLsLsssLsLsL
7L+7s - LsLsLsLsLsLsLs (MOS)
12L+2s - LLLLLLLsLLLLLs
15 tones
2L+13s - LssssssssLsssss
7L+8s - LsLLssLsLLssLss, LssLLsLssLLsLss
12L+3s - LsLLLLLsLLLLLsL
16 tones
2L+14s - LsssssssssLsssss
17 tones
2L+15s - LsssssssssLssssss (7+3+7), LssssssssssLsssss (6+5+6)
5L+12s - sLsssLssLssLsssLs (MOS)
7L+10s - sLsLsLssLssLsLsLs, sLssLLssLssLLssLs
10L+7s - LsLsLsLLsLLsLsLsL, LsLLssLLsLLssLLsL
12L+5s - LsLLLsLLsLLsLLLsL (MOS)
18 tones
2L+16s - LssssssssssLssssss
7L+11s - LLsssLsLLsssLsssLs, LsssLLsLsssLLsLsss
19 tones
2L+17s - LsssssssssssLssssss
5L+14s - sLssssLssLssLssssLs
7L+12s - sLssLsLssLssLsLssLs (MOS)
10L+9s - LsLLsssLLsLLsssLLsL
12L+7s - LsLLsLsLLsLLsLsLLsL (MOS)
14L+5s - LLLLsLLsLLLLsLLsLLs
17L+2s - LLLLLsLLLLLLLsLLLLL
20 tones
2L+18s - LsssssssssssLsssssss
7L+13s - sssLsssLssLLsssLssLL, sssLsssLsLsLsssLsLsL, sssLsssLLssLsssLLssL
12L+8s - sLLssLLsLLLssLLsLLLs, sLLssLLLsLLssLLLsLLs, sLsLsLLLsLsLsLLLsLsL
21 tones
2L+19s - LssssssssssssLsssssss
5L+16s - sLsssssLssLssLsssssLs
7L+14s - ssssLssssLsLLssssLsLL, ssssLssssLLsLssssLLsL
22 tones
2L+20s - LssssssssssssLssssssss (9+4+9), LsssssssssssssLsssssss (8+6+8)
5L+17s - ssssLsssLssssLsssLsssL (MOS)
7L+15s - LssLsLsssLssLsLsssLsss, LsssLsLssLsssLsLssLsss, LsssLLsssLsssLLsssLsss
10L+12s - LsLsLsLsLsLssLsLsLsLss
12L+10s - sLsLsLsLsLsLLsLsLsLsLL, sLLsLsLLssLLssLLsLsLLs, sLLssLLLssLLssLLLssLLs
15L+7s - sLLLssLLLsLLLssLLLsLLL
17L+5s - sLLLsLLLsLLLLsLLLsLLLL (MOS)
23 tones
2L+21s - LsssssssssssssLssssssss
7L+16s - ssssLssssLssLLssssLssLL, ssLsLsLssssLssssLsLsLss, ssssLssssLLssLssssLLssL
24 tones
2L+22s - LssssssssssssssLssssssss
5L+19s - sssssLsssLsssssLsssLsssL
7L+17s - sssLsssLssLssLsssLssLssL, sssLsssLsssLsLsssLsssLsL
10L+14s - LssLLssssLLssLLssssLLssL, sssLsLsLsLsssLsLsLsLsLsL
12L+12s - LsLsLsLsLsLsLsLsLsLsLsLs (MOS), ssLsLLssLLssLsLLssLLssLL, ssLLsLssLLssLLsLssLLssLL
14L+10s - LssLLLLssLLssLLssLLLLssL, LLsLsLsLsLsLsLLLsLsLsLsL
15L+9s - LLsssLLLsLLLsLLLsssLLLsL
17L+7s - LLLsLLsLLsLLLsLLsLLsLLLs, LLLsLsLLLsLLLsLLLsLsLLLs
19L+5s - LLLsLLLLLsLLLsLLLLLsLLLs
>24 tones
Many larger OTC L+s scales are believed to exist, but due to exponentially increasing computation time, these have not yet been studied in detail. Scales of the form 2L+ns are known to exist up to size 53, and assumed to exist for any larger size.
L/s matrix
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | L | |
| 1 | x | ||||||||||||||||||||||||
| 2 | x | x | x | x | x | x | x | ||||||||||||||||||
| 3 | x | x | x | ||||||||||||||||||||||
| 4 | x | ||||||||||||||||||||||||
| 5 | x | x | x | x | x | x | x | ||||||||||||||||||
| 6 | x | x | |||||||||||||||||||||||
| 7 | x | x | x | x | x | x | x | x | |||||||||||||||||
| 8 | x | x | x | ||||||||||||||||||||||
| 9 | x | x | x | x | |||||||||||||||||||||
| 10 | x | x | x | x | |||||||||||||||||||||
| 11 | x | x | |||||||||||||||||||||||
| 12 | x | x | x | x | x | ||||||||||||||||||||
| 13 | x | x | |||||||||||||||||||||||
| 14 | x | x | x | x | |||||||||||||||||||||
| 15 | x | x | |||||||||||||||||||||||
| 16 | x | x | x | ||||||||||||||||||||||
| 17 | x | x | x | ||||||||||||||||||||||
| 18 | x | ||||||||||||||||||||||||
| 19 | x | x | |||||||||||||||||||||||
| 20 | x | ||||||||||||||||||||||||
| 21 | x | ||||||||||||||||||||||||
| 22 | x | ||||||||||||||||||||||||
| 23 | x | ||||||||||||||||||||||||
| 24 | x | ||||||||||||||||||||||||
| s |
Scales with 3 step sizes
8 notes
ABCABCAB (Fokker block)
2L+3M+3s - LMsMsLMs, sMLsMsML
10 tones
ABACABACAB (3-SN)
2L+3M+5s - sMsLsMsMsL
2L+5M+3s - MsMLMsMsML
5L+2M+3s - LsLMLsLsLm
11 tones
ABACABACABA (3-SN)
2L+3M+6s - sMsLsMssMsL
2L+6M+3s - MsMLMsMMsML
ABCAABCAABA
2L+3M+6s - sMLssMssMLs, sLMssMssLMs
2L+6M+3s - MsLMMsMMsLM, MLsMMsMMLsM
12 tones
ABABCABABCAB (Fokker block)
5L+2M+5s - LsLsMLsLsLsM, MsLsLsLMsLsL
5L+5M+2s - LMLMsLMLMLMs, sMLMLMLsMLML
13 tones
ABACAABACAABA
2L+3M+8s - sMsLssMssMsLs, sLsMssMssLsMs
2L+8M+3s - MsMLMMsMMsMLM, MLMsMMsMMLMsM
14 tones
ABABACABABACAB (3-SN)
5L+2M+7s - sLsMsLsLsLsMsL
5L+7M+2s - MLMsMLMLMLMsML
15 tones
ABAACAABAACAABA (3-SN)
2L+3M+10s - sMssLssMssMssLs
2L+5M+3s - MsMMLMMsMMsMMLM
10L+2M+3s - LsLLMLLsLLsLLML
17 tones
ABAABACABAABACABA (3-SN)
2L+5M+10s - sMsLsMssMssMsLsMs
2L+10M+5s - MsMLMsMMsMMsMLMsM
5L+2M+10s - sLsMsLssLssLsMsLs
10L+2M+5s - LsLMLsLLsLLsLMLsL
ABAABCAABAABCAABA
2L+5M+10s - sMssMLssMssMLssMs
2L+10M+5s - MsMMsLMMsMMsLMMsM
5L+2M+10s - sLssLMssLssLMssLs
10L+2M+5s - LsLLsMLLsLLsMLLsL
ABACABCABACABCABC (Fokker block)
5L+7M+5s - MLMsMLsMLsMLMsMLs, sLMsMLMsLMsLMsMLM
7L+5M+5s - LMLsLMsLMsLMLsLMs, sMLsLMLsMLsMLsLML
ABCABCAABCABCAABC (Fokker block)
5L+7M+5s - MLsMLsMMLsMLsMMLs, sLMMsLMsLMMsLMsLM
7L+5M+5s - LMsLMsLLMsLMsLLMs, sMLLsMLsMLLsMLsML
19 tones
ABCBAABAABCBAABAABA (3-SN)
ABA=9/8
ABCBA=32/27, BC=256/243
10L+2M+7s: LsMsLLsLLsMsLLsLLsL
ABCABCABABCABCABCAB (Fokker block)
20 tones
ABACABABABABABACABAB
22 tones
ABABCABABABABABABCABAB
ACABBACABACABBACABACAB
AbcbAbcbAAbcbAbcbAbcbA
24 tones
ACABABACABACABABACABACAB (3-SN)
MET-24 has this structure as 5L+12M+7s: MLMsMsMLMsMLMsMsMLMsMLMs
AABAAABAACAABAAABAACAABA (3-SN)
29 tones
BABBABABBABCBABBABABBABCBABBA (3-SN)
2L+10m+17s: smsmssmsLsmssmsmssmsLsmssmsms
31 tones
BABBCBBABABBABABBCBBABABBABABBA (3-SN)
10L+19m+2s: mLmmLmsmLmmLmmLmLmmLmmLmsmLmmLm
10L+2m+19s: sLssLsmsLssLssLsLssLssLsmsLssLs
41 tones
BABCBABBABBABCBABBABCBABBABBABCBABBABCBAB (3-SN)
5L+12M+24s: sMsLsMssMssMsLsMssMsLsMssMssMsLsMssMsLsMs
BBABBBABBABBBABBCBBABBBABBABBBABBCBBABBBA (3-SN)
29L+2M+10s: LsLLLsLLMLLsLLLsLLsLLLsLLsLLLsLLMLLsLLLsL
2L+29M+10s: MsMMMsMMLMMsMMMsMMsMMMsMMsMMMsMMLMMsMMMsM
46 tones
CACACBCACACACBCACACCACACBCACACACBCACACCACACBCA (3-SN)
72 tones
ABAABABABAABABACABABAABABABAABABAABABABAABABAABABABAABABACABABAABABABAAB (3-SN)
29L+2M+41s: sLssLsLsLssLsLsMsLsLssLsLsLssLsLssLsLsLssLsLssLsLsLssLsLsMsLsLssLsLsLssL
2L+29M+41s: sMssMsMsMssMsMsLsMsMssMsMsMssMsMssMsMsMssMsMssMsMsMssMsMsLsMssMsMsMssM
Definitions and formulas
"Dual" refers to the "inverse" of a L+s scale pattern, where every L is replaced by s, and vice versa. For example, sLssL and LsLLs are duals. If a scale is OTC, its dual is often OTC as well, but not always!
"Perfect" means that values for L and s exist such that L > s and that every mode of the scale will contain a perfect (just) 3/2 or 4/3 (or both). (See also Eigenmonzo subgroup, or unchanged-interval basis.)
In this case the value P is given, where P = L/s. For a perfect scale, P > 1. Note that if a scale "a" is perfect (Pa = L/s), its dual "b" will have the value Pb = s/L = 1/Pa, and therefore must be imperfect (if Pa > 1, then Pb < 1 ).
In some cases, P may be less than zero. I'm not yet sure what this means :)
The value of P is calculated as follows:
a = the number of L steps per 2/1
b = the number of s steps per 2/1
c = the number of L steps per 4/3
d = the number of s steps per 4/3
x = log2(4/3) = ~0.41504 octaves = ~498.045 cents
aL+bs = log2(2/1) = 1
cL+ds = x
s = (c-ax)/(bc-ad)
L = (x-ds)/c
P = L/s
Note that the same procedure could be used to calculate the L/s ratio necessary to give any other just interval, such as 5/4, 11/8, etc.
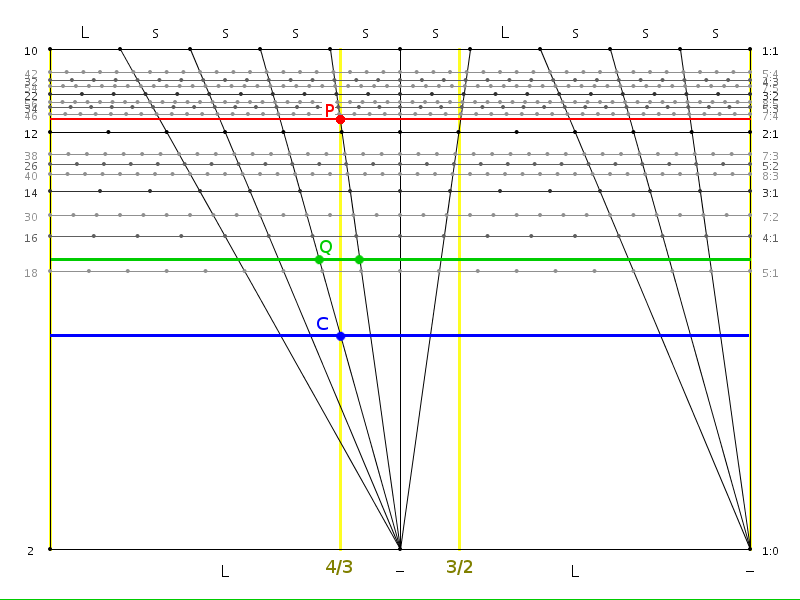
Q is calculated similarly to P, but indicates a limit of sorts -- a point on the L/s continuum beyond which the omnitetrachordality of the scale can be considered to 'break down' in some way.
Consider for example the OTC 2L+8s pajara MODMOS, LsssssLsss -- at P = L/s = 1.885, L+3s forms a just 4/3. As L/s increases, L gets larger and s smaller; at Q = L/s = 4.827, a just 4/3 is not L+3s, but L+2.5s. Past this point, L+2s will therefore be closer to a just 4/3 than L+3s:
For some scales, Q will not exist. For others, a second Q may exist that is less than P, placing a lower bound on the L/s ratio as well as an upper one.
We can also consider a point C, where the number of steps "crosses" the just 4/3 entirely - in the example above, this corresponds to 4/3 = L+2s. Q is then halfway between P and C, i.e.
C = 2Q-P
Q = (C+P)/2
P = 2Q-C
...which leads to the curious result that, although P is undefined when calculated by the normal method (due to division by zero) for a scale such as blackwood[10] (LsLsLsLsLs), C and Q can be calculated, and thus a value of P = -1 can be found anyway, even though it seems not to be of any use :)
"L/s range": For any L+s scale pattern, the ratio L/s may range from 1 (L=s, in which case the scale is (L+s)edo ) to ~infinity (s=0, in which case the scale is (L)edo ). A note such as "full L/s range is good" simply means that the approximation of 3/2 or 4/3 is reasonable across the entire range; no other assessment of the scale's "goodness" is intended.