AFDO
An AFDO (arithmetic frequency division of the octave) or ODO (otonal division of the octave) is a periodic tuning system which divides the octave according to an arithmetic progression of frequency.
For example, in 12afdo the first degree is 13/12, the second is 14/12 (7/6), and so on. For an AFDO system, the difference between interval ratios is equal (they form an arithmetic progression), rather than their ratios between interval ratios being equal as in EDO systems (a geometric progression). All AFDOs are subsets of just intonation, and up to transposition, any AFDO is a superset of a smaller AFDO and a subset of a larger AFDO (i.e. n-afdo is a superset of (n - 1)-afdo and a subset of (n + 1)-afdo for any integer n > 1).
When treated as a scale, the AFDO is equivalent to the overtone scale. However, an overtone scale often has an assumption of a tonic whereas an AFDO simply describes all the theoretically available pitch relations. Therefore, a passage built on 12::22 could be said to be in Mode 12, but is technically covered by 11afdo.
An AFDO is equivalent to an ODO (otonal division of the octave). It may also be called an EFDO (equal frequency division of the octave), however, this more general acronym is typically reserved for divisions of irrational intervals (unlike the octave) which are therefore not subsets of just intonation.
Formula
Within each period of any n-afdo system, the frequency ratio r of the m-th degree is
[math]\displaystyle{ \displaystyle r = (n + m)/n }[/math]
Alternatively, with common frequency difference d = 1/n, we have:
[math]\displaystyle{ r = 1 + md }[/math]
In particular, when m = 0, r = 1, and when m = n, r = 2.
Relation to string lengths
If the first division has ratio of r1 and length of l1 and the last, rn and ln , we have: ln = 1/rn and if rn > … > r3 > r2 > r1, then l1 > l2 > l3 > … > ln
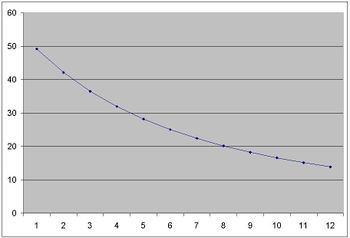
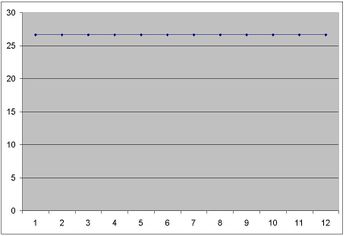
These lengths are related to the inverse of ratios in the system. The above picture shows the differences between divisions of length in 12ado system. On the contrary, we have equal divisions of length in EDL systems (→ EDL system):
Relation to superparticular ratios
An AFDO has step sizes of superparticular ratios with increasing numerators. For example, 5ado has step sizes of 6/5, 7/6, 8/7, and 9/8.
Relation to otonality & harmonic series
We can consider an AFDO system as an otonal system. Otonality is a term introduced by Harry Partch to describe chords whose notes are the overtones (multiples) of a given fixed tone. Considering AFDO, an otonality is a collection of pitches which can be expressed in ratios that have equal denominators. For example, 1/1, 5/4, and 3/2 form an otonality because they can be written as 4/4, 5/4, 6/4. Every otonality is therefore part of the harmonic series. An otonality corresponds to an arithmetic series of frequencies or a harmonic series of wavelengths or distances on a string instrument.
History
In the earliest materials, the AFDO was known as the ADO, for arithmetic division of the octave. The term was proposed by Shaahin Mohajeri in 2006, along with the term EDL (equal division of length)[1]. Previously, the set of pitch materials equivalent to n-ado's had been known as "mode n of the harmonic series", "over-n scales", and n-edl's had been known as "aliquot-n" scales, as the distinction between tunings and scales were not made. Neither of Shaahin's two new concepts were systematic extensions of the term EDO (equal division of the octave), and no one else used these two terms besides Shaahin himself.
In 2021, a team consisting of Douglas Blumeyer, Billy Stiltner, and Paul Erlich developed the first systematic extension of EDO from equal divisions of pitch to equal divisions of frequency and length, including special terms for divisions of rational intervals such as the octave; under this system, an n-ADO would be an n-ODO.
In 2023, Flora Canou revived the old term, reinterpreting the word "arithmetic" as a reference to the arithmetic mean in addition to arithmetic progressions, then extended it through the other Pythagorean means, and later through all power means. As it was shown that arithmetic alone was insufficient to define the object since frequency could not be assumed, the term was eventually changed to AFDO, showing both the type of power mean and the sampled resource.
Properties
- n-afdo has maximum variety n.
- Except for 1afdo and 2afdo, AFDOs are chiral. The inverse of n-afdo is n-ifdo.
- 1afdo is equivalent to 1ifdo and 1edo;
- 2afdo is equivalent to 2ifdo.
Individual pages for AFDOs
By size
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
| 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 |
| 20 | 21 | 22 | 23 | 24 | 25 | 26 | 27 | 28 | 29 |
| 30 | 31 | 32 | 33 | 34 | 35 | 36 | 37 | 38 | 39 |
| 40 | 41 | 42 | 43 | 44 | 45 | 46 | 47 | 48 | 49 |
| 50 | 51 | 52 | 53 | 54 | 55 | 56 | 57 | 58 | 59 |
| 60 | 61 | 62 | 63 | 64 | 65 | 66 | 67 | 68 | 69 |
| 70 | 71 | 72 | 73 | 74 | 75 | 76 | 77 | 78 | 79 |
| 80 | 81 | 82 | 83 | 84 | 85 | 86 | 87 | 88 | 89 |
| 90 | 91 | 92 | 93 | 94 | 95 | 96 | 97 | 98 | 99 |
| 100 | 101 | 102 | 103 | 104 | 105 | 106 | 107 | 108 | 109 |
| 110 | 111 | 112 | 113 | 114 | 115 | 116 | 117 | 118 | 119 |
| 120 | 121 | 122 | 123 | 124 | 125 | 126 | 127 | 128 | 129 |
| 130 | 131 | 132 | 133 | 134 | 135 | 136 | 137 | 138 | 139 |
| 140 | 141 | 142 | 143 | 144 | 145 | 146 | 147 | 148 | 149 |
| 150 | 151 | 152 | 153 | 154 | 155 | 156 | 157 | 158 | 159 |
| 160 | 161 | 162 | 163 | 164 | 165 | 166 | 167 | 168 | 169 |
| 170 | 171 | 172 | 173 | 174 | 175 | 176 | 177 | 178 | 179 |
| 180 | 181 | 182 | 183 | 184 | 185 | 186 | 187 | 188 | 189 |
| 190 | 191 | 192 | 193 | 194 | 195 | 196 | 197 | 198 | 199 |
By prime family
Over-2: 2, 4, 8, 16, 32, 64, 128, 256, 512
Over-3: 3, 6, 12, 24, 48, 96, 192, 384
Over-5: 5, 10, 20, 40, 80, 160, 320
Over-7: 7, 14, 28, 56, 112, 224, 448
Over-11: 11, 22, 44, 88, 176, 352
Over-13: 13, 26, 52, 104, 208, 416
Over-17: 17, 34, 68, 136, 272, 544
Over-29: 29, 58, 116, 232, 464
Over-31: 31, 62, 124, 248, 496
By other properties
Prime: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97, 101, 103, 107, 109, 113, 127, 131, 137, 139, 149, 151, 157, 163, 167, 173, 179, 181, 191, 193, 197, 199, 211, 223, 227, 229, 233, 239, 241, 251, 257, 263, 269, 271
Semiprime: 4, 6, 9, 10, 14, 15, 21, 22, 25, 26, 33, 34, 35, 38, 39, 46, 49, 51, 55, 57, 58, 62, 65, 69, 74, 77, 82, 85, 86, 87, 91, 93, 94, 95, 106, 111, 115, 118, 119, 121, 122, 123, 129, 133, 134, 141, 142, 143, 145, 146, 155, 158, 159, 161, 166, 169, 177, 178, 183, 185, 187
Odd squarefree semiprime: 15, 21, 33, 35, 39, 51, 55, 57, 65, 69, 77, 85, 87, 91, 93, 95, 111, 115, 119, 123, 129, 133, 141, 143, 145, 155, 159, 161, 177, 183, 185, 187, 201, 203, 205, 209, 213, 215, 217, 219, 221, 235, 237, 247, 249, 253, 259, 265, 267, 287, 291, 295, 299, 301, 303
Nonprime prime power: 4, 8, 9, 16, 25, 27, 32, 49, 64, 81, 121, 125, 128, 169, 243, 256, 289, 343, 361, 512, 529, 625, 729, 841, 961, 1024, 1331, 1369, 1681, 1849, 2048
See also
- AFS (arithmetic frequency sequence)
- IFDO (inverse-arithmetic frequency division of the octave)
- Frequency temperament
- 5- to 10-tone scales from the modes of the harmonic series
External links
- Approximate EDO and AFDO systems with each other (Excel sheet)[dead link]
- Fret position calculator (Excel sheet)
- Magic of Tone and the Art of Music by the late Dane Rhudyar