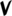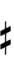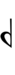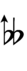62edo
| ← 61edo | 62edo | 63edo → |
62 equal divisions of the octave (abbreviated 62edo or 62ed2), also called 62-tone equal temperament (62tet) or 62 equal temperament (62et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 62 equal parts of about 19.4 ¢ each. Each step represents a frequency ratio of 21/62, or the 62nd root of 2.
Theory
62 = 2 × 31 and the patent val of 62edo is a contorted 31edo through the 11-limit, but it makes for a good tuning in the higher limits. In the 13-limit it tempers out 169/168, 1188/1183, 847/845 and 676/675; in the 17-limit 221/220, 273/272, and 289/288; in the 19-limit 153/152, 171/170, 209/208, 286/285, and 361/360. Unlike 31edo, which has a sharp profile for primes 13, 17, 19 and 23, 62edo has a flat profile for these, as it removes the distinction of otonal and utonal superparticular pairs of the primes (e.g. 13/12 vs 14/13 for prime 13) by tempering out the corresponding square-particulars. This flat tendency extends to higher primes too, as the first prime harmonic that is tuned sharper than its 5/4 is its 59/32. Interestingly, the size differences between consecutive harmonics are monotonically decreasing for all first 24 harmonics, and 62edo is one of the few meantone edos that achieve this, great for those who seek higher-limit meantone harmony.
It provides the optimal patent val for gallium, semivalentine and hemimeantone temperaments.
Using the 35\62 generator, which leads to the ⟨62 97 143 173] val, 62edo is also an excellent tuning for septimal mavila temperament; alternatively ⟨62 97 143 172] supports hornbostel.
Odd harmonics
| Harmonic | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 | 21 | 23 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -5.18 | +0.78 | -1.08 | +8.99 | -9.38 | -8.27 | -4.40 | -8.18 | -7.19 | -6.26 | -8.92 |
| Relative (%) | -26.8 | +4.0 | -5.6 | +46.5 | -48.5 | -42.7 | -22.7 | -42.3 | -37.2 | -32.4 | -46.1 | |
| Steps (reduced) |
98 (36) |
144 (20) |
174 (50) |
197 (11) |
214 (28) |
229 (43) |
242 (56) |
253 (5) |
263 (15) |
272 (24) |
280 (32) | |
Subsets and supersets
Since 62 factors into 2 × 31, 62edo does not contain nontrivial subset edos other than 2edo and 31edo. 186edo and 248edo are notable supersets.
Miscellany
62 years is the amount of years in a leap week calendar cycle which corresponds to a year of 365 days 5 hours 48 minutes 23 seconds, meaning it is both a simple cycle for a calendar, and 62 being a multiple of 31 makes it a harmonically useful and playable cycle. The corresponding maximal evenness scales are 15 & 62 and 11 & 62.
The 11 & 62 temperament is called mabon, named so because its associated year length corresponds to an autumnal equinoctial year. In the 2.9.7 subgroup tempers out 44957696/43046721, and the three generators of 17\62 correspond to 16/9. It is possible to extend this to the 11-limit with comma basis {896/891, 1331/1296}, where 17\62 is mapped to 11/9 and two of them make 16/11. In addition, three generators make the patent val 9/8, which is also created by combining the flat patent val fifth from 31edo with the sharp 37\62 fifth.
The 15 & 62 temperament, corresponding to the leap day cycle, is demivalentine in the 13-limit.
Intervals
| Steps | Cents | Approximate ratios* | Ups and downs notation |
|---|---|---|---|
| 0 | 0.00 | 1/1 | D |
| 1 | 19.35 | 65/64, 66/65, 78/77, 91/90, 105/104 | ^D, vE♭♭ |
| 2 | 38.71 | 33/32, 36/35, 45/44, 49/48, 50/49, 55/54, 56/55, 64/63 | ^^D, E♭♭ |
| 3 | 58.06 | 26/25, 27/26 | vD♯, ^E♭♭ |
| 4 | 77.42 | 21/20, 22/21, 23/22, 24/23, 25/24, 28/27 | D♯, vvE♭ |
| 5 | 96.77 | 17/16, 18/17, 19/18, 20/19 | ^D♯, vE♭ |
| 6 | 116.13 | 15/14, 16/15 | ^^D♯, E♭ |
| 7 | 135.48 | 13/12, 14/13 | vD𝄪, ^E♭ |
| 8 | 154.84 | 11/10, 12/11, 23/21 | D𝄪, vvE |
| 9 | 174.19 | 21/19 | ^D𝄪, vE |
| 10 | 193.55 | 9/8, 10/9, 19/17, 28/25 | E |
| 11 | 212.90 | 17/15 | ^E, vF♭ |
| 12 | 232.26 | 8/7 | ^^E, F♭ |
| 13 | 251.61 | 15/13, 22/19 | vE♯, ^F♭ |
| 14 | 270.97 | 7/6 | E♯, vvF |
| 15 | 290.32 | 13/11, 19/16, 20/17 | ^E♯, vF |
| 16 | 309.68 | 6/5 | F |
| 17 | 329.03 | 17/14, 23/19 | ^^F, G♭♭ |
| 18 | 348.39 | 11/9, 27/22, 28/23 | ^^F, G♭♭ |
| 19 | 367.74 | 16/13, 21/17, 26/21 | vF♯, ^G♭♭ |
| 20 | 387.10 | 5/4 | F♯, vvG♭ |
| 21 | 406.45 | 19/15, 24/19 | ^F♯, vG♭ |
| 22 | 425.81 | 9/7, 14/11, 23/18, 32/25 | ^^F♯, G♭ |
| 23 | 445.16 | 13/10, 22/17 | vF𝄪, ^G♭ |
| 24 | 464.52 | 17/13, 21/16, 30/23 | F𝄪, vvG |
| 25 | 483.87 | 25/19 | ^F𝄪, vG |
| 26 | 503.23 | 4/3 | G |
| 27 | 522.58 | 19/14, 23/17 | ^G, vA♭♭ |
| 28 | 541.94 | 11/8, 15/11, 26/19 | ^^G, A♭♭ |
| 29 | 561.29 | 18/13 | vG♯, ^A♭♭ |
| 30 | 580.65 | 7/5, 25/18, 32/23 | G♯, vvA♭ |
| 31 | 600.00 | 17/12, 24/17 | E |
| 32 | 619.35 | 10/7, 23/16, 36/25 | ^^G♯, A♭ |
| 33 | 638.71 | 13/9 | vG𝄪, ^A♭ |
| 34 | 658.06 | 16/11, 19/13, 22/15 | G𝄪, vvA |
| 35 | 677.42 | 28/19, 34/23 | ^G𝄪, vA |
| 36 | 696.77 | 3/2 | A |
| 37 | 716.13 | 38/25 | ^A, vB♭♭ |
| 38 | 735.48 | 23/15, 26/17, 32/21 | ^^A, B♭♭ |
| 39 | 754.84 | 17/11, 20/13 | vA♯, ^B♭♭ |
| 40 | 774.19 | 11/7, 14/9, 25/16, 36/23 | A♯, vvB♭ |
| 41 | 793.55 | 19/12, 30/19 | ^A♯, vB♭ |
| 42 | 812.90 | 8/5 | ^^A♯, B♭ |
| 43 | 832.26 | 13/8, 21/13, 34/21 | vA𝄪, ^B♭ |
| 44 | 851.61 | 18/11, 23/14, 44/27 | A𝄪, vvB |
| 45 | 870.97 | 28/17, 38/23 | ^A𝄪, vB |
| 46 | 890.32 | 5/3 | B |
| 47 | 909.68 | 17/10, 22/13, 32/19 | ^B, vC♭ |
| 48 | 929.03 | 12/7 | ^^B, C♭ |
| 49 | 948.39 | 19/11, 26/15 | vB♯, ^C♭ |
| 50 | 967.74 | 7/4 | B♯, vvC |
| 51 | 987.10 | 30/17 | ^B♯, vC |
| 52 | 1006.45 | 9/5, 16/9, 25/14, 34/19 | C |
| 53 | 1025.81 | 38/21 | ^C, vD♭♭ |
| 54 | 1045.16 | 11/6, 20/11, 42/23 | ^^C, D♭♭ |
| 55 | 1064.52 | 13/7, 24/13 | vC♯, ^D♭♭ |
| 56 | 1083.87 | 15/8, 28/15 | C♯, vvD♭ |
| 57 | 1103.23 | 17/9, 19/10, 32/17, 36/19 | ^C♯, vD♭ |
| 58 | 1122.58 | 21/11, 23/12, 27/14, 40/21, 44/23, 48/25 | ^^C♯, D♭ |
| 59 | 1141.94 | 25/13, 52/27 | vC𝄪, ^D♭ |
| 60 | 1161.29 | 35/18, 49/25, 55/28, 63/32, 64/33, 88/45, 96/49, 108/55 | C𝄪, vvD |
| 61 | 1180.65 | 65/33, 77/39, 128/65, 180/91, 208/105 | ^C𝄪, vD |
| 62 | 1200.00 | 2/1 | D |
* 23-limit patent val, inconsistent intervals in italic
Notation
Ups and downs notation
62edo can be notated with ups and downs, spoken as up, dup, downsharp, sharp, upsharp etc. and down, dud, upflat etc. Note that dup is equivalent to dudsharp and dud is equivalent to dupflat.
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |
|||||||||
| Flat symbol | |
|
Alternative symbols for ups and downs notation uses sharps and flats and quarter-tone accidentals combined with arrows, borrowed from extended Helmholtz–Ellis notation:
| Step offset | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|---|---|
| Sharp symbol | |
|
|
|
|
|
|
| ||
| Flat symbol | |
|
|
|
|
|
Sagittal notation
This notation uses the same sagittal sequence as EDOs 69 and 76, and is a superset of the notation for 31-EDO.
Evo flavor

Revo flavor

Evo-SZ flavor

In the diagrams above, a sagittal symbol followed by an equals sign (=) means that the following comma is the symbol's primary comma (the comma it exactly represents in JI), while an approximately equals sign (≈) means it is a secondary comma (a comma it approximately represents in JI). In both cases the symbol exactly represents the tempered version of the comma in this EDO.
Armodue notation
- Armodue nomenclature 8;3 relation
- Ɨ = Thick (1/8-tone up)
- ‡ = Semisharp (1/4-tone up)
- b = Flat (5/8-tone down)
- ◊ = Node (sharp/flat blindspot 1/2-tone)
- # = Sharp (5/8-tone up)
- v = Semiflat (1/4-tone down)
- ⌐ = Thin (1/8-tone down)
| # | Cents | Armodue notation | Associated ratio | |
|---|---|---|---|---|
| 0 | 0.0 | 1 | ||
| 1 | 19.4 | 1Ɨ | ||
| 2 | 38.7 | 1‡ (9#) | ||
| 3 | 58.1 | 2b | ||
| 4 | 77.4 | 1◊2 | ||
| 5 | 96.8 | 1# | ||
| 6 | 116.1 | 2v | ||
| 7 | 135.5 | 2⌐ | ||
| 8 | 154.8 | 2 | 11/10~12/11 | |
| 9 | 174.2 | 2Ɨ | ||
| 10 | 193.5 | 2‡ | ||
| 11 | 212.9 | 3b | 8/7 | |
| 12 | 232.3 | 2◊3 | ||
| 13 | 251.6 | 2# | ||
| 14 | 271.0 | 3v | ||
| 15 | 290.3 | 3⌐ | ||
| 16 | 309.7 | 3 | 6/5~7/6 | |
| 17 | 329.0 | 3Ɨ | ||
| 18 | 348.4 | 3‡ | ||
| 19 | · | 367.7 | 4b | 5/4 |
| 20 | 387.1 | 3◊4 | ||
| 21 | 406.5 | 3# | ||
| 22 | 425.8 | 4v (5b) | ||
| 23 | 445.2 | 4⌐ | ||
| 24 | 464.5 | 4 | ||
| 25 | 483.9 | 4Ɨ (5v) | ||
| 26 | 503.2 | 5⌐ (4‡) | ||
| 27 | · | 522.6 | 5 | 4/3~11/8 |
| 28 | 541.9 | 5Ɨ | ||
| 29 | 561.3 | 5‡ (4#) | ||
| 30 | 580.6 | 6b | 10/7 | |
| 31 | 600.0 | 5◊6 | ||
| 32 | 619.4 | 5# | 7/5 | |
| 33 | 638.7 | 6v | ||
| 34 | 658.1 | 6⌐ | ||
| 35 | · | 677.4 | 6 | 3/2~16/11 |
| 36 | 696.8 | 6Ɨ | ||
| 37 | 716.1 | 6‡ | ||
| 38 | 735.5 | 7b | ||
| 39 | 754.8 | 6◊7 | ||
| 40 | 774.2 | 6# | ||
| 41 | 793.5 | 7v | ||
| 42 | 812.9 | 7⌐ | ||
| 43 | · | 832.3 | 7 | 8/5 |
| 44 | 851.6 | 7Ɨ | ||
| 45 | 871.0 | 7‡ | ||
| 46 | 890.3 | 8b | 5/3~12/7 | |
| 47 | 909.7 | 7◊8 | ||
| 48 | 929.0 | 7# | ||
| 49 | 948.4 | 8v | ||
| 50 | 967.7 | 8⌐ | ||
| 51 | 987.1 | 8 | 7/4 | |
| 52 | 1006.5 | 8Ɨ | ||
| 53 | 1025.8 | 8‡ | ||
| 54 | 1045.2 | 9b | 11/6~20/11 | |
| 55 | 1064.5 | 8◊9 | ||
| 56 | 1083.9 | 8# | ||
| 57 | 1103.2 | 9v (1b) | ||
| 58 | 1122.6 | 9⌐ | ||
| 59 | 1141.9 | 9 | ||
| 60 | 1161.3 | 9Ɨ (1v) | ||
| 61 | 1180.6 | 1⌐ (9‡) | ||
| 62 | 1200.0 | 1 | ||
Approximation to JI
Zeta peak index
| Tuning | Strength | Octave (cents) | Integer limit | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| ZPI | Steps per 8ve |
Step size (cents) |
Height | Integral | Gap | Size | Stretch | Consistent | Distinct | |
| Tempered | Pure | |||||||||
| 314zpi | 61.938047 | 19.374198 | 6.262952 | 4.11259 | 0.952068 | 15.026453 | 1201.200285 | 1.200285 | 8 | 8 |
Regular temperament properties
62edo is contorted 31edo through the 11-limit.
| Subgroup | Comma list | Mapping | Optimal 8ve stretch (¢) |
Tuning error | |
|---|---|---|---|---|---|
| Absolute (¢) | Relative (%) | ||||
| 2.3.5.7.11.13 | 81/80, 99/98, 121/120, 126/125, 169/168 | [⟨62 98 144 174 214 229]] | +1.38 | 1.41 | 7.28 |
| 2.3.5.7.11.13.17 | 81/80, 99/98, 121/120, 126/125, 169/168, 221/220 | [⟨62 98 144 174 214 229 253]] | +1.47 | 1.32 | 6.83 |
| 2.3.5.7.11.13.17.19 | 81/80, 99/98, 121/120, 126/125, 153/152, 169/168, 209/208 | [⟨62 98 144 174 214 229 253 263]] | +1.50 | 1.24 | 6.40 |
| 2.3.5.7.11.13.17.19.23 | 81/80, 99/98, 121/120, 126/125, 153/152, 161/160, 169/168, 209/208 | [⟨62 98 144 174 214 229 253 263 280]] | +1.55 | 1.18 | 6.09 |
Rank-2 temperaments
| Periods per 8ve |
Generator* | Cents* | Associated ratio* |
Temperament |
|---|---|---|---|---|
| 1 | 3\62 | 58.1 | 27/26 | Hemisecordite |
| 1 | 7\62 | 135.5 | 13/12 | Doublethink |
| 1 | 13\62 | 251.6 | 15/13 | Hemimeantone |
| 1 | 17\62 | 329.0 | 16/11 | Mabon |
| 1 | 29\62 | 561.3 | 18/13 | Demivalentine |
| 2 | 3\62 | 58.1 | 27/26 | Semihemisecordite |
| 2 | 4\62 | 77.4 | 21/20 | Semivalentine |
| 2 | 6\62 | 116.1 | 15/14 | Semimiracle |
| 2 | 26\62 | 503.2 | 4/3 | Semimeantone |
| 31 | 29\62 (1\62) |
561.3 (19.4) |
11/8 (196/195) |
Kumhar (62e) |
| 31 | 19\62 (1\62) |
367.7 (19.4) |
16/13 (77/76) |
Gallium |
* Octave-reduced form, reduced to the first half-octave, and minimal form in parentheses if distinct
Instruments
Lumatone
Skip fretting
Skip fretting system 62 6 11 has strings tuned 11\62 apart, while frets are 6\62.
On a 4-string bass, here are your open strings:
0 11 22 33
A good supraminor 3rd is found on the 2nd string, 1st fret. A supermajor third is found on the open 3rd string. The major 6th can be found on the 4th string, 2nd fret.
5-string bass
51 0 11 22 33
This adds an interval of a major 7th (minus an 8ve) at the first string, 1st fret.
6-string guitar
0 11 22 33 44 55
”Major” 020131
7-string guitar
0 11 22 33 44 55 4
Skip fretting system 62 9 11 is another 62edo skip fretting system. The 5th is on the 5th string. The major 3rd is on the 2nd string, 1st fret.