Harmonic series
The harmonic series is a sequence of tones generated by whole-number frequency ratios over a fundamental: 1/1, 2/1, 3/1, 4/1, 5/1, 6/1, 7/1… ad infinitum. Each member of this series is a harmonic (which is short for "harmonic partial").
Note that the terms overtone and overtone series are not quite synonymous with harmonic and harmonic series, respectively, although interchangeable usage is also attested. Technically speaking, overtone series excludes the starting fundamental, so the 2nd harmonic is the 1st overtone. Because of that distinction, the math of the "overtone series" is off by one. So, "harmonic series" is arguably the preferred standard.
In just intonation theory, the harmonic series is often treated as the foundation of consonance.
The subharmonic series (or undertone series) is the inversion of the harmonic series: 1/1, 1/2, 1/3, 1/4, 1/5, 1/6, 1/7... ad infinitum.
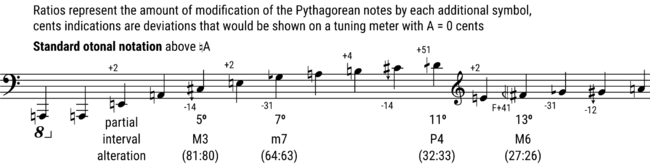
Chord of nature
Treated as a chord, the harmonic series is sometimes called the chord of nature; in German this has been called the Klang.
The q-limit chord of nature is 1:2:3:4:...:q up to some odd number q, and is the basic q-limit otonality which can be equated via octave equivalence to other versions of the complete q-limit otonal chord.
Music based on the harmonic series
The chord of nature is the name sometimes given to the harmonic series, or the series up to a certain stopping point, regarded as a chord.
Steps between adjacent members of the harmonic series are called "superparticular," and they appear in the form (n+1)/n (e.g. 4/3, 28/27, 33/32).
One might compose with the harmonic series by, for instance:
- Tuning to the first several harmonics over one fundamental;
- Tuning to an octave-repeating slice of the harmonic series for use as a scale (for instance harmonics 8 though 16, 12 through 24, 20 through 40... see overtone scales);
- Tuning to the overtones of the overtones & the undertones of the undertones. (This can produce complex scales such as Harry Partch's 43-tone Monophonic; this kind of thing is more often called "just intonation" than "overtone music".)
Music
- Various played with Fujara (slovak overtone flute)
- Various[which?]
- Various ("Snake" overtone flute)
- Various (meditative new age with overtone singing)
- Various (experimental alphorn and yodeling combined with overtone singing)
- Stimmung (1968)
- Sternklang (1971)
- Symphony No. 3 "Gloria" (1983)
See also
- Subharmonic series
- Gallery of just intervals
- Isoharmonic chords
- First Five Octaves of the Harmonic Series
- Overtone scales
- List of octave-reduced harmonics
- Prime harmonic series
- Mike Sheiman's Very Easy Scale Building From The Harmonic Series Page
- 8th Octave Overtone Tuning
- Johannes Kotschy
External links
- Spectral music article on Wikipedia
- www.naturton-musik.de[dead link] - web site dedicated to overtone music (by Austrian composer Johannes Kotschy) - a lot of theory material and practical guides to write music based on the overtone series
- Overtone music network - a portal for overtone music.
- Oberton-Netzwerk (Xing)[dead link] - German-speaking group dedicated to overtone music on the social network platform Xing. Microtonal music in general is welcome, too.
