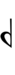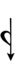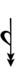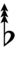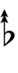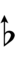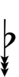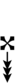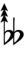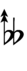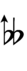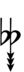168edo
| ← 167edo | 168edo | 169edo → |
168 equal divisions of the octave (abbreviated 168edo or 168ed2), also called 168-tone equal temperament (168tet) or 168 equal temperament (168et) when viewed under a regular temperament perspective, is the tuning system that divides the octave into 168 equal parts of about 7.14 ¢ each. Each step represents a frequency ratio of 21/168, or the 168th root of 2.
168edo is closely related to 84edo, but the patent vals differ on the mapping for 11 and 17. It is contorted in the 7-limit, tempering out 225/224, 1728/1715, and 78732/78125. Using the patent val, it tempers out 243/242, 2420/2401, 3025/3024, and 43923/43750 in the 11-limit; 351/350, 625/624, 640/637, 847/845, and 1573/1568 in the 13-limit; 375/374, 561/560, 715/714, 891/884, 936/935, and 1331/1326 in the 17-limit. Using the 168d val, it tempers out 3136/3125, 19683/19600, and 33075/32768 in the 7-limit; 243/242, 385/384, 3773/3750, and 9801/9800 in the 11-limit.
Stacking alternating steps of 43 and 53 produces an optimal whitewood[14] scale of 19 5 19 5 19 5 19 5 19 5 19 5 19 5 that spreads the overall flatness evenly between the major and minor thirds. Dotcom is also supported.
Odd harmonics
| Harmonic | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 | 21 | 23 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Error | Absolute (¢) | -1.96 | -0.60 | +2.60 | +3.23 | -1.32 | +2.33 | -2.55 | +2.19 | +2.49 | +0.65 | +0.30 |
| Relative (%) | -27.4 | -8.4 | +36.4 | +45.3 | -18.5 | +32.6 | -35.8 | +30.6 | +34.8 | +9.1 | +4.2 | |
| Steps (reduced) |
266 (98) |
390 (54) |
472 (136) |
533 (29) |
581 (77) |
622 (118) |
656 (152) |
687 (15) |
714 (42) |
738 (66) |
760 (88) | |
Notation
168edo can be notated using ups and downs notation, in which Helmholtz–Ellis arrow accidentals can be used.
| Semitones | 0 | 1⁄14 | 2⁄14 | 3⁄14 | 4⁄14 | 5⁄14 | 6⁄14 | 7⁄14 | 8⁄14 | 9⁄14 | 10⁄14 | 11⁄14 | 12⁄14 | 13⁄14 | 1 | 11⁄14 | 12⁄14 | 13⁄14 | 14⁄14 | 15⁄14 | 16⁄14 | 17⁄14 | 18⁄14 | 19⁄14 | 110⁄14 | 111⁄14 | 112⁄14 | 113⁄14 | 2 | 21⁄14 | 22⁄14 | 23⁄14 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sharp Symbol |  |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
| Flat Symbol |  |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
Subsets and supersets
Since 168 factors into 23 × 3 × 7, 168edo has subset edos 2, 3, 4, 6, 7, 8, 12, 14, 21, 24, 28, 42, 56, and 84.