User:VectorGraphics/Delkian scale

This is about the 34edo scale called "diatonic" in the fictional universe it's used in. For the MOS equivalent, see 10L 2s. For normal diatonic tuning in 34edo, see 34edo (internal link, I just don't know how to link to a specific section).

The Delkian scale is a scale used in Majestaz's Famana, with 12 notes: it is an octave-equivalent scale containing 10 large steps and 2 small steps. The small steps are unevenly spaced, with a pattern of LLLLsLLLLLLs, as such it is not formed by a generator, though 10 of its 12 degrees have a perfect fifth above them.

Name

The Delkian scale is referred to as "diatonic" in the fictional universe of Famana, as the 5L 2S diatonic of Western music theory is not used. It is called the "Delkian diatonic" due to an alternative popular tuning system called the Roklotian diatonic.

Notation

Intervals

Each interval class of the Delkian scale corresponds to an edostep of 12edo, just as each 12edo interval class corresponds to an edostep of 7edo. Each interval has 2 sizes, except for the tritone, which has 3.


Generic name (Western)	Specific name (Western)	Generic name (translated; in-universe)	Specific name (translated; in-universe)	Special name (in-universe)	Steps subtended	Size in 34-edosteps (34 edo)	Note name (starting on N)
unison	perfect unison	unison	perfect unison		0	0	N
minor 2nd	minor minor 2nd	1st	minor 1st	tertigrade	s	2	F-
minor 2nd	major minor 2nd	1st	major 1st	semigrade	L	3	F
major 2nd	minor major 2nd	2nd	minor 2nd	greater digrade	L+s	5	S-
major 2nd	major major 2nd	2nd	major 2nd	lacuna	2L	6	S
minor 3rd	minor minor 3rd	3rd	minor 3rd	prequadrant	2L+s	8	Z-
minor 3rd	major minor 3rd	3rd	major 3rd	quadrant	3L	9	Z
major 3rd	minor major 3rd	4th	minor 4th	terce	3L+s	11	G
major 3rd	major major 3rd	4th	major 4th	bilacuna	4L	12	G+
4th	perfect 4th	5th	perfect 5th	tersquance	4L+s	14	X
4th	augmented 4th	5th	augmented 5th		5L	15	X+
tritone	diminished tritone	6th	diminished 6th		4L+2s	16	V-
	perfect tritone		perfect 6th	median	5L+s	17	V
	augmented tritone		augmented 6th		6L	18	V+
5th	diminished 5th	7th	diminished 7th		5L+2s	19	M-
5th	perfect 5th	7th	perfect 7th	sesquance	6L+s	20	M
minor 6th	minor minor 6th	8th	minor 8th	antibilacuna	6L+2s	22	P-
minor 6th	major minor 6th	8th	major 8th	bess	7L+s	23	P
major 6th	minor major 6th	9th	minor 9th	dodrant	7L+2s	25	T
major 6th	major major 6th	9th	major 9th	postdodrant	8L+s	26	T+
minor 7th	minor minor 7th	10th	minor 10th	antilacuna	8L+2s	28	C
minor 7th	major minor 7th	10th	major 10th	greater antidigrade	9L+s	29	C+
major 7th	minor major 7th	11th	minor 11th	antisemigrade	9L+2s	31	W
major 7th	major major 7th	11th	major 11th	antitertigrade	10L+s	32	W+
octave	perfect octave	12th	perfect 12th	duplance	10L+2s	34	N

Note names

There are 12 base notes: N, F, S, Z, G, X, V/L (in equal-tempered tuning, these are the same note), M, P, T, C, and W, and then back to N. These are consonants found in a language spoken in the fictional setting. There are two accidentals: - and +. + raises the pitch, and - lowers it, by one chroma. In-universe, this is analyzed as simply a small change in pitch, unlike our # and b, which are generalized based on fifth stacking. (Though this is due to the accidentals being a simplified version of a more complex accidental system used for notating JI)

Theory

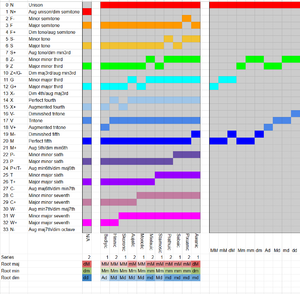
A chart of all 12 modes of the Delkian scale, including the 11 traditional modes, and what intervals and chords they contain. Each mode has 3 main triads on its root out of 10, one major, one minor, and one diminished.

The key features of the scale are that you now get both major and minor thirds in any given key, which can be either 17edo's more extreme thirds, or the more just thirds 34edo provides, or some mix of the options. These comma-sized variations in the intervals define the mode's distinct feel.

The scale was created taking both 3 and 5 into account. Naturally, the main harmonic tool is not a chain of fifths but rather a harmonic table of fifths and classical major thirds, as follows (described in terms of 34-edosteps here, with the Staimosic mode highlighted):


22	8	28	14	0	20	6	26	12	32	18	4	24	10	30	16	2
33	19	5	25	11	31	17	3	23	9	29	15	1	21	7	27	13
10	30	16	2	22	8	28	14	0	20	6	26	12	32	18	4	24
21	7	27	13	33	19	5	25	11	31	17	3	23	9	29	15	1
32	18	4	24	10	30	16	2	22	8	28	14	0	20	6	26	12
9	29	15	1	21	7	27	13	33	19	5	25	11	31	17	3	23
20	6	26	12	32	18	4	24	10	30	16	2	22	8	28	14	0
31	17	3	23	9	29	15	1	21	7	27	13	33	19	5	25	11

Note that a fifth can be built from 8 stacked 11-step thirds, so this table can be read column-by-column to count up by thirds. Note that fifths never cycle around to create a classical third ("minor major third"), so they just loop around the sides normally.

Additionally, note that multiple wolf fifths are necessary to cycle around the scale, these are just considered a variant of fifth (the "diminished fifth/seventh" mentioned) in-universe.

Modes

11 of the modes of the scale are used in traditional music composition and thus have names. These names are based on ancient tribes from the Famana setting:



Awanic	LsLLLLsLLLLL
Pixuanic	sLLLLLLsLLLL
Sebaic	LLsLLLLsLLLL
Prathuic	LsLLLLLLsLLL
Staimosic	LLLsLLLLsLLL
Mielauic	LLsLLLLLLsLL
Moriolic	LLLLsLLLLsLL
Aujalic	LLLsLLLLLLsL
Skoronic	LLLLLsLLLLsL
Hininic	LLLLsLLLLLLs
Bediyic	LLLLLLsLLLLs
N/A	sLLLLsLLLLLL