Kite Guitar Scales
Printable charts for the downmajor tuning of the Kite Guitar. One is of scale degrees, the other is of the three main heptatonic scales. In the latter, some scale degrees appear more than once. In general, use the one that agrees with the current chord.
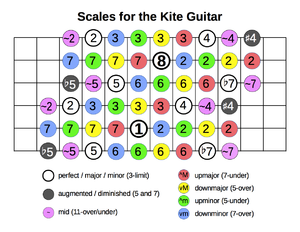
Overview
This is the practical guide to Kite Guitar scales. See also Kite Giedraitis's Categorizations of 41edo Scales, which is more theoretical.
There are many possible 41edo scales. Those discussed here are those with at least 5 notes, and which have a plain perfect 5th from the tonic. Scales that are awkward to play on the Kite guitar are avoided. An awkward scale has a step which requires a jump of more than four frets. Thus plain minor 2nds and plain/mid 3rds are avoided. A scale naturally hops from one string to the next as it goes up or down. Unlike other guitars, the Kite guitar doesn't let one hop freely. For example, the 3-limit scale fragment P1 M2 M3 P4 requires 3 hops, 2 upward and 1 downward. Any scale which doesn't have exactly three upward hops per octave will be awkward, because the downward hop will always be at least 6 frets, and usually 7 or more. Almost every scale with a low prime limit and/or a low odd limit is not awkward.
MOS (moment of symmetry) scales have only two step sizes, with the less frequent steps evenly distributed throughout the scale. MOS scales are an important part of microtonal scale theory. But almost every 41-edo MOS scale with a perfect 5th is awkward. The only exception is scales from the Laquinyo temperament, which have a small step of only one fret. They have either a very lopsided L/s ratio or more than 12 notes. They are discussed further in the Nineteen-tone section. See also the Checkerboard scale in the Eleven-tone section.
Every scale can be thought of as a chord, e.g. the 12edo major pentatonic scale is a 6add9 pentad. Many pentads and heptads have an innate comma which 41edo does not temper out. Thus many Kite Guitar scales have dual notes, meaning a note may vary by 1 arrow or edostep, in order to avoid an offperfect 5th. A scale with a dual note or two is called a fluid scale. In the tables below, a note that may be either a M2 or a vM2 is indicated by (v)M2.
In general, majorish scales have a dual 2nd and minorish scales have a dual 4th. But other notes can be dual as well. For example, Iv - IVv - Vv7 - Iv requires a downmajor scale with a dual 4th. And if the V chord is Vv9, the 6th must also be dual.
What is the default form of a fluid scale? In other words, if asked to play a downmajor scale, what notes would you play? Dual notes written as (v)M2 or (v)4 default to plain. But (◇)vM6 written with a plain sign defaults to downed.
Step sizes can also be dual, leading to near-MOS scales (see below).
The dualness of a specific note can often be understood by viewing the scale as arising from alternating generators. For example, the downmajor scale with a dual 2nd can be seen as a chain of alternating upminor and downmajor 3rds: vM2 - P4 - vM6 - P1 - vM3 - P5 - vM7 - M2. The chain comes very close to closing after 7 steps, making a 7-note scale with one dual note, the major 2nd.
- upminor/downmajor generators: ^m3 and vM3
- upmajor/downminor generators: vm3 and ^M3
- upminor/downmajor pentatonic generators: ^m3 and vM2
- upmajor/downminor pentatonic generators: vm3 and ^M2
The Format
The modes of a scale are grouped together. Not every mode is shown. Often two scales are modes only because of the dual notes, e.g. downmajor and upminor. Two modes of a scale will use the same prime subgroup, so modes are grouped by subgroup. Subgroups are explained on the theoretical scales page: Kite Giedraitis's Categorizations of 41edo Scales.
Each scale has steps of various sizes, shown as a list of step sizes in arrows. A dash separates the P1-P5 section of the scale from the P5-P8 section. An odd number always hops a string, an even number never does. Thus every non-awkward scale has exactly three odd-numbered steps. This chart translates the step sizes into 41-edo notation:
| Arrows | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Name | ^1 | vm2 | m2 | ^m2 | ~2 | vM2 | M2 | ^M2 | vm3 | m3 | ^m3 |
The steps that are affected by dual-ness are underlined. For example, the downmajor scale is P1 (v)M2 vM3 P4 P5 vM6 vM7 P8. The steps are listed as if the 2nd were plain: 7647-674. Downing the 2nd makes 6747-674. Almost always, the two underlined step sizes differ by only one, and upping or downing merely swaps the two numbers. Therefore the dual-ness rarely affects the moves (see below). The only exceptions are the dorian, locrian and dodecatonic scales, all of which are problematic anyway.
The step sizes column shows the sizes used. Two modes of a scale will have the same step sizes, so modes are also grouped by step sizes. The largest-to-smallest ratio L/s indicates how even the scale is. For example, the downminor heptatonic scale has a very large L/s ratio of 8/2 = 4, giving it a lopsided feel. But the downminor pentatonic scale has a very small L/s ratio of only 9/7 = 1.29, giving it an even equipentatonic feel.
The step count column analyzes the scale by the usual MOS notation of how many large and small steps there are. Some scales also have m for medium, and even XL for extra large and xs for extra small. Most scales are not actually MOS, but a near-MOS. For example, the first two pentatonic scales are 2L 1m 2s, where L=11, m=7 and s=6. The single m step can be thought of as a fluid version of the s step, making a 2L 3s near-MOS scale. Near-MOS scales are listed as alternates, e.g. "2L 1m 2s or 2L 3s".
Harmonic and subharmonic scales are contiguous segments of the harmonic and subharmonic series respectively. They are never fluid. Harmonic and subharmonic may be abbreviated as har- and subhar-, e.g. harmajor. Pentatonic scales use (sub)harmonics 5-10, and heptatonic scales use (sub)harmonics 7-14. In harmonic scales, the step sizes get smaller as you ascend. In subharmonic scales, they get larger. In general, given a choice between an Ls sequence and an sL sequence, the first is often more otonal, and more consonant. For example, P1-M2-vM3 vs. P1-vM2-vM3, or P1-vm3-P4 vs. P1-^M2-P4, or even P1-vM3-P5 vs. P1-^m3-P5. (One exception: P4-d5-P5 is more otonal that P4-A4-P5. But P1-^m2-M2 is better than P1-m2-M2.) Likewise for the choice between LLs and LsL and sLL, or between Lss and sLs and ssL, the first is generally more consonant.
Scales are loosely named similarly to how chords are named. Adding up or down to a scale name affects the 3rd, 6th and 7th. However, there are usually dual notes not implied by the name. Harmonic and subharmonic scales are named after the tonic triad, minus the up or down.
Some scales are listed as chains of 5ths. For example, the downmajor scale is P1 (v)M2 vM3 P4 P5 vM6 vM7 P8. There are two chains: P4-P1-P5-M2 and vM2-vM6-vM3-vM7. This is condensed to P415M2 vM2637. Here the two chains overlap on a dual note. However, the near-equidistant heptatonic scales do not, and have an offperfect 5th.
The moves column is perhaps the most practical information in the table. It says how many frets to move up or down as you ascend the scale. Positive numbers refer to forward moves that move up the fretboard on a single string. Negative numbers refer to backwards moves that move up a string, then down the fretboard. The moves are not listed in order of size. Rather, forward moves are listed, then backward moves. In each category, they are listed by how often they occur in the scale. In case of a tie, the largest step size is listed first. The first move in the list is the primary forward move, and the first negative number is the primary backward move. All other moves are secondary. Because there are only 3 string-hops in an octave, there are at most 3 backwards moves. There are at most 2 secondary backwards moves, and usually only 1. A scale that doesn't have any secondary moves (i.e. has only two step sizes) is usually one of the rare MOS scales.
To see how this works, consider the two ya pentatonic scales. Their two primary moves are +3 and -1. Any short sequence of moves that uses both +3 and -1 (or -3 and +1 if descending) will be some fragment of these scales. Likewise +4 and -2 moves evoke a za pentatonic feel. For longer sequences, one's natural inclination to stay in the same region of the fretboard, and to repeat at the octave, will guide one when to include the secondary moves.
There is a saying in the arts, "learn the rules, then break them." Often a striking melody is striking because it doesn't conform to a standard scale. Don't be afraid to experiment!
Pentatonic Scales
Every pentatonic scale has 5 modes, but only those modes with a non-dual 5th are listed.
Major and minor scales
The za scales are nearly equipentatonic, dividing the P4 into two nearly equal steps of 8 and 9 arrows (^M2 and vm3). Since one is a 2nd and the other a 3rd, this can conflict with one's categorical perception of intervals. Best to think of them both as "penta-2nds".
| Subgroup | Name | Scale | As a chord | As chains of 5ths | Steps | Step sizes | Step count | Moves | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| ya (2.3.5) | downmajor | P1 | (v)M2 | vM3 | P5 | vM6 | P8 | v6,(v)9 chord | P15M2 vM263 | 7 6 11 - 6 11 | 11 7 6 L/s = 1.83 |
2L 1m 2s or 2L 3s |
+3, -1, -3 |
| " | upminor | P1 | ^m3 | (^)4 | P5 | ^m7 | P8 | ^m7,(^)11 chord | ^m37^4 P415 | 11 6 7 - 11 6 | |||
| za (2.3.7) | downminor | P1 | vm3 | (v)4 | P5 | vm7 | P8 | vm7,(v)11 chord | vm37v4 P415 | 9 8 7 - 9 8 | 9 8 7 L/s = 1.29 |
2L 2m 1s or 2L 3s or 5L |
+4, -2, -3 |
| " | upmajor | P1 | (^)M2 | ^M3 | P5 | ^M6 | P8 | ^6,(^)9 chord | P15M2 ^M263 | 7 8 9 - 8 9 | |||
Pentharmonic and pentsubharmonic scales
These scales are named after the triad implied by the 3rd and 5th, minus the up or down. Note that the pentharmajor scale contains a downminor 7th, and the pentharminor scale contains a downmajor 6th. Likewise with the subharmajor and subharminor scales. A penthardim scale would be P1 ^m3 d5 ^m6 ^m7 P8 = 5:6:7:8:9:10. But it's not very plausible, and would be heard as one of the other modes.
| Subgroup | Name | Scale | As a chord | Steps | Step sizes | Step count | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| yaza (2.3.5.7) |
pentharmajor | P1 | M2 | vM3 | P5 | vm7 | P8 | v9 = 8:9:10:12:14 | 7 6 11 - 9 8 | 11 9 8 7 6 L/s = 1.83 |
1XL 1L 1m 1s 1xs |
| pentharminor | P1 | vm3 | P4 | P5 | vM6 | P8 | vm6,11 = 6:7:8:9:10 | 9 8 7 - 6 11 | |||
| " | pentsubharmajor | P1 | M2 | ^M3 | P5 | ^m7 | P8 | ^9 = 9/(9:8:7:6:5) | 7 8 9 - 11 6 | " | " |
| pentsubharminor | P1 | ^m3 | P4 | P5 | ^M6 | P8 | ^m6,11 = 12/(12:10:9:8:7) | 11 6 7 - 8 9 | |||
| pentsubhardim | P1 | vm3 | d5 | vm6 | vm7 | P8 | vm7(b5),vm6 = 14/(14:12:10:9:8) | 9 11 - 6 7 8 | |||
All five of these scales are "anti-MOS", meaning that each scale step has a unique size. There are many secondary moves, and thus too many types of moves to list. These scales are half ya, half za.
Heptatonic Scales
Major and minor scales
The two ya scales are only modes of each other if the dual notes are included. Same with the two za scales. See below for the other 5 modes (dorian, lydian, etc).
| Subgroup | Name | Scale | As a chord | Steps | Step sizes | Step count | Moves | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
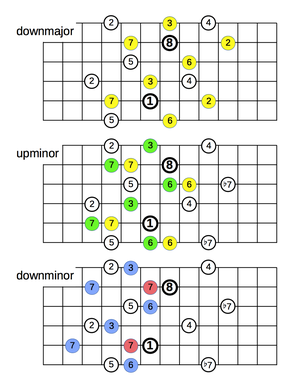
| ya (2.3.5) | downmajor | P1 | (v)M2 | vM3 | P4 | P5 | vM6 | vM7 | P8 | P415M2 vM2637 | 7647-674 | 7 6 4 L/s = 1.75 |
3L 2M 2s or 5L 2s |
+3, +2, -3 |
| " | upminor | P1 | M2 | ^m3 | (^)4 | P5 | ^m6 | ^m7 | P8 | ^m637^4 P415M2 | 7467-476 | |||
| za (2.3.7) | downminor | P1 | M2 | vm3 | (v)4 | P5 | vm6 | vm7 | P8 | vm637v4 P415M2 | 7287-278 | 8 7 2 L/s = 4 |
2L 3M 2s or 5L 2s |
+4, +1, -3 |
| " | upmajor | P1 | (^)M2 | ^M3 | P4 | P5 | ^M6 | ^M7 | P8 | P415M2 ^M2637 | 7827-872 | |||
Altered minor scales
The conventional 12-edo melodic minor and harmonic minor scales are MODMOS scales, modified MOS scales. This is a sort of "macro-dual-ness", where one scale step can vary by an entire semitone. The two harmonic downminor scales have an enormous L/s ratio!
| Subgroup | Name | Scale | As a chord | Steps | Step sizes | Step count | Moves | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| ya (2.3.5) |
harmonic upminor |
P1 | M2 | ^m3 | P4 | P5 | ^m6 | vM7 | P8 | ^m63 P415M2 vM7 | 7467-494 | 9 7 6 4 L/s = 2.25 |
1XL 2L 1m 3s or 1L 3m 2s |
+2, +3, -3, -2 |
| " | melodic upminor |
P1 | M2 | ^m3 | P4 | P5 | vM6 | vM7 | P8 | ^m3 P415M2 vM6 vM7 | 7467-674 | 7 6 4 L/s = 1.75 |
3L 2m 2s or 5L 2s |
+3, +2, -3 |
| za (2.3.7) |
harmonic downminor |
P1 | M2 | vm3 | P4 | P5 | vm6 | ^M7 | P8 | vm63 P415M2 ^M7 | 7287-2,13,2 | 13 8 7 2 L/s = 6.5 |
1XL 1L 2m 3s or 1L 3m 3s |
+1, +4, -3, -0 |
| " | harmonic downminor ^#4 |
P1 | M2 | vm3 | ^A4 | P5 | vm6 | ^M7 | P8 | vm63 P15M2 ^M7^A4 | 7,2,13,2-2,13,2 | 13 7 2 L/s = 6.5 |
2L 1m 4s | +1, -0, -3 |
| " | melodic downminor |
P1 | M2 | vm3 | P4 | P5 | ^M6 | ^M7 | P8 | vm3 P415M2 ^M6 ^M7 | 7287-872 | 8 7 2 L/s = 4 |
2L 3m 2s or 5L 2s |
+4, +1, -3 |
Harmonic and subharmonic scales
These all have the same prime subgroup, yazalatha (2.3.5.7.11.13). They use harmonics 7-14. Adding the 15th harmonic (the bolded note) makes an oct-harmonic scale that uses harmonics 8-16. Again, the scales are named after the triad implied by the 3rd and 5th, minus the up or down. Each scale contains the similarly-named pentatonic scale, e.g. the harmajor scale contains the pentharmajor scale. Subhardim = 14/(14:13:12:11:10:9:8) is a theoretical possibility.
One of the hallmarks of harmonic and subharmonic scales is that each step has a unique size. Unfortunately, in 41edo, these scales do not have unique step sizes, especially the octotonic ones.
| Subgroup | Name | Scale | As a chord | Steps | Step sizes | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| harmajor | P1 | M2 | vM3 | ~4 | P5 | ~6 | vm7 | P8 | 8:9:10:11:12:13:14 | 7665-548 | 8 7 6 5 4 L/s = 2 |
| harminor | P1 | ~2 | vm3 | P4 | P5 | vM6 | ~7 | P8 | 12:13:14:16:18:20:22 | 5487-665 | |
| subharmajor | P1 | M2 | ^M3 | ~4 | P5 | ~6 | ^m7 | P8 | 18/(18:16:14:13:12:11:10) | 7845-566 | |
| subharminor | P1 | ~2 | ^m3 | P4 | P5 | ^M6 | ~7 | P8 | 24/(24:22:20:18:16:14:13) | 5667-845 | |
Oct-harmonic scales can have two 3rds. If so, the scale is named after the one that isn't derived from harmonic 15. This ensures that the oct-harminor scale contains the harminor scale.
| Name | Scale | As a chord | Steps | Step sizes | Step count | Moves | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| oct-harmajor | P1 | M2 | vM3 | ~4 | P5 | ~6 | vm7 | vM7 | P8 | 8:9:10:11:12:13:14:15 | 7665-5444 | 7 6 5 4 L/s = 1.75 |
| oct-harminor | P1 | ~2 | vm3 | vM3 | P4 | P5 | vM6 | ~7 | P8 | 12:13:14:15:16:18:20:22 | 54447-665 | |
| oct-subharmajor | P1 | M2 | ^m3 | ^M3 | ~4 | P5 | ~6 | ^m7 | P8 | 18/(18:16:15:14:13:12:11:10) | 74445-566 | |
| oct-subharminor | P1 | ~2 | ^m3 | P4 | P5 | ^m6 | ^M6 | ~7 | P8 | 24/(24:22:20:18:16:15:14:13) | 5667-4445 | |
The seven diatonic modes
Generalizing major and minor to 41edo is fairly straightforward. The 3rd, 6th and 7th are all grouped together on one end of the genchain of 5ths, and upping or downing them only breaks the genchain of 5ths once. Hence there is only one offperfect 5th, and only one note need become dual to avoid it. But with the other five modes, the chain gets broken twice, and there are two offperfect 5ths, and thus two dual notes. The dual notes are chosen to get six similar triads with a P5. The scales are all yaza except where noted. Most of these scales are not actually modes of each other.
| Name | Scale | As chains of 5ths | Steps | Step sizes | L/s | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| downlydian | P1 | M2 | vM3 | (v)A4 | P5 | (◇)vM6 | vM7 | P8 | P15M26 vM637vA4 A4 | 7683-674 | 8 7 6 4 3 | 2.66 |
| downmajor (ya) | P1 | (v)M2 | vM3 | P4 | P5 | vM6 | vM7 | P8 | P415M2 vM2637 | 7647-674 | 7 6 4 | 1.75 |
| downmixolydian | P1 | (v)M2 | vM3 | P4 | P5 | vM6 | (◇)vm7 | P8 | vm7 m7P415M2 vM263 | 7647-638 | 8 7 6 4 3 | 2.66 |
| updorian | P1 | M2 | ^m3 | (^)4 | P5 | (◇)^M6 | ^m7 | P8 | ^m37^4 P415M26 ^M6 | 7467-836 | 8 7 6 4 3 | 2.66 |
| upminor (ya) | P1 | M2 | ^m3 | (^)4 | P5 | ^m6 | ^m7 | P8 | ^m637^4 P415M2 | 7467-476 | 7 6 4 | 1.75 |
| upphrygian | P1 | (^)m2 | ^m3 | P4 | P5 | ^m6 | (◇)^m7 | P8 | m2 ^m2637 m7P415 | 3867-476 | 8 7 6 4 3 | 2.66 |
| uplocrian | P1 | (^)m2 | ^m3 | P4 | d5 | ^m6 | (◇)^m7 | P8 | d5m2 ^m2637 m7P41 | 3863-876 | 8 7 6 (4) 3 | 2.66 |
| uplydian | P1 | M2 | ^M3 | (^)A4 | P5 | (◇)^M6 | ^M7 | P8 | P15M26 ^M637^A4 A4 | 7863-872 | 8 7 6 3 2 | 4 |
| upmajor (za) | P1 | (^)M2 | ^M3 | P4 | P5 | ^M6 | ^M7 | P8 | P415M2 ^M2637 | 7827-872 | 8 7 2 | 4 |
| upmixolydian | P1 | (^)M2 | ^M3 | P4 | P5 | ^M6 | (◇)^m7 | P8 | ^m7 m7P4152 ^M263 | 7827-836 | 8 7 6 3 2 | 4 |
| downdorian | P1 | M2 | vm3 | (v)4 | P5 | (◇)vM6 | vm7 | P8 | vm37v4 P415M26 vM6 | 7287-638 | 8 7 6 3 2 | 4 |
| downminor (za) | P1 | M2 | vm3 | (v)4 | P5 | vm6 | vm7 | P8 | vm637v4 P415M2 | 7287-278 | 8 7 2 | 4 |
| downphrygian | P1 | (v)m2 | vm3 | P4 | P5 | vm6 | (◇)vm7 | P8 | m2 vm2637 m7P415 | 3687-278 | 8 7 6 3 2 | 4 |
| downlocrian | P1 | (v)m2 | vm3 | P4 | d5 | vm6 | (◇)vm7 | P8 | d5m2 vm2637 m7P41 | 3683-678 | 8 7 6 3 (2) | (4) |
Whereas the major and minor modes have only 3 step sizes, the other modes have 5. Furthermore, the dual-ness affects the step sizes, and the moves can vary. It's possible to make the scales more uniform. They mostly have only 3 step sizes. They mostly become either ya or za. They mostly become modes of each other. The dorian and locrian modes are somewhat problematic.
Five of the seven ya modes (all but dorian and locrian) are formed from this collection of notes:
D ----- A ----- E ----- B \ / \ / \ / \ \ / \ / \ / \ \ / \ / \ / \ ^F ---- ^C ---- ^G ---- ^D
Five of the seven za modes are formed from this collection:
------- ------- ------- \ / \ / \ / \ \ / \ / \ / \ vF \ / vC \ / vG \ / vD \ D ----- A ----- E ----- B
In both cases, the D is dual. But the two dorian scales and the two locrian scales are not from these lattices, and are not actually modes of the other scales.
To be consistent, the two dorian scales should have a dual tonic. To avoid this, and to provide all six triads, there are two dual notes. Note that the 6th of the updorian scale can be downed. Note that this dual-ness affects the step sizes, and 7467-746 can become 7467-656. Thus the moves vary.
To be consistent, the two locrian scales should have an upflat or downflat 5th. To get a plain flat 5th, and thus a more consonant 5:6:7 or 7/(7:6:5) tonic triad, the 5th is dual as well as the 3rd. Again, this dual-ness affects the step sizes and the moves.
| Subgroup | Name | Scale | As a chord | Steps | Step sizes | Step count | Moves | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| ya (2.3.5) |
downlydian | P1 | M2 | vM3 | vA4 | P5 | (v)M6 | vM7 | P8 | P15M26 vM637vA4 | 7674-764 | 7 6 4 L/s = 1.75 |
3L 2M 2s or 5L 2s |
+3, +2, -3 |
| downmajor | P1 | (v)M2 | vM3 | P4 | P5 | vM6 | vM7 | P8 | P415M2 vM2637 | 7647-674 | ||||
| downmixolydian | P1 | vM2 | vM3 | P4 | (v)5 | vM6 | m7 | P8 | m7P415 v5vM263 | 6747-647 | ||||
| upminor | P1 | M2 | ^m3 | (^)4 | P5 | ^m6 | ^m7 | P8 | ^m637^4 P415M2 | 7467-476 | ||||
| upphrygian | P1 | ^m2 | ^m3 | P4 | P5 | ^m6 | (^)m7 | P8 | ^m2637 m7P415 | 4767-467 | ||||
| " | updorian | P1 | M2 | ^m3 | (^)4 | P5 | (v)M6 | ^m7 | P8 | ^m37^4 P415M26 vM6 | 7467-746 | 7 6 (5) 4 | varies | varies |
| " | uplocrian | P1 | ^m2 | (^)m3 | P4 | (^)d5 | ^m6 | m7 | P8 | d5 ^d5^m263 m37P41 | 467 3-867 | (8) 7 6 4 (3) | varies | varies |
| za (2.3.7) |
uplydian | P1 | M2 | ^M3 | ^A4 | P5 | (^)M6 | ^M7 | P8 | P15M26 ^M637^A4 | 7872-782 | 8 7 2 L/s = 4 |
2L 3M 2s or 5L 2s |
+4, +1, -3 |
| upmajor | P1 | (^)M2 | ^M3 | P4 | P5 | ^M6 | ^M7 | P8 | P415M2 ^M2637 | 7827-872 | ||||
| upmixolydian | P1 | ^M2 | ^M3 | P4 | (^)5 | ^M6 | m7 | P8 | m7P415 ^5^M263 | 8727-827 | ||||
| downminor | P1 | M2 | vm3 | (v)4 | P5 | vm6 | vm7 | P8 | vm637v4 P415M2 | 7287-278 | ||||
| downphrygian | P1 | vm2 | vm3 | P4 | P5 | vm6 | (v)m7 | P8 | vm2637 m7P415 | 2787-287 | ||||
| yaza | downdorian | P1 | M2 | vm3 | (v)4 | P5 | (v)M6 | vm7 | P8 | vm37v4 P415M26 vM6 | 7287-728 | 8 7 (6) (3) 2 | varies | varies |
| " | downlocrian | P1 | vm2 | (v)m3 | P4 | (v)d5 | vm6 | m7 | P8 | d5 vd5vm263 m37P41 | 287 3-687 | " | varies | varies |
Near-equidistant Scales
Certain Asian music uses very "lopsided" scales such as P1 M3 P4 P5 M7 P8 (SE Asia) and P1 M2 m3 P5 m6 P8 (Japan). While there is a certain charm to these, scales with equal or roughly equal sizes are also attractive. The only such 12edo scales are the whole tone scale and the full 12-note gamut. Since 41 is a prime number, it has no strictly equal scales. But there are many nearly-equal scales, or near-edos.
If N goes into 41 X times with a remainder of Y, then the near-N-edo scale has steps YL and (N-Y)s, where L=X+1 and s=X. This near-N-edo scale is altered slightly so that there are only 3 odd numbers, and the rest are even. This avoids an awkward scale and also tends to make the intervals well tuned. For example, the unaltered whole-tone scale would have thirds of mostly 14/11 (plain M3) with some 5/4, but the the altered one has thirds of mostly 5/4 with some 9/7.
The alteration is done so that it produces only 1 additional step size which is either 1 arrow larger than L or else 1 arrow smaller than s. If possible (and it often is), the alteration is done so that this new step size occurs only once. This is ideal because almost all steps are within the original L-to-s range, and the original (small) L/s ratio still describes the overall sound of the scale. If the new step size occurs more than once, the 3 step sizes are named L, m and s. If it only occurs once, the new step size is named either XL or xs, for extra large/small. The new step is bolded in the table below. It occurs more than once for near-edos 8 and 12-17, and not at all for near-edos 11 and 19.
| Near-edo | Step sizes | Step count | L/s | # of 5ths | Moves | As chords |
|---|---|---|---|---|---|---|
| 3 | 15 13 | 1L 2s | 1.16 | 0 | -0, --1 | an aug triad |
| 4 | 11 10 9 | 2L 1s 1xs | 1.1 | 0 | +5, -1, -2 | a dim6 or dim7 tetrad |
| 5 | 9 8 7 | 2L 2s 1xs | 1.125 | 3-4 | +4, -2, -3 | a za pentad |
| 6 | 8 7 6 | 1XL 3L 2s | 1.17 | 0 | +3, +4, -3 | 2 aug triads |
| 7 | 7 6 5 | 1XL 4L 2s | 1.2 | 4-5 | +3, -4, -3 | |
| 8 | 6 5 4 | 3L 3m 2s | 1.5 | 1 | +3, +2, -4 | 2 dim6/dim7 tetrads |
| 9 | 6 5 4 | 1XL 3L 5s | 1.25 | +2, +3, -4 | 3 aug triads | |
| 10 | 5 4 3 | 2L 7s 1xs | 1.25 | 5-6 | +2, -4, -5 | 2 za pentads |
| 11 | 4 3 | 8L 3s | 1.33 | 0 | +2, -5 | |
| 12 | 4 3 2 | 7L 3m 2s | 2.0 | +2, +1, -5 | ||
| 19 | 3 2 | 3L 16s | 1.5 | +1, -5 |
These near-equal scales can be used to translate music from a small edo to the Kite guitar.
Tritonic (1L 2s)
Tritonic scales are augmented triads, which are discussed on the chords page. Both moves hop strings, hence have a negative sign. The moves are -0 and --1, meaning same fret up 1 string, and up 1 fret up 1 string.
Tetratonic (2L 1s 1xs)
Tetratonic scales are dim6/dim7 tetrads, which are discussed on the chords page.
Pentatonic (2L 2s 1xs)
We've already seen how the upmajor and downminor pentatonic scales are nearly equi-pentatonic.
Hexatonic (whole tone) (1XL 3L 2s)
There are no perfect 5ths, only tritones. Thus there are no off-5ths, and no motivation for dual-ness. There is only one scale which distributes the 3 large steps equally. There are six modes of this scale. Each mode is a pair of augmented triads. The three 4thward modes have a triad 3 frets above the tonic triad, and the three 5thward modes have it below. All six modes sound similar and are not named individually.
The whole-tone scale is very comfortable to play physically with its alternating +3 and -3 moves. It only spans 4-5 frets and each string has exactly two notes.
| Subgroup | Category | Scale | As augmented triads | Steps | Step sizes | Step count | Moves | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| yaza (2.3.5.7) |
4thward whole-tone |
P1 | vM2 | vM3 | ~4 | vm6 | m7 | P8 | Ivhalf-aug + vIIvaug | 676-787 | 8 7 6 L/s = 1.4 |
1XL 3L 2s or 4L 2s or 6L |
+3, +4, -3 |
| P1 | vM2 | vM3 | A4 | ^m6 | m7 | P8 | Ivaug + vII^aug | 678-767 | |||||
| P1 | ^M2 | ^M3 | A4 | ^m6 | m7 | P8 | I^aug + ^IIvhalf-aug | 876-767 | |||||
| 5thward whole-tone |
P1 | M2 | vM3 | d5 | vm6 | vm7 | P8 | Ivhalf-aug + vbVII^aug | 767-678 | ||||
| P1 | M2 | vM3 | d5 | ^m6 | ^m7 | P8 | Ivaug + ^bVIIvhalf-aug | 767-876 | |||||
| P1 | M2 | ^M3 | ~5 | ^m6 | ^m7 | P8 | I^aug + ^bVIIvaug | 787-676 | |||||
Heptatonic (1XL 4L 2s)
These are reminiscent of 7-edo. The 4th is divided into three nearly equal steps of two vM2's and a ~2 (6 6 5), thus it's also reminiscent of the third-4th pergen and the Triyo temperament. Unfortunately, obvious near-equal scales like P1 ~2 ~3 P4 P5 ~6 ~7 P8 = 5757-575 or P1 vM2 ~3 ^4 P5 vM6 ~7 P8 = 6666-665 are very awkward to play.
The two main scales are equi-major and equi-minor. Equi-minor is somewhat like maqam Huseyni or maqam Bayati. Equi-major is equi-minor octave-inverted.
These scales can be derived from the seven ya modes by widening the two smallest steps by 1 or 2 arrows, from an upminor 2nd to a mid or downmajor 2nd. The tonic triad is never altered by the widening, thus equi-lydian and equi-mixolydian would be the same as equi-major, and equi-phrygian the same as equi-minor.
The standard downmajor and upminor scales have two chains of 5ths. Only one dual note linking them is needed to avoid an offperfect fifth. But these scales have three chains, and would need two dual notes. They are shown here with only one dual note, thus they have an offperfect 5th. To avoid that 5th, either the 3rd must be dual, which destroys the character of the scale, or else there must be a step of 4 arrows which inflates the L/s ratio and destroys the near-equal feel. The two equi-mid scales each have two offperfect 5ths.
These scales are harmonic or subharmonic series fragments. Equi-major is (8:9:10:11:12)/8 plus (9:10:11:12)/6. Equi-mid is (9:10:11:12)/9 + (8:9:10:11:12)/6. Equi-minor is 12/(12:11:10:9:8) + 18/(12:11:10:9).
| Subgroup | Name | Scale | As chains of 5ths | Steps | Step sizes | Step count | M oves | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| yala (2.3.5.11) |
equi-major | P1 | (v)M2 | vM3 | ~4 | P5 | vM6 | ~7 | P8 | P152 vM263 ~74 | 7665-665 | 7 6 5 L/s = 1.4 |
1L 4m 2s or 5L 2s or 7L |
+3, -4, -3 |
| equi-mid | P1 | vM2 | ~3 | P4 | P5 | vM6 | ~7 | P8 | P415 vM26 ~37 | 6657-665 | ||||
| equi-dorian | P1 | vM2 | ^m3 | (^)4 | P5 | vM6 | ^m7 | P8 | ^m37^4 P415 vM26 | 6567-656 | ||||
| " | equi-minor | P1 | ~2 | ^m3 | (^)4 | P5 | ~6 | ^m7 | P8 | ~26 ^m37^4 P415 | 5667-566 | " | " | " |
| equi-mid ~4 | P1 | vM2 | ~3 | ~4 | P5 | vM6 | ~7 | P8 | P15 vM26 ~374 | 6675-665 | ||||
Octotonic (3L 3m 2s)
The Bohlen–Pierce 13-edt scale is a non-octave scale that is contained in 41-edo. (Technically, the 41edo scale is 13-edt stretched by half a cent.) B-P has only one step size, a mid 2nd of 5 arrows. These steps are very near to one-eighth of an octave, so it can be thought of as a near-8edo scale. Unfortunately B-P is very awkward to play on the Kite guitar. It also has the dissonant ^5, v8 and M10 intervals. As with the other scales, we can avoid the dissonance by shifting some of the notes by a single arrow. We must shift three notes, and shifting one more also makes the scale non-awkward. Instead of 13 equal steps 5 5 5 5 5 5 5 5 5 5 5 5 5, we have 5 6 4 5 6 4 5 6 4 5 6 4 5. The moves are +3 +2 -4. This scale now includes a perfect 8ve, which implies an octave-repeating version of it that is 5 6 4 5 6 4 5 6. This is one form of the octotonic 3L 3m 2s scale.
The 3rd step size (4 arrows) occurs twice in octotonic scales, making them less near-equal and less MOS-like than the other near-equal scales so far. Every scale contains a section of 2L 1m, making that section identical to an equi-heptatonic tetrachord. For example, the first scale in the table below has P5 ~6 ^m7 P8, as does the equi-minor scale. Every scale also contains a section of 2L 1s, making a down-4th, and thus an up-5th upon octave inversion. Every scale also contains 1L 2m, which makes another down-4th. Fortunately, octotonic chords are naturally constructed from stacking "octa-thirds", i.e. using every other note of the scale. Chords avoid both perfect and off-perfect 5ths in favor of the dim 5th. The down-4th is likewise avoided.
Because of the prominence of the "octa-5th" (i.e. tritone) in octatonic chords, this interval plays a role analogous to the perfect 5th in other scales. Every octotonic scale contains eight tritones. The most consonant tritone is the dim 5th = 7/5. Of course all eight tritones can't be dim 5ths without dual-ness, but half of them can be. In particular, the tonic chord can be a dim7 chord that contains two dim 5ths. The only two such chords that are playable are the ^dim7 and vdim7 chords. If we require that the remaining four notes of the scale make another such chord, there are only three near-equal octotonic scales. Each has two main modes, depending on which of the dim7 chords is considered to be the tonic chord.
The scales are named after the root of the non-tonic dim7 chord. This chord is always upped or downed (^d7 vs. vd7) to match the root. If the tonic chord is upped or downed the opposite way, the two dim7 chords, and hence the entire scale, can easily be deduced from the name: the upflat-2 octotonic scale has an updim7 chord on the upflat II and a downdim7 chord on the I. The octave inverse of ^b2 is vM7, thus the other main mode of the upflat-2 scale is the down-7 scale. If the tonic chord is upped or downed the same way, we must add that direction to the name: the up-3 up scale has an updim7 chord on up-III and an updim7 chord on I.
| Subgroup | Name | Scale | As dim7 tetrads | Steps | Step sizes | Step count | Moves | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| yaza (2.3.5.7) |
upflat-2 | P1 | ^m2 | vm3 | ^M3 | d5 | P5 | ~6 | ^m7 | P8 | Ivd7 + ^bII^d7 | 4565-4566 | 6 5 4 L/s = 1.5 |
3L 3m 2s or 8L |
+3, +2, -4 |
| down-7 | P1 | ~2 | ^m3 | v4 | d5 | ^5 | M6 | vM7 | P8 | I^d7 + vVIIvd7 | 5654-5664 | ||||
| " | down-2 | P1 | vM2 | ^m3 | ^M3 | d5 | vm6 | M6 | ^m7 | P8 | I^d7 + vIIvd7 | 6545-6546 | " | " | " |
| upflat-7 | P1 | ~2 | vm3 | M3 | d5 | ^5 | ~6 | ^m7 | P8 | Ivd7 + ^bVII^d7 | 5456-5466 | ||||
| " | up-3 up | P1 | ~2 | ^m3 | ^M3 | d5 | vm6 | M6 | ^m7 | P8 | I^d7 + ^III^d7 | 5645-6546 | " | " | " |
| downflat-6 up | P1 | ~2 | ^m3 | v4 | d5 | vm6 | M6 | vM7 | P8 | I^d7 + vbVI^d7 | 5654-6564 | ||||
Enneatonic (nine-tone)
(TODO: expand) C vD vEb ^E ^F G ^Ab vBb vB C
Dodecatonic (twelve-tone) (7L 3m 2s)
"The Flight of the Bumblebee" has simple 5-limit triads, but a scale that is clearly dodecatonic. The evenly-spaced 12edo scale is quite fitting for this piece, nicely evoking the random movements of flying insects. How would this piece translate to the Kite Guitar? Poorly, because the scale would be either very awkward to play (all plain notes, lots of hopping between strings), or very uneven, with an L/s ratio of at least 2.
Here is one mode of the 3-limit pythagorean dodecatonic scale, which is the closest one can get to 12-edo on the Kite Guitar. There are 7 small steps of 3 arrows, each of which requires a string-hop. The moves column is deceptive, because repeated -5 moves will push one to the nut and make one run out of frets. Thus some of the -5 moves will have to be +8 frets but -1 string.
| Subgroup | Name | Scale | As dim7 tetrads | Steps | Step sizes | Step count | Moves | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| wa (2.3) |
3-limit dodecatonic |
P1 | m2 | M2 | m3 | M3 | P4 | A4 | P5 | m6 | M6 | m7 | M7 | P8 | m2637P415M2637A4 | 3434-343-
34343 |
4 3 L/s = 1.33 |
5L 7s or 12L |
+2, -5 |
The obvious uneven scale is the harmonic duodene, with 3 dual notes to avoid offperfect 5ths. Note that the dual major 2nd and dual minor 7th affect the step sizes. Both vM2-^m3 and vM6-^m7 are 5 arrows. The step count and moves are affected as well.
| Subgroup | Name | Scale | As chains of 5ths | Steps | Step sizes | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| ya (2.3.5) |
harmonic duodene |
P1 | ^m2 | (v)M2 | ^m3 | vM3 | P4 | (v)A4 | P5 | ^m6 | vM6 | (^)m7 | vM7 | P8 | A4^m2637 m7P415M2 vM2637vA4 | 4342-443-
42434 |
(5) 4 3 2 L/s = 2.5 |
For an even scale with small steps that's not awkward, see the next section.
Decatonic - the semitonal scale or twin pentatonic scale (2L 7s 1xs)
Is there an easily playable chromatic-sounding scale with nearly equal steps? One such is the decatonic scale. The precise term for these scales is not chromatic but semitonal, because the steps are roughly the size of a 12edo semitone. Fretwise refers to movement by a single fret, see the section on 19-tone scales. Microtonal refers to movement by a half-fret, see the final section. Chromatic includes semitonal, fretwise, and microtonal.
If the steps are nearly equal, it follows that every other note will make a nearly-equal pentatonic scale. Thus these scales consist of two intertwined za pentatonic scales. If we further require that the two scales be either upmajor or downminor, there are only 3 such scales, each with two primary modes. The modes are named after the "one" of the non-tonic scale, similar to how octotonic scales are named.
The twin downminor scale consists of two downminor pentatonic scales, offset from each other by two frets. Likewise with the twin upmajor scale. The twin downminor's down-7 mode is (12:13:14:15:16:17:18)/12 plus (12:13:14:15:16)/8, except that prime 17 isn't well tuned.
The "mixed" scale that uses both upmajor and downminor is distributionally even, and each string has 3 or 4 notes. The two unmixed "twin" scales are less even, and one string holds only 2 notes. In both the mixed and unmixed scales, there are only three types of each interval, except the "deca-6th" which has 4 types (~4, d5, A4 and ~5).
| subgroup | Name | Scale | Steps | Step sizes | Step count | Moves | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| yazala (2.3.5.7.11) |
twin downminor down-7 | P1 | ~2 | vm3 | vM3 | P4 | d5 | P5 | ~6 | vm7 | vM7 | P8 | 544-434-5444 | 5 4 3 L/s = 1.67 |
2L 7m 1s or 2L 8s or 10L |
+2, -4, -5 |
| twin downminor upflat-2 | P1 | ^m2 | vm3 | vM3 | P4 | A4 | P5 | ^m6 | vm7 | vM7 | P8 | 454-443-4544 | ||||
| " | twin upmajor down-7 | P1 | m2 | M2 | ^m3 | ^M3 | d5 | P5 | ^m6 | ^M6 | vM7 | P8 | 344-454-4454 | " | " | " |
| twin upmajor upflat-2 | P1 | ^m2 | M2 | ^m3 | ^M3 | ~4 | P5 | ^m6 | ^M6 | ~7 | P8 | 434-445-4445 | ||||
| " | downminor + down-3 upmajor | P1 | ^m2 | vm3 | vM3 | P4 | d5 | P5 | ^m6 | vm7 | vM7 | P8 | 454-434-4544 | " | " | " |
| upmajor + upflat-6 downminor | P1 | ^m2 | M2 | ^m3 | ^M3 | d5 | P5 | ^m6 | ^M6 | vM7 | P8 | 434-454-4454 | ||||
The twin downminor down-7 scale works well for the blues. It lacks a M2, so over the V chord, shift the scale so that it's rooted on the 5th. Likewise shift the root to the 4th over the IV chord.
| Subgroup | Name | Scale | Steps | Step sizes | Step count | Moves | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| yazala (2.3.5.7.11) |
twin downminor down-7 on I | P1 | ~2 | vm3 | vM3 | P4 | d5 | P5 | ~6 | vm7 | vM7 | P8 | 544-434-5444 | 5 4 3 L/s = 1.67 |
2L 7m 1s or 2L 8s or 10L |
+2, -4, -5 |
| twin downminor down-7 on IV | P1 | ~2 | vm3 | vM3 | P4 | ~5 | vm6 | vM6 | m7 | vM7 | P8 | 544-45-44434 | ||||
| twin downminor down-7 on V | P1 | m2 | M2 | ~3 | v4 | d5 | P5 | ~6 | vm7 | vM7 | P8 | 345-444-5444 | ||||
Eleven-tone - The checkerboard scale (8L 3s)
This scale gets its name from the fact that it uses every other fret of each string, and each string's notes are offset by one fret from the neighboring strings -- thus the scale chart looks like an actual checkerboard. It's a MOS scale and therefore doesn't need a 3rd step size or a 3rd move. It's generated by the ^M3, which is 15\41. The complete genchain containing all 11 modes runs from -10 generators to +10:
M3 ~6 m2 ^4 vm7 M2 ~5 vM7 ^m3 vm6 P1 ^M3 vM6 ^m2 ~4 m7 ^M2 v5 M7 ~3 m6
P4 and P5 do not appear here. Eventually, P5 occurs at +18 ~9/7 generators, which would imply the Sasa-tritribizo comma [5 -35 0 18> and the extremely complex pergen (P8, c6P5/18). However, one 3-limit interval occurs much sooner. 16/9 = m7 occurs at only +5 generators, meaning that this temperament can be considered to be the 2.9.15.7 subgroup with 225/224 & 245/243 vanishing. This implies the much simpler and hence much more useful pergen (P8, cm7/5). The color name is Laquinyo & Ruyoyo wawa, where "wawa" is a slangy term for using 9 not 3 in the subgroup. Adding prime 11 to the subgroup adds the Luyoyo comma to the name.
Checkerboard[5] and Checkerboard[8] are also MOS scales, with L/s ratios of 2.75 and 1.75 respectively. Checkerboard[8] is 3L 5s (TAMNAMS name checkertonic) and Checkerboard[5] is 3L 2s.
11 generators add up to 4 octaves and an ^1, showing how very near 11-edo this scale is. 7 generators add up to a down-5th, thus all modes contain 4 down-5ths. The 11 modes of Checkerboard[11] are listed here in order from sharpest to flattest. The notes that differ from the neighboring modes are bolded.
| subgroup | mode | scale | genchain | steps | step sizes | step count | moves | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| yazala (2.3.5.7.11) |
#1 | P1 | ^m2 | ^M2 | ~3 | ^M3 | ~4 | v5 | m6 | vM6 | m7 | M7 | P8 | P1 ... m6 | 444-3444-344-3 | 4 3 L/s = 1.33 |
8L 3s | +2, -5 |
| #2 | P1 | ^m2 | ^M2 | ~3 | ^M3 | ~4 | v5 | vm6 | vM6 | m7 | M7 | P8 | vm6 ... ~3 | 444-344-3444-3 | ||||
| #3 | P1 | ^m2 | ^M2 | ^m3 | ^M3 | ~4 | v5 | vm6 | vM6 | m7 | M7 | P8 | ^m3 ... M7 | 44-3444-3444-3 | ||||
| #4 | P1 | ^m2 | ^M2 | ^m3 | ^M3 | ~4 | v5 | vm6 | vM6 | m7 | vM7 | P8 | vM7 ... v5 | 44-3444-344-34 | ||||
| #5 | P1 | ^m2 | ^M2 | ^m3 | ^M3 | ~4 | ~5 | vm6 | vM6 | m7 | vM7 | P8 | ~5 ... ^M2 | 44-344-3444-34 | ||||
| #6 | P1 | ^m2 | M2 | ^m3 | ^M3 | ~4 | ~5 | vm6 | vM6 | m7 | vM7 | P8 | M2 ... m7 | 4-3444-3444-34 | ||||
| #7 | P1 | ^m2 | M2 | ^m3 | ^M3 | ~4 | ~5 | vm6 | vM6 | vm7 | vM7 | P8 | vm7 ... ~4 | 4-3444-344-344 | ||||
| #8 | P1 | ^m2 | M2 | ^m3 | ^M3 | ^4 | ~5 | vm6 | vM6 | vm7 | vM7 | P8 | ^4 ... ^m2 | 4-344-3444-344 | ||||
| #9 | P1 | m2 | M2 | ^m3 | ^M3 | ^4 | ~5 | vm6 | vM6 | vm7 | vM7 | P8 | m2 ... vM6 | 3444-3444-344 | ||||
| #10 | P1 | m2 | M2 | ^m3 | ^M3 | ^4 | ~5 | vm6 | ~6 | vm7 | vM7 | P8 | ~6 ... ^M3 | 3444-344-3444 | ||||
| #11 | P1 | m2 | M2 | ^m3 | M3 | ^4 | ~5 | vm6 | ~6 | vm7 | vM7 | P8 | M3 ... P1 | 344-3444-3444 | ||||
The scale completely lacks perfect 4ths and 5ths, giving it a very unusual sound. But it's full of low-odd-limit ratios, so it sounds both alien and harmonious. There are many augmented and diminished chords. Any three adjacent notes on the genchain form Bohlen–Pierce's up-sesquiaug chord P1 ^M3 ^^#5 (aka up-down6-no5 = P1 ^M3 vM6), thus every scale has nine such chords. Likewise nine each of that chord's homonyms, up-halfaug (P1 ^M3 ^^5=vm6) and upminor-halfaug (P1 ^m3 ^^5=vm6). There are also seven upminor-mid5 chords (P1 ^m3 ~5) and five upminor-down7-mid5 chords (P1 ^m3 ~5 vm7).
Because it's a MOS scale, each column between P1 and P8 in the table above contains only two types of interval. Melodically, the smaller intervals sound vaguely like 12-edo:
- 1 step = minorish 2nd = 88¢ or 117¢
- 2 steps = majorish 2nd = 205¢ or 234¢
- 3 steps = minorish 3rd = 322¢ or 351¢
- 4 steps = majorish 3rd = 410¢ or 439¢
- 5 steps = middish 4th = 527¢ or 556¢
- 6 steps = middish 5th = 644¢ or 673¢
Nineteen-tone - The fretwise scale (3L 16s)
There's not much to say about these scales. All the modes sound fairly similar, and there's not much reason to name them individually. Using the full 19 note scale is somewhat overkill, unless your song is about bumblebees.
The one-fret step implies several different ratios, and doesn't imply any particular prime subgroup. The L/s ratio is 1.5. The moves are +1 and -5. If there are 6 or 7 notes per string, it's a MOS scale of the Laquinyo temperament, which has a (P8, P12/5) pergen. If not, it's a MODMOS scale of Laquinyo. For example, the 2nd scale in the table is MODMOS because the large steps are not evenly distributed throughout the scale.
| Name | Scale | Steps | |||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| fretwise[19] | P1 | vm2 | ^m2 | (v)M2 | vm3 | ^m3 | vM3 | ^M3 | P4 | d5 | ~5 | P5 | vm6 | ^m6 | vM6 | vm7 | ^m7 | vM7 | ^M7 | P8 | 223222-22322-2223-2222 |
| " | P1 | vm2 | ^m2 | vM2 | ^M2 | ^m3 | vM3 | ^M3 | P4 | ~4 | ~5 | P5 | vm6 | ^m6 | vM6 | ^M6 | ^m7 | vM7 | ^M7 | P8 | 222232-22232-2222-3222 |
Although the full scale is a bit much, pieces of it are nice. Fretwise melodies with movements of a single fret are quite novel and pleasant. 59¢ is just barely large enough to sound like a 2nd and not a quartertone. Any of the 5, 7, and 10-note scales can be spiced up with fretwise movement.
The fretwise scale can be used to translate a 19edo piece to 41edo. Just write out the piece in tablature for a 19edo guitar tuned in major thirds, and play the tablature on the Kite guitar.
One can make a scale that's not very even, but still quite interesting, by using a smaller MOS, for example 13. This scale is 3 fretwise runs separated by 3 major 2nds.
| Name | Scale | Steps | Step sizes | Step count | Moves | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| fretwise[13] | P1 | vm2 | ^m2 | ^m3 | vM3 | ^M3 | P4 | P5 | vm6 | ^m6 | vM6 | ^M6 | ^M7 | P8 | 2272-227-222272 | 7 2, L/s = 3.5 | 3L 10s | +2, -3 |
Microtonal scales
These scales use step sizes of 1 and 2 arrows only. They are quite awkward to play, with much string-hopping and fret-leaping. Unless there are only 3 small steps, in which case it is a 22-note MOS or MODMOS of the Laquinyo temperament.
Non-awkward MOS scales
See the discussion at Kite Giedraitis's Categorizations of 41edo Scales. It also discusses the many non-octave MOS scales. Checkerboard[11] is discussed in the eleven-tone section. Checkerboard can be thought of as every other note of the Laquinyo genchain. Likewise, Tritriyo is every fourth note, and Pythagorean is every fifth note.
| Name | Pergen | Henerator | MOS scales | L & s | Moves | Fretboard footprint | Notes | ||
|---|---|---|---|---|---|---|---|---|---|
| Tritriyo (Smitonic) |
(P8, ccP4/9) | 11\41 | ^m3 | (+1,-1) | 7 = 4L 3s | 8 3 | +4, -5 | backwards diagonal lines 4 frets apart |
no perfect 4ths or 5ths |
| Laquinyo (Magic) |
(P8, P12/5) | 13\41 | vM3 | (+1,0) | 7 = 3L 4s | 11 2 | +1, -1 | straight lines 1 fret apart |
fretwise scales |
| 10 = 3L 7s | 9 2 | +1, -2 | |||||||
| 13 = 3L 10s | 7 2 | +1, -3 | |||||||
| 16 = 3L 13s | 5 2 | +1, -4 | |||||||
| 19 = 3L 16s | 3 2 | +1, -5 | |||||||
| Checkerboard (Laquinyo wawa) |
(P8, cm7/5) | 15\41 | ^M3 | (+1,+1) | 5 = 3L 2s | 11 4 | +2, -1 | forwards (and backwards) diagonal lines 2 frets apart |
uses the 2.9.15.7 subgroup, hence no perfect 4ths or 5ths |
| 8 = 3L 5s | 7 4 | +2, -3 | |||||||
| 11 = 8L 3s | 4 3 | +2, -5 | |||||||
| Pythagorean | (P8, P5) | 17\41 | P4 | (+1,+2) | 5 = 2L 3s | 10 7 | +5, -3 | extremely forwards diagonal lines 5 frets apart |
very close to 12-edo |
Other scales
6L1s scales
41edo contains an exact 6L1s scale with steps 6666-665, but it's very awkward to play. 41edo can approximate 6L1s with L = 6\41 or 7\41 and s = 3\41. There are only three patterns that avoid adjacent odd numbers, which would make three consecutive notes on different strings:
- up pattern: 6 7 6 7 6 6 3
- sym pattern: 6 7 6 6 7 6 3 (sym = inversionally symmetric)
- down pattern: 6 6 7 6 7 6 3
The scales sound somewhat similar to the hexatonic scales discussed above. No mode contains a P4 or P5.
In general, upping a single note in the sym pattern creates the up pattern of that mode. Likewise for the down pattern. For each mode, notes that differ between the up and sym pattern are bolded. Notes that differ between the sym and down pattern are underlined.
| down pattern | sym pattern | up pattern | ||||
|---|---|---|---|---|---|---|
| scale | steps | scale | steps | scale | steps | |
| #1 | P1 vM2 ~3 ~4 ^5 ^M6 M7 P8 | 6 6 7 6 7 6 3 | P1 vM2 vM3 ~4 ^5 ^M6 M7 P8 | 6 7 6 6 7 6 3 | P1 vM2 vM3 ~4 ^^5 ^M6 M7 P8 | 6 7 6 7 6 6 3 |
| #2 | P1 vM2 vM3 ~4 ^^5 ^M6 ^m7 P8 | 6 7 6 7 6 3 6 | P1 M2 vM3 ~4 ^^5 ^M6 ^m7 P8 | 7 6 6 7 6 3 6 | P1 M2 vM3 vA4 ^^5 ^M6 ^m7 P8 | 7 6 7 6 6 3 6 |
| #3 | P1 M2 vM3 vA4 ^^5 ~6 ^m7 P8 | 7 6 7 6 3 6 6 | P1 vM2 ~3 ~4 ^5 ^m6 m7 P8 | 6 6 7 6 3 6 7 | P1 vM2 vM3 ~4 ^5 ^m6 m7 P8 | 6 7 6 6 3 6 7 |
| #4 | P1 vM2 vM3 ~4 ~5 ^m6 m7 P8 | 6 7 6 3 6 6 7 | P1 vM2 vM3 ~4 ~5 ^m6 ^m7 P8 | 6 7 6 3 6 7 6 | P1 M2 vM3 ~4 ~5 ^m6 ^m7 P8 | 7 6 6 3 6 7 6 |
| #5 | P1 M2 vM3 v4 ~5 ^m6 ^m7 P8 | 7 6 3 6 6 7 6 | P1 M2 vM3 v4 ~5 ~6 ^m7 P8 | 7 6 3 6 7 6 6 | P1 vM2 ~3 vv4 ^d5 ^m6 m7 P8 | 6 6 3 6 7 6 7 |
| #6 | P1 vM2 vm3 vv4 ^d5 ^m6 m7 P8 | 6 3 6 6 7 6 7 | P1 vM2 vm3 vv4 ~5 ^m6 m7 P8 | 6 3 6 7 6 6 7 | P1 vM2 vm3 vv4 ~5 ^m6 ^m7 P8 | 6 3 6 7 6 7 6 |
| #7 | P1 m2 vm3 vv4 ~5 ^m6 ^m7 P8 | 3 6 6 7 6 7 6 | P1 m2 vm3 v4 ~5 ^m6 ^m7 P8 | 3 6 7 6 6 7 6 | P1 m2 vm3 v4 ~5 ~6 ^m7 P8 | 3 6 7 6 7 6 6 |
To find the mode with the most notes in common, read the table from left to right, top to bottom. There are three series of 7 modes each:
- down #1 -- sym #1 -- up #1 -- down #2 -- sym #2 -- up #2 -- down #3
- sym #3 -- up #3 -- down #4 -- sym #4 -- up #4 -- down #5 -- sym #5
- up #5 -- down #6 -- sym #6 -- up #6 -- down #7 -- sym #7 -- up #7
5L 3s scales
41edo contains an exact 5L 3s scale with steps 77277272, but it's very awkward to play. 41edo can approximate 5L3s with L = 6\41 or 8\41 and s = 3\41. This gives rise to five patterns, each of which has 8 modes. The LLsLLsLs mode of each pattern:
- upper pattern: 8 6 3 6 6 3 6 3
- up pattern: 6 8 3 6 6 3 6 3
- down pattern: 6 6 3 8 6 3 6 3
- downer pattern: 6 6 3 6 8 3 6 3
- sym pattern: 6 6 3 6 6 3 8 3 (sym = inversionally symmetric)
Each pattern contains three P5's except the sym pattern which has only two. Modes that contain a P5 from the tonic are bolded.
| upper | up | down | downer | sym | |
|---|---|---|---|---|---|
| #1 | 8 6 3 6 6 3 6 3 | 6 8 3 6 6 3 6 3 | 6 6 3 8 6 3 6 3 | 6 6 3 6 8 3 6 3 | 6 6 3 6 6 3 8 3 |
| #2 | 6 6 3 6 3 8 6 3 | 6 6 3 6 3 6 8 3 | 8 6 3 6 3 6 6 3 | 6 8 3 6 3 6 6 3 | 6 6 3 8 3 6 6 3 |
| #3 | 6 3 8 6 3 6 6 3 | 6 3 6 8 3 6 6 3 | 6 3 6 6 3 8 6 3 | 6 3 6 6 3 6 8 3 | 8 3 6 6 3 6 6 3 |
| #4 | 6 3 6 6 3 6 3 8 | 8 3 6 6 3 6 3 6 | 6 3 8 6 3 6 3 6 | 6 3 6 8 3 6 3 6 | 6 3 6 6 3 8 3 6 |
| #5 | 6 3 6 3 8 6 3 6 | 6 3 6 3 6 8 3 6 | 6 3 6 3 6 6 3 8 | 8 3 6 3 6 6 3 6 | 6 3 8 3 6 6 3 6 |
| #6 | 3 8 6 3 6 6 3 6 | 3 6 8 3 6 6 3 6 | 3 6 6 3 8 6 3 6 | 3 6 6 3 6 8 3 6 | 3 6 6 3 6 6 3 8 |
| #7 | 3 6 6 3 6 3 8 6 | 3 6 6 3 6 3 6 8 | 3 8 6 3 6 3 6 6 | 3 6 8 3 6 3 6 6 | 3 6 6 3 8 3 6 6 |
| #8 | 3 6 3 8 6 3 6 6 | 3 6 3 6 8 3 6 6 | 3 6 3 6 6 3 8 6 | 3 6 3 6 6 3 6 8 | 3 8 3 6 6 3 6 6 |
Alternatively, one of the small steps can be 4-arrow (^m2 or A1). There are only four patterns that avoid adjacent odd numbers, which would make three consecutive notes on different strings:
- up pattern: 7 6 3 6 6 3 6 4
- down pattern: 6 6 3 6 7 4 6 3
- up2 pattern: 6 7 4 6 6 3 6 3
- down2 pattern: 6 6 4 7 6 3 6 3
The last two patterns have 4 notes on just one string, awkwardly spanning 8 frets. Again, modes with a P5 from the tonic are bolded. The upper tetrachord of these modes is often a familiar diatonic one like 6 7 4 or 7 4 6 or 4 7 6.
| up | down | up2 | down2 | |
|---|---|---|---|---|
| #1 | 7 6 3 6 6 3 6 4 | 6 6 3 6 7 4 6 3 | 6 7 4 6 6 3 6 3 | 6 6 4 7 6 3 6 3 |
| #2 | 6 6 3 6 4 7 6 3 | 6 7 4 6 3 6 6 3 | 6 6 3 6 3 6 7 4 | 7 6 3 6 3 6 6 4 |
| #3 | 6 4 7 6 3 6 6 3 | 6 3 6 6 3 6 7 4 | 6 3 6 7 4 6 6 3 | 6 3 6 6 4 7 6 3 |
| #4 | 6 3 6 6 3 6 4 7 | 6 3 6 7 4 6 3 6 | 7 4 6 6 3 6 3 6 | 6 4 7 6 3 6 3 6 |
| #5 | 6 3 6 4 7 6 3 6 | 7 4 6 3 6 6 3 6 | 6 3 6 3 6 7 4 6 | 6 3 6 3 6 6 4 7 |
| #6 | 4 7 6 3 6 6 3 6 | 3 6 6 3 6 7 4 6 | 3 6 7 4 6 6 3 6 | 3 6 6 4 7 6 3 6 |
| #7 | 3 6 6 3 6 4 7 6 | 3 6 7 4 6 3 6 6 | 4 6 6 3 6 3 6 7 | 4 7 6 3 6 3 6 6 |
| #8 | 3 6 4 7 6 3 6 6 | 4 6 3 6 6 3 6 7 | 3 6 3 6 7 4 6 6 | 3 6 3 6 6 4 7 6 |