The 16 most stable triads of 22edo

The 16 most stable triads of 22-TET, from empirical data, notated in Ups and Downs. Triads represent inversional equivalence classes and are written in normal form, with a root of zero. The second and third numbers in the triple represent the lower and outer intervals in degrees of 22-TET respectively. Triples describe the inversion for which the outer interval is the smallest, and, in the case that two inversion have the same sized outer interval, that the lower interval is the smallest. Triads are notated in root position [Note: The inversion of triads are notated in does not always match the inversion their normal form most immediately describes

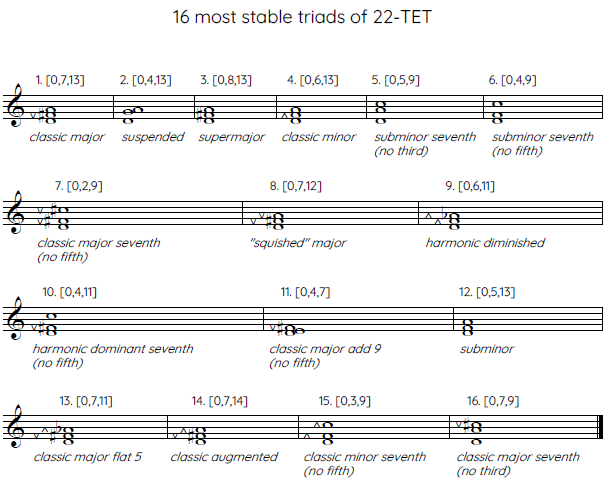
Click the triad for an audio sample

Rank	Triad	Name	Size in cents	Ratios approximated	Relative Instability
1	[0,7,13]	Classic major triad	382 709	5/4, 6/5, 4/3, 4:5:6	0
2	[0,4,13]	Suspended triad	491 709	4/3, 8/7, 4/3, 6:8:9	0.34
3	[0,8,13]	Supermajor triad	436 709	9/7, 7/6, 4/3, 14:18:21	0.40
4	[0,6,13]	Classic minor triad	327 709	6/5, 5/4, 4/3, 10:12:15	0.53
5	[0,5,9]	Subminor seventh (no third)	709 982	3/2, 7/6, 8/7, 4:6:7	0.53
6	[0,4,9]	Subminor seventh (no fifth)	273 982	7/6, 3/2, 8/7, 12:14:21	0.71
7	[0,2,9]	Classic major seventh (no fifth)	382 1091	5/4, 3/2, 15/14, 8:10:15	0.78
8	[0,7,12]	"Squished" major triad	382 655	5/4, 7/6, 11/8, 24:30:35	0.84
9	[0,6,11]	Harmonic diminished triad	327 600	6/5, 7/6, 7/5, 5:6:7	0.86
10	[0,4,11]	Harmonic dominant seventh (no fifth)	382 982	5/4, 7/5, 8/7, 4:5:7	0.96
11	[0,4,7]	Classic major add 9 (no fifth)	218 382	8/7, 10/9, 8/5, 8:9:10	0.99
12	[0,5,13]	Subminor triad	273 709	7/6, 9/7, 4/3, 6:7:9	1.03
13	[0,7,11]	Classic major flat 5	382 600	5/4, 8/7, 7/5, 12:15:17	1.06
14	[0,7,14]	Classic augmented triad	382 764	5/4, 5/4, 9/7, 16:20:25	1.08
15	[0,3,9]	Classic minor seventh (no fifth)	327 1036	6/5, 3/2, 10/9, 5:6:9	1.09
16	[0,7,9]	Classic major seventh (no third)	709 1091	3/2, 5/4, 15/14, 8:12:15	1.10