Armodue theory
(summary translation from the Italian language site Armodue )
Armodue theory is a xenharmonic notation and theory system for 16-note tuning, not only in the sense of 16-tone equal temperament, but also regarding half-equal tuning, Lou Harrison's Just intonation 16 note scale, the natural octave division by Andrián Pertout, and the 16-to-31 overtone scale.
Notation
In order to make Armodue approachable, while also making its notation distinct from Western diatonic notation, the Italian creators of the Armodue system named the notes using numbers from 1 to 9:
1, 1#, 2, 2#, 3, 3#, 4, 5, 5#, 6, 6#, 7, 7#, 8, 8#, 9
This is the recommended system for Armodue theory, though in technicality any notation system can be used.
Relative notation is simply done by specifying the number of edosteps, in order to support alternative scale constructions. For example, one refers not to the perfect fourth or fifth (at least in the specific sense of Armodue intervals) but to the intervals of 7-eka and 9-eka.
However, the interval between a note at frequency n and other at frequency 2n is called a tenth, decave, or decim, as it is the tenth note of the Mavila[9] scale.
The basic semitone of Armodue, whatever concrete temperament is used, is always called eka (from Sanskrit eka: one, unit). In the chromatic Armodue scale, one eka always corresponds to the interval between any two consecutive notes.
The standard staff notation for Armodue uses 4 lines, so that 1 is always found below the first line, and 9 above the last line.

The notes without accidentals form a Mavila armotonic scale (7L 2s).
This notation is similar to the KISS notation using numbers for this scale, except with the staff laid out differently (i.e. in KISS, the standard treble and bass clefs are used, and the staff has 6 lines instead of 4)
When multiple staves are necessary, the notes will be written in the same way in every staff.
In other words, the "1" note will be written immediately under the first line in every tenth.
In Armodue we have only a numeric clef, that shows us the tenth:
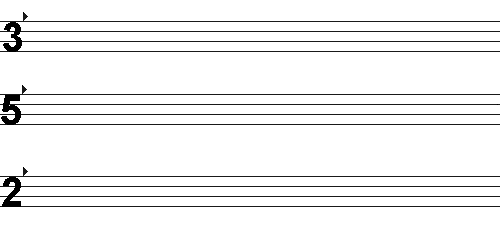
The clefs 1,2,3... refers to the tenths: first, second, third...
So, in the illustrated example above, the first staff (from top) refers to the 3rd tenth (central tenth, corresponding to the octave C3-C4), the second tetragram to the 5th tenth and the third to the 2nd. If we need to write simultaneously on several staves, we will draw normal braces.
Note that you cannot connect staves like in standard notation, because the lines are offset from one decave to the next.
This is a keyboard layout for Armodue, which can also be seen as a standard mavila keyboard layout for 16edo.
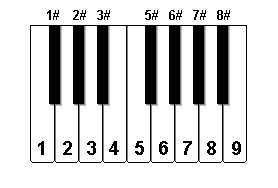
The white keys, corresponding to the notes without accidentals in the notation system, form again a Mavila superdiatonic scale (7L 2s).
